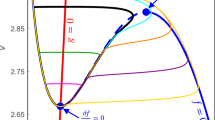
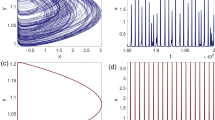
Abstract
Synchronization and desynchronization is of great interest in the study of circadian rhythms, metabolic oscillations and time-dependent cell aggregate behaviors. Several recent studies examine synchronization and other dynamics in models of repressilators coupled by a quorum sensing mechanism that uses a diffusive signal. Their numerical simulations have shown the complexity of the collective behavior depends sensitively on which protein upregulates diffusive signal. In this paper, we rigorously prove that the collective dynamics indeed strongly depends on how the signaling network integrates into the repressilator network. In fact we prove a general result for a class of negative cyclic feedback systems with signaling of which the repressilator is but one example. We show that if the feedback along the signaling loop is also negative, the resulting negative feedback, negative signaling (Nf–Ns) system admits either unique stable equilibrium, or a stable oscillation. When a positive signaling feedback is included, the system is no longer (Nf–Ns) and numerically exhibits multistable dynamics (Ullner et al. in Phys Rev Lett 99:148103, 2007; Phys Rev E 78:031904, 2008). We demonstrate that this multistability emerges through saddle node bifurcations of a sole cubic curve—as in generic bistable models.









Similar content being viewed by others
Notes
Or CI and LacI, respectively.
When the equilibria \(\bar{\mathbf{x}}\) is synchronous, \(S_i=S\) for all i.
Of equilibria and periodic orbits.
References
Buse O, Kuznetsov A, Perez R (2009) Existence of limit cycles in the repressilator equations. Int J Bifurc Chaos 19(12):4097–4106
Buse O, Perez R, Kuznetsov A (2010) Dynamical properties of the repressilator model. Phys Rev E 81:066206
De Monte S, d’Ovidio F, Danø S, Sørensen PG (2007) Dynamical quorum sensing: Population density encoded in cellular dynamics. PNAS 104(47):18377
Dias APS, Rodrigues A (2009) Hopf bifurcation with \(S_N\)-symmetry. Nonlinearity 22:627–666
Ellowitz M, Leibler S (2000) A synthetic oscillatory network of transcription regulators. Nature 403:335–338
Garcia-Ojalvo J, Ellowitz MB, Strogatz SH (2004) Modeling a synthetic multicellular clock: repressilators coupled by quorum sensing. PNAS 101(30):10955–10960
Golubitsky M, Stewart I, Schaeffer D (1988) Singularities and groups in bifurcation theory, Appl. Math. Sci. 69, vol II. Springer, NewYork
Gedeon T, Mischaikow K (1995) Structure of the global attractor of cyclic feedback systems. J. Dyn. Differ. Equ. 7:141–190
Gedeon T (1998) Cyclic feedback systems, vol 637. Memoirs of AMS, Providence
Gonze D (2013) Modeling the effect of cell division on genetic oscillators. J Theor Biol 325:22–33
Koseska A, Ullner E, Volkov E, Garcia-Ojalvo J (2010) Cooperative differentiation through clustering in multicellular populations. J Theor Biol 263:189–202
Levine J, Lin Y, Elowitz M (2013) Functional roles of pulsing in genetic circuits. Science 342:1193–1200
Mallet-Paret J, Smith H (1990) The Poincare–Bendixson theorem for monotone cyclic feedback systems. J Dyn Differ Equ 2:367–421
Muller S, Hofbauer J, Endler L, Flamm C, Widder S, Schuster P (2006) A generalized model of the represillator. J Math Biol 53:905–937
Nandagopal N, Elowitz M (2011) Synthetic biology: integrated gene circuits. Science 333:1244–1248
Pecora L, Carroll T (1998) Master stability functions for synchronized coupled systems. Phys Rev Lett 80(10):2109–2112
Potapov I, Volkov E, Kuznetsov A (2011) Dynamics of coupled repressilators: the role of mRNA kinetics and transcripton cooperativity. Phys Rev E 83:031901
Potapov I, Zhurov BI (2012) Volkov Quorum sensing generated multistability and chaos in a synthetic genetic oscillator. Chaos 22:023117
Stewart I (1996) Symmetry methods in collisionless many-body problems. J Nonlinear Sci 6:543–563
Strelkowa N, Barahona M (2011) Transient dynamics around unstable periodic orbits in the generalized repressilator model. Chaos 21:023104
Thron CD (1991) The secant condition for instability in biochemical feedback control: Parts I and II. Bull Math Biol 53:383–424
Tyson JJ, Othmer HG (1978) The dynamics of feedback control circuits in biochemical pathways. In: Rosen R, Snell FM (eds) Progress in theoretical biology, vol 5. Academic Press, New York
Taylor AF, Tinsley MR, Wang F, Huang Z, Showalter K (2009) Dynamical quorum sensing and synchronization in large populations of chemical oscillators. Science 323(614):614–619
Ullner E, Zaikin A, Volkov E, Garcia-Ojalvo J (2007) Multistability and clustering in a population of synthetic genetic oscillators via phase-repulsive cell-to-cel communication. Phys Rev Lett 99:148103
Ullner E, Koseska A, Kurths J, Volkov E, Kantz H, Garcia-Ojalvo J (2008) Multistability of synthetic genetic networks and repressive cell-to-cell communication. Phys Rev E 78:031904
Yang Y, Kuznetsov A (2009) Characterization and merger of oscillatory mechanisms in an artificial genetic regulatory network. Chaos 19:033115
Zhou T, Zhang J, Yuan Z, Chen L (2008) Synchronization of genetic oscillators. Chaos 18:037126
Acknowledgments
We would like to thank anonymous referees for thoughtful suggestions that substantially improved the paper.
Author information
Authors and Affiliations
Corresponding author
Additional information
We also wish to acknowledge Shuai Zhao for his efforts on this project at its inception.
Rights and permissions
About this article
Cite this article
Gedeon, T., Pernarowski, M. & Wilander, A. Cyclic Feedback Systems with Quorum Sensing Coupling. Bull Math Biol 78, 1291–1317 (2016). https://doi.org/10.1007/s11538-016-0187-8
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11538-016-0187-8