Reaction-Diffusion Patterns in Plant Tip Morphogenesis: Bifurcations on Spherical Caps

Abstract

We study a chemical reaction-diffusion model (the Brusselator) for pattern formation on developing plant tips. A family of spherical cap domains is used to represent tip flattening during development. Applied to conifer embryos, we model the chemical prepatterning underlying cotyledon (“seed leaf”) formation, and demonstrate the dependence of patterns on tip flatness, radius, and precursor concentrations. Parameters for the Brusselator in spherical cap domains can be chosen to give supercritical pitchfork bifurcations of patterned solutions of the nonlinear reaction-diffusion system that correspond to the cotyledon patterns that appear on the flattening tips of conifer embryos.

Appendix: Reduction to the Bifurcation Equation

In this Appendix, we outline the reduction of the Brusselator system (4)–(6) to the bifurcation equation (22),

$$\dot{x}=\sigma_{\max}x+Cx^3, $$

and in particular the calculation of the cubic coefficient C. The calculation is standard, but it seems useful to give some details. For theoretical background, see, e.g., Carr (1981).

First, we choose parameter values so that all the eigenvalues σ of the linearized Brusselator (12)–(13) are real, and the largest eigenvalue σ _max is 0 and corresponds to a normal mode with mode numbers m=m _c and n=n _c . For example, we could use the values (17)–(18), $A=A^{\max}_{\sigma=0}=76.5466$, γ=γ _c =0.5, R=R _c =0.981310, then m _c =5 and n _c =1. We consider only vector functions U=(U(θ,ϕ,t),V(θ,ϕ,t)) that satisfy the homogeneous Dirichlet boundary conditions

$$U(\arcsin\gamma_c,\phi,t)=0,\qquad V(\arcsin\gamma_c,\phi,t)=0, \quad \mbox{for all $0\leq\phi\leq2\pi$, $t>0$}. $$

Due to the symmetries (7), we may simplify our calculations by additionally restricting to functions of spherical polar coordinates (θ,ϕ) that are even in ϕ about ϕ=0. Then we can choose a real normal mode U ⁽⁰⁾ (the real part of a complex normal mode), and both the center eigenspace E ^c and the local center manifold $W_{\mathrm{loc}}^{c}$ become one-dimensional, parameterized by the real variable x.

We solve the linear system

$$ \left ( \begin{array}{cc} -D_X\mu_{m_c,n_c}+k_1 & k_2 \\ k_3 & -D_Y\mu_{m_c,n_c}+k_4 \end{array} \right ) \left ( \begin{array}{c} u^{(0)} \\ v^{(0)} \end{array} \right ) = \left ( \begin{array}{c} 0 \\ 0 \end{array} \right ), $$

finding a specific normal mode

$$ \mathbf{U}^{(0)}= \left ( \begin{array}{c} u^{(0)} \\ v^{(0)} \end{array} \right ) \cos (m_c\phi) \, P^{m_c}_{\lambda_{m_c,n_c}(\gamma_c)}(\cos\theta), $$

(23)

where

$$u^{(0)}=\frac{2k_2}{N^{(0)}}, \qquad v^{(0)}=\frac{2k_5}{N^{(0)}}, \quad k_5=D_X\mu_{m_c,n_c}-k_1, $$

and

$$N^{(0)}=\sqrt{ M\bigl(k_2^2+k_5^2 \bigr)},\qquad M= \int_0^{\arcsin\gamma_c} \bigl[P^{m_c}_{\lambda_{m_c,n_c}(\gamma_c)}(\cos\theta) \bigr]^2\sin\theta \,d\theta. $$

For any two vector functions U _j =(U _j (θ,ϕ,t),V _j (θ,ϕ,t)), j=1, 2, we define their inner product as the integral

$$\begin{aligned} &\langle \mathbf{U}_1,\mathbf{U}_2 \rangle\\ &\quad =\int _0^{2\pi}\int_0^{\arcsin\gamma_c} \bigl[U_1(\theta,\phi,t)U_2(\theta,\phi,t) +V_1(\theta,\phi,t)V_2(\theta,\phi,t)\bigr] \sin\theta \,d\theta\,d\phi. \end{aligned}$$

We solve the adjoint linear system

$$ \left ( \begin{array}{cc} -D_X\mu_{m_c,n_c}+k_1 & k_3 \\ k_2 & -D_Y\mu_{m_c,n_c}+k_4 \end{array} \right ) \left ( \begin{array}{c} u^{(*)} \\ v^{(*)} \end{array} \right ) = \left (\begin{array}{c} 0 \\ 0 \end{array} \right ), $$

and find the adjoint normal mode

$$\mathbf{U}^{(*)}= \left ( \begin{array}{c} u^{(*)} \\ v^{(*)} \end{array} \right ) \cos (m_c \phi) \, P^{m_c}_{\lambda_{m_c,n_c}(\gamma_c)}(\cos\theta) $$

where

$$u^{(*)}=\frac{k_3}{N^{(*)}},\qquad v^{(*)}=\frac{k_5}{N^{(*)}}, $$

and

$$N^{(*)}=2\pi \sqrt{M}\,\frac{k_2k_3+k_5^2}{\sqrt{k_2^2+k_5^2}}. $$

The normalization constant N ^(∗) for the adjoint normal mode is chosen so that

$$\bigl\langle \mathbf{U}^{(0)},\mathbf{U}^{(*)} \bigr\rangle = 1. $$

We can then define the projection P ^c of any vector function U onto the normal mode U ⁽⁰⁾ by

$$\mathbf{P}^c\,\mathbf{U}=x\mathbf{U}^{(0)}, $$

where

$$x=\bigl\langle\mathbf{U},\mathbf{U}^{(*)}\bigr\rangle. $$

We can expand a solution U of (20) near the patternless solution U=0 in a Taylor series in the real variable x about x=0 as

$$\mathbf{U}=x\mathbf{U}^{(0)}+x^2\mathbf{U}^{(1)}+ \mathcal{O}\bigl(x^3\bigr), $$

where terms at second and higher orders in x are orthogonal to U ^(∗). Inserting this Taylor series expansion into the evolution equation (20), using the projection P ^c and collecting coefficients of powers of x, we find at second order in x that U ⁽¹⁾ is the unique solution to the nonhomogeneous linear equation

$$ \mathbf{A}\mathbf{U}^{(1)}= -\bigl(\mathbf{I}-\mathbf{P}^c\bigr)\mathbf{B} \bigl(\mathbf{U}^{(0)},\mathbf{U}^{(0)}\bigr). $$

(24)

At third order in x, we find that the cubic coefficient C in the bifurcation equation (22) is given by

$$ C=2\bigl\langle\mathbf{B}\bigl(\mathbf{U}^{(0)}, \mathbf{U}^{(1)}\bigr),\mathbf{U}^{(*)}\bigr\rangle +\bigl\langle \mathbf{C}\bigl(\mathbf{U}^{(0)},\mathbf{U}^{(0)}, \mathbf{U}^{(0)}\bigr),\mathbf{U}^{(*)}\bigr\rangle. $$

(25)

Substituting the normal mode (23) into the right-hand side of the nonhomogeneous equation (24), we have

$$ \bigl(\mathbf{I}-\mathbf{P}^c\bigr) \mathbf{B}\bigl(\mathbf{U}^{(0)},\mathbf{U}^{(0)}\bigr) = \left ( \begin{array}{c} 1 \\ -1 \end{array} \right ) 2\delta \bigl[\,1+\cos (2m_c\phi)\, \bigr] \, \bigl[P^{m_c}_{\lambda_{m_c,n_c}(\gamma_c)}(\cos\theta) \bigr]^2, $$

(26)

where

$$\delta= \biggl(\frac{bBd}{aA}k_2^2+ \frac{2aAc}{d}k_2k_5 \biggr) \bigl(N^{(0)}\bigr)^{-2}, $$

and then (24) can be solved for U ⁽¹⁾ using infinite normal mode series expansions

$$\begin{aligned} \bigl[P^{m_c}_{\lambda_{m_c,n_c}(\gamma_c)}(\cos\theta) \bigr]^2&= \sum_{n=1}^\infty c_{0,n}P^0_{\lambda_{0,n}(\gamma_c)}(\cos\theta) , \\ \cos (2m_c\phi)\, \bigl[P^{m_c}_{\lambda_{m_c,n_c}(\gamma_c)}(\cos\theta) \bigr]^2&= \cos (2m_c\phi)\sum _{n=1}^\infty c_{2m_c,n}P^{2m_c}_{\lambda_{2m_c,n}(\gamma_c)}( \cos\theta). \end{aligned} $$

In practice, if δ≠0, we approximate the solution U ⁽¹⁾ using truncated, finite normal mode series expansions.

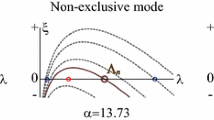
If we fix the parameters D _X , D _Y , a, b, B, and c, and then choose other parameters so that (16), (19), and $A=A^{\max}_{\sigma=0}$ hold, then the coefficient δ in (26) can be expressed as a function of the remaining parameter d. It is easy to show in this case that if

$$d=\frac{1}{4}\,bB, $$

for example, as in the parameter choices (17), then we have

$$\delta=0, $$

and then using (26) the solution to (24) is

$$\mathbf{U}^{(1)}=\mathbf{0}. $$

In this case, the formula (25) for the cubic coefficient C in the bifurcation equation simplifies to

$$C=\bigl\langle\mathbf{C}\bigl(\mathbf{U}^{(0)},\mathbf{U}^{(0)}, \mathbf{U}^{(0)}\bigr),\mathbf{U}^{(*)}\bigr\rangle, $$

and we have

$$ C=6\pi c \,\frac{(k_3-k_5)k_2^2k_5}{N^{(*)} (N^{(0)} )^3}\, \int _0^{\arcsin\gamma_c} \bigl[P^{m_c}_{\lambda_{m_c,n_c}(\gamma_c)}(\cos \theta) \bigr]^4\sin\theta\, d\theta. $$

(27)

Note that many expressions above such as u ⁽⁰⁾, v ⁽⁰⁾, N ⁽⁰⁾, k ₅, M, u ^(∗), v ^(∗), and N ^(∗) depend on parameters, including m _c and n _c , but to simplify notation we have not explicitly shown the dependence. We used Maple to find numerical approximations of roots λ=λ _m,n (γ), associated Legendre functions $P^{m}_{\lambda}(\cos\theta)$, definite integrals, and (when δ≠0) coefficients for truncated, finite normal mode series expansions.