1 Introduction

It is well established now that the higher-dimensional nonlinear wave fields have richer behavior than one-dimensional ones. It was verified that the existence of two solitons having the structures peculiar to a higher-dimensionality may contribute to the variety of the dynamics of nonlinear waves [1,2,3]. Thereby, seeking for exact solution and studying dynamical behavior [4,5,6,7] of solutions are very significant in physics, mathematics, and nonlinear science fields for understanding the complexity and variety of dynamics determined by high-dimensional nonlinear evolution equation [8,9,10]. In soliton theory, the soliton solutions are obtained by the use of the inverse scattering method, Bäcklund transformation, Darboux transformation, Painlevè method, Hirota method, the tanh method, the generalized Riccati equation expansion method, homoclinic test method, etc. [11,12,13,14,15,16,17,18]. In this work, we would like to use the parameter perturbation method for seeking dynamical feature of soliton solution for the \((3+1)\)-dimensional Yu–Toda–Sasa–Fukuyama (YTSF) equation.

The YTSF equation has been presented as

$$ \bigl(-4u_{t}+\varPhi (u)u_{z} \bigr)_{x}+3u_{yy}=0, \qquad \varPhi (u)=\partial _{x}^{2}+4u+2u_{x}\partial _{x}^{-1}, $$
(1)

where \(\partial _{x}^{-1}\) represents the integral with respect to x. This equation was introduced by Yu, Toda, Sasa, and Fukuyama [19] as a generalization from the Bogoyavlenskii–Schif equation [20]. Linearly traveling solitary wave solution for Eq. (1) was found by using tanh-function method [21]. The optimistic quadratic polynomial function lump solutions, some soliton-like solutions, nontravelling wave solutions, and a new kink solution for the potential form of Eq. (1) were obtained by a Backlund transformation, an auto-Backlund transformation, and the extended homoclinic test method [22,23,24,25].

This paper focuses on the exact solutions and spatiotemporal dynamics of solution for Eq. (1). Three types of exact solutions including cross-soliton, breather soliton (periodic solitary solution), and doubly periodic solutions to YTSF equation are constructed by bilinear form and extended homoclinic test approach, a technique of searching for exact homoclinic orbit solution, for nonlinear integrable equation [23, 26]. It is explicitly exhibited that the dynamical feature of the solutions is different on the both sides of an arbitrary constant equilibrium solution (point) of the YTSF equation. Cross-soliton solution is degenerated into periodic solitary wave and breather soliton with different directions and even double periodic solution when the equilibrium point \(u_{0} \) varies from one side of \(-\frac{1}{6}(4 \alpha + cp^{2})\) or \(-\frac{2\alpha - 2cp^{2}}{3c}\) to another side, where α is the propagation velocity of wave, p is a wave number, and c is a fixed constant. To the best of our knowledge, these results have not been studied yet.

2 Cross soliton of YTSF

Using the potential transformation \(u=v_{x}\), a \((3 + 1)\)-dimensional potential YTSF equation has been derived [27, 28]:

$$ -4v_{xt}+v_{xxxz}+4v_{x}v_{xz}+2v_{xx}v_{z}+3v_{yy}=0. $$
(2)

We suppose that \(\eta =x+cz-\alpha t\), then Eq. (2) can be transformed into

$$ 4\alpha v_{ \eta \eta }+c v_{ \eta \eta \eta \eta }+3c \bigl(v_{ \eta } ^{2} \bigr)_{\eta }+3 v_{yy}=0. $$
(3)

By using Painlevé analysis Eq. (3), we suppose

$$ \textstyle\begin{cases} v=u_{0}+2(\ln f)_{\eta }, \\ f=b_{1}e^{-p_{1}(\beta _{1}y+\mu _{1} \eta )}+b_{0}\cos (p_{2}(\beta _{2}y+\mu _{2}\eta ))+b_{2}e^{p_{1}(\beta _{1}y+\mu _{1}\eta )}, \end{cases} $$
(4)

where all of \(p_{1}\), \(p_{2}\), \(\beta _{1}\), \(\beta _{2}\), \(\mu _{1}\), \(\mu _{2}\), \(b _{0}\), \(b_{1}\), \(b_{2}\), \(k_{1}\), \(k_{2}\), and \(k_{3}\) are parameters to be determined later. Substituting Eq. (4) into Eq. (3) and equating all the coefficients of different powers of \(\cos (p_{2}(\beta _{2}y+\mu _{2} \eta ))\), \(\sin (p_{2}(\beta _{2}y+\mu _{2}\eta ))\), \(e^{jp_{1}(\beta _{1}y+ \mu _{1}\eta )}\), \(j=1,2,3,4\) and constant term to zero, we can obtain a set of algebraic equations:

$$ \textstyle\begin{cases} 2c\mu _{1}^{5}p_{1}^{4}-2(10cp_{2}^{2}\mu _{1}^{2}\mu _{2}^{2}-2(3ck _{2}+2\alpha )\mu _{1}^{2}-3\beta _{1}^{2})\mu _{1}p_{1}^{2} \\ \quad {}+2(5cp _{2}^{2}\mu _{1}\mu _{2}^{4}-6(3ck_{2}+2\alpha )\mu _{2}^{2}-3\beta _{2} ^{2})\mu _{1}-6\mu _{2}\beta _{1}\beta _{2})p_{2}^{2}=0, \\ c\mu _{2}^{5}p _{2}^{4}-2(5cp_{1}^{2}\mu _{1}^{2}+3ck_{2}+2\alpha )\mu _{2}^{3}p_{2} ^{2}+6\mu _{1}\beta _{1}\beta _{2}p_{1}^{2} \\ \quad {}+(5cp_{1}^{4}\mu _{1}^{4}+((18ck _{2}+12\alpha )\mu _{1}^{2}+3\beta _{1}^{2})p_{1}^{2}-3p_{2}^{2}\beta _{2}^{2})\mu _{2}=0 , \\ (b_{0}^{2}-\frac{1}{4}b_{1}b_{2})cp_{2}^{4} \beta _{2}^{5}-(\frac{3}{2}(u_{1}^{2}p_{1}^{2}-k_{2})c+\alpha )b_{1}b _{2}+b_{0}^{2}(\frac{3}{2}ck_{2}+\alpha ))p_{2}^{2}\mu _{2}^{3} \\ \quad {}+ \frac{9}{2}b_{1}b_{2}\beta _{1}\beta _{2}\mu _{1}p_{1}^{2}+\frac{3}{4}(3b _{1}b_{2}\mu _{1}^{2}p_{1}^{2}(3c\mu _{1}^{2}p_{1}^{2}+6ck_{2}+4\alpha) \\ \quad {}+b_{1}b_{2}(3\beta _{1}^{2}p_{1}^{2}+\beta _{2}^{2}p_{2}^{2})-b _{0}^{2}\beta _{2}^{2}p_{2}^{2})\mu _{2}=0, \\ 2cb_{1}b_{2}\mu _{1}^{5}p _{1}^{4}+\frac{1}{2}(-cb_{0}^{2}\mu _{2}^{2}p_{2}^{2}+2b_{1}b_{2}(3ck _{2}+2\alpha ))p_{1}^{2}\mu _{1}^{3} \\ \quad {}-\frac{3}{4}\mu _{2}\beta _{1}\beta _{2}b_{0}^{2}p_{2}^{2} \frac{1}{8}(2cb_{0}^{2}\mu _{2}^{2}p_{2}^{2}(4c \mu _{2}^{2}p_{2}^{2}-9ck_{2}-6\alpha ) \\ \quad {}-3b_{0}^{2}\beta _{2}^{2}p_{2} ^{2}+12b_{1}b_{1}\beta _{1}^{2}p_{1}^{2})\mu _{1}=0. \end{cases} $$
(5)

Solving the set of algebraic equations for p, Ω, \(b_{1}\), \(b_{2}\), A yields the exact solution of Eq. (1) as follows:

$$ u=u_{0}+\frac{2cp^{2}[b_{1}^{2}+b_{1}(e^{ip\eta }+e^{-ip\eta })(b_{2}e ^{\varOmega y+\gamma }+e^{-\varOmega y-\gamma })]}{[b_{1}(e^{ip\eta }+e^{-ip \eta }) +(b_{2}e^{\varOmega y+\gamma }+e^{-\varOmega y-\gamma })]^{2}}, $$
(6)

where parameters A, p, Ω, γ, \(b_{1}\), and \(b_{2}\) satisfy the dispersive relations

$$ A=0, \qquad 3\varOmega ^{2}=-cp^{4}-(4\alpha +6u_{0})p^{2}, \qquad b_{1}^{2}= \frac{4\varOmega ^{2}b_{2}}{\varOmega ^{2}-cp^{4}}. $$
(7)

It is obvious that \(u_{0}<-\frac{1}{6}(cp^{2}+4\alpha )\) is required so that the conditions \(\varOmega ^{2}>0\), \(b_{1}^{2}>0\), and \(0< p^{2}<-\frac{4 \alpha +6u_{0}}{c}\) can be satisfied in Eq. (9). Notice that \(u_{0}\) can be taken as an arbitrary real number because the speed of propagating wave α can be arbitrary (only corresponding to the direction and speed propagating wave on the x-axis).

Taking \(\eta =i(x+cz-\alpha t)\) into Eq. (6), the exact solution to the YTSF equation is expressed by

$$ u(x,y,z,t)=\frac{2cp^{2}(b_{1}^{2}+H_{1}H_{2})}{(H_{1}+H_{2})^{2}}, $$
(8)

where

$$ \textstyle\begin{cases} u_{0}< \operatorname{Min}\{-\frac{1}{6}(4\alpha +cp^{2}),-\frac{1}{3}(2\alpha +2cp ^{2})\}, \\ 0< p^{2}< -\frac{4\alpha +6u_{0}}{c}, \\ 3\varOmega ^{2}=-cp ^{4}-(4\alpha +6u_{0})p^{2}, \\ b_{1}^{2}==\frac{4\varOmega ^{2}b_{2}}{ \varOmega ^{2}-cp^{4}}, \\ H_{1}=b_{1}(e^{p(x+cz-\alpha t)}+e^{-p(x+cz- \alpha t)}), \\ H_{2}=b_{2}e^{\varOmega y+\gamma }+e^{-\varOmega y-\gamma}. \end{cases} $$
(9)

Especially, taking \(\gamma =0\), \(b_{2}=1\) in expression Eq. (8) of u, the cross-soliton solution to YTSF is obtained as follows:

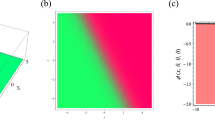
$$ u(x,y,z,t)=\frac{cp^{2}[b_{1}^{2}+4b_{1}\cosh {(p(x+cz-\alpha t))} \cosh {(\varOmega y)}]}{2[b_{1}\cosh {(p(x+cz-\alpha t))} +\cosh {( \varOmega y)}]^{2}}. $$
(10)

The solution represented by Eq. (10) is a cross soliton which contains one soliton and one solitary wave with different propagation direction (see Fig. 1).

Figure 1
figure 1

The cross-soliton solution (10) with \(u_{0}= -0.5\), \(c=-1\), \(\alpha =\frac{1}{4}\), \(p=0.6\), \(t=-1\), \(z=0.1\)

Full size image

3 Periodic soliton of YTSF

Let \(\varOmega =i\varOmega _{1} \) in Eq. (7), where \(\varOmega _{1} \) is a real number, then replacing \(\varOmega _{1} \) with Ω, Eq. (7) changes into

$$ A=0, \qquad 3\varOmega ^{2}=cp^{4}+(4\alpha +6u_{0})p^{2}, \qquad b_{1}^{2}= \frac{4\varOmega ^{2}b_{2}}{\varOmega ^{2}+cp^{4}}. $$
(11)

Here, it is obvious that \(u_{0}>\operatorname{Max}\{-\frac{1}{6}(4\alpha +cp^{2}),- \frac{1}{3}(2\alpha +2cp^{2})\}\) is required so that the conditions \(\varOmega ^{2}>0\) and \(b_{1}^{2}>0\) can be satisfied. Notice that \(u_{0}\) also can be taken as an arbitrary real number by the same argument as in the above cross-soliton case. Taking \(\varOmega =i\varOmega _{1}\) in Eq. (10), replacing \(\varOmega _{1} \) with Ω, we get the solution of Eq. (1) as follows:

$$ u(x,y,z,t)=\frac{cp^{2}[b_{1}^{2}+4b_{1}\cosh {(p(x+cz-\alpha t))} \cos {(\varOmega y)}]}{2[b_{1}\cosh {(p(x+cz-\alpha t))}+\cos {(\varOmega y)}]^{2}}, $$
(12)

where

$$ \textstyle\begin{cases} u_{0}>-\frac{1}{6}(4\alpha +cp^{2}), \\ 3\varOmega ^{2}=cp^{4}+(4 \alpha +6u_{0})p^{2}, \\ b_{1}^{2}=\frac{4\varOmega ^{2}b_{2}}{\varOmega ^{2}+cp^{4}}. \end{cases} $$
(13)

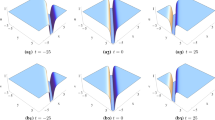
The solution represented by Eq. (12) is a periodic solitary solution which contains one solitary wave and one periodic wave, its amplitude occurs periodically, oscillation varying with variable y (see Fig. 2).

Figure 2
figure 2

The periodic solitary solution (12) with \(u_{0}= \frac{-1}{18}\), \(c=-1\), \(\alpha =\frac{1}{2}\), \(p=\sqrt{\frac{1}{3}}\), \(t=-1\), \(z=0\)

Full size image

It is interesting that \(u_{0}\) plays an important role in the dynamics of cross soliton, cross soliton degenerates into periodic solitary solution when \(u_{0}\) passes through \(-\frac{1}{6}(4\alpha +cp^{2}) \) from the left side to the right side. This shows a kind of bifurcation phenomenon with parameter \(u_{0} \) at the special value \(- \frac{1}{6}(4\alpha +cp^{2}) \).

4 Breather soliton of YTSF

Setting \(p=ip_{1} \) in Eq. (7), where \(p_{1} \) is a real number, then replacing \(p_{1} \) with p, Eq. (7) changes into

$$ A=0,\qquad 3\varOmega ^{2}=-cp^{4}+(4\alpha +6u_{0})p^{2},\qquad b_{1}^{2}= \frac{4 \varOmega ^{2}b_{2}}{\varOmega ^{2}-cp^{4}}. $$
(14)

In this case, \(u_{0}> \frac{1}{6}(cp^{2}-4\alpha ) \) is required so that the conditions \(\varOmega >0 \) and \(b_{1}^{2}>0 \) are satisfied. Taking \(p=ip_{1}\) in Eq. (10) and replacing \(p_{1} \) with p, we get the solution of Eq. (1) as follows:

$$ u(x,y,z,t)=\frac{cp^{2}[b_{1}^{2}+4b_{1}\cos {(p(x+cz-\alpha t))} \cosh {(\varOmega y)}]}{2[b_{1}\cos {(p(x+cz-\alpha t))}+\cosh {(\varOmega y)}]^{2}}, $$
(15)

where

$$ \textstyle\begin{cases} u_{0}> \frac{1}{6}(cp^{2}-4\alpha ), \\ 3\varOmega ^{2}=-cp^{4}+(4 \alpha +6u_{0})p^{2}, \\ b_{1}^{2}=\frac{4\varOmega ^{2}b_{2}}{\varOmega ^{2}-cp ^{4}}. \end{cases} $$
(16)

The solution represented by Eq. (15) is a breather soliton which is a soliton when the trajectory defined by Eq. (15) propagating along the straight line \(x+cz-\alpha t=\mathrm{constant}\), and it also is a periodic wave as \(y=\mathrm{constant} \) (see Fig. 3).

Figure 3
figure 3

The periodic solitary solution (15) with \(u_{0}=0\), \(c=-1\), \(\alpha =\frac{1}{4}\), \(p=0.6\), \(t=-1\), \(z=1\)

Full size image

Combining the above results, we show two important dynamical features of cross soliton, cross soliton degenerates into periodic solitary wave when \(u_{0}\) passes through \(\frac{1}{6}(cp^{2}-4\alpha )\) from the left side to the right side.

5 Doubly periodic solution

Setting \(\varOmega =i\varOmega _{1}\), \(p=ip_{1} \) in Eq. (7) and then replacing \(\varOmega _{1} \) with Ω, \(p_{1}\) with p, Eq. (7) changes into

$$ A=0, \qquad 3\varOmega ^{2}=cp^{4}-(4\alpha +6u_{0})p^{2},\qquad b_{1}^{2}= \frac{4 \varOmega ^{2}b_{2}}{\varOmega ^{2}+cp^{4}}. $$
(17)

Here, \(u_{0}< \frac{1}{6}(cp^{2}-4\alpha ) \) is required so that the conditions \(\varOmega >0 \) and \(b_{1}^{2}>0 \) can be satisfied. Taking \(\varOmega =i\varOmega _{1}\), \(p=ip_{1} \) in Eq. (10) and replacing \(p_{1} \) with p, we get the solution of Eq. (1) as follows:

$$ u(x,y,z,t)=\frac{cp^{2}[b_{1}^{2}+4b_{1}\cos {(p(x+cz-\alpha t))} \cos {(\varOmega y)}]}{2[b_{1}\cos {(p(x+cz-\alpha t))} +\cos {(\varOmega y)}]^{2}}, $$
(18)

where

$$ \textstyle\begin{cases} u_{0}< \frac{1}{6}(cp^{2}-4\alpha ), \\ 3\varOmega ^{2}=cp^{4}+(4\alpha +6u_{0})p^{2}, \\ b_{1}^{2}= \frac{4\varOmega ^{2}b_{2}}{\varOmega ^{2}+cp^{4}}. \end{cases} $$
(19)

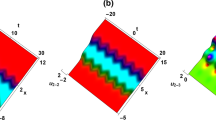
The solution represented by Eq. (18) is a doubly periodic solution. This result shows the breather soliton represented by Eq. (15) degenerated into doubly periodic as \(u_{0}\) passes through \(\frac{1}{6}(cp^{2}-4 \alpha )\) from the right side to the left side. This is also a bifurcation phenomenon of breather soliton with parameter \(u_{0}\) at the special value \(\frac{1}{6}(cp^{2}-4\alpha ) \). This is a new dynamical feature of cross soliton.

By verifying that all the functions represented by Eq. (10), Eq. (12), Eq. (15), and Eq. (18) are the solutions of the YTSF equation under the constraint Eq. (9), Eq. (13), Eq. (16), and Eq. (19), respectively, it is important that the existence of cross-soliton solution Eq. (10), periodic solitary solution Eq. (10), breather soliton Eq. (15), and doubly periodic solution Eq. (18) to YTSF equation is dependant on the different ranges of \(u_{0} \), respectively. If we put one and the same velocity α, p, then the structure of solution is different in a small neighborhood of \(u_{0}= \frac{1}{6}(cp^{2}-4\alpha )\) and \(u_{0}=- \frac{1}{6}(cp^{2}+4\alpha )\), respectively. Cross soliton Eq. (10) changes to periodic solitary solution Eq. (12) when the parameter \(u_{0}\) varies from the left side of \(u_{0}=- \frac{1}{6}(cp^{2}+4 \alpha )\) to the right side, which shows soliton degeneracies of cross soliton of the YTSF equation. And when \(u_{0}>\operatorname{Max}\{ \frac{1}{6}(cp ^{2}-4\alpha ), \frac{1}{6}(cp^{2}+4\alpha )\}\), there occurs coexistence of two kinds of periodic and breather soliton Eq. (12) and Eq. (15). Similarly, the structure of solution is also different in an arbitrary small neighborhood of \(u_{0}= \frac{1}{6}(cp^{2}-4\alpha )\), doubly periodic solution Eq. (18) changes to breather soliton Eq. (15) as \(u_{0}\) varies from the left side of \(u_{0}= \frac{1}{6}(cp^{2}-4 \alpha )\) to the right side. The above results show that the higher dimensional nonlinear evolution equation YTSF has rich dynamics of cross soliton.

6 Concluding

In this paper, we employ the parameter perturbation method for seeking dynamical feature of a general nonlinear partial differential equation. According to the above discussion, we draw the conclusion that for constant equilibrium solution (as a parameter) \(u_{0}\) of the YTSF equation there exist two bifurcation values: one is \(\frac{1}{6}(cp ^{2}-4\alpha )\) and another is \(- \frac{1}{6}(cp^{2}+4\alpha )\), which is the value of soliton degeneracy of cross soliton and retroflexion of breather soliton (light periodic breather changes into dark periodic breather), respectively. Around the both sides at \(u_{0}\), the dynamics of solutions is all changed. The dynamics of cross soliton of the YTSF equation is dependent on the value range of equilibrium solution \(u_{0} \) in the equilibrium solution space of the YTSF equation. This is a new dynamical feature in a nonlinear spatiotemporal dynamical system. In the future, we intend to study other kinds of dynamics for the YTSF equation.