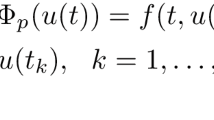Abstract
In this paper, we present a theorem for some impulsive boundary problems with derivative dependence by the upper and lower solutions method. Using the theorem obtained, we consider the existence of positive solutions of some class of singular impulsive boundary problems.
Similar content being viewed by others
1 Introduction
In this paper, we consider the existence of positive solutions of the following nonlinear impulsive differential equations:
where \(\Delta u|_{t=\frac{1}{2}}= u(\frac{1}{2}+)-u(\frac{1}{2}-)\), \(~\Delta u'|_{t=\frac{1}{2}}=u'(\frac{1}{2}+)-u'(\frac{1}{2}-)\), \(p\in (0,1)\), \(q\in (0,1)\), \(\mu \in [0,2]\).
On the one hand, this paper is motivated by [14, 15] in which Ghergu and Rădulescu considered the following elliptic problems:
where \(\Omega \in \mathbb{R}^{N}\) is a bounded domain with smooth boundary and g is singular at \(u=0\), presented the existence of positive solutions via the method of upper and lower solutions.
On the other hand, in recent years, the impulsive differential equation has been studied extensively for its background it being subject to sudden changes in their states, such as population dynamics, biological systems, optimal control, chemotherapeutic treatment in medicine, mechanical systems with impact, financial systems; see [2, 3, 5, 13, 18, 19, 23, 25, 26, 31–33, 36, 39, 42–44, 47, 50, 51] and the references therein. Many of them are on boundary value problems; see [7, 8, 13, 21, 22, 24, 29, 33, 37, 39–43]. But the impulses lead to some interesting phenomena. In fact, it is well known that the following problem:
has the unique solution \(u(t)=\frac{1}{2}t(1-t) \). Now we consider two corresponding impulsive problems
where
and
where
Let
Obviously, \(u_{c}\) is a solution of problem (1.3) for all \(c\in \mathbb{R}\), i.e. problem (1.3) has infinite solutions.
Now we consider the problem (1.4) and assume that problem (1.4) has a solution \(u(t)\). It is easy to see that \(u(t)=c_{0}+c_{1}t+c_{2}t^{2}\) for all \(t\in [0,\frac{1}{2}]\). By \(u(0)=0\) and \(u''(t)=-1\), we have \(u(t)=c_{1}t-\frac{1}{2}t^{2}\) and then \(u(\frac{1}{2})=\frac{1}{2}c_{1}-\frac{1}{8}\) and \(u'(\frac{1}{2}-)=c_{1}-\frac{1}{2}\). Hence, by the definition of \(I_{1}\) and \(N_{1}\), we have \(u(\frac{1}{2}+)=u(\frac{1}{2})+I (u (\frac{1}{2} ) )=-\frac{1}{4}\) and \(u'(\frac{1}{2}+)=u'(\frac{1}{2}-)-u'(\frac{1}{2}-)=0\). From \(-u''(t)\equiv 1\) for all \(t\in (\frac{1}{2},1]\), we have \(u(t)=-\frac{3}{8}+\frac{1}{2}t-\frac{1}{2}t^{2}\). This contradicts \(u(1)=0\). This means that problem (1.4) has no solution.
The above examples show that it is interesting to consider the existence of solutions for differential equations with impulses. As is well known, the methods to be used for the existence of solutions for boundary value problems mainly include bifurcation theory (see [28, 34, 46]), method of lower and upper solutions (see [10, 11, 14, 15]), topological degree theory (see [16, 20, 48, 49, 53, 54]) and variational methods (see [45]). But the impulses lead to some difficulties in the use of the method of lower and upper solutions for impulsive boundary value problems and some authors worked hard at proving some new theorems for the method of lower and upper solutions. For example, Erbe and Liu [13] considered the following problem:
and using the one-side Lipschitz condition
they got the extremal solutions of problem (1.5) with the help of lower and upper solutions; in [39], under the condition that f has time singularities at \(t =0\) and \(t =1\), the authors proved the existence of a solution to this problem under the assumption that there exist lower and upper functions associated with the problem; in [23], Lee and Liu established the method of lower and upper solutions for the following problem:
where f is singular at \(t=0\) and \(t=1\). There are other references for the methods of lower and upper solutions for impulsive differential equations; see [1, 21, 22, 27, 38].
We note that in problem (1.1) the nonlinearity f is dependent on \(u'\) and is singular at \(u=0\), and the impulsive function \(N(u,v)\) is dependent on \(u'\). And so we cannot use the method of lower and upper solutions for problem (1.5) and problem (1.6) to study problem (1.1) directly. Since problem (1.1) is a special case the following problem:
and
if the method of lower and upper solutions for problem (1.7)–(1.8) can be obtained, by constructing suitable lower and upper solutions, we can get the existence of solutions of problem (1.1).
The paper is organized as follows. In Sect. 2, we present a theorem for the upper and lower solutions method for problem (2.1)–(2.2) which is a generalization of problem (1.7)–(1.8). In Sect. 3, we get the existence of positive solutions of problem (1.1) when \(K(t)<0\) on \(t\in [0,1]\). In Sect. 4, we prove the existence of positive solutions of problem (1.1) when \(K(t)>0\) on \(t\in [0,1]\). Some ideas of our proofs come from [12, 14, 15, 30, 35] and [48].
2 The method of lower and upper solutions for impulsive differential equations
To obtain the lower and upper solutions for problem (1.7)–(1.8), we consider the generalization case such as
and
The solution is defined as \(u\in PC^{1}([0,1],\mathbb{R})\cap PC^{2}((0,1),\mathbb{R})\) satisfying (2.1)–(2.2), where \(PC([0,1],\mathbb{R})=\{u:[0,1]\to \mathbb{R}:u(t)\text{ is continuous at } t\neq t_{1}, u(t_{1}-0)=\lim_{t\to t_{1}^{-}}u(t)\mbox{ and } u(t_{1}+0)=\lim_{t\to t_{1}^{+}}u(t)\mbox{ exist}\}\) (denoted by \(PC[0,1]\) simply), \(PC^{1}([0,1], \mathbb{R})=\{u\in PC[0,1]| u'(t)\mbox{ is continuous on } [0,t_{1})\cup (t_{1},1], \lim_{t\to t_{1}^{-}}u'(t)\mbox{ and } \lim_{t\to t_{1}^{+}}u'(t)\mbox{ exist}\}\) with the norm \(\|u\|_{1}=\max \{\sup_{t\in [0,1]}|u(t)|,\sup_{t\in [0,1]}|u'(t)| \}\) (denoted by \(PC^{1}[0,1]\) simply), and \(PC^{2}((0,1), \mathbb{R})=\{u\in PC([0,1],\mathbb{R})|u''(t)\mbox{ is continuous on }(0,t_{1})\cup (t_{1},1)\mbox{ and } \lim_{t\to t_{1}^{-}}u''(t), \lim_{t\to t_{1}^{+}}u''(t)\mbox{ exist}\}\) (denoted by \(PC^{2}(0,1)\) simply).
If \(\alpha \in PC^{1}[0,1]\cap PC^{2}(0,1)\) satisfies
it is a lower solution of problem (2.1)–(2.2), while the upper solution is defined as \(\beta \in PC^{1}[0,1]\cap PC^{2}(0,1)\) satisfying
Denote
where α, \(\beta \in PC^{1}([0,1],\mathbb{R})\) with \(\alpha (t)\leq \beta (t)\) for all \(t\in [0,1]\). Then we give the lower and upper solution theorem of problem (2.1)–(2.2).
Now we list a condition for convenience:
\((A_{0})\)\(I\in C(\mathbb{R},\mathbb{R})\) and \(u+I(u)\) is nondecreasing.
To establish a fundamental theorem of the upper and lower solution method for problem (2.1)–(2.2), we need to use a corresponding theorem for the following singular boundary value problem:
The definitions of upper and lower solutions of problem (2.3) can be found in [11, 39]. Now we list the following lemma [45].
Lemma 2.1
(see [45])
Let \(u\in H^{2,1}[0,1]\)and \(M>0\)be a constant such that \(|u^{\prime \prime }(t)|\leq K|u'(t)|^{2}+f_{0}(t)\)with \(K>0\), \(f_{0}\in L^{1}[0,1]\)and \(\sup_{t\in [0,1]}|u(t)|\leq M\). Then there exists a constant \(k_{0}>0\)such that
Lemma 2.2
Assume that the function \(f(t, u, v)\)is continuous in \((0,1)\times (0,+\infty )\times \mathbb{R}\). Furthermore, suppose that problem (2.3) has a pair of upper and lower solutions \(\beta (t)\)and \(\alpha (t) \)satisfying the conditions
-
(1)
α, \(\beta \in C^{1}[0,1]\cap C^{2}(0,1)\);
-
(2)
\(0<\alpha (t)\leq \beta (t)\), \(\forall t\in (0,1)\);
-
(3)
there exist a nonnegative function \(h\in L^{1}[0,1]\)and a positive constant \(K>0\)such that
$$ \bigl\vert f(t,u,v) \bigr\vert \leq h(t)+K \vert v \vert ^{2},\quad \forall (t,u)\in D^{\beta }_{\alpha }, v\in \mathbb{R}.$$(2.4)Then problem (2.3) has a solution \(u(t)\)belonging to \(C^{1}[0,1]\)and satisfying
$$ \alpha (t)\leq u(t)\leq \beta (t),\quad t\in [0,1]. $$
Proof
Let \(\overline{f}:(0,1)\times \mathbb{R}\times \mathbb{R}\) be defined by
Now we consider the following problem:
First, we show that the solution of problem (2.5) is the solution of problem (2.3).
Now we prove that \(\alpha (t)\leq u(t)\leq \beta (t)\), \(t\in (0,1)\). In fact, if there is a \(t_{0}\in (0,1)\) with \(u(t_{0})<\alpha (t_{0})\), let \(t_{*}=\inf \{t< t_{0}|u(s)<\alpha (s), \forall s\in [t,t_{0}]\}\) and \(t^{*}=\sup \{t>t_{0}|u(s)<\alpha (s), \forall s\in [t_{0},t]\}\). Since \(\alpha (0)\leq a=u(0)\), \(\alpha (1)\leq b=u(1)\), we have \(u(t)<\alpha (t)\), \(\forall t\in (t_{*},t^{*})\) and \(u(t_{*})=\alpha (t_{*})\) and \(u(t^{*})=\alpha (t^{*})\). Suppose that \(t'\in (t_{*},t^{*})\) satisfies \(u(t')-\alpha (t')=\min_{t\in [t_{*},t^{*}]}(u(t)-\alpha (t))<0\). Then \(\alpha '(t')=u'(t')\). Hence,
This is a contradiction. Thus, \(0<\alpha (t)\leq u(t)\) for all \(t\in [0,1]\).
A same argument shows that \(u(t)\leq \beta (t)\) for all \(t\in [0,1]\).
Hence, \(\forall t\in (0,1)\), we have \(\alpha (t)\leq u(t)\leq \beta (t)\), which
that is, \(u(t)\) is a solution of problem (2.3).
Next, we show that problem (2.5) has at least one solution.
It follows from \(h\in L[0,1]\), (2.4) and the definition of f̅ that
Hence, for \(u\in C^{1}[0,1]\), the A can be defined well as
where \(\psi (t)=a+t(b-a)\) and
It is easy to see that the fixed point of A is the solution of problem (2.5). Moreover, since
and f̅ is continuous, the Arzela–Ascoli theorem and the dominated convergence theorem guarantee that \(A:C^{1}[0,1]\to C^{1}[0,1] \) is compact.
For \(u\in C^{1}[0,1]\) and \(\lambda \in (0,1)\), if \(u=\lambda Au\), we claim that
(1) Suppose that there exists a \(t_{1}\in (0,1)\) such that \(u(t_{1})<0\). Then there exists a \(t'_{1}\in (0,1)\) such that \(u'(t'_{1})=\min_{t\in [0,1]}u(t)<0\) and \(u'(t'_{1})=0\), which together with \(u=\lambda Au\) implies that
This is a contradiction.
(2) Suppose that there exists a \(t_{2}\in (0,1)\) such that \(u(t_{2})>2\max_{t\in [0,1]}\beta (t)\). Then there exists a \(t'_{2}\in (0,1)\) such that \(u'(t'_{2})=\max_{t\in [0,1]}u(t)>2\max_{t\in [0,1]}\beta (t)\) and \(u'(t'_{2})=0\), which together with \(u=\lambda Au\) implies that
This is a contradiction also.
Then, if \(u=\lambda Au\), from (2.6) and
Lemma 2.1 means that there is a \(k_{0}>0\) such that \(|u|_{H^{2,1}[0,1]}\leq k_{0}\). The interpolation inequality lemma guarantees that there exists an \(R>0\) such that
The Leray–Schauder fixed point theorem guarantees that A has at least one fixed point u in \(C^{1}[0,1]\).
Consequently, problem (2.3) has at least one solution \(u\in C^{1}[0,1]\) such that \(\alpha (t)\leq u(t)\leq \beta (t)\) for all \(t\in [0,1]\). □
Remark 2.3
The idea of this lemma comes from [4, 6, 9, 10, 17, 52].
Remark 2.4
If \(f(t,u,v)=u^{-\frac{1}{3}}+u^{2}+v^{2}\), let \(\alpha (t)=\sin \pi t\), \(t\in [0,1]\) and \(\beta (t)\equiv 2\), \(t\in [0,1]\) and \(D_{\alpha }^{\beta }=\{(t,u)\in (0,1)\times \mathbb{R}| \alpha (t) \leq u\leq \beta (t)\}\). Then
Obviously, \(h\in L^{1}[0,1]\). Hence, (2.4) is true.
Lemma 2.5
Suppose that α, \(\beta \in PC^{1}[0, 1]\cap PC^{2}(0, 1)\)are, respectively, lower and upper solutions for problem (2.1)–(2.2) with \(\alpha (t)\leq \beta (t)\)and \(\alpha (t_{1})=\beta (t_{1})\). Moreover, u is a solution of problem (2.1) such that \(\alpha (t)\leq u(t)\leq \beta (t)\)for all \(t\in [0,1]\). Then u satisfies (2.2), i.e. \(u(t)\)is a solution of problem (2.1)–(2.2) such that \(\alpha (t)\leq u(t)\leq \beta (t)\)on \([0, 1]\).
Proof
Since \(\alpha (t_{1})=\beta (t_{1})\) and \(\alpha (t)\leq u(t)\leq \beta (t)\) for all \(t\in [0,1]\), we have \(u(t_{1})=\alpha (t_{1})=\beta (t_{1})\) and \(\alpha '(t_{1}-)\geq u'(t_{1}-)\), \(\alpha '(t_{1}+)\leq u'(t_{1}+)\) and \(\beta '(t_{1}-)\leq u'(t_{1}-)\), \(\beta '(t_{1}+)\geq u'(t_{1}+)\). Thus,
and
that is, \(u(t)\) satisfies (2.2). Consequently, \(u(t)\) is a solution of problem (2.1)–(2.2). □
Theorem 2.6
Assume \((A_{0})\) and also assume
-
(1)
\(f(t,u,v)\)is continuous in u, \(v\in \mathbb{R}\), \(t\neq t_{1}\). Furthermore, \(\lim_{t\to t_{1}-} f(t,u, v)=f(t_{1},u,v)\)and \(\lim_{t\to t_{1}+}f(t,u,v)\)exists.
-
(2)
\(N(u,v)\in C(\mathbb{R}\times \mathbb{R},\mathbb{R})\)is nondecreasing in v.
-
(3)
α and β are, respectively, lower and upper solutions of problem (2.1)–(2.2) such that \(\alpha (t)\leq \beta (t)\)for all \(t\in [0, 1]\).
-
(4)
There exists a function \(h\in L^{1}[0,1]\)such that \(|f(t,u,v)| \leq h(t)+K|v|^{2}\)for all \((t, u)\in D_{\alpha }^{\beta }\)and \(v\in \mathbb{R}\).
Then problem (2.1)–(2.2) has at least one solution u such that \(\alpha (t)\leq u(t)\leq \beta (t)\)for all \(t\in [0,1]\).
Proof
Let \(C\in [\alpha (t_{1}),\beta (t_{1})]\). Condition (3) implies that α and β are, respectively, lower and upper solutions of the following problem:
Thus by Lemma 2.2, problem (2.7) has a solution \(v\in C^{1}[0,t_{1}]\cap C^{2}(0,t_{1})\) satisfying
By \((A_{0})\), we get
Define
and
It is easy to see that α̃ and β̃ are lower and upper solutions of the following problem:
Thus again by Lemma 2.2, problem (2.9) has a solution \(w\in C^{1}[t_{1}, 1]\cap C^{2}(t_{1}, 1)\) satisfying
Define
which guarantees that
From (2.8)–(2.10), u is a solution of (2.1) satisfying \(u(t_{1})=C\) and \(\alpha (t)\leq u(t)\leq \beta (t)\) for all \(t\in [0,1]\).
Consequently, for each \(C\in [\alpha (t_{1}),\beta (t_{1})]\), there exists a solution \(u_{C}\) of problem (2.1) satisfying \(u_{C}(t_{1})=C\).
We now show that one of the solutions \(u_{C}\) satisfies condition (2.2). Let \(X(C) =\{u| u\mbox{ is a solution of problem (2.1) such that }u(t_{1})=C\mbox{ and }\alpha (t)\leq u(t)\leq \beta (t)\mbox{ for all } t\in [0, 1]\}\). Then, for each \(C\in [\alpha (t_{1}),\beta (t_{1})]\), \(X(C)\neq\emptyset \). There are two cases: (A) \(\alpha (t_{1})=\beta (t_{1})=C\); (B) \(\alpha (t_{1})<\beta (t_{1})\).
First we consider the case (A). Since \(\alpha (t_{1})=\beta (t_{1})=C\), by Lemma 2.3, \(u_{C}(t)\) is a solution of problem (2.1)–(2.2).
Now we consider the case (B). Since \(\alpha (t_{1})<\beta (t_{1})\), we define \(S=\{\overline{C}\in [\alpha (t_{1}), \beta (t_{1})): C\in ( \overline{C},\beta (t_{1}))\mbox{ implies } \Delta u'_{C}|_{t=t_{1}}< N(u_{C}(t_{1}), u'_{C}(t_{1}-)),\mbox{ for all }u_{C}\in X(C)\}\). There are two cases also: (\(B_{1}\)) \(S\neq\emptyset \); (\(B_{2}\)) \(S=\emptyset \).
Now we consider the case (\(B_{1}\)). Let \(C^{*}=\inf S\), then \(\alpha (t_{1})\leq C^{*}<\beta (t_{1})\).
If \(C^{*}>\alpha (t_{1})\), by the definition of \(C^{*}\), we can choose sequences \(C_{n}\in (\alpha (t_{1}), C^{*})\) and \(u_{C_{n}}\in X(C_{n})\) such that \(C_{n}\to C^{*}\) and
Obviously \(u_{C_{n}}(t)\) satisfies
and
where \(G_{1}(t, s)\) and \(G_{2}(t, s)\) are the Green’s functions of linear homogeneous problem with Dirichlet boundary condition corresponding to (2.7) and (2.9). From \(\alpha (t)\leq u_{C_{n}}(t)\leq \beta (t)\), \(t\in [0,1]\), Lemma 2.1 together with condition (4) of Theorem 2.6 guarantees that there is a \(k_{0}>0\) such that
Now the Embedding theorem implies that there is an \(R>0\) such that \(|u'_{C_{n}}(t)|\leq R\), \(\forall t\in [0,1]\). Condition (4) implies that
also. Since \(h\in L^{1}[0,1]\), the functions belonging to \(\{u_{C_{n}}(t)\}\) and the functions belonging to \(\{u'_{C_{n}}(t)\}\) are equicontinuous on \([0,t_{1}]\) and \((t_{1},1]\), respectively.
Therefore, without loss of generality, we assume that
Letting \(n\to +\infty \) in (2.12) and (2.13), we have
and
Obviously, \(u_{0}\) is a solution of problem (2.1) satisfying \(u_{0}(t_{1})=C^{*}\) and \(\alpha (t)\leq u_{1}(t)\leq \beta (t)\) on \([0,1]\). And from (2.12) and (2.13), we get
Letting \(n\to +\infty \), we have
Letting \(n\to +\infty \) in (2.11), we obtain
Equations (2.14) and (2.15) indicate
which implies that \(u_{0}\) is a lower solution of problem (2.1)–(2.2) satisfying \(u_{0}(t_{1})=C^{*}\).
By the definition of \(C^{*}\), for \(D_{n}\in [C^{*},\beta (t_{1})]\) with \(D_{n}\to C^{*}\) as \(n\to +\infty \), we have
and
By a similar argument to the construction of \(u_{0}\), we obtain \(u_{1}\), a solution of problem (2.1) satisfying \(u_{1}(t_{1})=C^{*}\), \(u_{0}(t)\leq u_{1}(t)\leq \beta (t)\) on \([0,1]\), \(u_{D_{n}}(t)\rightrightarrows u_{1}(t)\), \(u'_{D_{n}}(t)\rightrightarrows u'_{1}(t)\) as a subsequence if necessary and
Thus \(u_{1}\) is an upper solution of problem (2.1)–(2.2).
Consequently, \(u_{0}\) and \(u_{1}\) are lower and upper solutions of problem (2.1)–(2.2), respectively, satisfying \(u_{0}(t_{1})= C^{*}=u_{1}(t_{1})\) and \(u_{0}(t)\leq u_{1}(t)\) on \([0,1]\).
Therefore, by the argument of (A), problem (2.1)–(2.2) has at least one solution between \(u_{0}\) and \(u_{1}\).
If \(C^{*}=\alpha (t_{1})\), by the definition of \(C^{*}\), we can choose sequences \(C_{n}\in (\alpha (t_{1}), \beta (t_{1}))\) and \(u_{C_{n}}\in X(C_{n})\) such that \(C_{n}\to \alpha (t_{1})+\) as \(n\to +\infty \) and
By the same argument as for \(u_{1}\) and \(\{u_{D_{n}}\}\), there is a \(u_{2}\), a solution of problem (2.1) satisfying \(u_{2}(t_{1})=\alpha (t_{1})\), \(\alpha (t)\leq u_{2}(t)\leq \beta (t)\) on \([0,1]\), and \(u_{C_{n}}(t)\rightrightarrows u_{2}(t)\), \(u'_{C_{n}}(t)\rightrightarrows u'_{2}(t)\) as a subsequence if necessary and
Consequently, α and \(u_{2}\) are lower and upper solutions of problem (2.1)–(2.2), respectively, satisfying \(\alpha (t_{1})=C^{*}=u_{2}(t_{1})\) and \(\alpha (t)\leq u_{2}(t)\) on \([0,1]\).
Therefore, by the argument of (A), problem (2.1)–(2.2) has at least one solution between α and \(u_{2}\).
The above proof shows that problem (2.1)–(2.2) has at least one solution if \(S\neq\emptyset \).
Next, we consider (\(B_{2}\)). Since \(S=\emptyset \), we may choose sequences \(C_{n}\in (\alpha (t_{1}),\beta (t_{1}))\) and \(u_{C_{n}}\in X(C_{n})\) satisfying \(C_{n}\to \beta (t_{1})\) as \(n\to +\infty \) and \(\Delta u'_{C_{n}}|_{t=t_{1}}\geq N(u_{C_{n}}(t_{1}), u'_{C_{n}}(t_{1}-))\).
By a similar limit argument to the one before, we obtain \(u_{3}\), a solution of problem (2.1) satisfying \(u_{3}(t_{1})=\beta (t_{1})\), \(\alpha (t)\leq u_{3}(t)\leq \beta (t)\), \(t\in [0, 1]\), \(u_{C_{n}}(t)\rightrightarrows u_{3}(t)\) and \(u'_{C_{n}}(t)\rightrightarrows u'_{3}(t)\) as a subsequence if necessary and
Therefore, \(u_{3}\) and β are lower and upper solutions of problem (2.1)–(2.2). By the proof of (A) also, problem (2.1)–(2.2) has at least one solution between \(u_{3}\) and β.
Consequently, problem (2.1)–(2.2) has at least one solution if \(S=\emptyset \).
The proof is completed. □
Remark 2.7
The idea of our theorem comes from [23].
Remark 2.8
In the problem (1.1), let \(f(t,u,v)=\lambda u^{p}-K(t)u^{-q}-|v|^{\mu }\). Since \(\mu \in [0,2]\), there is a \(C'>0\) such that
In Sects. 3 and 4, we will obtain the positive solutions of problem (1.1) by constructing a pair of lower and upper solutions which satisfy Theorem 2.6.
Throughout the paper, we always assume that \(\mu \in [0,2]\), \(p\in (0,1)\), \(q\in (0,1)\).
3 The positive solutions for problem (1.1) when \(K(t)<0\) on \(t\in [0,1]\)
In this section, we consider existence of positive solutions for problem (1.1) when \(K(t)<0\) on \(t\in [0,1]\). We have the following theorem.
Theorem 3.1
Assume \(K\in C[0,1]\)with \(K(t)<0\)for all \(t\in [0,1]\)and the following conditions hold:
-
(1)
\(I\in C(\mathbb{R},\mathbb{R})\)and \(u+I(u)\)is nondecreasing, \(I(0)=0\)and \(I(u)\geq 0\)for all \(u\in [0,+\infty )\);
-
(2)
\(N(u,v)\in C(\mathbb{R}\times \mathbb{R},\mathbb{R})\)is nondecreasing in v, \(N(0,0)<0\)and there is a \(m_{0}>0\)such that \(N(u,0)>0\)for all \(u\geq m_{0}\).
Then problem (1.1) has at least one positive solution u for all \(\lambda >0\).
Proof
The proof is divided into three steps.
Step 1. We construct a lower positive solution of problem (1.1).
Since \(N(0,0)<0\), there exists a \(\varepsilon _{0}>0\) such that
From \(p\in (0,1)\), for fixed \(\lambda >0\), there exists a positive constant \(\varepsilon _{1}\) such that
Moreover, since \(K(t)<0\) for all \(t\in [0,1]\), there is a \(\varepsilon _{2}>0\) such that
Choose a positive constant \(\varepsilon <\min \{\varepsilon _{0},\varepsilon _{1},\varepsilon _{2} \}\). Since \(I(0)=0\), \(I(u)+u\) is increasing on \([0,+\infty )\) and \(\lim_{u\to +\infty }(I(u)+u)=+\infty \), there exists a unique \(0<\varepsilon '<\varepsilon \) such that
Let
and
From (3.2), we have
and
Combining (3.4), (3.5), (3.6) and (3.7), we have
i.e. \(\underline{u}\) is a lower solution of problem (1.1).
Step 2. We construct an upper positive solution of problem (1.1).
Choose a positive constant \(C>\sup_{t\in [0,1]}\underline{u}(t)\). Since \(p\in (0,1)\), \(q\in (0,1)\), there exists \(k_{0}>\max \{ \varepsilon _{0},\varepsilon _{1},\varepsilon _{2}\}\) such that
Let \(k>\max \{m_{0},k_{0}\}\) and \(k'\) satisfy
Since \(I(u)\geq 0\) for all \(u\in [0,+\infty )\), we have \(k'\geq k\). Define
Now (3.10) implies that
Since \(k+C>m_{0}\) (note \(N(k+C,0)>0\)), we have
From (3.9), we have
and
Combining (3.11), (3.12), (3.13) and (3.14), we have
i.e. u̅ is an upper solution of problem (1.1).
Step 3. We claim that problem (1.1) has at least one positive solution.
Let
and
Then \(h\in L^{1}[0,1]\) and from (2.18), we have
By Theorem 2.6, combining (3.8), (3.15) and (3.16), problem (1.1) has at least one positive solution u with \(\underline{u}(t)\leq u(t)\leq \overline{u}(t)\) for all \(t\in [0,1]\).
The proof is completed. □
Remark 3.2
Let \(I(u)=u^{2}\) and \(N(u,v)=-1+u+v\). Obviously, I and N satisfy the conditions of Theorem 3.1.
4 The positive solutions for problem (1.1) when \(K(t)>0\) on \(t\in [0,1]\)
In this section, we consider the existence of positive solutions for problem (1.1) when \(K(t)>0\) on \(t\in [0,1]\).
Theorem 4.1
Assume \(K\in C[0,1]\)with \(K(t)>0\)for all \(t\in [0,1]\)and the following conditions hold:
-
(1)
\(I\in C(\mathbb{R},\mathbb{R})\)and \(u+I(u)\)is nondecreasing and \(\lim_{u\to +\infty }I(u)=+\infty \);
-
(2)
\(N(u,v)\in C(\mathbb{R}\times \mathbb{R},\mathbb{R})\)is nondecreasing in v, \(N(u,0)\geq 0\)for all \(u\in [0,+\infty )\)and \(\lim_{u\to +\infty }\frac{N(u,0)}{u}=0\).
Then there is a \(\lambda ^{*}>0\)such that problem (1.1) has at least one positive solution u for all \(\lambda \geq \lambda ^{*}\).
Proof
The proof is divided into three steps.
Step 1. We construct a lower positive solution of problem (1.1).
Since \(|\cos \pi t|\geq \frac{\sqrt{2}}{2}\) for all \(t\in [0,\frac{1}{4}]\cup [\frac{3}{4},1]\), \(N(u,0)\geq 0\) for all \(u\in [0,+\infty )\) and \(\lim_{u\to +\infty }I(u)=+\infty \), \(\lim_{u\to +\infty }\frac{N(u,0)}{u}=0\), there is a \(k_{1}>0\) big enough such that
and
Let \(\gamma (t)\) be a smooth function on \([\frac{1}{3},1]\) such that \(\gamma (t)=t-\frac{1}{3}\) if \(t\in [\frac{1}{3},\frac{2}{3}]\) and \(\gamma (t)=1-t\) if \(t\in [\frac{5}{6},1]\), and \(\gamma (t)>0\) for all \(t\in [\frac{2}{3},\frac{5}{6}]\). Define
Then, for \(t\in [0,\frac{1}{2}]\), we have
and, for \(t\in (\frac{1}{2},1]\), we have
Choose \(0<\delta <\frac{1}{6}\) small enough such that
and (note \(\gamma ''(t)=0\) for all \(t\in [1-\delta ,1)\))
Since \(\min_{t\in [0,\delta ]\cup [1-\delta ,1]}|\cos \pi t|\geq \min_{t \in [0,\frac{1}{4}]\cup [\frac{3}{4},1]}|\cos \pi t|\) for all \(\delta \in (0,\frac{1}{6})\), combining (4.1), (4.5) and (4.6), we have
and
And then, combining (4.3), (4.4), (4.7) and (4.8), there is a \(\lambda _{1}>0\) such that for all \(\lambda \geq \lambda _{1}\)
Since \(\min_{t\in [\delta ,1-\delta ]}\sin \pi t>0\), we can choose \(\lambda _{2}\) big enough such that, for \(\lambda \geq \lambda _{2}\),
Let \(\lambda ^{*}=\max \{\lambda _{1},\lambda _{2}\}\). Combining (4.9) and (4.10), we have
By the definition of \(\underline{u}\) and (4.2), we have
and (note (4.2))
From (4.11), (4.12) and (4.13), we have
i.e. \(\underline{u}\) is a lower solution of problem (1.1).
Step 2. We construct an upper solution of problem (1.1).
Since \(p\in (0,1)\), for \(\lambda \geq \lambda ^{*}\), we can choose \(k_{2}>k_{1}\) such that
Let
From (4.15), we have
and
By the definition of u̅ and \(N(u,0)\geq 0\) for all \(u\geq 0\), we have
and
Combining (4.16), (4.17), (4.18) and (4.19), we have
which implies that u̅ is a upper solution of problem (1.1).
Step 3. We claim that problem (1.1) has at least one positive solution.
Let
and
where \(C'\) is defined in (2.18). Then \(h\in L^{1}[0,1]\) and
From (4.14), (4.20) and (4.21), Theorem 2.6 guarantees that problem (1.1) has at least one positive solution u with \(\underline{u}(t)\leq u(t)\leq \overline{u}(t)\) for all \(t\in [0,1]\).
The proof is completed. □
Remark 4.2
Let \(I(u)=u^{2}\) and \(N(u,v)=|u|^{\frac{1}{2}}+v\). Obviously, I and N satisfy the conditions of Theorem 2.6.
References
Agarwal, R., Franco, D., O’Regan, D.: Singular boundary value problems for first and second order impulsive differential equations. Aequ. Math. 69, 83–96 (2005)
Agarwal, R.P., O’Regan, D.: Multiple nonnegative solutions for second-order impulsive differential equations. Appl. Math. Comput. 114, 51–59 (2000)
Ahmad, B.: Existence of solutions for second-order nonlinear impulsive boundary-value problems. Electron. J. Differ. Equ. 2009, 68 (2009)
Amann, H.: Existence and multiplicity theorems for semilinear elliptic boundary value problems. Math. Z. 150, 567–597 (1976)
Bainov, D.D., Simeonov, P.S.: Impulsive Differential Equations: Periodic Solutions and Applications. Longman Scientific and Technical, Essex (1993)
Bobisud, L.E., O’Regan, D.: Positive solutions for a class of nonlinear singular boundary value problems at resonance. J. Math. Anal. Appl. 184(2), 263–284 (1994)
Cabada, A., Liz, E.: Boundary value problems for higher order ordinary differential equations with impulses. Nonlinear Anal. 32, 775–786 (1998)
Cabada, A., Nieto, J.: A generalization of the monotone method for second order periodic boundary value problem with impulses at fixed points. Dyn. Contin. Discrete Impuls. Syst. 7, 145–158 (2000)
Cui, S.: Existence and nonexistence of positive solutions for singular semilinear elliptic boundary value problems. Nonlinear Anal. 41, 149–176 (2000)
De Coster, C.: Existence and localization of solution for second order elliptic BVP in presence of lower and upper solutions without any order. J. Differ. Equ. 145, 420–452 (1998)
De Coster, C., Habets, P.: The lower and upper solutions method for boundary value problems. In: Handbook of Differential Equations: Ordinary Differential Equations, vol. 1, pp. 69–160 (2004)
Di, K., Yan, B.: The existence of positive solution for singular Kirchhoff equation with two parameters. Bound. Value Probl. 2019, 40 (2019)
Erbe, L.H., Liu, X.: Existence results for boundary value problems of second order impulsive differential equations. J. Math. Anal. Appl. 149, 56–69 (1990)
Ghergu, M., Rădulescu, V.: Sublinear singular elliptic problems with two parameters. J. Differ. Equ. 195(2), 520–536 (2003)
Ghergu, M., Rădulescu, V.: On a class of sublinear singular elliptic problems with convection term. J. Math. Anal. Appl. 311, 635–646 (2005)
Guo, D., Lakshmikantham, V., Liu, X.: Nonlinear Integral Equations in Abstract Spaces. Kluwer Academic, Norwell (1996)
Habets, P., Zanolin, F.: Lower and upper solutions for a generalized Emden-Fowler equation. J. Math. Anal. Appl. 181(3), 684–700 (1994)
Lakmeche, A., Boucherif, A.: Boundary value problems for impulsive second order differential equations. Dyn. Contin. Discrete Impuls. Syst., Ser. A Math. Anal. 9, 313–319 (2000)
Lakshmikantham, V., Bainov, D.D., Simeonov, P.S.: Theory of Impulsive Differential Equations. World Scientific, Singapore (1989)
Lee, E.K., Lee, Y.H.: Multiple positive solutions of singular Gelfand type problem for second-order impulsive differential equations. Appl. Math. Comput. 40, 307–328 (2004)
Lee, E.K., Lee, Y.H.: Multiplicity results of the Gelfand type singular boundary value problems for impulsive differential equations. Dyn. Contin. Discrete Impuls. Syst. 11, 451–467 (2004)
Lee, E.K., Lee, Y.H.: Multiple positive solutions of singular two point boundary value problems for second order impulsive differential equations. Appl. Math. Comput. 158, 745–759 (2004)
Lee, Y., Liu, X.: Study of singular boundary value problems for second order impulsive differential equations. J. Math. Anal. Appl. 331, 159–176 (2007)
Li, J., Nieto, J.J., Shen, J.: Impulsive periodic boundary value problems of first-order differential equations. J. Math. Anal. Appl. 325(1), 226–236 (2007)
Li, X., Shen, J., Rakkiyappan, R.: Persistent impulsive effects on stability of functional differential equations with finite or infinite delay. Appl. Math. Comput. 329, 14–22 (2018)
Li, X., Yang, X., Huang, T.: Persistence of delayed cooperative models: impulsive control method. Appl. Math. Comput. 342, 130–146 (2019)
Lin, X., Jiang, D.: Multiple positive solutions of Dirichlet boundary-value problems for second-order impulsive differential equations. J. Math. Anal. Appl. 321, 501–514 (2006)
Liu, Y., O’Regan, D.: Multiplicity results using bifurcation techniques for a class of boundary value problems of impulsive differential equations. Commun. Nonlinear Sci. Numer. Simul. 16, 1769–1775 (2011)
Liz, E., Nieto, J.: The monotone iterative technique for periodic boundary value problems of second order impulsive differential equations. Comment. Math. Univ. Carol. 34, 405–411 (1993)
Ma, T., Yan, B.: Positive global solutions of nonlocal boundary value problems for the nonlinear convection reaction-diffusion equations. Bound. Value Probl. 2017, 9 (2017)
Miao, C., Ge, W.: Existence of positive solutions for singular impulsive differential equations with integral boundary conditions. Math. Methods Appl. Sci. 38(6), 1146–1157 (2015)
Nieto, J.J.: Periodic boundary value problems for first-order impulsive ordinary differential equations. Nonlinear Anal. 51, 1223–1232 (2002)
Nieto, J.J., Rodriguez-Lopez, R.: Periodic boundary value problem for non-Lipschitzian impulsive functional differential equations. J. Math. Anal. Appl. 318, 593–610 (2006)
Niu, Y., Yan, B.: Global structure of solutions to boundary value problems of impulsive differential equations. Electron. J. Differ. Equ. 55(1), 1 (2016)
Niu, Y., Yan, B.: The existence of positive solutions for the singular two-point boundary value problem. Topol. Methods Nonlinear Anal. 48(1), 1 (2017)
Pandit, S.G., Deo, S.G.: Differential Systems Involving Impulses. Lecture Notes in Math., vol. 954. Springer, Berlin (1982)
Qian, D., Li, X.: Periodic solutions for ordinary differential equations with sublinear impulsive effects. J. Math. Anal. Appl. 303, 288–303 (2005)
Rachůnková, I.: Singular Dirichlet second order boundary value problems with impulses. J. Differ. Equ. 193, 435–459 (2003)
Rachůnková, I., Tomec̆ek, J.: Singular Dirichlet problem for ordinary differential equations with impulses. Nonlinear Anal. 65, 210–229 (2006)
Rachůnková, I., Tomec̆ek, J.: State-Dependent Impulses: Boundary Value Problems on Compact Interval (2015). https://doi.org/10.2991/978-94-6239-127-7. http://www.springer.com/in/book/9789462391260; ISBN 978-946239127-7; 978-946239126-0.
Rachůnková, I., Tvrdý, M.: Non-ordered lower and upper functions in impulsive second order periodic problems. Dyn. Contin. Discrete Impuls. Syst. 12, 397–415 (2005)
Rogovchenko, Y.V.: Impulsive evolution systems: main results and new trends. Dyn. Contin. Discrete Impuls. Syst. 3, 57–88 (1997)
Samoilenko, A.M., Perestyuk, N.A.: Impulsive Differential Equations. World Scientific, Singapore (1995)
Tomec̆ek, J.: Nonlinear boundary value problem for nonlinear second order differential equations with impulses. Electronic J. Qual. Theo. Diff. Equ. 2005(10), 1–22 (2005)
Trpianiello, G.M.: Elliptic Differential Equations and Obstacle Problems. Plenum, New York (1987)
Wang, J., Yan, B.: Global properties and multiple solutions for boundary value problems of impulsive differential equations. Electronic Journal of Differential Equations 2013(171), 1 (2013)
Xu, X., Liu, Y., Li, H., Alsaadi, F.E.: Synchronization of switched Boolean networks with impulsive effects. International Journal of Biomathematics 11(6), 1–18 (2018)
Yan, B., Ma, T.: The existence and multiplicity of positive solutions for a class of nonlocal elliptic problems. Bound. Value Probl. 2016, 165 (2016)
Yan, B., Wang, D.: The multiplicity of positive solutions for a class of nonlocal elliptic problem. J. Math. Anal. Appl. 442(1), 72–102 (2016)
Yang, D., Li, X., Qiu, J.: Output tracking control of delayed switched systems via state-dependent switching and dynamic output feedback. Nonlinear Analysis: Hybrid Systems 32, 294–305 (2019)
Yang, X., Li, X., Xi, Q., Duan, P.: Review of stability and stabilization for impulsive delayed systems. Mathematical Biosciences and Engineering 15(6), 1495–1515 (2018)
Zhang, Z., Yu, J.: A singular nonlinear Dirichlet problem with a convection term. Siam J. Math. Anal. 32(4), 916–927 (2000)
Zhao, D., Liu, Y.: Eigenvalues of nonlinear singular boundary value problems. International Journal of Mathematics and Computation 22(1), 34–46 (2014)
Zhao, D., Liu, Y.: Twin solutions to semipositone boundary value problems for fractional differential equations with coupled integral boundary conditions. J. Nonlinear Sci. Appl. 10, 3544–3565 (2017)
Acknowledgements
We thank the referees for their valuable suggestions. The work is supported by the NSFC of China (61603226) and the Fund of Natural Science of Shandong Province (ZR2018MA022).
Availability of data and materials
Not applicable
Authors’ information
Not applicable
Funding
Not applicable.
Author information
Authors and Affiliations
Contributions
All authors contributed equally to the writing of this paper. All authors read and approved the final manuscript.
Corresponding author
Ethics declarations
Competing interests
The authors declare that they have no competing interests.
Rights and permissions
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons licence, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons licence, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons licence and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this licence, visit http://creativecommons.org/licenses/by/4.0/.
About this article
Cite this article
Jin, F., Yan, B. Existence of positive solutions for singular Dirichlet boundary value problems with impulse and derivative dependence. Bound Value Probl 2020, 157 (2020). https://doi.org/10.1186/s13661-020-01454-w
Received:
Accepted:
Published:
DOI: https://doi.org/10.1186/s13661-020-01454-w