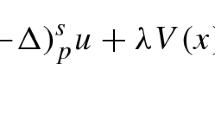1 Introduction and the main result

The Kirchhoff-type equation has wide applications in population dynamics, optimization, anomalous diffusion, continuum mechanics, etc. In recent years, research on this subject has been very active; see, for example, [1,2,3,4,5,6,7,8,9,10] and the references therein. This paper discuss the existence of infinitely many weak solutions for the following problem:

$$\begin{aligned} \textstyle\begin{cases} \mathcal{M} (\int_{\mathbb{R}^{2N}}\frac { \vert u(x)-u(y) \vert ^{p}}{ \vert x-y \vert ^{N+ps}}\,dx\,dy )(-\triangle)^{s}_{p}u(x) -\lambda \vert u \vert ^{p-2}u=g(x,u)& \mbox{in }\varOmega,\\ u=0& \mbox{in }\mathbb{R}^{N}\backslash\varOmega, \end{cases}\displaystyle \end{aligned}$$
(1.1)

where \(\varOmega\subset\mathbb{R}^{N}\) is a bounded smooth domain, \(0< s<1<p<\infty\), \(ps< N\). λ is a real parameter and \((-\triangle)_{p}^{s}\) is the fractional p-Laplacian operator defined by

$$(-\triangle)_{p}^{s}u(x)=2\lim_{\varepsilon\rightarrow0^{+}} \int _{\mathbb{R}^{N}\backslash B_{\varepsilon}(x)}\frac{ \vert u(x)-u(y) \vert ^{p-2}(u(x)-u(y))}{ \vert x-y \vert ^{N+ps}}\,dx\,dy, $$

where \(B_{\varepsilon}(x)=\{y\in \mathbb{R}^{n}:|x-y|<\varepsilon\}\). The function \(\mathcal{M}\) satisfies:

\((M_{1})\) :

\(\mathcal{M}\in C(\mathbb{R}_{0}^{+},\mathbb{R}^{+})\), \(\inf_{t\in\mathbb{R}^{+}_{0}}\mathcal{M}(t)\geq m_{0}>0\), \(m_{0}\) is a positive constant.

\((M_{2})\) :

There exists \(\theta\in[1,\frac{N}{N-ps})\), such that

$$\theta\mathcal{\widetilde{M}}(t)=\theta \int^{t}_{0}\mathcal {M}(s)\,ds\geq\mathcal{M}(t)t ,\quad \forall t\in\mathbb{R}^{+}_{0}. $$

In the study of the problems (1.1), the following Ambrosetti–Rabinowitz condition [11] is used widely:

$$\begin{aligned} 0< \mu G(x,t)=\mu \int^{t}_{0}g(x,\tau)\,d\tau\leq g(x,t)t,\quad x\in \varOmega, t\geq r, \end{aligned}$$
(AR)

where \(\mu>p\theta\) and \(r>0\).

It is well known that (AR) condition is very important for variational method, but cannot be satisfied in many cases. There have been some contributions attempting to replace this condition by new ones, we can consult the references [12,13,14,15,16]. Motivated by this work, in this paper we investigate the existence of infinitely many solutions of problem (1.1) without the (AR) condition. Our result extends Theorem 1 of [17] and Theorem 1.1 of [18].

We assume that \(g:\overline{\varOmega}\times\mathbb{R}\) is a continuous function satisfying:

\((g_{1})\) :

There exist constants \(1<\eta_{1}<\eta_{2}<\cdots<\eta_{m}<p<\alpha<p^{*}_{s}\), and functions \(v_{0}(x)\in L^{\beta}(\varOmega)\), where \(p^{*}_{s}=\frac{pN}{N-ps}\), \(\frac{1}{\alpha}+\frac{1}{\beta}=1\), \(v_{i}(x)\in L^{\frac{\alpha}{\alpha-\eta_{i}}}(\varOmega), i=1,\ldots,m\), and \(v_{m+1}>0\) is a constant such that

$$\begin{aligned} \bigl\vert g(x,u) \bigr\vert \leq \bigl\vert v_{0}(x) \bigr\vert +\sum_{i=1}^{m} \bigl\vert v_{i}(x) \bigr\vert \vert u \vert ^{\eta _{i}-1}+v_{m+1} \vert u \vert ^{\alpha-1}, \quad (x,u)\in\varOmega\times\mathbb{R}. \end{aligned}$$
\((g_{2})\) :

There are two constants \(\mu>p \theta\) and \(\varpi_{0}>0\) such that

$$G(x,t)= \int^{t}_{0}g(x,s)\,ds\leq\frac{1}{\mu}g(x,t)t+ \varpi _{0} \vert t \vert ^{p} \quad \mbox{for any }x\in\varOmega, t \in\mathbb{R}. $$
\((g_{3})\) :

\(\lim_{|t|\rightarrow\infty}\frac{G(x,t)}{|t|^{p\theta }}\rightarrow+\infty\) uniformly for \(x\in\varOmega\).

\((g_{4})\) :

\(g(x,t)\) is odd for t, i.e. \(g(x,-t)=-g(x,t)\) for any \(x\in \varOmega\) and \(t\in\mathbb{R}\).

Remark

(i) Note that condition \((g_{2})\) is different from the (AR) condition, and is weaker than the condition of [17, 19] and [18].

(ii) The function \(g(x,t)=|t|^{p\theta-2}t\ln(1+|t|)\) satisfies the conditions \((g_{2})\) and \((g_{3})\), but it does not satisfy the (AR) condition.

Now we state our main result.

Theorem 1.1

Set \(s\in(0,1)\), \(N>ps\). If \((M_{1})\)–\((M_{2})\) and \((g_{1})\)–\((g_{4})\) hold. Then, for any \(\lambda\in\mathbb{R}\), the problem (1.1) has infinitely many weak solutions \(\{u_{k}\}\) in \(X_{0}\) with unbounded energy.

The definition of weak solution will be given in the next section. The framework of this paper is as follows. In Sect. 2 we give the variational framework. Section 3 verifies the Cerami condition. In Sect. 4, we establish the existence of infinitely many weak solutions for problem (1.1) by the fountain theorem.

2 Variational framework

In this section, we first review some basic variational frameworks and main Lemmas that will be used in the next section for problem (1.1). Denote \(W=\mathbb{R}^{2N}\backslash\mathcal{O}\), where \(\mathcal{O}=\mathcal{C}(\varOmega)\times\mathcal{C}(\varOmega)\subset \mathbb{R}^{2N}\), and \(\mathcal{C}(\varOmega)=\mathbb{R}^{N}\backslash\varOmega\). Define a normed linear space X by

$$X=\biggl\{ u\in L^{p}(\varOmega)\Big| \int_{W}\frac { \vert u(x)-u(y) \vert ^{p}}{ \vert x-y \vert ^{N+ps}}\,dx\,dy< \infty\biggr\} $$

with norm

$$\begin{aligned} \Vert u \Vert _{X}= \Vert u \Vert _{L^{p}(\varOmega)}+ {\biggl(} \int_{W}\frac { \vert u(x)-u(y) \vert ^{p}}{ \vert x-y \vert ^{N+ps}}\,dx\,dy {\biggr)}^{\frac{1}{p}}. \end{aligned}$$

Then X is a normed linear space and \(C^{\infty}_{0}(\varOmega)\subset X\) (see [10], Lemma 2.1). Define a subspace \(X_{0}\subset X\) by

$$X_{0}=\bigl\{ u\in X:u(x)=0\mbox{ a.e. in }\mathbb{R}^{N}\backslash \varOmega\bigr\} . $$

Under the equivalent norm

$$\Vert u \Vert _{X_{0}}= {\biggl(} \int_{W}\frac { \vert u(x)-u(y) \vert ^{p}}{ \vert x-y \vert ^{N+ps}}\,dx\,dy {\biggr)}^{\frac{1}{p}},\quad u \in X_{0}. $$

\(X_{0}\) is a uniformly convex reflexive Banach space ([10], Lemma 2.4).

Now, we give the definition of weak solutions for problem (1.1).

Definition 2.1

We say that \(u\in X_{0}\) is a weak solution of problem (1.1), if

$$\begin{aligned} &\mathcal{M} \bigl( \Vert u \Vert _{X_{0}}^{p} \bigr) \int_{W}\frac { \vert u(x)-u(y) \vert ^{p-2}(u(x)-u(y))(\phi(x)-\phi(y))}{ \vert x-y \vert ^{N+ps}}\,dx\,dy \\ &\quad{} -\lambda \int_{\varOmega} \bigl\vert u(x) \bigr\vert ^{p-2}u(x) \phi(x)\,dx- \int_{\varOmega }g\bigl(x,u(x)\bigr)\phi(x)\,dx=0,\quad \forall \phi\in X_{0}. \end{aligned}$$

Subsequently we review some of the properties of the eigenvalue problem and the spectrum of the operator. Consider the problem:

$$ \textstyle\begin{cases} (-\triangle)_{p}^{s}u=\lambda_{k} \vert u \vert ^{p-2}u &\mbox{in }\varOmega,\\ u=0& \mbox{in }\mathbb{R}^{N}\backslash\varOmega, \end{cases} $$
(2.1)

there is a divergent positive eigenvalue sequence.

$$\lambda_{1}< \lambda_{2}\leq\cdots\leq \lambda_{k}\leq \lambda_{k+1}\leq\cdots, $$

whose eigenvalues are the critical values of the functional

$$T_{p}(u)= \Vert u \Vert _{X_{0}}^{p},\quad u\in \varSigma=\biggl\{ u\in X_{0}: \int_{\varOmega } \vert u \vert ^{p}\,dx=1\biggr\} . $$

We notice that the first eigenvalue \(\lambda_{1}:=\inf_{u\in\varSigma}T_{p}(u)>0\). The corresponding eigenfunctions will be denoted by \(e_{j}\). More details can be found in [20].

Let \(X_{j}=\operatorname{span}\{e_{j}\}\), define

$$X_{0}=\overline{\bigoplus_{i=1}^{\infty}X_{i}},\qquad Y_{k}=\bigoplus_{i=1}^{k}X_{i},\qquad Z_{k} =\overline{\bigoplus_{i=k}^{\infty}X_{i}},\quad k=1,2,\ldots. $$

And let \(W_{k}:=\{u\in Y_{k}:\|u\|_{X_{0}}\leq\rho_{k}\}\), \(N_{k}:=\{ u\in Z_{k}:\|u\|_{X_{0}}=\gamma_{k}\}\), where \(\rho_{k}>\gamma_{k}>0\).

Lemma 2.2

(Fountain theorem, [21])

Consider an even functional \(T\in C^{1}(X_{0},\mathbb{R})\). Assume for each \(k\in\mathbb{N}\), there exist \(\rho_{k}>\gamma _{k}>0\) such that

\((\varPhi_{1})\) :

\(a_{k}:=\max_{u\in Y_{k},\|u\|_{X_{0}}=\rho _{k}}T(u)\leq0\),

\((\varPhi_{2})\) :

\(b_{k}:=\inf_{u\in Z_{k},\|u\|_{X_{0}}=\gamma _{k}}T(u)\rightarrow+\infty, k\rightarrow+\infty\),

\((\varPhi_{3})\) :

T satisfies the \((PS)_{c}\) condition for every \(c>0\).

Then T has an unbounded sequence of critical values.

Define the energy functional \(T:X_{0}\rightarrow\mathbb{R}\) corresponding to the problem (1.1) by

$$T(u)=I(u)-J(u)-H(u), $$

where

$$I(u)=\frac{1}{p}\widetilde{\mathcal{M}}\bigl( \Vert u \Vert _{X_{0}}^{p}\bigr),\qquad J(u)=\frac{\lambda}{p} \int_{\varOmega} \vert u \vert ^{p}\,dx,\qquad H(u) = \int_{\varOmega}G(x,u)\,dx. $$

Lemma 2.3

([10])

If \((M_{1})\) holds, then \(I: X_{0}\rightarrow\mathbb{R}\) is of class \(C^{1}(X_{0},\mathbb{R})\), and

$$\bigl\langle I^{\prime}(u),\phi\bigr\rangle =\mathcal{M} \bigl( \Vert u \Vert _{X_{0}}^{p} \bigr) \int_{W}\frac{ \vert u(x)-u(y) \vert ^{p-2}(u(x)-u(y))(\phi(x) -\phi(y))}{ \vert x-y \vert ^{N+ps}}\,dx\,dy $$

for all \(u,\phi\in X_{0}\).

Lemma 2.4

([22], Lemma 2)

Assume that g is a continuous function. Let \((g_{1})\) holds, then the functional H is of class \(C^{1}(X_{0},\mathbb{R})\), and

$$\bigl\langle H^{\prime}(u),\phi\bigr\rangle = \int_{\varOmega}g(x,u)\phi \,dx $$

for all \(u, \phi\in X_{0}\).

Combining Lemma 2.3 with Lemma 2.4, we get \(T\in C^{1}(X_{0},\mathbb{R})\) and

$$\begin{aligned} \bigl\langle T^{\prime}(u),\phi\bigr\rangle ={}&\mathcal{M} \bigl( \Vert u \Vert _{X_{0}}^{p} \bigr) \int_{W}\frac{ \vert u(x)-u(y) \vert ^{p-2}(u(x)-u(y))(\phi(x) -\phi(y))}{ \vert x-y \vert ^{N+ps}}\,dx\,dy \\ &{}-\lambda \int_{\varOmega} \bigl\vert u(x) \bigr\vert ^{p-2}u(x) \phi(x)\,dx- \int_{\varOmega }g\bigl(x,u(x)\bigr)\phi(x)\,dx=0 \end{aligned}$$

for any \(u,\phi\in X_{0}\). Clearly, weak solutions of problem (1.1) are the critical points of energy functional T.

3 Verification of compactness conditions

We firstly state two definitions.

Definition 3.1

([23, 24])

Let \(T\in C^{1}(X_{0},\mathbb{R})\), we say that T satisfies the \((PS)_{c}\) condition at the level \(c\in\mathbb{R}\), if any sequence \(\{u_{n}\}_{n}\subset X_{0}\) such that

$$ T(u_{n})\rightarrow c,\quad \sup_{ \Vert \phi \Vert _{X_{0}=1}}\bigl\{ \bigl\vert \bigl\langle T^{\prime}(u_{n}),\phi\bigr\rangle \bigr\vert \bigr\} \rightarrow0\quad \mbox{as }n\rightarrow\infty, $$

possesses a convergent subsequence in \(X_{0}\); T satisfies the (PS) condition if T satisfies the \((PS)_{c}\) for all \(c\in\mathbb{R}\).

Definition 3.2

([25, 26])

Let \(T\in C^{1}(X,\mathbb{R})\), we say that T satisfies the \((Ce)_{c}\) condition at the level \(c\in\mathbb{R}\), if any sequence \(\{u_{n}\}_{n}\subset X_{0}\) such that

$$ T(u_{n})\rightarrow c,\quad \bigl(1+ \Vert u_{n} \Vert \bigr)\sup_{ \Vert \phi \Vert _{X_{0}=1}}\bigl\{ \bigl\vert \bigl\langle T^{\prime}(u_{n}),\phi\bigr\rangle \bigr\vert \bigr\} \rightarrow0 \quad\mbox{as }n\rightarrow\infty, $$

possesses a convergent subsequence in \(X_{0}\); T satisfies the (Ce) condition if T satisfies the \((Ce)_{c}\) for all \(c\in\mathbb{R}\).

When T fulfills the (AR) condition, we know the corresponding energy functional T fulfills the Palais-Smale compactness assumptions, however we dropped the (AR) condition, we show that T fulfills the (Ce) condition.

Lemma 3.3

Let \(g:\overline{\varOmega}\times\mathbb{R}\rightarrow\mathbb{R}\) be a continuous function satisfying conditions \((M_{1})\)–\((M_{2})\) and \((g_{1})\)–\((g_{3})\). Then T fulfills the (Ce) condition at level \(c\in\mathbb{R}\).

Proof

Set \(c\in\mathbb{R}\). Suppose \(\{u_{n}\}\) satisfies

$$ T(u_{n})\rightarrow c,\quad \bigl(1+ \Vert u_{n} \Vert \bigr)\sup_{ \Vert \phi \Vert _{X_{0}=1}}\bigl\{ \bigl\vert \bigl\langle T^{\prime}(u_{n}),\phi\bigr\rangle \bigr\vert \bigr\} \rightarrow0 $$
(3.1)

as \(n\rightarrow\infty\).

Step 1. We prove the sequence \(\{u_{n}\}\) is bounded in \(X_{0}\).

Arguing by contradiction, if \(\{u_{n}\}_{n\in\mathbb{N}}\) is unbounded in \(X_{0}\). Up to subsequence, still denoted by \(\{u_{n}\}_{n\in\mathbb{N}}\), suppose

$$ \Vert u_{n} \Vert _{X_{0}}\rightarrow+\infty. $$
(3.2)

It follows from (3.1) and (3.2) that

$$\begin{aligned} \sup_{ \Vert \phi \Vert _{X_{0}=1}}\bigl\{ \bigl\vert \bigl\langle T^{\prime}(u_{n}),\phi\bigr\rangle \bigr\vert \bigr\} \rightarrow0. \end{aligned}$$
(3.3)

Thus

$$\begin{aligned} \Vert u_{n} \Vert _{X_{0}}\sup _{ \Vert \phi \Vert _{X_{0}=1}}\bigl\{ \bigl\vert \bigl\langle T^{\prime}(u_{n}), \phi\bigr\rangle \bigr\vert \bigr\} \rightarrow0 \end{aligned}$$
(3.4)

as \(n\rightarrow\infty\). For any \(n\in\mathbb{N}\), we consider \(\nu _{n}:=\frac{u_{n}}{\|u_{n}\|_{X_{0}}}\), then \(\|\nu_{n}\|_{X_{0}}=1\), so \(\{\nu_{n}\}_{n\in\mathbb{N}}\) is bounded in \(X_{0}\). Similarly to Lemma 1 in [22]. Going if necessary to a subsequence, there exists \(\nu_{\infty}\) such that

$$\begin{aligned} &\nu_{n}\rightarrow\nu_{\infty}\quad \mbox{in } L^{p}\bigl(\mathbb{R}^{N}\bigr), \end{aligned}$$
(3.5)
$$\begin{aligned} &\nu_{n}\rightarrow\nu_{\infty} \quad\mbox{in }L^{\alpha}\bigl( \mathbb{R}^{N}\bigr), \end{aligned}$$
(3.6)
$$\begin{aligned} &\nu_{n}\rightarrow\nu_{\infty}\quad \mbox{in }\mathbb{R}^{N}, \end{aligned}$$
(3.7)

as \(n\rightarrow\infty\). We discuss two cases.

Case 1. \(\nu_{\infty}\equiv0\).

By \((g_{2})\), \((M_{2})\) and (3.1)–(3.2), we obtain

$$\begin{aligned} &\frac{1}{ \Vert u_{n} \Vert _{X_{0}}^{p}} {\biggl(}T(u_{n})-\frac{1}{\mu }T^{\prime}(u_{n})u_{n} {\biggr)} \\ &\quad\geq\frac{1}{ \Vert u_{n} \Vert _{X_{0}}^{p}} {\biggl(}\frac{1}{p}\widetilde {\mathcal{M}} \bigl( \Vert u_{n} \Vert _{X_{0}}^{p}\bigr) -\frac{1}{\mu} \mathcal{M}\bigl( \Vert u_{n} \Vert _{X_{0}}^{p}\bigr) \Vert u_{n} \Vert _{X_{0}}^{p} \\ &\qquad{}+\lambda\biggl(\frac{1}{\mu}- \frac{1}{p}\biggr) \Vert u_{n} \Vert _{p}^{p}- \int_{\varOmega} \biggl(G\bigl(x,u_{n}(x)\bigr)- \frac {1}{\mu}g\bigl(x,u_{n}(x)\bigr)u_{n}(x) \biggr) \,dx {\biggr)} \\ &\quad\geq\frac{1}{ \Vert u_{n} \Vert _{X_{0}}^{p}} {\biggl(}\biggl(\frac{1}{p\theta }-\frac{1}{\mu} \biggr)\mathcal{M}\bigl( \Vert u_{n} \Vert _{X_{0}}^{p}\bigr) \Vert u_{n} \Vert _{X_{0}}^{p} {\biggr)}-\lambda\biggl( \frac{1}{\mu}-\frac{1}{p}\biggr) \int _{\varOmega}\nu_{n}^{p}\,dx- \varpi_{0} \int_{\varOmega} \vert \nu_{n} \vert ^{p}\,dx \\ &\quad \geq m_{0}\biggl(\frac{1}{p\theta}-\frac{1}{\mu}\biggr), \end{aligned}$$

which implies \(0\geq m_{0}(\frac{1}{p\theta}-\frac{1}{\mu})\). This is a contradiction.

Case 2. \(\nu_{\infty}\not\equiv0\).

Setting \(\varOmega_{1}=\{x\in\varOmega:\nu_{\infty}\neq0\}\), it is easy to see that \(|\varOmega_{1}|>0\) and

$$ \bigl\vert u_{n}(x) \bigr\vert = \vert \nu_{n} \vert \Vert u_{n} \Vert _{X_{0}} \rightarrow+\infty\quad \mbox{on }\varOmega_{1} $$
(3.8)

as \(n\rightarrow\infty\), thanks to (3.2), (3.7). From (3.1) and (3.2), we get \(\frac{T(u_{n})}{\|u_{n}\|^{p\theta}_{X_{0}}}\rightarrow0\), that is,

$$\begin{aligned} 0={}&\lim_{n\rightarrow\infty}\frac{1}{ \Vert u_{n}(x) \Vert _{X_{0}}^{p\theta }} {\biggl(} \frac{1}{p}\widetilde{\mathcal{M}} \bigl( \Vert u_{n} \Vert _{X_{0}}^{p}\bigr) -\frac{\lambda}{p} \Vert u_{n} \Vert ^{p}_{p}- \int_{\varOmega _{1}}G(x,u_{n})\,dx \\ &{}- \int_{\varOmega\backslash\varOmega_{1}}G(x,u_{n})\,dx {\biggr)}. \end{aligned}$$
(3.9)

Note that

$$0< \lambda_{1}=\min_{u\in X_{0}\backslash\{0\}}\frac{\int_{\varOmega }\frac{ \vert u(x)-u(y) \vert ^{p}}{ \vert x-y \vert ^{n+ps}}\,dx\,dy}{ \int_{\varOmega} \vert u(x) \vert ^{p}\,dx}, $$

which implies

$$ \Vert u \Vert ^{p}_{L^{p}(\varOmega)}\leq \frac{1}{\lambda_{1}} \Vert u \Vert ^{p}_{X_{0}}. $$
(3.10)

Because of \((M_{2})\), we get

$$ \widetilde{\mathcal{M}}(t)\leq\widetilde{\mathcal{M}}(1)t^{\theta },\quad \forall t\in[1,+\infty). $$
(3.11)

By (3.9)–(3.11), we obtain

$$\begin{aligned} 0={}&\lim_{n\rightarrow\infty}\frac{1}{ \Vert u_{n} \Vert _{X_{0}}^{p\theta}} {\biggl(}\frac{1}{ p} \widetilde{\mathcal{M}}\bigl( \Vert u_{n} \Vert _{X_{0}}^{p}\bigr) -\frac{\lambda}{p} \Vert u_{n} \Vert ^{p}_{p}- \int_{\varOmega _{1}}G\bigl(x,u_{n}(x)\bigr)\,dx \\ &{}- \int_{\varOmega\backslash\varOmega_{1}}G\bigl(x,u_{n}(x)\bigr)\,dx {\biggr)} \\ \leq{}&\frac{1}{p}\widetilde{\mathcal{M}}(1) -\lim_{n\rightarrow\infty} \frac{1}{ \Vert u_{n} \Vert _{X_{0}}^{p\theta }} {\biggl(}\frac{\lambda}{p} \Vert u_{n} \Vert ^{p}_{p} + \int_{\varOmega_{1}}G\bigl(x,u_{n}(x)\bigr)\,dx \\ &{}+ \int_{\varOmega\backslash\varOmega_{1}}G\bigl(x,u_{n}(x)\bigr)\,dx {\biggr)}. \end{aligned}$$
(A)

When \(\lambda\geq0\), from (A), we have

$$\begin{aligned} 0\leq{}&\frac{1}{p}\widetilde{\mathcal{M}}(1) -\lim _{n\rightarrow\infty}\frac{1}{ \Vert u_{n} \Vert _{X_{0}}^{p\theta }} {\biggl(}\frac{\lambda}{p} \Vert u_{n} \Vert ^{p}_{p} + \int_{\varOmega_{1}}G\bigl(x,u_{n}(x)\bigr)\,dx \\ &{}+ \int_{\varOmega\backslash\varOmega_{1}}G\bigl(x,u_{n}(x)\bigr)\,dx {\biggr)} \\ \leq{}&\frac{1}{p}\widetilde{\mathcal{M}}(1) -\lim_{n\rightarrow\infty} \frac{1}{ \Vert u_{n} \Vert _{X_{0}}^{p\theta }} {\biggl(} \int_{\varOmega_{1}}G\bigl(x,u_{n}(x)\bigr)\,dx \\ &{}+ \int_{\varOmega\backslash\varOmega_{1}}G\bigl(x,u_{n}(x)\bigr)\,dx {\biggr)}. \end{aligned}$$
(3.12)

When \(\lambda<0\), from (A) and (3.10), we get

$$\begin{aligned} 0\leq{}&\frac{1}{p}\widetilde{\mathcal{M}}(1) -\lim _{n\rightarrow\infty}\frac{1}{ \Vert u_{n} \Vert _{X_{0}}^{p\theta }} {\biggl(}\frac{\lambda}{p} \Vert u_{n} \Vert ^{p}_{p} + \int_{\varOmega_{1}}G\bigl(x,u_{n}(x)\bigr)\,dx \\ &{}+ \int_{\varOmega\backslash\varOmega_{1}}G\bigl(x,u_{n}(x)\bigr)\,dx {\biggr)} \\ \leq{}&\frac{1}{p}\widetilde{\mathcal{M}}(1) -\lim_{n\rightarrow\infty} \frac{\lambda}{p\lambda_{1} \Vert u_{n} \Vert ^{p\theta-p}_{X_{0}}} -\lim_{n\rightarrow\infty}\frac{1}{ \Vert u_{n} \Vert _{X_{0}}^{p\theta }} {\biggl(} \int_{\varOmega_{1}}G\bigl(x,u_{n}(x)\bigr)\,dx \\ &{}+ \int_{\varOmega\backslash\varOmega_{1}}G\bigl(x,u_{n}(x)\bigr)\,dx {\biggr)} \\ ={}&\frac{1}{p}\widetilde{\mathcal{M}}(1) -\lim_{n\rightarrow\infty} \frac{1}{ \Vert u_{n} \Vert _{X_{0}}^{p\theta }} {\biggl(} \int_{\varOmega_{1}}G\bigl(x,u_{n}(x)\bigr)\,dx \\ &{}+ \int_{\varOmega\backslash\varOmega_{1}}G\bigl(x,u_{n}(x)\bigr)\,dx {\biggr)}. \end{aligned}$$
(3.13)

It follows from \((g_{3})\) and (3.8) that

$$\frac{G(x,u_{n}(x))}{ \Vert u_{n} \Vert ^{p\theta}_{X_{0}}}=\frac {G(x,u_{n}(x))}{ \vert u_{n}(x) \vert ^{p\theta}}\frac{ \vert u_{n}(x) \vert ^{p\theta}}{ \Vert u_{n} \Vert ^{p\theta}_{X_{0}}}=\frac {G(x,u_{n}(x))}{ \vert u_{n}(x) \vert ^{p\theta}} \bigl\vert \nu_{n}(x) \bigr\vert ^{p\theta }\rightarrow+\infty\quad \mbox{a.e. } x\in\varOmega_{1} $$

as \(n\rightarrow\infty\). Making use of the Fatou lemma, we obtain

$$ \lim_{n\rightarrow\infty} \int_{\varOmega_{1}}\frac {G(x,u_{n}(x))}{ \vert u_{n}(x) \vert ^{p\theta}}\,dx\rightarrow+\infty. $$
(3.14)

By \((g_{3})\), we know

$$ \lim_{t\rightarrow+\infty}G(x,t)=+\infty $$
(3.15)

uniformly for every \(x\in\overline{\varOmega}\). Therefore, (3.15) means that there are two positive constants \(t_{1}\) and D such that

$$ G(x,t)\geq D $$
(3.16)

for any \(x\in\overline{\varOmega}\) and \(|t|>t_{1}\). In addition, since the continuity of G on \(\overline{\varOmega}\times\mathbb{R}\), we get

$$ G(x,t)\geq\min_{(x,t)\in\overline{\varOmega}\times [-t_{1},t_{1}]}G(x,t), \quad \forall \vert t \vert \leq t_{1}. $$
(3.17)

Hence, in view of (3.16) and (3.17), we have

$$ G(x,t)\geq\min\Bigl\{ D,\min_{(x,t)\in\overline{\varOmega}\times [-t_{1},t_{1}]}G(x,t)\Bigr\} := \varrho, \quad\forall(x,t)\in\overline{\varOmega}\times\mathbb{R}. $$
(3.18)

By (3.2) and (3.18), we obtain

$$ \lim_{n\rightarrow\infty} \int_{\varOmega\backslash\varOmega_{1}}\frac {G(x,u_{n}(x))}{ \vert u_{n}(x) \vert ^{p\theta}}\,dx\geq0. $$
(3.19)

Combining (3.19) and (3.12)–(3.14), we have a contradiction.

Step 2. We prove \(\{u_{n}\}\rightarrow u \) in \(X_{0}\) for some u.

Let \(K(x-y)=|x-y|^{-N-ps}\). For every fixed \(\varphi\in X_{0}\), define

$$Q_{\varphi}(v)= \int_{\mathbb{R}^{2N}} \bigl\vert \varphi(x)-\varphi (y) \bigr\vert ^{p-2}\bigl(\varphi(x)-\varphi(y)\bigr) (v(x)-v(y)K(x-y)\,dx\,dy $$

for any \(v\in X_{0}\). By the Hölder inequality and the continuity of \(Q_{\varphi}\), we get

$$\bigl\vert Q_{\varphi}(v) \bigr\vert \leq \Vert \varphi \Vert _{X_{0}}^{p-1} \Vert v \Vert _{X_{0}}, \quad\forall v\in X_{0}. $$

Since \(u_{n}\rightharpoonup u\) in \(X_{0}\), we have

$$ \lim_{n\rightarrow\infty}Q_{u}(u_{n}-u)=0. $$
(3.20)

Obviously, \(\langle T^{\prime}(u_{n}),u_{n}-u\rangle\rightarrow0\). Then we have

$$\begin{aligned} \bigl\langle T^{\prime}(u_{n}),u_{n}-u \bigr\rangle ={}&\mathcal{M}\bigl( \bigl\Vert u_{n}(x) \bigr\Vert _{X_{0}}^{p}\bigr)Q_{u_{n}}(u_{n}-u)- \lambda \int_{\varOmega} \vert u_{n} \vert ^{p-2}u_{n}(u_{n}-u) \,dx \\ &{}- \int_{\varOmega}g(x,u_{n}) (u_{n}-u)\,dx \rightarrow0 \end{aligned}$$
(3.21)

as \(n\rightarrow\infty\). Due to the reflexivity of \(X_{0}\), similarly to Lemma 8 in [28], there is a subsequence, still denoted by \(\{u_{n}\}_{n}\), such that

$$\begin{aligned} &u_{n}\rightharpoonup u\quad \mbox{in }X_{0}, \\ &u_{n}\rightarrow u \quad\mbox{in }L^{r}(\varOmega), 1\leq r< p_{s}^{*}, \\ &u_{n}\rightarrow u \quad\mbox{a.e. in }\mathbb{R}^{N} \end{aligned}$$

as \(n\rightarrow\infty\). So, \(g(x,u_{n})(u_{n}-u)\rightarrow0\) a.e. in Ω as \(n\rightarrow\infty\). The sequence \(\{g(x,u_{n})(u_{n}-u)\}\) is uniformly bounded and equi-integrable in \(L^{1}(\varOmega)\). By the Vitali Convergence Theorem (see [27]),

$$\lim_{n\rightarrow\infty} \int_{\varOmega}g(x,u_{n}) (u_{n}-u)\,dx=0. $$

By (3.21), we have

$$\mathcal{M}\bigl( \bigl\Vert u_{n}(x) \bigr\Vert _{X_{0}}^{p}\bigr)Q_{u_{n}}(u_{n}-u) \rightarrow0 $$

as \(n\rightarrow0\). It follows from \((M_{1})\),

$$ Q_{u_{n}}(u_{n}-u)\rightarrow0 $$
(3.22)

as \(n\rightarrow\infty\). Combining (3.20) with (3.22), we get

$$ \bigl(Q_{u_{n}}(u_{n}-u)-Q_{u}(u_{n}-u) \bigr)\rightarrow0 $$
(3.23)

as \(n\rightarrow0\). Using the Simon inequalities

$$\begin{aligned} &\bigl( \vert \varrho \vert ^{p-2}\varrho- \vert \sigma \vert ^{p-2}\sigma\bigr)\cdot(\varrho-\sigma )\geq D_{P} \vert \varrho-\sigma \vert ^{p},\quad p\geq2, \\ &\bigl( \vert \varrho \vert ^{p-2}\varrho- \vert \sigma \vert ^{p-2}\sigma\bigr)\cdot(\varrho-\sigma )\geq\widehat{D_{P}} \frac{ \vert \varrho -\sigma \vert ^{2}}{( \vert \varrho \vert + \vert \sigma \vert )^{2-p}},\quad 1< p< 2, \end{aligned}$$

for all \(\varrho, \sigma\in\mathbb{R}^{N}\), where \(D_{P}, \widehat {D_{P}}>0\) only rely on p.

If \(p\geq2\), By (3.23), for n large enough,

$$\begin{aligned} \Vert u_{n}-u \Vert _{X_{0}}^{p} \leq{}&D_{P} \int_{\mathbb{R}^{2N}} \bigl( \bigl\vert u_{n}(x)-u_{n}(y) \bigr\vert ^{p-2}\bigl(u_{n}(x)-u_{n}(y)\bigr) \\ &{}- \bigl\vert u(x)-u(y) \bigr\vert ^{p-2}\bigl(u(x)-u(y)\bigr) \bigr) \\ &{}\times \bigl(u_{n}(x)-u(x)-u_{n}(y)+u(y) \bigr)K(x-y)\,dx \,dy \\ ={}&D_{P} \bigl(Q_{u_{n}}(u_{n}-u)-Q_{u}(u_{n}-u) \bigr)=o(1). \end{aligned}$$

Then \(\|u_{n}-u\|_{X_{0}}^{p}\rightarrow0\).

If \(1< p<2\), though the Hölder inequality, the Simon inequality, and (3.23), we have

$$\begin{aligned} \Vert u_{n}-u \Vert _{X_{0}}^{p}\leq{}& \widehat{D_{P}} \bigl(Q_{u_{n}}(u_{n}-u)-Q_{u}(u_{n}-u) \bigr)^{\frac{p}{2}} \bigl( \Vert u_{n} \Vert _{X_{0}}^{p} + \Vert u \Vert _{X_{0}}^{p} \bigr)^{\frac{2-p}{2}} \\ \leq{}&\widehat{D_{P}} \bigl(Q_{u_{n}}(u_{n}-u) -Q_{u}(u_{n}-u) \bigr)^{\frac{p}{2}} \bigl( \Vert u_{n} \Vert _{X_{0}}^{p(2-p)/2}+ \Vert u \Vert _{X_{0}}^{p(2-p)/2} \bigr) \\ ={}&C \bigl(Q_{u_{n}}(u_{n}-u)-Q_{u}(u_{n}-u) \bigr)^{\frac{p}{2}}=o(1), \end{aligned}$$

as \(n\rightarrow\infty\), where \(C>0\). Combining the above two cases, thus, \(u_{n}\rightarrow u\) in \(X_{0}\). The proof of Lemma 3.3 is completed. □

4 Proof of Theorem 1.1

Similarly to [5], by a direct calculation, we have the following lemma.

Lemma 4.1

Set \(1\leq q< p_{s}^{*}\) and, for every \(k\in\mathbb{N}\), let

$$\mu_{k}:=\sup\bigl\{ \Vert u \Vert _{q}:u\in Z_{k}, \Vert u \Vert _{X_{0}}=1\bigr\} . $$

Then \(\mu_{k}\rightarrow0\textit{ as }k\rightarrow\infty\).

Proof of Theorem 1.1

We only need to verify the conditions \((\varPhi_{1})\) and \((\varPhi_{2})\) of Lemma 2.2.

Verification of \((\varPhi_{1})\): Since \(Y_{k}\) is finite dimensional, there exist positive constants \(B_{k,q}\) and \(\widetilde{B_{k,q}}\) depending on \(k,q\), such that for each \(u\in Y_{k}\)

$$ B_{k,q} \Vert u \Vert _{X_{0}}\leq \Vert u \Vert _{q}\leq\widetilde{B_{k,q}} \Vert u \Vert _{X_{0}}. $$
(4.1)

In view of \((g_{3})\), for every \(c>\frac{\widetilde{\mathcal {M}}(1)}{pB_{k,p\theta}^{p\theta}}\), there exists \(\delta_{c}>0\) such that

$$ G(x,t)\geq c \vert t \vert ^{p\theta} $$
(4.2)

for all \(x\in\overline{\varOmega}, |t|>\delta_{c}\). According to the Weierstrass theorem, we get

$$ G(x,t)\geq m_{c}:=\min_{x\in\overline{\varOmega},|t|\leq\delta_{c}}G(x,t) $$
(4.3)

for any \(|t|\leq\delta_{c}\). We claim that \(m_{c}\leq0\), since \(G(x,0)=0\) for all \(x\in\overline{\varOmega}\). Therefore, by (4.2) and (4.3), we obtain

$$ G(x,t)\geq c \vert t \vert ^{p\theta}-H_{c} $$
(4.4)

for all \((x,t)\in\overline{\varOmega}\times\mathbb{R}\), for suitable positive constant \(H_{c}\geq c\delta_{c}-m_{c}\).

By (4.1), (4.4) and (3.11), we get

$$\begin{aligned} T(u)\leq{}&\frac{1}{p}\widetilde{\mathcal{M}}\bigl( \Vert u \Vert _{X_{0}}^{p}\bigr) -\frac{\lambda}{p} \Vert u \Vert ^{p}_{p}-c \Vert u \Vert ^{p\theta}_{p\theta }+H_{c} \vert \varOmega \vert \\ \leq{}&\frac{\widetilde{\mathcal{M}}(1)}{p} \Vert u \Vert _{X_{0}}^{p\theta }- \frac{\lambda}{p} \Vert u \Vert ^{p}_{p}-c \Vert u \Vert ^{p\theta}_{p\theta} +H_{c} \vert \varOmega \vert \\ \leq{}&\frac{\widetilde{\mathcal{M}}(1)}{p} \Vert u \Vert _{X_{0}}^{p\theta }-cB_{k,p\theta}^{p\theta} \Vert u \Vert ^{p\theta}_{X_{0}} -\frac{\lambda}{p}B_{k,p}^{p} \Vert u \Vert ^{p}_{X_{0}}+H_{c} \vert \varOmega \vert . \end{aligned}$$

So, we have, for any \(u\in Y_{k}\) with \(\|u\|_{X_{0}}=\rho_{k}\geq1\) for big enough, such that \(T\leq0\). The condition \((\varPhi_{1})\) holds.

Verification of \((\varPhi_{2})\): There exists \(\gamma_{k}>0\) such that

$$b_{k}:=\inf\bigl\{ T(u):u\in Z_{k}, \Vert u \Vert _{X_{0}}=\gamma_{k}\bigr\} \rightarrow +\infty $$

as \(k\rightarrow+\infty\). It follows from \((g_{1})\), \((M_{1})\) and the Hölder inequality that

$$\begin{aligned} T(u)\geq{}&\frac{1}{p}\widetilde{\mathcal{M}}\bigl( \Vert u \Vert _{X_{0}}^{p}\bigr) -\frac{\lambda}{p} \Vert u \Vert ^{p}_{p}- \Vert v_{0} \Vert _{\beta} \Vert u \Vert _{\alpha }-\sum_{i=1}^{m} \bigl\Vert v_{i}(x) \bigr\Vert _{\frac{\alpha}{\alpha-\eta_{i}}} \Vert u \Vert ^{\eta_{i}}_{\alpha}-v_{m+1} \Vert u \Vert ^{\alpha}_{\alpha} \\ \geq{}&C_{K,\lambda} \Vert u \Vert _{X_{0}}^{p}- \Vert v_{0} \Vert _{\beta} { \biggl\Vert }\frac {u}{ \Vert u \Vert _{X_{0}}} { \biggr\Vert }_{\alpha} \Vert u \Vert _{X_{0}} \\ &{}- \sum_{i=1}^{m} \bigl\Vert v_{i}(x) \bigr\Vert _{\frac{\alpha}{\alpha-\eta_{i}}} { \biggl\Vert } \frac{u}{ \Vert u \Vert _{X_{0}}} { \biggr\Vert }_{\alpha}^{\eta_{i}} \Vert u \Vert _{X_{0}}^{\eta_{i}} -v_{m+1} { \biggl\Vert } \frac{u}{ \Vert u \Vert _{X_{0}}} { \biggr\Vert }_{\alpha}^{\alpha} \Vert u \Vert _{X_{0}}^{\alpha} \\ \geq{}&C_{K,\lambda} \Vert u \Vert _{X_{0}}^{p}- \mu_{k} \Vert v_{0} \Vert _{\beta} \Vert u \Vert _{X_{0}}- \sum_{i=1}^{m} \bigl\Vert v_{i}(x) \bigr\Vert _{\frac{\alpha}{\alpha-\eta_{i}}}\mu _{k}^{\eta_{i}} \Vert u \Vert _{X_{0}}^{\eta_{i}} -v_{m+1}\mu_{k}^{\alpha} \Vert u \Vert _{X_{0}}^{\alpha}, \end{aligned}$$

where \(\mu_{k}\) is defined in Lemma 4.1 and

$$\begin{aligned} C_{K,\lambda}=&\textstyle\begin{cases} \frac{1}{2}m_{0} &\mbox{if }\lambda\leq0, \\ \frac{1}{2} (m_{0}-\frac{\lambda}{\lambda_{1}} ) &\mbox{if } 0< \lambda< \lambda_{1},\\ \frac{1}{2} (m_{0}-\frac{\lambda}{\lambda_{k}} ) &\mbox{if } 0< \lambda_{k}< \lambda_{k+1}. \end{cases}\displaystyle \end{aligned}$$

We define \(\gamma_{k}\) as

$$\gamma_{k}= \biggl(\frac{pC_{K,\lambda}}{\alpha v_{m+1}\mu _{k}^{\alpha}} \biggr)^{\frac{1}{\alpha-p}}. $$

Thus \(\gamma_{k}\rightarrow+\infty\) as \(k\rightarrow\infty\). Note that \(\alpha>p\), so for every \(\|u\|_{X_{0}}=\gamma_{k}\), we get

$$\begin{aligned} T(u)\geq{}& \Vert u \Vert _{X_{0}}^{p}\bigl(C_{K,\lambda}-v_{m+1} \mu_{k}^{\alpha} \Vert u \Vert _{X_{0}}^{\alpha-p} \bigr)-\mu_{k} \Vert v_{0} \Vert _{\beta} \gamma_{k}- \sum_{i=1}^{m} \bigl\Vert v_{i}(x) \bigr\Vert _{\frac{\alpha}{\alpha-\eta_{i}}}\mu _{k}^{\eta_{i}} \gamma_{k}^{\eta_{i}} \\ ={}& \biggl(1-\frac{p}{\alpha} \biggr)C_{K,\lambda}\gamma_{k}^{p}- \mu _{k} \Vert v_{0} \Vert _{\beta} \gamma_{k}- \sum_{i=1}^{m} \bigl\Vert v_{i}(x) \bigr\Vert _{\frac{\alpha}{\alpha-\eta_{i}}}\mu _{k}^{\eta_{i}} \gamma_{k}^{\eta_{i}}\rightarrow+\infty \end{aligned}$$

as \(k\rightarrow\infty\). Therefore, the condition \((\varPhi_{2})\) is satisfied. The proof is completed. □

5 Conclusion

In this article, the existence of infinitely many solutions to Eq. (1.1) is established by using the variational methods (i.e. the fountain theorem). We consider fractional p-Kirchhoff problems with more general nonlinearity g in Ω, which improves the previous results. In order to overcome new difficulties, we need to adopt special techniques and methods in our paper.