Modeling Rabies Transmission in Spatially Heterogeneous Environments via $\theta$-diffusion

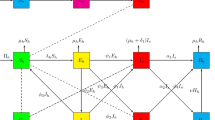
Abstract

Rabies among dogs remains a considerable risk to humans and constitutes a serious public health concern in many parts of the world. Conventional mathematical models for rabies typically assume homogeneous environments, with a standard diffusion term for the population of rabid animals. It has recently been recognized, however, that spatial heterogeneity plays an important role in determining spatial patterns of rabies and the cost-effectiveness of vaccinations. In this paper, we develop a spatially heterogeneous dog rabies model by using the $\theta$-diffusion equation, where $\theta$ reflects the way individual dogs make movement decisions in the underlying random walk. We numerically investigate the dynamics of the model in three diffusion cases: homogeneous, city-wild, and Gaussian-type. We find that the initial conditions affect whether traveling waves or epizootic waves can be observed. However, different initial conditions have little impact on steady-state solutions. An “active” interface is observed between city and wild regions, with a “ridge” on the city side and a “valley” on the wild side for the infectious dog population. In addition, the progressing speed of epizootic waves changes in heterogeneous environments. It is impossible to eliminate rabies in the entire spatial domain if vaccination is focused only in the city region or only in the wild region. When a seasonal transmission is incorporated, the dog population size approaches a positive time-periodic spatially heterogeneous state eventually.

Appendix: supplementary figures

In this Appendix, we provide supplementary figures. For the homogeneous diffusion in Sect. 4, the spatiotemporal dynamics of dogs under the homogeneous initial condition and the stepwise initial condition are given in Figs. 17 and 18 , respectively. For the city-wild diffusion in Sect. 5, Fig. 19 gives the long-term spatial dynamics of dogs under the homogeneous initial condition. Figures 20, 21, and 22 give the long-term spatial dynamics of dogs under the initial conditions with infections in city region, in wild region, and around the city-wild interface, respectively. Figure 23 compares nine different strengths of city-focused vaccinations, and Fig. 24 compares nine different strengths of wild-focused vaccinations. For the Gaussian-type diffusion in Sect. 6, the long-term dynamics of dogs under the homogeneous initial condition and the stepwise initial condition are given in Figs. 25 and 26, respectively. For model (6) with seasonal biting rate and city-wild diffusion, the spatially seasonal dynamics of dogs is given in Figure 27.

Fig. 17

Long-term spatial distribution of susceptible, exposed, infectious, and vaccinated dogs with homogeneous initial condition given in Sect. 4.1.1. $D_I(x)=5$, $k=0.09$, and other parameter values are the same as those in Table 1

Fig. 18

Long-term spatial distribution of susceptible, exposed, infectious and vaccinated dogs with stepwise initial condition given in Sect. 4.1.2. $D_I(x)=5$, $k=0.09$, and the other parameter values are the same as those in Table 1

Fig. 19

Long-term spatial distribution of susceptible, exposed, infectious, and vaccinated dogs with diffusion of infectious dogs in city and wild regions with homogeneous initial condition given in Sect. 5.1.1. $D_I(x)=\frac{13}{6}\arctan (x-50)+\frac{15}{4}$, $k=0.09$, and the other parameter values are the same as those in Table 1

Fig. 20

Long-term spatial distribution of susceptible, exposed, infectious, and vaccinated dogs with initial infections in the city region given in Sect. 5.1.2. $D_I(x)=\frac{13}{6}\arctan (x-50)+\frac{15}{4}$, $k=0.09$, and the other parameter values are the same as those in Table 1

Fig. 21

Long-term spatial distribution of susceptible, exposed, infectious and vaccinated dogs with initial infections in the wild region given in Sect. 5.1.3. $D_I(x)=\frac{13}{6}\arctan (x-50)+\frac{15}{4}$, $k=0.09$, and the other parameter values are the same as those in Table 1

Fig. 22

Long-term spatial distribution of susceptible, exposed, infectious and vaccinated dogs with initial infections around the interface between city and wild regions given in Sect. 5.1.4. $D_I(x)=\frac{13}{6}\arctan (x-50)+\frac{15}{4}$, $k=0.09$, and the other parameter values are the same as those in Table 1

Fig. 23

Long-term spatial distribution of susceptible, exposed, infectious, and vaccinated dogs with diffusion of infectious dogs in city and wild regions and city-focused vaccination $k=\alpha (-0.0327\arctan (x-50)+0.051)$. a $\alpha =0$; b $\alpha =1$; c $\alpha =2$; d $\alpha =3$; e $\alpha =4$; f $\alpha =5$; g $\alpha =6$; h $\alpha =7$; i $\alpha =8$. $D_I(x)=\frac{13}{6}\arctan (x-50)+\frac{15}{4}$, and the other parameter values are the same as those in Table 1. The initial condition is $S(x,0)=800$, $E(x,0)= 5$, $I(x,0)=2$, $R(x,0)=10$

Fig. 24

Long-term spatial distribution of susceptible, exposed, infectious, and vaccinated dogs with diffusion of infectious dogs in city and wild regions and wild-focused vaccination $k=\alpha (0.0327\arctan (x-50)+0.051)$. a $\alpha =0$; b $\alpha =1$; c $\alpha =2$; d $\alpha =3$; e $\alpha =4$; f $\alpha =5$; g $\alpha =6$; h $\alpha =7$; i $\alpha =8$. $D_I(x)=\frac{13}{6}\arctan (x-50)+\frac{15}{4}$, and the other parameter values are the same as those in Table 1. The initial condition is $S(x,0)=800$, $E(x,0)= 5$, $I(x,0)=2$, $R(x,0)=10$

Fig. 25

Long-term spatial distribution of susceptible, exposed, infectious and vaccinated dogs with homogeneous initial condition given in Sect. 4.1.1. $D_I(x)=2+\frac{50}{\sqrt{2\pi }}\exp \left( -\frac{(x-30)^2}{8}\right) +\frac{100}{3\sqrt{2\pi }}\exp \left( -\frac{(x-70)^2}{18}\right)$, $k=0.09$, and the other parameter values are the same as those in Table 1

Fig. 26

Long-term spatial distribution of susceptible, exposed, infectious and vaccinated dogs with stepwise initial condition given in Sect. 4.1.2. $D_I(x)=2+\frac{50}{\sqrt{2\pi }}\exp \left( -\frac{(x-30)^2}{8}\right) +\frac{100}{3\sqrt{2\pi }}\exp \left( -\frac{(x-70)^2}{18}\right)$, $k=0.09$, and the other parameter values are the same as those in Table 1

Fig. 27

Spatial distribution of dogs from the 30th to the 40th year. Here $\beta (t)=0.25\times (1+0.41\sin (2\pi t+5.5))$, $D_I(x)=\frac{13}{6}\arctan (x-50)+\frac{15}{4}$, $k=0.09$, and the other parameter values are the same as those in Table 1. The initial condition is $S(x,0)=800$, $E(x,0)=5$, $I(x,0)=1$, $R(x,0)=10$