A Mathematical Study to Control Visceral Leishmaniasis: An Application to South Sudan

Abstract

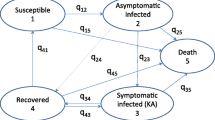
In this manuscript, we propose and analyze a compartmental model of visceral leishmaniasis (VL). We model the human population with six compartments including asymptomatic, symptomatic and PKDL-infected, animal population as second host and sandfly population as the vector. Furthermore, the non-adult stage of the sandfly population is introduced in the system, which was not considered before in the literature. We show that the increase in the number of host of sandfly population generates a backward bifurcation. Thus, multiple hosts will cause disease persistence even if the basic reproduction number ($R_{0}$) is below unity. We perform a sensitivity analysis of important model parameters with respect to some epidemiologically significant responses. We validate our model by calibrating it to weekly VL incidence data from South Sudan for the year 2013. We perform cost-effectiveness analysis on different interventions: treatment, non-adult control, adult control and their different layered combinations based on their implementation cost (in USD) and case reduction. We also use a global sensitivity analysis technique to understand the effect of important parameters of our model on the implementation cost of different controls. This cost-effectiveness study and cost–sensitivity analysis are relatively new in existing literature of this disease.

Appendices

Appendix 1

Proof of Lemma 1

System (1)–(5) can be rewritten in the following form:

$$\begin{aligned} \displaystyle \frac{{\hbox {d}}X}{{\hbox {d}}t} = \displaystyle AX + B, \end{aligned}$$

where $X = (S_\mathrm{H}, A_\mathrm{H}, K_\mathrm{H}, D_\mathrm{H}, P_\mathrm{H}, R_\mathrm{H}, S_\mathrm{A}, I_\mathrm{A}, F_\mathrm{NA}, S_{V}, I_{V})^\mathrm{T}$ and

$$\begin{aligned} A= & {} \left[ \begin{array}{ccccccccccc} \displaystyle -(\mu _\mathrm{H} + Q_\mathrm{H}) &{} 0 &{} 0 &{} 0 &{} 0 &{} &{} &{} &{} &{} &{}\\ \displaystyle \sigma bQ_\mathrm{H} &{} \displaystyle -(\mu _\mathrm{H} + \gamma _\mathrm{H}) &{} 0 &{} 0 &{} 0 &{} &{} &{} &{} &{} &{}\\ \displaystyle (1-\sigma ) Q_\mathrm{H} &{} 0 &{} \displaystyle -(\alpha _{1} + \delta +\mu _\mathrm{H}) &{} 0 &{} 0 &{} &{} &{} &{} &{} &{}\\ 0 &{} \displaystyle \gamma _\mathrm{H}\epsilon _{2} &{} \displaystyle \alpha _{1}\epsilon _{1} &{} \displaystyle -(\tau _\mathrm{H}+\mu _\mathrm{H}) &{} 0 &{} &{} &{} &{} &{} &{}\\ 0 &{} 0 &{} 0 &{} \displaystyle \tau _\mathrm{H} &{}\displaystyle -(\alpha _{2}+\mu _\mathrm{H}) &{} &{} &{} &{} &{} &{} \\ 0 &{} \displaystyle (1-\epsilon _{2})\gamma _\mathrm{H} &{} \displaystyle (1-\epsilon _{1})\alpha _{1} &{} 0 &{} \displaystyle \alpha _{2} &{} &{} &{} &{} &{} &{} \\ 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} &{} &{} &{} &{} &{} \\ 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} &{} &{} &{} &{} &{} \\ 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} &{} &{} &{} &{} &{} \\ 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} &{} &{} &{} &{} &{} \\ 0 &{} \displaystyle b p_{v}^{H} \frac{\lambda S_{V}}{N_\mathrm{H}} &{} \displaystyle b p_{v}^{H} \frac{S_{V}}{N_\mathrm{H}} &{} 0 &{} \displaystyle b p_{v}^{H} \frac{S_{V}}{N_\mathrm{H}} &{} &{} &{} &{} &{} &{}\\ \end{array}\right. \nonumber \\&\qquad \left. \begin{array}{ccccccccccc} &{} &{} &{} &{} &{} \displaystyle \beta &{} 0 &{} 0 &{} 0 &{} 0 &{} 0\\ &{} &{} &{} &{} &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} 0\\ &{} &{} &{} &{} &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} 0\\ &{} &{} &{} &{} &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} 0\\ &{} &{} &{} &{} &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 \\ &{} &{} &{} &{} &{} \displaystyle -(\beta + \mu _\mathrm{H}) &{} 0 &{} 0 &{} 0 &{} 0 &{} 0\\ &{} &{} &{} &{} &{} 0 &{} \displaystyle -\mu _\mathrm{A} - bp_\mathrm{A}^{v}\frac{I_{V}}{N_\mathrm{A}} &{} 0 &{} 0 &{} 0 &{} 0\\ &{} &{} &{} &{} &{} 0 &{} \displaystyle bp_\mathrm{A}^{v}\frac{I_{V}}{N_\mathrm{A}} &{} \displaystyle -\mu _\mathrm{A} &{} 0 &{} 0 &{} 0\\ &{} &{} &{} &{} &{} 0 &{} 0 &{} 0 &{} \displaystyle -(\eta + \mu _\mathrm{NA}) &{} a_{9} &{}a_{9} \\ &{} &{} &{} &{} &{} 0 &{} 0 &{} 0 &{} \displaystyle \eta &{} a_{10} &{} 0\\ &{} &{} &{} &{} &{} 0 &{} 0 &{} \displaystyle b p_{v}^{A} \frac{S_{V}}{N_\mathrm{A}} &{} 0 &{} 0 &{} \displaystyle - \mu _{F}\\ \end{array}\right] \\ \end{aligned}$$

where $Q_\mathrm{H}=b p_\mathrm{H}^{v} \frac{I_{V}}{N_\mathrm{H}}$, $a_{9}=\displaystyle r \kappa _{F}\left( 1- \frac{F_\mathrm{NA}}{C}\right)$, $a_{10}=\displaystyle -\mu _{F} - b p_{v}^{H} \frac{(K_\mathrm{H} + P_\mathrm{H} + \lambda A_\mathrm{H})}{N_\mathrm{H}} - b p_{v}^{A}\frac{I_\mathrm{A}}{N_\mathrm{A}}.$

The vector $\displaystyle B = (\pi _\mathrm{H},0,0,0,0,0,\pi _\mathrm{A},0,0,0,0)^\mathrm{T}$ is positive. Note that A(X) has all off-diagonal entries nonnegative, i.e., A(X) is a Metzler matrix for all $X \in \mathbb {R}_{+}^{11}$, since $B \ge 0$ system (1)–(5) is positively invariant in $\mathbb {R}_{+}^{11}$ (Abate et al. 2009). Therefore, any trajectory of the system (1)–(5) starting from an initial state in $\mathbb {R}_{+}^{11}$ remains trapped forever in $\mathbb {R}_{+}^{11}$.

Adding the first six equations of the model system (1)–(5), we get

$$\begin{aligned} \displaystyle \frac{{\hbox {d}}N_\mathrm{H}}{{\hbox {d}}t} = \varPi _\mathrm{H} - \mu _\mathrm{H}N_\mathrm{H} - \delta K_\mathrm{H} \le \varPi _\mathrm{H} - \mu _\mathrm{H}N_\mathrm{H}. \end{aligned}$$

Hence, $0 \le N_\mathrm{H}(t) \le \frac{\varPi _\mathrm{H}}{\mu _\mathrm{H}} + \left( N_\mathrm{H}(0) - \frac{\varPi _\mathrm{H}}{\mu _\mathrm{H}}\right) e^{-\mu _\mathrm{H} t}$. Therefore, as $t \rightarrow \infty$, $0 \le N_\mathrm{H}(t) \le \frac{\varPi _\mathrm{H}}{\mu _\mathrm{H}}$ and, for any $t>0$, $0 \le N_\mathrm{H}(t) \le Z_1$ , where $Z_1 = \max \left\{ \frac{\varPi _\mathrm{H}}{\mu _\mathrm{H}},N_\mathrm{H}(0)\right\}$.

By adding the equations in the $S_\mathrm{A}$ and $I_\mathrm{A}$ compartments of system (1)–(5), we get

$$\begin{aligned} \frac{{\hbox {d}}N_\mathrm{A}}{{\hbox {d}}t} = \varPi _\mathrm{A} - \mu _\mathrm{A}N_\mathrm{A}. \end{aligned}$$

By similar arguments, we have, for any $t>0$, $0 \le N_\mathrm{A}(t) \le Z_2$, where $Z_2 = \max \left\{ \frac{\varPi _\mathrm{A}}{\mu _\mathrm{A}},N_\mathrm{A}(0)\right\}$.

Assume that $Z_3=\max \left\{ C,F_\mathrm{NA}(0)\right\}$. Then $F_\mathrm{NA} \le Z_3$. Adding the equations in $S_{V}$ and $I_{V}$ compartments of system (1)–(5), we get:

$$\begin{aligned} \begin{array}{llll} \frac{{\hbox {d}}(S_{V} + I_{V})}{{\hbox {d}}t} &{}=&{} \eta F_\mathrm{NA} - \mu _{F}(S_{V} + I_{V}),\\ &{}\le &{} \eta Z_3 - \mu _{F}(S_{V} + I_{V}),\\ \end{array} \end{aligned}$$

which implies $0 \le (S_{V} + I_{V}) \le \frac{\eta Z_3}{\mu _{F}}$.

Therefore, all mathematically and biologically feasible solutions of the model system (1)–(5) enter the region $\varSigma$; i.e, $\varSigma$ is attracting. Hence, it is now sufficient to study the dynamical properties of the model (1)– (5) in $\varSigma$. $\square$

Appendix 2

Proof of Theorem 1

Assuming $M = S_{V} + I_{V}$, we consider the subsystem of the system (1)–(5) as follows:

$$\begin{aligned} \begin{array}{llll} \displaystyle \frac{{\hbox {d}}F_\mathrm{NA}}{{\hbox {d}}t} &{}=&{} \displaystyle r \kappa _{F} \left( 1- \frac{F_\mathrm{NA}}{C} \right) M - (\eta + \mu _\mathrm{NA}) F_\mathrm{NA},\\ \\ \displaystyle \frac{{\hbox {d}}M}{{\hbox {d}}t} &{}=&{} \displaystyle \eta F_\mathrm{NA} -\mu _{F} M.\\ \end{array} \end{aligned}$$

(17)

To study the global stability of $E_{0}$, we consider the following Lyapunov function for subsystem (17) in $\varSigma$:

$$\begin{aligned} \displaystyle V = \displaystyle \eta F_\mathrm{NA} + (\eta + \mu _\mathrm{NA})M. \end{aligned}$$

The total derivative of V is given by

$$\begin{aligned} \displaystyle \dot{V} = \displaystyle - \mu _{F}(\eta + \mu _\mathrm{NA})M\left[ \frac{F_\mathrm{NA}R_{C}}{C} + (1 - R_{C})\right] , \end{aligned}$$

since $R_{C} \le 1$, $\displaystyle \dot{V} \le 0$.

Also, $\dot{V} = 0$ if and only if $F_\mathrm{NA} = M = 0$. Applying Lasalle’s invariance principle (LaSalle 1968), we get $F_\mathrm{NA} \rightarrow 0$ and $M \rightarrow 0$ as $t \rightarrow \infty$.

Again, $S_{V} \ge 0$, $I_{V} \ge 0$ and $M = S_{V} + I_{V} \rightarrow 0$ as $t \rightarrow \infty$, so it follows that $S_{V} \rightarrow 0$ and $I_{V} \rightarrow 0$.

Substituting $F_\mathrm{NA} = S_{V} = I_{V} = 0$ in the original system (1)–(5), we get

$(S_\mathrm{H}, A_\mathrm{H}, K_\mathrm{H}, D_\mathrm{H}, P_\mathrm{H}, R_\mathrm{H}, S_\mathrm{A}, I_\mathrm{A}) \rightarrow \left( \frac{\pi _\mathrm{H}}{\mu _\mathrm{H}},0,0,0,0,0,\frac{\pi _\mathrm{A}}{\mu _\mathrm{A}},0\right)$ as $t \rightarrow \infty$.

As $\varSigma$ is positively invariant and attracting, the trivial vector-free equilibrium $E_{0}$ is globally asymptotically stable in $\varSigma$ if $R_{C} \le 1$. $\square$

Appendix 3

Proof of Theorem 2

Let us consider the bounded set

$$\begin{aligned} \displaystyle G= & {} \displaystyle \{( S_\mathrm{H}, A_\mathrm{H}, K_\mathrm{H}, D_\mathrm{H}, P_\mathrm{H}, R_\mathrm{H}, S_\mathrm{A}, I_\mathrm{A}, F_\mathrm{NA}, S_{V}, I_{V}) \in \mathbb {R}_{+}^{11} : \frac{\pi _\mathrm{H}}{\mu _\mathrm{H} + \delta } \\\le & {} S_\mathrm{H}+A_\mathrm{H}+K_\mathrm{H}+ D_\mathrm{H}+P_\mathrm{H}+R_\mathrm{H} \le \max \left\{ \frac{\varPi _\mathrm{H}}{\mu _\mathrm{H}},N_\mathrm{H}(0)\right\} ; \\ S_\mathrm{A}+I_\mathrm{A}\le & {} \max \left\{ \frac{\varPi _\mathrm{A}}{\mu _\mathrm{A}},N_\mathrm{A}(0)\right\} ;\\ F_\mathrm{NA}\le & {} Z_3; S_{V}+I_{V} \le \frac{\eta Z_3}{\mu _{F}}; Z_3=\max \{C,F_\mathrm{NA}(0)\}\}. \end{aligned}$$

The compartments of the uninfected individuals of the system (1)–(5) can be written in the following way

$$\begin{aligned} \displaystyle \frac{{\hbox {d}}X}{{\hbox {d}}t} = \displaystyle F(X,0), \end{aligned}$$

(18)

with $X = (S_\mathrm{H}, R_\mathrm{H}, S_\mathrm{A}, F_\mathrm{NA}, S_{V})^\mathrm{T}$.

From system (18), it is clear that $(S_\mathrm{H}, R_\mathrm{H}, S_\mathrm{A}) \rightarrow \left( \frac{\pi _\mathrm{H}}{\mu _\mathrm{H}},0,\frac{\pi _\mathrm{A}}{\mu _\mathrm{A}}\right)$ as $t \rightarrow \infty$.

The remaining two compartments of the system (18)) are

$$\begin{aligned} \begin{array}{llll} \displaystyle \frac{{\hbox {d}}F_\mathrm{NA}}{{\hbox {d}}t} &{}=&{} \displaystyle r \kappa _{F} \left( 1- \frac{F_\mathrm{NA}}{C} \right) S_{V} - (\eta + \mu _\mathrm{NA}) F_\mathrm{NA}\\ \\ \displaystyle \frac{{\hbox {d}}S_{V}}{{\hbox {d}}t} &{}=&{} \displaystyle \eta F_\mathrm{NA} -\mu _{F} S_{V}.\\ \end{array} \end{aligned}$$

(19)

Clearly, the non-trivial equilibrium $\bigg (C\left( 1 - \frac{1}{R_{C}}\right) ,\frac{\eta C}{\mu _{F}}\left( 1 - \frac{1}{R_{C}}\right) \bigg )$ is a stable node of the system (19). Also, a direct application of Bendixon’s nonexistence criterion shows that system (19) contains no closed path. Therefore, the equilibrium $(C(1 - \frac{1}{R_{C}}),\frac{\eta C}{\mu _{F}}(1 - \frac{1}{R_{C}}))$ of the system (19) is globally asymptotically stable. Thus, $E_{1}$ is globally asymptotically stable for system (18).

Therefore, for our model system (1)–(5) the first two conditions are satisfied. Furthermore, in G, $A_{2}^{0}$ can be written as follows:

$$\begin{aligned} \displaystyle A_{2}^{0} = \left[ \begin{array}{cccccc} \displaystyle -(\mu _\mathrm{H}+\gamma _\mathrm{H}) &{} 0 &{} 0 &{} 0 &{} 0 &{} \displaystyle \sigma b p_\mathrm{H}^{v} \\ 0 &{} \displaystyle -(\alpha _{1}+\delta +\mu _\mathrm{H}) &{} 0 &{} 0 &{} 0 &{} (1 - \sigma ) b p_\mathrm{H}^{v}\\ \displaystyle \gamma _\mathrm{H}\epsilon _{2} &{} \alpha _{1}\epsilon _{1} &{} -(\tau _\mathrm{H} + \mu _\mathrm{H}) &{} 0 &{} 0 &{} 0 \\ 0 &{} 0 &{} \tau _\mathrm{H} &{} -(\alpha _{2} + \mu _\mathrm{H}) &{} 0 &{} 0 \\ 0 &{} 0 &{} 0 &{} 0 &{} -\mu _\mathrm{A} &{} bp_\mathrm{A}^{v} \\ \lambda M_{1} &{} M_{1} &{} 0 &{} M_{1} &{} bp_{v}^{A}\frac{\eta C\mu _\mathrm{A}}{\mu _{F}\pi _\mathrm{A}} &{} -\mu _{F} \\ \end{array} \right] , \end{aligned}$$

with $\displaystyle M_{1} = \displaystyle bp_{v}^{H}\frac{\eta C\pi _\mathrm{H}}{\mu _{F}(\mu _\mathrm{H}+\delta )}$.

We can write $A_{2}^{0} = Q + P$, where Q and P have the following forms:

$$\begin{aligned} \displaystyle Q = \left[ \begin{array}{cccccc} \displaystyle -(\mu _\mathrm{H}+\gamma _\mathrm{H}) &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 \\ 0 &{} \displaystyle -(\theta \alpha _{1}+\delta +\mu _\mathrm{H}) &{} 0 &{} 0 &{} 0 &{} 0\\ \displaystyle \gamma _\mathrm{H}\epsilon _{2} &{} \alpha _{1}\epsilon _{1} &{} -(\tau _\mathrm{H} + \mu _\mathrm{H}) &{} 0 &{} 0 &{} 0 \\ 0 &{} 0 &{} \tau _\mathrm{H} &{} -(\alpha _{2} + \mu _\mathrm{H}) &{} 0 &{} 0 \\ 0 &{} 0 &{} 0 &{} 0 &{} -\mu _\mathrm{A} &{} 0 \\ 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} -\mu _{F} \\ \end{array} \right] \end{aligned}$$

and

$$\begin{aligned} \displaystyle P = \left[ \begin{array}{cccccc} \displaystyle 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} \displaystyle \sigma b p_\mathrm{H}^{v} \\ 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} (1 - \sigma ) b p_\mathrm{H}^{v}\\ 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 \\ 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 \\ 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} bp_\mathrm{A}^{v} \\ \lambda M_{1} &{} M_{1} &{} 0 &{} M_{1} &{} bp_{v}^{A}\frac{\eta C\mu _\mathrm{A}}{\mu _{F}\pi _\mathrm{A}} &{} 0 \\ \end{array} \right] , \end{aligned}$$

with $\displaystyle M_{1} = \displaystyle bp_{v}^{H}\frac{\eta C\pi _\mathrm{H}}{\mu _{F}(\mu _\mathrm{H}+\delta )}$

Q is Metzler stable and $P \ge 0$. Therefore, using Proposition 3.3 of Kamgang and Sallet (2008), we get $\alpha (A_{2}^{0}) \le 0 \Leftrightarrow \rho (-PQ^{-1})\le 1$.

After a few calculations, we get $\rho (-PQ^{-1})\le 1$, giving $R_{*} \le 1$, with

$$\begin{aligned} \begin{array}{llll} \displaystyle R_{*}^{2} &{}=&{} \displaystyle \frac{R_{C}(\mu _\mathrm{H} + \delta )}{\mu _\mathrm{H}(R_{C} - 1)}R_{0}^{2} +\frac{b^{2}p_\mathrm{A}^{v}p_{v}^{A}\eta }{\mu _{F}^{2}\pi _\mathrm{A}R_{C}}.\\ \end{array} \end{aligned}$$

(20)

From the expression (20), it is clear that $R_{*}^{2} > R_{0}^{2}$. Therefore, if $R_{C} > 1$ and $R_{0}< R_{*} < 1$, then the non-trivial disease-free equilibrium $E_{1}$ is globally asymptotically stable in G. $\square$

Appendix 4

Proof of Theorem 3

Putting the values of $I_{V}^{*}$ and $N_\mathrm{H}^{*}$ in the expression

$$\begin{aligned} \displaystyle \lambda _\mathrm{H} =\displaystyle \frac{b p_\mathrm{H}^{v} I_{V}^{*}}{N_\mathrm{H}^{*}}, \end{aligned}$$

we get a quadratic equation in $\lambda _\mathrm{H}$:

$$\begin{aligned} \displaystyle A\lambda _\mathrm{H}^{2} + B \lambda _\mathrm{H} + C = 0, \end{aligned}$$

where

$$\begin{aligned} \displaystyle A= & {} \displaystyle -\mu _{F}\varPi _\mathrm{H}[(\alpha _{1}+\delta +\mu _\mathrm{H})(1-\beta F_{1})-\delta (1-\sigma )]\\&\qquad \left[ ( \alpha _{1}+\delta +\mu _\mathrm{H})\left( \mu _{F}(1-\beta F_{1})+\frac{\mu _{F}^{2}\varPi _\mathrm{H}R_{0}^{2}}{b p_\mathrm{H}^{v}\eta F_\mathrm{NA}^{*}}\right) - \delta (1-\sigma )\right] ,\\ \displaystyle B= & {} \displaystyle -\mu _\mathrm{H}\mu _{F}^{2}\varPi _\mathrm{H}(\alpha _{1}+\delta +\mu _\mathrm{H})^{2}\\&\qquad \left[ 2(1-\beta F_{1}) +\frac{\mu _{F} \varPi _\mathrm{H}R_{0}^{2}}{b p_\mathrm{H}^{v}\eta F_\mathrm{NA}^{*}} - \frac{2\delta (1-\sigma )}{( \alpha _{1}+\delta +\mu _\mathrm{H})} - \frac{R_{0}^{2}(1-\beta F_{1})}{F_\mathrm{NA}^{*}}\right] ,\\ \displaystyle C= & {} \mu _\mathrm{H}^{2}\mu _{F}^{2}\varPi _\mathrm{H}( \alpha _{1}+\delta +\mu _\mathrm{H})^{2}(R_{0}^{2}-1). \end{aligned}$$

When $\delta = 0$ and $\beta = 0$, $\displaystyle N_\mathrm{H}^{*}= \frac{\varPi _\mathrm{H}}{\mu _\mathrm{H}}$. The expression of $\lambda _\mathrm{H}$ takes the form

$$\begin{aligned} \displaystyle \lambda _\mathrm{H} =\displaystyle \frac{b p_\mathrm{H}^{v}\mu _\mathrm{H}\eta F_\mathrm{NA}^{*}(R_{0}^{2}-1)}{b p_\mathrm{H}^{v}\eta F_\mathrm{NA}^{*} + \mu _{F}\varPi _\mathrm{H}R_{0}^{2}}. \end{aligned}$$

Since $F_\mathrm{NA}^{*} > 0$ for $R_{C}>1$, it follows that $\lambda _\mathrm{H}>0$ if and only if $R_{0}>1$. Hence Case 1 follows.

Similarly, when $\delta = 0$ and $\beta > 0$, the expression of $\lambda _\mathrm{H}$ is obtained as follows

$$\begin{aligned} \displaystyle \lambda _\mathrm{H} =\displaystyle \frac{b p_\mathrm{H}^{v}\mu _\mathrm{H}\eta F_\mathrm{NA}^{*}(R_{0}^{2}-1)}{b p_\mathrm{H}^{v}\eta F_\mathrm{NA}^{*}(1-\beta F_{1}) + \mu _{F}\varPi _\mathrm{H}R_{0}^{2}}, \end{aligned}$$

which is positive if $R_{0}>1$ and $\beta F_{1} < 1$ or if $R_{0}>1$ and $\displaystyle \beta F_{1}> 1 + \frac{\mu _{F} \varPi _\mathrm{H} R_{0}^{2}}{b p_\mathrm{H}^{v} \eta F_\mathrm{NA}^{*}}$. This proves Case 2.

Finally, when $\delta > 0$ and $\beta > 0$,

$$\begin{aligned} \displaystyle A\lambda _\mathrm{H}^{2} + B \lambda _\mathrm{H} + C = 0 . \end{aligned}$$

Note that $\lambda _\mathrm{H}$ always possesses a positive solution if $C > 0$ and $A < 0$ or if $C < 0$ and $A > 0$. Clearly, $C > 0$ if $R_{0}>1$ and $A < 0$ if $\sigma< \beta F_{1} < 1 - \frac{\delta (1-\sigma )}{( \alpha _{1}+\delta +\mu _\mathrm{H})}$.

Also $A < 0$ if $R_{0}^{2} < \displaystyle \frac{b p_\mathrm{H}^{v} \eta F_\mathrm{NA}^{*}}{\mu _{F} \varPi _\mathrm{H}} \left[ \frac{\delta (1-\sigma ) }{ \alpha _1 + \delta + \mu _\mathrm{H}} +(\beta F_{1} -1) \right]$.

This proves Case 3a(i) and Case 3a(ii).

Again $C < 0$ if $R_{0}<1$ and $A > 0$ if $1 - \frac{\delta (1-\sigma ) }{ \alpha _1 + \delta + \mu _\mathrm{H}}< \beta F_{1} < \displaystyle 1+ \frac{\mu _{F} \varPi _\mathrm{H} R_{0}^{2}}{b p_\mathrm{H}^{v} \eta F_\mathrm{NA}^{*}}$; i.e, if Case 3a(iii) is satisfied.

If $B^{2}>4AC$, then, depending on the sign of B, the cases when two endemic equilibria can occur are given by $B<0,A>0,C>0$ and $B>0,A<0,C<0$. It is clear from the expressions of A, B and C that the above two inequalities hold only when Case 3b(i) and Case 3b(ii) are satisfied, respectively. This completes the proof. $\square$

Appendix 5

Proof of Theorem 4

For $\delta =0$, putting the values of $I_{V}^{*}$ and $N_\mathrm{H}^{*}$ in the expression

$$\begin{aligned} \displaystyle \lambda _\mathrm{H} =\displaystyle \frac{b p_\mathrm{H}^{v} I_{V}^{*}}{N_\mathrm{H}^{*}}, \end{aligned}$$

we get a quadratic equation in $\lambda _\mathrm{H}$:

$$\begin{aligned} \displaystyle A_{0}\lambda _\mathrm{H}^{2} + B_{0} \lambda _\mathrm{H} + C_{0} = 0 , \end{aligned}$$

(21)

where

$$\begin{aligned} A_{0}&= -\mu _{F}\varPi _\mathrm{H}^{2}p_\mathrm{A}^{v}\left[ (1-\beta F_{1})(\mu _{F}+bp_{v}^{A})+\frac{\mu _{F}^{2}\varPi _\mathrm{H}R_{0}^{2}}{bp_\mathrm{H}^{v}\eta F_\mathrm{NA}^{*}}-\frac{bp_\mathrm{A}^{v}p_{v}^{A}\varPi _\mathrm{H}}{\varPi _\mathrm{A}p_\mathrm{H}^{v}}\right] ,\\ B_{0}&= \varPi _\mathrm{H}^{2}\mu _{F}^{2}\mu _\mathrm{H}p_\mathrm{A}^{v}(R_{0}^{2}-1)+\varPi _\mathrm{H}b^{2}p_\mathrm{A}^{v}p_{v}^{A}\mu _\mathrm{H}\eta F_\mathrm{NA}^{*}\left[ p_\mathrm{H}^{v}(1-\beta F_{1})-\frac{\varPi _\mathrm{H}p_\mathrm{A}^{v}}{\varPi _\mathrm{A}}\right] \\&\qquad -\varPi _\mathrm{H}\mu _\mathrm{H}\mu _{F}^{2}\varPi _\mathrm{A}\left[ p_\mathrm{H}^{v}(1-\beta F_{1})+\frac{\mu _{F}\varPi _\mathrm{H}R_{0}^{2}}{b\eta F_\mathrm{NA}^{*}}\right] ,\\ C_{0}&= \mu _{F}^{2}\mu _\mathrm{H}\varPi _\mathrm{H}\varPi _\mathrm{A}p_\mathrm{H}^{v}(R_{0}^{2}-1). \end{aligned}$$

when $\delta =0$ and $\beta =0$, it is clear that model (1)–(5) has exactly one endemic equilibrium whenever $R_{0}>1$ and has two endemic equilibria whenever $B_{0}>0$, $R_{0} < 1$ and $B_{0}^{2}>4A_{0}C_{0}$. Hence Case 1 follows.

For $\delta =0$ and $\beta >0$, it follows that $\lambda _\mathrm{H}$ always possesses a positive solution if $C_{0} > 0$ and $A_{0} < 0$ or if $C_{0} < 0$ and $A_{0} > 0$. From the expressions of $A_{0}$, $B_{0}$ and $C_{0}$, we find that $A_{0} < 0$ if $\beta F_{1}<1$ and $C_{0} > 0$ if $R_{0}>1$.

Again $A_{0} > 0$ if $\displaystyle \beta F_{1}> 1 + \frac{1}{\mu _\mathrm{H}+p_{v}^{A}}\left( \frac{\mu _{F}^{2} \varPi _\mathrm{H} R_{0}^{2}}{b p_\mathrm{H}^{v} \eta F_\mathrm{NA}^{*}}-\frac{bp_\mathrm{A}^{v}p_{v}^{A}\varPi _\mathrm{H}}{\varPi _\mathrm{H}p_\mathrm{H}^{v}}\right)$ and $C_{0} < 0$ if $R_{0}^{2}< 1$.

This proves Case 2a. If $B_{0}^{2}>4A_{0}C_{0}$, model (1)–(5) possesses two endemic equilibria whenever $B_{0}<0$, $A_{0}>0$, $C_{0}>0$ or $B_{0}>0$, $A_{0}<0$, $C_{0}<0$. It is clear from the expressions of $A_{0}$, $B_{0}$ and $C_{0}$ that the above two inequalities hold only when Case 2b(i) and Case 2b(ii) are satisfied, respectively. This completes the proof. $\square$