Modeling the Capacitive Deionization Process in Dual-Porosity Electrodes

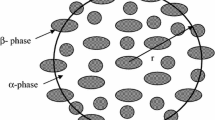
Abstract

In many areas of the world, there is a need to increase water availability. Capacitive deionization (CDI) is an electrochemical water treatment process that can be a viable alternative for treating water and for saving energy. A model is presented to simulate the CDI process in heterogeneous porous media comprising two different pore sizes. It is based on a theory for capacitive charging by ideally polarizable porous electrodes without Faradaic reactions or specific adsorption of ions. A two steps volume averaging technique is used to derive the averaged transport equations in the limit of thin electrical double layers. A one-equation model based on the principle of local equilibrium is derived. The constraints determining the range of application of the one-equation model are presented. The effective transport parameters for isotropic porous media are calculated solving the corresponding closure problems. The source terms that appear in the average equations are calculated using theoretical derivations. The global diffusivity is calculated by solving the closure problem.

Appendices

Appendix 1

1.1 Constraints for Salt Concentration One-Equation Model

An approximate form for the two-equation model can be written using the following form of the concentration deviations (Quintard and Whitaker 1993b):

$$\begin{aligned} {\tilde{c}}_{i}= & {} {\underline{{g}}}_{i} \,{\bullet }\,\underline{\nabla } \langle {c}_{\kappa } \rangle ^{{\kappa }}+{\underline{{h}}}_{i} \,{\bullet }\,\underline{\nabla } \langle {c}_{\gamma } \rangle ^{{\gamma }}+{s}_{i} (\langle {c}_{\kappa } \rangle ^{{\kappa }}-\langle {c}_{\gamma } \rangle ^{{\gamma }}) + \cdots +\cdots + \psi _i, i = \kappa , \gamma .\qquad \end{aligned}$$

(103)

Introducing (103) into Eqs. (21) and (27) we get,

$$\begin{aligned} \varepsilon _\kappa (1+\langle {F}_1 \rangle ) \partial \langle {c}_{\kappa } \rangle ^{{\kappa }}/\partial t= & {} \underline{\nabla } \,{\bullet }\,\left\{ \varepsilon _\kappa \underline{\underline{D}}_{\kappa \kappa }^{*} \,{\bullet }\,\underline{\nabla } \langle {c}_{\kappa } \rangle ^{{\kappa }}\right\} +\underline{\nabla } \,{\bullet }\,\left\{ \varepsilon _\kappa \underline{\underline{D}}_{\kappa \gamma }^{*} \,{\bullet }\,\underline{\nabla } \langle {c}_{\gamma } \rangle ^{{\gamma }}\right\} \nonumber \\&-\, \varepsilon _\kappa \langle {F}_2 \rangle \partial \langle \phi _{\kappa } \rangle ^{{\kappa }}/\partial t + \frac{{1}}{{V}_\mathrm{M}} \int \limits _{{A}_{{\gamma \kappa }}} {{\underline{{n}}}_{{\kappa \gamma }} \,{\bullet }\,{\underline{\underline{D}}}_{\mathrm{eff}} \,{\bullet }\, \underline{\nabla }{c}_{\kappa } \,{\hbox {d}A}}. \end{aligned}$$

(104)

$$\begin{aligned} \varepsilon _\gamma \partial \langle {c}_{\gamma } \rangle ^{{\gamma }}/\partial t= & {} \underline{\nabla } \,{\bullet }\,\left\{ \varepsilon _\gamma \underline{\underline{D}}_{\gamma \gamma }^{*} \,{\bullet }\,\underline{\nabla } \langle {c}_{\gamma } \rangle ^{{\gamma }}\right\} +\underline{\nabla } \,{\bullet }\,\left\{ \varepsilon _\gamma \underline{\underline{D}}_{\gamma \kappa }^{*} \,{\bullet }\,\underline{\nabla } \langle {c}_{\kappa } \rangle ^{{\kappa }}\right\} \nonumber \\&+\, \frac{{1}}{{V}_\mathrm{M}} \int \limits _{{A}_{{\gamma \kappa }}} {{\underline{{n}}}_{{\gamma \kappa }} \,{\bullet }\,{D}_{\gamma } \underline{\underline{I}} \underline{\nabla }{c}_{\gamma } \,{\hbox {d}A}}. \end{aligned}$$

(105)

The $\underline{\underline{D}}_{ij}^{*}$ tensors are calculated in the usual way as combinations of a transport coefficient and the integral of a closure parameter.

We follow Whitaker (1999) defining:

$$\begin{aligned} \langle {c}_\kappa \rangle ^{\kappa }=\langle {c}\rangle ^{{*}} +{ \hat{{c}}}_{k}, \end{aligned}$$

(106)

$$\begin{aligned} \langle {c}_{\gamma } \rangle ^{{\gamma }}=\langle {c}\rangle ^{{*}} +{ \hat{{c}}}_{\gamma }. \end{aligned}$$

(107)

Summation of Eqs. (104) and (105) plus introduction of (106) and (107) into the resulting equations leads after algebraic manipulations to,

$$\begin{aligned} (1+\varepsilon _\kappa \langle {F}_1 \rangle ) \partial \langle {c}\rangle ^{{*}}/\partial t= & {} \underline{\nabla } \,{\bullet }\,\left\{ \underline{\underline{{D}}}^{*} \,{\bullet }\,\underline{\nabla } \langle {c}\rangle ^{{*}}\right\} - \varepsilon _\kappa \langle {F}_2 \rangle \partial \langle \phi \rangle ^{{*}}/\partial t - \varepsilon _\kappa \langle {F}_1 \rangle \partial { \hat{{c}}}_{k} /\partial t\nonumber \\&+\,\underline{\nabla } \,{\bullet }\,\left\{ \varepsilon _\kappa (\underline{\underline{D}}_{\kappa \kappa }^{*} -\underline{\underline{D}}_{\gamma \gamma }^{*} )\,{\bullet }\,\underline{\nabla } { \hat{{c}}}_{k} \right\} - \varepsilon _\kappa \langle {F}_2 \rangle \partial \hat{{\phi }}_{k} /\partial t. \end{aligned}$$

(108)

Comparison of Eq. (108) with (44) shows that the last three terms in the right-hand side of Eq. (108) should be negligible for the one-equation model to be applicable, therefore:

• (I) $\varepsilon _\kappa \langle {F}_2 \rangle \partial \hat{{\phi }}_{k} /\partial t\ll \varepsilon _\kappa \langle {F}_2 \rangle \partial \langle \phi \rangle ^{{*}}/\partial t$

• (II) $\varepsilon _\kappa \langle {F}_1 \rangle \partial { \hat{{c}}}_{k} /\partial t \ll (1 + \varepsilon _\kappa \langle {F}_1 \rangle ) \partial \langle {c}\rangle ^{{*}}/\partial t$

• (III) $\underline{\nabla } \,{\bullet }\,\left\{ \varepsilon _\kappa (\underline{\underline{D}}_{\kappa \kappa }^{*} -\underline{\underline{D}}_{\gamma \gamma }^{*} )\,{\bullet }\,\underline{\nabla } { \hat{{c}}}_{k} \right\} \ll \underline{\nabla } \,{\bullet }\,\left\{ \underline{\underline{{D}}}^{*} \,{\bullet }\,\underline{\nabla } \langle {c}\rangle ^{{*}}\right\}$.

The first constraint will be discussed in “Appendix 2”. We will carry on (II) and (III) an order of magnitude analysis. We will use the assumptions that the characteristic length scales for the deviation $({ \hat{{c}}}_{k} )$ and for the spatial average variable $(\langle {c}\rangle ^{{*}})$ are of the same order plus that the characteristic times for variation of the deviations of salt concentration and potential are also of the same order. After algebraic manipulations we get,

• (IV) $\hat{{\phi }}_{k} /\Delta \langle \phi \rangle ^{{*}}\ll 1$

• (V) ${ \hat{{c}}}_{k} /\Delta \langle {c}\rangle ^{{*}}\ll 1$

• (VI) $\varepsilon _\kappa (\underline{\underline{D}}_{\kappa \kappa }^{*} -\underline{\underline{D}}_{\gamma \gamma }^{*} )/\underline{\underline{{D}}}^{*} { \hat{{c}}}_{k} /\Delta \langle {c}\rangle ^{{*}}\ll 1$.

Considering that $\varepsilon _{\kappa }\;(\underline{\underline{D}}_{\kappa \kappa }^{*} -\underline{\underline{D}}_{\gamma \gamma }^{*} )/ \underline{\underline{{D}}}^{*}\le \odot \left\{ 1\right\}$ leads to the conclusion that the condition of local equilibrium will be dominated by the value of ${\hat{{c}}}_{k}/\Delta \langle {c}\rangle ^{{*}}$. In conclusion, we need to estimate the ${\hat{{c}}}_{k}/\Delta \langle {c}\rangle ^{{*}}$ ratio to determine application conditions for the one-equation model. In order to do so we start from Eqs. (104) and (105). We first, follow Quintard and Whitaker (1993b) replacing the exchange terms in both equations by a mass transfer term as shown below. Second, we subtract Eq. (105) divided by $\varepsilon _{\gamma }$ from Eq. (104) divided by $\varepsilon _{\kappa }$ to get,

$$\begin{aligned}&\left( 1 +\varepsilon _\gamma \langle {F}_1 \rangle \right) \partial \left[ \langle {c}_{\kappa } \rangle ^{{\kappa }}-\langle {c}_\gamma \rangle ^{\gamma }\right] /\partial t -\underline{\nabla } \,{\bullet }\,\left\{ {\underline{\underline{D}}}^\circ \,{\bullet }\,\underline{\nabla } \left[ \langle {c}_{\kappa } \rangle ^{{\kappa }}-\langle {c}_\gamma \rangle ^{\gamma }\right] \right\} \nonumber \\&\qquad +\, a_\mathrm{vM} k\varepsilon *\left[ \langle {c}_{\kappa } \rangle ^{{\kappa }}-\langle {c}_\gamma \rangle ^{\gamma }\right] \nonumber \\&\quad = -\langle {F}_1 \rangle \partial \langle {c}\rangle ^{{*}}/\partial t+\underline{\nabla } \,{\bullet }\,\left\{ \left( \underline{\underline{D}}_{\kappa \kappa }^{*} -\underline{\underline{D}}_{\gamma \gamma }^{*} \right) \,{\bullet }\,\underline{\nabla } \langle {c}\rangle ^{{*}}\right\} -\langle {F}_2 \rangle \partial \langle \phi _\kappa \rangle ^{\kappa }/\partial t. \end{aligned}$$

(109)

Here, ${\underline{\underline{D}}}^\circ =\left\{ \varepsilon _\gamma \underline{\underline{D}}_{\kappa \kappa }^{*} - \underline{\underline{D}}_{\kappa \gamma }^{*} + \varepsilon _\kappa \underline{\underline{D}}_{\gamma \gamma }^{*} \right\} ,\varepsilon ^*= 1/\left( {\varepsilon _\kappa \varepsilon _\gamma } \right) , k$ is a mass transfer coefficient calculated from the closure problem for the parameters $s_{i}$,

$$\begin{aligned} k ={-}\frac{{1}}{{{ A}}_{{\gamma \kappa }}} \int \limits _{{A}_{{\gamma \kappa }}} {{\underline{{n}}}_{{\kappa \gamma }} \,{\bullet }\,{\underline{\underline{D}}}_{\mathrm{eff}} \,{\bullet }\, \underline{\nabla }{s}_{\kappa } \,{\hbox {d}A}} = \frac{{1}}{{A}_{{\gamma \kappa }}} \int \limits _{{A}_{{\gamma \kappa }}} {{\underline{{n}}}_{{\gamma \kappa }} \,{\bullet }\,{D}_{\gamma } \underline{\nabla }{s}_{\gamma } \,{\hbox {d}A}}. \end{aligned}$$

(110)

An order of magnitude analysis is conducted in all terms in Eq. (109). The absolute value of all estimates is combined to produce the following estimate:

$$\begin{aligned} \frac{{\left[ \langle {{c}}_{{{\kappa }}} \rangle ^{{{\kappa }}} - \langle {{c}}_{\gamma } \rangle ^{\gamma } \right] }}{{\Delta \langle {{c}}\rangle ^{*}}}= & {} {{\odot }}\left\{ \left[ {\frac{{{{-}}\langle {{F}}_{1} \rangle /{{t}}^{*} + \left( \underline{\underline{{{D}}}}_{{\kappa \kappa }}^{*} {{-}}\underline{\underline{{{D}}}}_{{\gamma \gamma }}^{*} \right) /L_{{{c}}} {{/L}}_{{\Delta {{c}}}} {{-}}\langle {{F}}_{2} \rangle \Delta \langle \phi _{\kappa } \rangle ^{\kappa } {{/}}\Delta \langle {{c}}\rangle ^{*} {{/ t}}^{*} }}{{\left( 1 + \varepsilon _{\gamma } \langle {{F}}_{1} \rangle \right) /{{t}}^{*} {{- (}}\underline{\underline{{{D}}}}^{\circ } {{/L}}_{{{{cd}}}} {{/L}}_{{\Delta {{cd}}}} + {{a}}_{{{\mathrm{vM}}}} {{k}}^{*} \varepsilon ^{*} }}} \right] \right\} .\nonumber \\ \end{aligned}$$

(111)

Here, we can use, ${t}^{{*}}\cong L_c L_{\Delta c} /\underline{\underline{D}}^{{*}}\cong L_c L_{\Delta c} /(\underline{\underline{D}}_{\kappa \kappa }^{*} -\underline{\underline{D}}_{\gamma \gamma }^{*} )\cong L_{cd} L_{\Delta cd} /{\underline{\underline{D}}}^\circ$, to get

$$\begin{aligned}&\frac{{\left[ \langle {{c}}_{{{\kappa }}} \rangle ^{{{\kappa }}} - \langle {{c}}_{\gamma } \rangle ^{\gamma } \right] }}{{\Delta \langle {{c}}\rangle ^{*}}}~ = {{\odot }}\left\{ \left[ {\frac{{{{-}}\langle {{F}}_{1} \rangle + 1{{-}}\langle {{F}}_{2} \rangle \langle \phi _{\kappa } \rangle ^{\kappa } /\Delta \langle {{c}}\rangle ^{*}}}{{(1 + \varepsilon _{\gamma } \langle {{F}}_{1} \rangle ){{-}}1 + {{a}}_{{{\mathrm{vM}}}} {{k}}^{*} \varepsilon ^{*} {{t}}^{*} }}} \right] \right\} ,\hbox {or}\end{aligned}$$

(112)

$$\begin{aligned}&\frac{{l_{k}^{2}}}{{L^{2}}}\left[ {\frac{{{{-}}\langle {{F}}_{1} \rangle + 1{{-}}\langle {{F}}_{2} \rangle \langle \phi _{\kappa } \rangle ^{\kappa } /\Delta \langle {{c}}\rangle ^{*}}}{{\frac{{l_{k}^{2} \varepsilon _{\gamma } \langle {{F}}_{1} \rangle }}{{L^{2}}} + 1}}} \right] \ll 1. \end{aligned}$$

(113)

Here, $L^{2}$ is the transport process characteristic length, $L^{2}\cong \,L_{c}\,L_{\Delta c}, l_k^2={{D}_{{xx}}^{*}}/{a_\mathrm{vM} k\varepsilon ^{{*}}}$ is a mixed length scale combining interfacial and mass transport. The area per unit volume $(a_\mathrm{vM})$ is of $\odot \left\{ 1/l_{\gamma \kappa }\right\}$, with $l_{\gamma \kappa }$ a combined small geometrical length scale. It is easy to demonstrate that $a_\mathrm{vM} \rightarrow \infty$ as $l_{\gamma \kappa }$ tends toward zero (Quintard and Whitaker 1995), thus local equilibrium occurs when $l_{\gamma \kappa }$ is very small.

Appendix 2

1.1 Constraints for Electrostatic Potential One-Equation Model

An approximate form for the two-equation model can be written using the following form of the potential deviations (del Rìo and Whitaker 2000b):

$$\begin{aligned} {\tilde{\phi }}_{i} ={\underline{{g}}}_{i} \,{\bullet }\,\underline{\nabla } \langle \phi _{\kappa } \rangle ^{{\kappa }}+{\underline{{h}}}_{i} \,{\bullet }\,\underline{\nabla } \langle \phi _{\gamma } \rangle ^{{\gamma }}+{s}_{i} (\langle \phi _{\kappa } \rangle ^{{\kappa }}-\langle \phi _{\gamma } \rangle ^{{\gamma }}) + \cdots +\cdots + \psi _i, i = \kappa , \gamma .\qquad \end{aligned}$$

(114)

Introducing (114) into Eqs. (50) and (56) we get,

$$\begin{aligned} \varepsilon _\kappa \langle {G}_2 \rangle ^{}\partial \langle \phi _{\kappa } \rangle ^{{\kappa }}/\partial t= & {} \underline{\nabla } \,{\bullet }\,\left\{ \varepsilon _\kappa \underline{\underline{{U}}}_{\kappa \kappa }^{*} \,{\bullet }\,\langle {c}_{\kappa } \rangle ^{{\kappa }}\underline{\nabla } \langle \phi _{\kappa } \rangle ^{{\kappa }}\right\} +\underline{\nabla } \,{\bullet }\,\left\{ \varepsilon _\kappa \underline{\underline{{U}}}_{\kappa \gamma }^{*} \,{\bullet }\,\langle {c}_{\kappa } \rangle ^{{\kappa }}\underline{\nabla } \langle \phi _{\gamma } \rangle ^{{\gamma }}\right\} \nonumber \\&+\, \frac{{1}}{{V}_\mathrm{M}} \int \limits _{{A}_{{\gamma \kappa }}} {{\underline{{n}}}_{{\kappa \gamma }} \,{\bullet }\,{\underline{\underline{{U}}}}_{\mathrm{eff}} \,{\bullet }\,{c}_{\kappa } \underline{\nabla }\phi _{\kappa } \,{\hbox {d}A}} - \varepsilon _\kappa \langle {G}_1 \rangle ^{}\partial \langle {c}_{\kappa } \rangle ^{{\kappa }}/\partial t. \end{aligned}$$

(115)

$$\begin{aligned} 0= & {} \underline{\nabla } \,{\bullet }\,\left\{ \varepsilon _\gamma \underline{\underline{{U}}}_{\gamma \gamma }^{*} \,{\bullet }\,\langle {c}_{\gamma } \rangle ^{{\gamma }}\underline{\nabla } \langle \phi _{\gamma } \rangle ^{{\gamma }}\right\} +\underline{\nabla } \,{\bullet }\,\left\{ \varepsilon _\gamma \underline{\underline{{U}}}_{\gamma \kappa }^{*} \,{\bullet }\,\langle {c}_{\gamma } \rangle ^{{\gamma }}\underline{\nabla } \langle \phi _{\kappa } \rangle ^{{\kappa }}\right\} \nonumber \\&+\, \frac{{1}}{{V}_\mathrm{M}} \int \limits _{{A}_{{\gamma \kappa }}} {{\underline{{n}}}_{{\gamma \kappa }} \,{\bullet }\,{U}_{\gamma } {c}_{\gamma } \underline{\nabla }\phi _{\gamma } \,{\hbox {d}A}}. \end{aligned}$$

(116)

We follow Whitaker (1999) defining:

$$\begin{aligned} \langle \phi _\kappa \rangle ^{\kappa }= & {} \langle \phi \rangle ^{{*}} + \hat{{\phi }}_{k}, \end{aligned}$$

(117)

$$\begin{aligned} \langle \phi _{\gamma } \rangle ^{{\gamma }}= & {} \langle \phi \rangle ^{{*}} + \hat{{\phi }}_{\gamma }. \end{aligned}$$

(118)

The same procedure used in “Appendix 1” produces the following estimate:

$$\begin{aligned}&\displaystyle \frac{[\langle \phi _{\kappa } \rangle ^{{\kappa }}-\langle \phi _\gamma \rangle ^{\gamma }]}{\Delta \langle \phi \rangle ^{{*}}}= \odot \left\{ \left[ {\frac{{-}\langle {G}_2 \rangle +\langle {c}\rangle ^{{*}}{-}\langle {G}_1 \rangle \langle {c}\rangle ^{{*}}/\Delta \langle \phi \rangle ^{{*}}}{\varepsilon _\gamma \langle {G}_2 \rangle {-}\varepsilon _\gamma \langle {c}\rangle ^{{*}}+{a}_{\mathrm{vM}} { k}\varepsilon ^{{*}}{t}^{{*}}\langle {c}\rangle ^{{*}}}} \right] \right\} , \hbox {or} \end{aligned}$$

(119)

$$\begin{aligned}&\displaystyle \left[ {\frac{{-}\langle {G}_2 \rangle +\langle {c}\rangle ^{{*}}{-}\langle {G}_1 \rangle \langle {c}\rangle ^{{*}}/\Delta \langle \phi \rangle ^{{*}}}{\varepsilon _\gamma \langle {G}_2 \rangle {-}\varepsilon _\gamma \langle {c}\rangle ^{{*}}+{a}_{\mathrm{vM}} { k}\varepsilon ^{{*}}{t}^{{*}}\langle {c}\rangle ^{{*}}}} \right] \ll 1. \end{aligned}$$

(120)

The introduction of a mixed length scale with similar meaning to the one defined in “Appendix 1” leads to the following constraint for the potential:

$$\begin{aligned} \frac{l_k^2}{L^{2}}\left[ {\frac{{-}\langle {G}_2 \rangle +\langle {c}\rangle ^{{*}}{-}\langle {G}_1 \rangle \langle {c}\rangle ^{{*}}/\Delta \langle \phi \rangle ^{{*}}}{\frac{l_k^2 \varepsilon _\gamma }{L^{2}}(\langle {G}_2 \rangle {-}\langle {c}\rangle ^{{*}})+\langle {c}\rangle ^{{*}}}} \right] \ll 1. \end{aligned}$$

(121)

Appendix 3

1.1 Closure Problem Derivation

We start from Eqs. (39) to (42). In the case of infinite cylinders, the problem becomes independent of the axial direction $(z/r_{2})$ and, following the useful relationships presented by Ochoa-Tapia et al. (1993), we can rewrite the closure problem as:

$$\begin{aligned}&\frac{{1}}{{r}^{{*}}} \frac{\partial {G}_{{\gamma ,x}}}{\partial {r}^{{*}}}+\frac{\partial ^{2}{G}_{{\gamma ,x}}}{\partial {r}^{{*2}}}+\frac{{1}}{{r}^{{*2}}} \frac{\partial ^{2}{G}_{{\gamma ,x}}}{\partial {\theta }^{{2}}}={ 0}, \quad \hbox { in the }\gamma \hbox {-phase} \end{aligned}$$

(122)

$$\begin{aligned}&\frac{\partial {G}_{{\gamma , x}}}{\partial {r}^{{*}}} = \delta \frac{\partial {G}_{{\kappa , x}}}{\partial {r}^{{*}}}{ - { cos } \theta (1 -}\delta {)}, \quad @ r^{*}= r_1 /r_2 \end{aligned}$$

(123)

$$\begin{aligned}&{G}_{{\gamma , x}} ={ G}_{{\kappa , x}} \quad @ r^{*}= r_1 /r_2 \end{aligned}$$

(124)

$$\begin{aligned}&\frac{{1}}{{r}^{{*}}} \frac{\partial {G}_{{\kappa , x}}}{\partial {r}^{{*}}}+\frac{\partial ^{2}{G}_{{\kappa , x}}}{\partial {r}^{{*2}}}+\frac{{1}}{{r}^{{*2}}} \frac{\partial ^{2}{G}_{{\kappa , x}}}{\partial {\theta }^{{2}}}={ 0},\quad \hbox { in the }\kappa \hbox {-phase} \end{aligned}$$

(125)

$$\begin{aligned}&\frac{\partial {G}_{{\kappa , x}}}{\partial {r}^{{*}}} ={ 0}, \quad @ r^{*}= 0 \end{aligned}$$

(126)

$$\begin{aligned}&{G}_{{\gamma , x}} =0, \quad @ r^{*}= 1. \end{aligned}$$

(127)

Here, the dimensionless closure variable $(G_{i,x})$ is given by $G_{i,x} = g_{i,x} /r_{2}, r^{*}= r/r_{2}, g_{i,x}$ the dimensional closure variable is given by ${g}_{{i,x}} ={\underline{{n}}}_{{\gamma \kappa }} \,{\bullet }\, \underline{{g}}_{i}, r^{*}= r/r_{2,}\delta$ is the ratio of phase diffusivities, and $\delta = D_{\kappa , eff}/D_{\gamma }$.

Equation (127) replaces the periodic boundary conditions in Chang’s unit cell for cylindrical coordinates. The equation used to determine ${{D}_{{xx}}^{*}}/{{D}_{\gamma }}$ is given by:

$$\begin{aligned} \left. \frac{{D}_{{xx}}^{*}}{{D}_{\gamma }}= \left\{ \varepsilon _\gamma + \left( 1-\varepsilon _\gamma \right) \delta \right\} + \left( {\delta - 1} \right) \frac{{1}}{{V}_\mathrm{M}}\int \limits _{{A}_{{\gamma \kappa }}} {{ cos }\left( {\theta } \right) { G}_{{\gamma , x}} \,{\hbox {d}A}} \right) . \end{aligned}$$

(128)