Abstract
Classical propositional logic can be characterized, indirectly, by means of a complementary formal system whose theorems are exactly those formulas that are not classical tautologies, i.e., contradictions and truth-functional contingencies. Since a formula is contingent if and only if its negation is also contingent, the system in question is paraconsistent. Hence classical propositional logic itself admits of a paraconsistent characterization, albeit “in the negative”. More generally, any decidable logic with a syntactically incomplete proof theory allows for a paraconsistent characterization of its set of theorems. This, we note, has important bearing on the very nature of paraconsistency as standardly characterized.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
Notes
Some systems of paraconsistent logic have more than one negation operator, and \(\mathsf {ECQ}\) need not fail for all. For instance, da Costa’s C-systems (da Costa 1974) have a “strong negation” that satisfies \(\mathsf {ECQ}\). Of course, the question of what counts as a bona fide negation operator is by itself a delicate matter in this context (see e.g. Lenzen 1998; Béziau 2002b); we shall come back to this below.
We take a system \({\mathsf {S}}\) to be syntactically incomplete if and only if there is a formula \(\alpha \) in the language of \({\mathsf {S}}\) and a negation operator \(\lnot \) such that neither \(\vdash _{\mathsf {S}}\alpha \) nor \(\vdash _{\mathsf {S}}\lnot \alpha \).
It is also possible to consider a stronger notion of complementarity, whereby \({\mathsf {S}}\) and \(\overline{{\mathsf {S}}}\) are complementary just in case \(\Gamma \nvdash _{\mathsf {S}}\alpha \) if and only if
(or, more generally, \(\Gamma \nvdash _{\mathsf {S}}\Delta \) if and only if 
, where \(\Gamma \) and \(\Delta \) are sets of formulas). In that case, 
is just the complement of \(\vdash _{{\mathsf {S}}}\). For our purposes, however, it will suffice to restrict our attention to the notion defined in \(\mathsf {COMP}\), which is generally weaker unless both \({\mathsf {S}}\) and \(\overline{{\mathsf {S}}}\) satisfy the deduction theorem (i.e., unless \(\Gamma \cup \{ \alpha \} \vdash _{\mathsf {S}}\beta \) implies \(\Gamma \vdash _{\mathsf {S}} \alpha \rightarrow \beta \) for all \(\Gamma , \alpha , \beta \), and similarly for 
).Derivation in \(\overline{\mathsf {CL}}\) is defined as usual:
if and only if there is a finite sequence \(\alpha _1, \dots , \alpha _m\) such that \(\alpha _m = \alpha \) and every \(\alpha _k\) is either a member of \(\Gamma \cup \{\bot \}\) or else follows from some \(\alpha _i\), \(i < k\), by one of the rules. When \(\Gamma = \varnothing \), \(\alpha \) counts as a \(\overline{\mathsf {CL}}\)-theorem and the sequence \(\alpha _1, \dots , \alpha _m\) as a \(\overline{\mathsf {CL}}\)-proof of \(\alpha \).Gentzen-style complementary systems are typically defined in terms of the stronger notion of complementarity mentioned in note 3. They differ, therefore, from the version of \(\overline{\mathsf {CL}}\) defined above, which does not satisfy the deduction theorem. (We have, for instance,
but not 
.)An axiomatization of the classical contingencies can also be obtained from the version of \(\overline{\mathsf {CL}}\) given above by taking as axiom an arbitrary sentence variable, \(p_i\), and counting as equivalent the following pairs of formulas for \(\alpha \in \{\bot , \lnot \bot , p_i, \lnot p_i\}\): \(\lnot \lnot \alpha , \alpha \) | \(\alpha \vee \bot , \alpha \) | \(\bot \vee \alpha , \alpha \) | \(\alpha \vee \lnot \bot , \lnot \bot \) | \(\lnot \bot \vee \alpha , \lnot \bot \). See (Varzi 1990, §5).
Here it is important to keep in mind that \(\lnot \) admits of a perfectly standard semantic characterization as a negation connective. Certainly there are ways of interpreting this operator that would not justify the claim of paraconsistency. For instance, with \(\lnot \) understood as a modal connective for necessity \(\Box \), or possibility \(\Diamond \), any normal modal logic would be non-explosive in the present sense, but of course such connectives do not qualify as negations in any reasonable sense. See also below, note 11.
As with the users of the Cretan Manual envisaged in Massey (1978), or the characters of the dialogue in Casati and Varzi (2000). The standard notion of logical consequence as truth preserving, by contrast, goes back to Tarski (1936). Of course, the validity of \(\mathsf {ECQ}\) is not just a byproduct of the standard notion; it depends also on specific logical principles. For example, C. I. Lewis’s classic argument for \(\mathsf {ECQ}\) requires disjunction introduction (\(\alpha \vdash \alpha \vee \beta \)) and disjunctive syllogism (\(\alpha , \lnot \alpha \vee \beta \vdash \beta \)); see (Lewis and Langford 1932, p. 250).
As already pointed out, Gentzen-style complementary systems are typically defined in terms of the stronger notion of \(\mathsf {COMP}\) mentioned in note 3. Thus, in that case the contrast between Alice’s and Bob’s attitudes is more naturally put in terms of validity and invalidity rather than truth and falsity: “Is that sequent valid [resp. invalid]? Then so is this one.”
There are other ways of blending paraconsistency and classicality. For instance, Béziau (2002a) points out that in classical first-order logic one can define a non-explosive negation operator \({\sim }\) just by setting \({\sim } \alpha =_{df} \exists x \lnot \alpha \). Our point, here, is that there is a paraconsistent side already to the classical negation operator \(\lnot \).
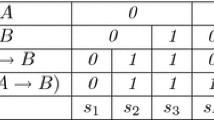
Note that \(\mathsf {AT}\) and \(\mathsf {BT}\) are not only classically invalid; they have instances that are classical contradictions, e.g. when \(\alpha \) is \(p_i \wedge \lnot p_i\).
To our knowledge, all familiar paraconsistent systems are syntactically incomplete, hence the argument at the end of Sect. 2 applies mutatis mutandis: if \({\mathsf {S}}\) is such a system and \(\mathsf {\overline{{\mathsf {S}}}}\) satisfies \(\mathsf {COMP}\), then \(\mathsf {\overline{{\mathsf {S}}}}\) must be paraconsistent, too. For the incompleteness of \({\mathsf {S}}\) implies the existence of some
and 
, and from this we can conclude that 
for any \({\mathsf {S}}\)-theorem \(\beta \).This would not be true if complementarity were defined in the stronger sense mentioned in note 3, yielding what Béziau and Buchsbaum (2016) call “anti-classical consequence relation”. For this reason, the following observations do not apply to Gentzen-style complementaries of \(\mathsf {CL}\), though it should be noted that such calculi, too, preserve several important properties of their classical counterparts, including the subformula property and, as already noted, cut elimination (Carnielli and Pulcini 2017).
We are most thankful to Walter Carnielli, Graham Priest, and to the referees of Synthese for they helpful comments and suggestions on earlier versions of this paper. G.P. also thankfully acknowledges the support from the Portuguese Science Foundation, FCT, through the project “Hilbert’s 24th Problem” PTDC/MHC-FIL/2583/2014.
(or, more generally, 
, where 
is just the complement of 
).
if and only if there is a finite sequence 
but not 
.)
and 
, and from this we can conclude that 
for any References
Arieli, O., Avron, A., & Zamansky, A. (2011). Ideal paraconsistent logics. Studia Logica, 99, 31–60.
Arruda, A. I., & da Costa, N. C. A. (1970). Sur le schéma de la séparation. Nagoya Mathematical Journal, 38, 71–84.
Batens, D. (1980). Paraconsistent extensional propositional logics. Logique et Analyse, 23, 195–234.
Béziau, J.-Y. (2000). What is paraconsistent logic? In D. Batens, C. Mortensen, G. Priest, & J.-P. van Bendegem (Eds.), Frontiers of paraconsistent logic (pp. 95–111). Baldock: Research Studies Press.
Béziau, J.-Y. (2002a). S5 is a paraconsistent logic and so is first-order classical logic. Logical Investigations, 9, 301–309.
Béziau, J.-Y. (2002b). Are paraconsistent negations negations? In W. A. Carnielli, M. E. Coniglio, & I. M. L. D’Ottaviano (Eds.), Paraconsistency: The logical way to the inconsistent. Proceedings of the 2nd world congress (pp. 465–486). New York: Dekker.
Béziau, J.-Y., & Buchsbaum, A. (2016). Let us be antilogical: Anti-classical logic as a logic. In A. Moktefi, A. Moretti, & F. Schang (Eds.), Soyons logiques/Let’s be logical (pp. 1–10). London: College Publications.
Bonatti, P. (1993). A Gentzen system for non-theorems. Technical Report CD-TR 93/52. Technische Universität Wien, Institut für Informationssysteme.
Bonatti, P., & Olivetti, N. (2002). Sequent calculi for propositional nonmonotonic logics. ACM Transactions on Computational Logic, 3, 226–278.
Brady, R. T. (2008). A rejection system for the first-degree formulae of some relevant logics. Australasian Journal of Logic, 6, 55–69.
Brown, D. J., & Suszko, R. (1973). Abstract logics. Dissertationes Mathematicae, 102, 9–42.
Bryll, G., & Maduch, M. (1969). Aksjomaty odrzucone dla wielowartościowych logik Łukasiewicza. Zeszyty Naukowe Wyższej Szkoły Pedagogicznej w Opolu, Seria A, Matematyka, 6, 3–19.
Bueno, O. (2007). Troubles with trivialism. Inquiry, 50, 655–667.
Caferra, R., & Peltier, N. (2008). Accepting/rejecting propositions from accepted/rejected propositions: A unifying overview. International Journal of Intelligent Systems, 23, 999–1020.
Caicedo, X. (1978). A formal system for the non-theorems of the propositional calculus. Notre Dame Journal of Formal Logic, 19, 147–151.
Carnielli, W. A., Coniglio, M. E., & Marcos, J. (2007). Logics of formal inconsistency. In D. M. Gabbay & F. Guenthner (Eds.), Handbook of Philosophical Logic (2nd ed., Vol. 14, pp. 1–93). Dordrecht: Kluwer.
Carnielli, W. A., & Marcos, J. (2002). A taxonomy of C-systems. In W. A. Carnielli, M. E. Coniglio, & I. M. L. D’Ottaviano (Eds.), Paraconsistency: The logical way to the inconsistent. Proceedings of the 2nd world congress (pp. 1–94). New York: Dekker.
Carnielli, W. A., & Pulcini, G. (2017). Cut-elimination and deductive polarization in complementary classical logic. Logic Journal of the IGPL, 25, 273–282.
Casati, R., & Varzi, A. C. (2000). True and false: An exchange. In A. Gupta & A. Chapuis (Eds.), Circularity, definition, and truth (pp. 365–370). New Delhi: Indian Council of Philosophical Research.
Citkin, A. (2015). A meta-logic of inference rules: Syntax. Logic and Logical Philosophy, 24, 313–337.
da Costa, N. C. A. (1974). On the theory of inconsistent formal systems. Notre Dame Journal of Formal Logic, 15, 497–510.
Dutkiewicz, R. (1989). The method of axiomatic rejection for intuitionistic propositional logic. Studia Logica, 48, 449–459.
Girard, J.-Y. (1987). Linear logic. Theoretical Computer Science, 50, 1–101.
Girard, J.-Y., Lafont, Y., & Taylor, P. (1989). Proofs and types. Cambridge: Cambridge University Press.
Goranko, V. (1994). Refutation systems in modal logic. Studia Logica, 53, 299–324.
Hailperin, T. (1961). A complete set of axioms for logical formulas invalid in some finite domain. Zeitschrift für mathematische Logik und Grundlagen der Mathematik, 7, 84–96.
Jaśkowski, S. (1948). Rachunek zdań dla systemów dedukcyjnych sprzecznych. Studia Societatis Scientiarum Torunesis, Sectio A, 1, 55–77. (Eng. trans. by Wojtasiewicz, O. (1969). Propositional calculus for contradictory deductive systems. Studia Logica, 24, 143–157.)
Johansson, I. (1936). Der Minimalkalkül, ein reduzierter intuitionistischer Formalismus. Compositio Mathematica, 4, 119–136.
Lenzen, W. (1998). Necessary conditions for negation-operators (with particular applications to paraconsistent negation). In D. M. Gabbay & P. Smets (Eds.), Handbook of defeasible reasoning and uncertainty management systems (pp. 211–239). Berlin: Springer.
Lewis, C. I., & Langford, C. H. (1932). Symbolic logic. New York: Century.
Łukasiewicz, J. (1939). O sylogistyce Arystotelesa. Sprawozdania z czynności i posiedzeń Polskiej Akademii Umiejętności, 44, 220–227 (published in 1946).
Łukasiewicz, J. (1951). Aristotle’s syllogistic from the standpoint of modern formal logic. Oxford: Clarendon Press.
Massey, G. (1978). Indeterminacy, inscrutability, and ontological relativity. In N. Rescher (Ed.), Studies in ontology (pp. 43–55). Oxford: Basil Blackwell.
Morgan, C. G. (1973). Sentential calculus for logical falsehoods. Notre Dame Journal of Formal Logic, 14, 347–353.
Morgan, C. G., Hertel, A., & Hertel, P. (2007). A sound and complete proof theory for propositional logical contingencies. Notre Dame Journal of Formal Logic, 48, 521–530.
Oetsch, J., & Tompits, H. (2011). Gentzen-type refutation systems for three-valued logics with an application to disproving strong equivalence. In J. P. Delgrande & W. Faber (Eds.), Logic programming and nonmonotonic reasoning. Proceedings of the 11th conference (pp. 254–259). Berlin: Springer.
Priest, G. (1979). Logic of paradox. Journal of Philosophical Logic, 8, 219–241.
Priest, G. (2000). Could everything be true? Australasian Journal of Philosophy, 78, 189–195.
Priest, G. (2002). Paraconsistent logic. In D. M. Gabbay & F. Guenthner (Eds.), Handbook of philosophical logic (2nd ed., Vol. 6, pp. 287–393). Dordrecht: Kluwer.
Priest, G. (2007). Paraconsistency and dialetheism. In D. M. Gabbay & J. Woods (Eds.), Handbook of the history of logic (Vol. 8, pp. 129–204). Amsterdam: North-Holland.
Priest, G., Tanaka, K. & Weber, Z. (2015). Paraconsistent logic. In E. N. Zalta (Ed.), The Stanford encyclopedia of philosophy. Spring 2015 Edition. http://plato.stanford.edu/archives/spr2015/entries/logic-paraconsistent
Puga, L. Z., & da Costa, N. C. A. (1988). On the imaginary logic of N. A. Vasiliev. Zeitschrift für mathematische Logik und Grundlagen der Mathematik, 34, 205–211.
Scott, D. (1957). Completeness proofs for the intuitionistic sentential calculus. In Summaries of talks presented at the summer institute for symbolic logic (pp. 231–242). Princeton: Institute for Defense Analyses.
Sette, A. M. (1973). On the propositional calculus P1. Mathematica Japonicae, 18, 173–180.
Skura, T. (1991). On decision procedures for sentential logics. Studia Logica, 50, 173–179.
Skura, T. (1999). Aspects of refutation procedures in the intuitionistic logic and related systems. Wrocław: Wydawnictwo Uniwersytetu Wrocławskiego.
Skura, T. (2011). Refutation systems in propositional logic. In D. M. Gabbay & F. Guenthner (Eds.), Handbook of philosophical logic (2nd ed., Vol. 16, pp. 115–157). Dordrecht: Kluwer.
Skura, T. (2013). Refutation methods in modal propositional logic. Warsaw: Semper.
Słupecki, J., Bryll, G., & Wybraniec-Skardowska, U. (1971). Theory of rejected propositions, I. Studia Logica, 29, 75–119.
Słupecki, J., Bryll, G., & Wybraniec-Skardowska, U. (1972). Theory of rejected propositions, II. Studia Logica, 30, 97–145.
Tamminga, A. (1994). Logics of rejection: Two systems of natural deduction. Logique et Analyse, 146, 169–208.
Tarski, A. (1928). Remarques sur les notions fondamentales de la méthodologie des mathématiques. Annales de la Société Polonaise de Mathématique, 7, 270–273. (Eng. trans. by Purdy, R., & Zygmunt, J. (2012). Remarks on the fundamental concepts of the methodology of mathematics. In J.-Y. Béziau (Ed.), Universal logic: An anthology (pp. 67–68). Basel: Birkhäuser).
Tarski, A. (1936). O pojciu wynikania logiznego. Przegląd Filozoficzny, 39, 58–68. (Eng. trans. by Woodger, J. H. (1956). On the concept of logical consequence. In Tarski’s Logic, semantics, metamathematics. Papers from 1923 to 1938 (pp. 409–420). Oxford: Clarendon Press).
Tiomkin, M. L. (1988). Proving unprovability. In Proceedings of the third annual symposium on logic in computer science (LICS ’88) (pp. 22–26). Edinburgh: IEEE Computer Society Press.
Tiomkin, M. L. (2013). A sequent calculus for a logic of contingencies. Journal of Applied Logic, 11, 530–535.
Urbas, I. (1990). Paraconsistency. Studies in Soviet Thought, 39, 434–454.
Varzi, A. C. (1990). Complementary sentential logics. Bulletin of the Section of Logic, 19, 112–116.
Varzi, A. C. (1992). Complementary logics for classical propositional languages. Kriterion, 4, 20–24.
Wansing, H. (2005). Connexive modal logic. In R. Schmidt, I. Pratt-Hartmann, M. Reynolds, & H. Wansing (Eds.), Advances in modal logic (Vol. 5, pp. 367–383). London: King’s College Publications.
Wybraniec-Skardowska, U., & Waldmajer, J. (2011). On pairs of dual consequence operations. Logica Universalis, 5, 177–203.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Pulcini, G., Varzi, A.C. Paraconsistency in classical logic. Synthese 195, 5485–5496 (2018). https://doi.org/10.1007/s11229-017-1458-0
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11229-017-1458-0