Skip to main content
SpringerLink
Log in

Idealizations, essential self-adjointness, and minimal model explanation in the Aharonov–Bohm effect

  • Published:
Synthese Aims and scope Submit manuscript

Abstract

Two approaches to understanding the idealizations that arise in the Aharonov–Bohm (AB) effect are presented. It is argued that a common topological approach, which takes the non-simply connected electron configuration space to be an essential element in the explanation and understanding of the effect, is flawed. An alternative approach is outlined. Consequently, it is shown that the existence and uniqueness of self-adjoint extensions of symmetric operators in quantum mechanics have important implications for philosophical issues. Also, the alleged indispensable explanatory role of said idealizations is examined via a minimal model explanatory scheme. Last, the idealizations involved in the AB effect are placed in a wider philosophical context via a short survey of part of the literature on infinite and essential idealizations.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7

Similar content being viewed by others

Notes

  1. An astute reader will notice that, as defined, the AB effect cannot be manifested in the laboratory, but the effect is by now almost universally accepted as empirically confirmed. The tension between the AB effect as a concrete, phenomenological effect that has been confirmed, on the one hand, and its conventional definition, on the other, will be the main focus of this paper. Compare the situation with phase transitions (Shech 2013): Boiling kettles and magnetized iron are finite systems that we interact with regularly. Yet, according to our best theories of phase transitions “... the existence of a phase transition requires an infinite system. No phase transitions occur in systems with a finite number of degrees of freedom” (Kadanoff 2000, p. 238). In addition, it should be added that whether one considers the “AB effect” to have been confirmed will depend on controversial issues such as the local or non-local nature and physical basis (i.e., reality of vector potential) of the effect. E.g., see recent Magni (1995, p. 186):

    Finally, the reader might wish that a definite answer be given to the vexed question about the existence or nonexistence of the Aharonov–Bohm effect. In order to answer it we have to split the question in two:

    1. (a)

      If, under the name of the Aharonov–Bohm effect a shifting of interference pattern is meant, then our answer is that, at any rate, the Schrödinger equation leads exactly to this prediction.

    2. (b)

      If, on the other hand, what is meant is a shifting due to any independent effect of the vector potential then our answer is no, there is no such thing coming from the Schrödinger equation.

  2. This paper is a development of my Shech (2015, Section 5). See Earman (2016) for similar claims, a masterful philosophical analysis of the AB effect controversy in the physics literature, and an identification of the connection between the AB effect idealizations and the existence of unitarily inequivalent representations of the canonical commutation relations.

  3. This is the magnetic AB effect. There is an analogue electric AB effect, implied by Lorentz covariance, which arises from electric (instead of magnetic) fields. See Peshkin and Tonomura (1989) and Tonomura (1999, 2010) for a review of the effect and its experimental confirmation, along with a selective review of the debate that arose with respect to the reality of the effect in the physics literature. Ehrenberg and Siday (1949) are usually credited with first noting the effect and Chambers (1960) with being the first experimental confirmation. See Hiley (2013) for the early history of the AB effect. Tonomura et al. (1982, 1986) are considered the first definitive confirmation of the effect and Caprez et al. (2007) further confirms the effect’s purely quantum mechanical origin. As Tonomura (1999, Ch. 6) explains, the definitive confirmation of the AB effect by Tonomura et al. (1982, 1986) did not make use of infinite and impenetrable solenoids. Instead, superconducting toroidal solenoids where used. Such solenoids are prepared so as to minimize magnetic field leakage and maximally shield the solenoid from an electron beam with a copper and niobium coating. A shift in interference pattern was observed. Although part of the shift could be explained in terms of the unideal conditions and other sources of error, these aspects could not account for the entire shift in the pattern. For example, it was calculated that the magnetic field leakage can affect the phase of an incident electron beam by at most \(\pi \), but the observed relative phase shift was about \(12\,\,\pi \). For these reasons there is little doubt now that the (concrete) AB effect is a real physical effect and not an artifact of idealizations.

  4. Roberts (2016) notes that there is a generalized Stone’s theorem (see Cooper 1947, 1948) for maximal symmetric operators. An operator H is symmetric if it has the property that \(H\Psi =H^{*}\Psi \) for all \(\Psi \) in the common domain of H and its adjoint \(H^{*}\). An operator is maximal symmetric if it does not admit any self-adjoint extensions. Since, in the context discussed here, \(H_{AB}^I\) does have self-adjoint extensions, the generalized Stone’s theorem is inapplicable.

  5. See Earman (2009) for a primer on essential self-adjointness. For quantization on multiply connected configuration spaces and the relevance of essential self-adjointness also see Earman (2010) and Landsman (2016).

  6. The domain of \({\bar{H}}_{AB}^I\) is \(Dom({\bar{H}}_{AB}^I)=\mathcal{H}^{2}( {S_{out} })\cap \mathcal{H}_0^1( {S_{out} })\) where \(\mathcal{H}^{d}\) denotes the usual Sobolev space of square integrable functions so that \(\mathcal{H}^{2}\) is the domain of the free Hamiltonian (i.e., the negative Laplacian \(-\Delta )\) in \(L^{2}( {S_{out} })\) and \(\mathcal{H}_0^1( {S_{out} } )\) is a subspace of \(\mathcal{H}^{1}( {S_{out} })\) with elements vanishing (in the sense of Sobolev traces) at the solenoid boundary of \(S_{in} \). Note that the Dirichlet boundary conditions are selected because of the space \(\mathcal{H}_0^1( {S_{out} })\) in the limit operator domain. More precisely, \({\bar{H}}\) is the Friedrichs extensions of the formal operator \(\mathop \sum \nolimits _{i=1}^3( {-i\frac{\partial }{\partial x_i }+\frac{q}{c}A_i })^{2}\) with homogeneous Dirichlet conditions at the boundary of \(S_{in} \). Also notice that \(Dom({\bar{H}}_{AB}^I)\supset C_0^\infty ( {S_{out} } )\). See Magni and Valz-Gris (1995) and de Oliveira and Pereira (2008, 2011) for details.

  7. See Healey (2007, Ch. 1–2) for an introduction.

  8. Sentiments of this sort arise in, among others, Aharonov and Bohm (1959), Batterman (2003), Belot (1998, p. 544), Lyre (2001, 2009), Nounou (2003), Peshkin and Tonomura (1989), Ryder (1996), and Wu and Yang (1975, p. 3845). Healey (2007), while agreeing that topological considerations may be important, disagrees with Batterman’s (2003) and Nounou’s (2003) claim that the AB effect occurs because of the topology of the base manifold.

  9. The idea that “different self-adjoint extensions of a symmetric operator may lead to very different physics” was studied in detail first in Ruijsenaars (1983, p. 3).

  10. Butterfield (2011, Section 3) discusses similar distinctions. In particular, he makes a distinction between a system\(\sigma \left( N \right) \) that depends on some parameter N (let \(\{\sigma \left( N \right) \)} denote a sequence of such systems), a quantity defined on the system \(f\left( {\sigma \left( N \right) } \right) \) (let \(\left\{ {f\left( {\sigma \left( N \right) } \right) } \right\} \) denote a sequence of quantities on successive systems), and a (real number) value\(v\left( {f\left( {\sigma \left( N \right) } \right) } \right) \) of quantities on successive systems (where a sequence of states on \(\sigma \left( N \right) \) is implicitly understood; let \(\left\{ {v\left( {f\left( {\sigma \left( N \right) } \right) } \right) } \right\} \) denote a sequence of values on successive systems). A limit system\(\sigma \left( \infty \right) \) arises when \(\mathop {\lim }\nolimits _{N\rightarrow \infty } \left\{ {\sigma \left( N \right) } \right\} \) is well-defined-otherwise there is no limit system. A property of a limit system refers to the value \(v(f\left( {\sigma \left( \infty \right) } \right) \) of the (natural) limit quantity \(f\left( {\sigma \left( \infty \right) } \right) \) (in the natural limit state) on \(\sigma \left( \infty \right) \). A limit property\(v(f\left( {\sigma \left( N \right) } \right) \) is a limit of a sequence of values of quantities on successive systems (or, values on the systems on the way to the limit) and is well-defined when \(\mathop {\lim }\nolimits _{N\rightarrow \infty } \left\{ {v\left( {f\left( {\sigma \left( N \right) } \right) } \right) } \right\} \) exists. The question of whether a property of a limit system equals the corresponding limit property asks if the following holds: \(v(f\left( {\sigma \left( \infty \right) } \right) =\mathop {\lim }\nolimits _{N\rightarrow \infty } \left\{ {v\left( {f\left( {\sigma \left( N \right) } \right) } \right) } \right\} \) (assuming both are well-defined).

  11. In discussing limiting procedures I’m mostly concentrating on the cylindrical solenoid case as in Magni and Valz-Gris (1995) and de Oliveira and Pereira (2008, 2010, 2011). See Fig. 4 for the toroidal solenoid scenario where the limit taken is that of infinite impenetrability as in Ballesteros and Weder (2009, 2011) and de Oliveira and Pereira (2011).

  12. Notwithstanding the work of de Oliveira and Pereira (2010) which surely puts in question our right to assume a limit system in which Dirichlet boundary conditions hold, my point here is that evenif we allow for such a limit system, the claim that an idealized solenoid well-approximates actual systems is left without justification.

  13. An anonymous referee notes that a finite solenoid topology gives \({\mathbb {R}}^{3}\) minus a point or minus a closed ball, and is topologically \(S^{2}\times {\mathbb {R}}\), which is simply connected by not homeomorphic to \({\mathbb {R}}^{3}\). Also, whether or not the limiting procedures advertised in Sect. 4 will succeed or fail may well depend on the choice of configuration space that we begin with. From my perspective, if we take my instances of referring to \({\mathbb {R}}^{3}\) to implicitly mean \({\mathbb {R}}^{3}\) minus a point or minus a closed ball so that the limiting procedures work, my arguments would still hold since both spaces are simply connected and the dispensability of an idealized non-simply connected space succeeds.

  14. Compare with Shech (2013) for the paradox of phase transitions.

  15. Since \(\{ {H_{L,n} }\}\) are unbounded operators, there is no obvious way of defining a limiting procedure. Magni and Valz-Gris (1995, p. 180) note that, at the very least, \(\mathop {\lim }\nolimits _{n,L\rightarrow \infty } \{ {H_{L,n} }\}=H_{\infty ,\infty } \) should be the generator of the limit time evolution, and it is for this reason that I discuss the strong resolvent limit in the text. In other words, and roughly, if our system is initially in the state \(u_0 \), then the self-adjointness of \(H_{L,n} \) means that the time evolution \(u( t)=e^{-iH_{L,n} t}u_0 \) is well defined and we can consider the associated one parameter groups \(\{ {U( t )} \}\) such that \(u( t)=U( t)u_0\) . \(\mathop {\lim }\nolimits _{n,L\rightarrow \infty } \{ {H_{L,n} }\}\) is then defined to be the generator of the limit evolution group \(\{ {U( t)}\}\) so that \(u( t)=e^{-iH_{\infty ,\infty } t}u_0 \). The limiting procedure that Magni and Valz-Gris (1995, pp. 181–182) discuss is one in which \(H_n =\mathop \sum \nolimits _{i=1}^3 ( {-i\frac{\partial }{\partial x_i }+\frac{q}{c}A_i } )^{2}+nV\) has domain \(Dom( {H_n })=\mathcal{H}^{2}( {{\mathbb {R}}^{3}})\forall n\) and \(\mathop {\lim }\nolimits _{n\rightarrow \infty }\{ {H_n }\}=H_\infty \) has domain \(Dom( {H_\infty })=\mathcal{H}^{2}( {S_{out} })\cap \mathcal{H}_0^1 ( {S_{out} })\), where \(\mathcal{H}^{d}\) is the usual Sobolev space of square summable functions with square summable derivatives up to order d (although it seems we can equally consider the space of square integrable functions), and \(\mathcal{H}_0^1 ( {S_{out} })\) is the subspace of \(\mathcal{H}^{1}( {S_{out} })\) with elements vanishing (in the sense of Sobolev traces) at the boundary of \(S_{in} \). They then explain that \(H_\infty \) is nothing but a precise form of the operator \({\bar{H}}_{AB}^I\) introduced by Aharonov and Bohm (1959) with homogenous Dirichlet conditions at the boundary of \(S_{in} \), which has the domain \(Dom({\bar{H}}_{AB}^I )=\mathcal{H}^{2}( {S_{out} } )\cap \mathcal{H}_0^1 ( {S_{out} })\). de Oliveira and Pereira (2008)’s discussion builds upon the results of Magni and Valz-Gris (1995) and is more general. \(S_{in}\) is defined as \(S_{in} =\{( {x_1 ,x_2 ,x_3 }):x_1^2 +x_2^2<r_0 ^{2},| {x_3 }|<L\}\), where \((x_1 ,x_2 ,x_3 )\) denote Cartesian coordinates in \({\mathbb {R}}^{3}\), \(r_0 >0\) is radius of solenoid S with finite length \(2L>0\), and \(S_{out} \) is the exterior region. Note that, whereas in the main text I assume \(S_{in} \) includes the boundary \(S=\partial S_{in} \), here \(S_{out} ={\mathbb {R}}^{3}\backslash S\cup S_{in}\). The Hamiltonians \(\{ {H_{L,n} }\}\) have a domain \(Dom( {H_{L,n} } )=\mathcal{H}^{2}( {{\mathbb {R}}^{3}})\), where \(\mathcal{H}^{2}\) denotes the usual Sobolev space domain of the free Hamiltonian (i.e., the negative Laplacian \(-\Delta \)) in \(L^{2}( {S_{out} })\). de Oliveira and Pereira (2011) note that \(C_0^\infty ( {{\mathbb {R}}^{3}} )\) is the core of \(H_{L,n} \) (i.e., \(H_{L,n} \) restricted to \(C_0^\infty ( {{\mathbb {R}}^{3}})\) has just one self-adjoint extension). \({\varvec{A}}_{\varvec{L}} \) is chosen such that as \(L\rightarrow \infty \), there is a pointwise convergence, i.e., a convergence in the pointwise topology, of \({\varvec{A}}_{\varvec{L}}\) to \({\varvec{A}}\). de Oliveira and Pereira (2008) show that the domain of \(\mathop {\lim }\nolimits _{n,L\rightarrow \infty } \{ {H_{L,n} }\}=H_{\infty ,\infty } \) is \(Dom( {H_{\infty ,\infty } } )=\mathcal{H}^{2}( {S_{out} })\cap \mathcal{H}_0^1 ( {S_{out} })\) so that \(\mathop {\lim }\nolimits _{n,L\rightarrow \infty }\{ {H_{L,n} } \}={\bar{H}}_{AB}^I\) holds in the strong resolvent sense. They note that, except in the case of the \(L\rightarrow \infty \) in \({\mathbb {R}}^{3}\) (since they assume that the border region of the solenoid is a bounded set), \(H_{L,n} \) uniformly converges to \({\bar{H}}_{AB}^I\), i.e., with the uniform topology, and this is why de Oliveira and Pereira (2011) are able to extend said results to the norm resolvent sense in the \(n\rightarrow \infty \) case. An important point in the work of Magni and Valz-Gris (1995) and de Oliveira and Pereira (2008, 2011) is that the selection of Dirichlet boundary conditions arises through the modeling of the impenetrability process of \(n\rightarrow \infty \) in which the repulsive barrier becomes infinitely impenetrable and creates a hole in the space; they are not just chosen without justification. Last, for completeness, note that an additional idealization that I do not discuss was studied by Weisskopf (1961) via a limiting process and concerns the fact that the electromagnetic field associated with the solenoid will interact with surrounding particles when the solenoid is being turned on and off.

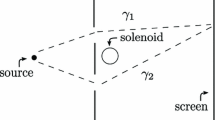
  16. More generally, we may think of the AB effect as a dependency of the behavior of electrons, such as in scattering experiments or in double-slit experiments, on magnetic flux in regions where the wavefunction and flux do not overlap (at least not to the extent that the overlapping can account for the dependency).

  17. See Kadanoff (2000) for accounts of phase transitions and critical phenomena, and renormalization group techniques.

  18. Trigger Warning: notions such as “fixed point” and “universality class” are being used loosely here, extended well beyond their usual scope.

  19. See Landsman (2013) for details regarding different possible notions of spontaneous symmetry breaking with associated lower- and higher-level theories, as well as a discussion of the classical-quantum divide, which is also discussed in Bokulich (2008). See Batterman (2002) for other examples.

  20. See Wayne (2009) for a criticism of the idea that singular limits are a mark of emergence.

  21. The name is due to Nobel laureate Wilczek (1982). Unfortunately, it is beyond the scope of this paper to discuss anyons (which are not paraparticles) or the FQHE. See Wilczek (1990) for a classic collection of papers on anyons, Ezawa (2013) (and references therein) for the FQHE, and Shech (2015) and Bain (2016) for a philosophical analysis.

  22. Shech (2015) and Earman (2016) make the same point. See Gelfert (2016) for four other senses of exploration particularly in discussion of models, and see Shech and Gelfert (2016) for a more thorough discussion of the exploratory role of idealization.

  23. Note that the purely quantum mechanical origin of the AB effect, although by now a common view, is still contested among some, e.g., Boyer (2008) and Lyre (2009). Lyre (2009) specifically notes that the AB effect is not quantum mechanical in origin because of the analogue classical gravitational AB effect. Moreover, notice that I’m not saying that the issue of non-locality or non-separability has been settled—see Healey (2007), especially Ch. 1–2, for a review of the state of the literature on this matter and Wallace (2014) for a recent local account. Instead, I only claim that part of what is at stake in the debate about non-locality/non-separability becomes clearer once it is realized that quantum and classical theories make strikingly different predictions about highly idealized systems (viz., an infinitely long and absolutely impenetrable cylindrical solenoid or an absolutely impenetrable toroidal solenoid).

References

  • Aharonov, Y., & Bohm, D. (1959). Significance of electromagnetic potentials in the quantum theory. Physical Review, 115, 485–491.

    Article  Google Scholar 

  • Bain, J. (2016). Emergence and the mechanism in the fractional quantum Hall effect. Studies in History and Philosophy of Modern Physics, 56, 27–38.

    Article  Google Scholar 

  • Ballentine, L. E. (1998). Quantum mechanics: A modern development. Singapore: World Scientific.

    Book  Google Scholar 

  • Ballesteros, M., & Weder, R. (2009). The Aharonov–Bohm effect and Tonomura et al. experiments: Rigorous results. Journal of Mathematical Physics, 50, 122108.

    Article  Google Scholar 

  • Ballesteros, M., & Weder, R. (2011). Aharonov–Bohm effect and high-velocity estimates of solutions to the Schrodinger equation. Communications in Mathematical Physics, 303(1), 175–211.

    Article  Google Scholar 

  • Bangu, S. (2009). Understanding thermodynamic singularities: Phase transitions, date and phenomena. Philosophy of Science, 76, 488–505.

    Article  Google Scholar 

  • Batterman, R. (2002). The devil in the details: Asymptotic reasoning in explanation, reduction, and emergence. London: Oxford University Press.

    Google Scholar 

  • Batterman, R. (2003). Falling cats, parallel parking, and polarized light. Studies in History and Philosophy of Science Part B: Studies in History and Philosophy of Modern Physics, 34, 527–557.

    Article  Google Scholar 

  • Batterman, R. (2005). Critical phenomena and breaking drops: Infinite idealizations in physics. Studies in History and Philosophy of Modern Physics, 36, 225–244.

    Article  Google Scholar 

  • Batterman, R. (2015). Autonomy and scales. In B. Falkenburg & M. Morrison (Eds.), Why more is different: Philosophical issues in condensed matter physics and complex systems (pp. 115–136). Heidelberg: Springer.

    Google Scholar 

  • Batterman, R. (2017). Autonomy of theories: An explanatory problem. Noûs. doi:10.1111/nous.12191.

  • Batterman, R., & Rice, C. (2014). Minimal model explanations. Philosophy of Science, 81(3), 349–376.

    Article  Google Scholar 

  • Belot, G. (1998). Understanding electromagnetism. British Journal for Philosophy of Science, 49(4), 531–555.

    Article  Google Scholar 

  • Berry, M. V. (1986). The Aharonov–Bohm effect is real physics not ideal physics. In V. Gorini & A. Frigerio (Eds.), Fundamental aspects of quantum theory (Vol. 144, pp. 319–320). New York: Plenum.

    Chapter  Google Scholar 

  • Bocchieri, P., & Loinger, A. (1978). Nonexistence of the Aharonov–Bohm effect. Nuovo Cimento, 47A(4), 475–482.

    Article  Google Scholar 

  • Bokulich, A. (2008). Re-examining the quantum-classical relation: Beyond reductionism and pluralism. Cambridge: Cambridge University Press.

    Book  Google Scholar 

  • Borrmann, P., Mülken, O., & Harting, J. (2000). Classification of phase transitions in small systems. Physical Review Letters, 84, 3511–3514.

    Article  Google Scholar 

  • Boyer, T. H. (2008). Comment on the experiments related to the Aharonov–Bohm phase shift. Foundations of Physics, 38, 398–505.

    Article  Google Scholar 

  • Bub, J. (1988). How to solve the measurement problem in quantum mechanics. Foundations of Physics, 18, 701–722.

    Article  Google Scholar 

  • Butterfield, J. (2011). Less is different: Emergence and reduction reconciled. Foundations of Physics, 41(6), 1065–1135.

    Article  Google Scholar 

  • Caprez, A., Barwick, B. B., & Batelaan, H. (2007). Macroscopic test of the Aharonov–Bohm effect. Physical Review Letters, 99, 210401.

    Article  Google Scholar 

  • Chambers, R. G. (1960). Shift of an electron interference pattern by enclosed magnetic flux. Physical Review Letter, 5(1), 3–5.

    Article  Google Scholar 

  • Chomaz, P., Gulminelli, F., & Duflot, V. (2001). Topology of event distributions as a generalized definition of phase transitions in finite systems. Physical Review E, 64, 046114.

    Article  Google Scholar 

  • Cooper, J. L. B. (1947). One-parameter semigroups of isometric operators in Hilbert space. Annals of Mathematics, 48(4), 827–842.

    Article  Google Scholar 

  • Cooper, J. L. B. (1948). Symmetric operators in Hilbert space. Proceedings of the London Mathematical Society, 2(1), 11–55.

    Article  Google Scholar 

  • de Oliveira, C. R., & Pereira, M. (2008). Mathematical justification of the Aharonov–Bohm Hamiltonian. Journal of Statistical Physics, 133, 1175–1184.

    Article  Google Scholar 

  • de Oliveira, C. R., & Pereira, M. (2010). Scattering and self-adjoint extensions of the Aharonov–Bohm Hamiltonian. Journal of Physics A: Mathematical and Theoretical, 43, 1–29.

    Article  Google Scholar 

  • de Oliveira, C. R., & Pereira, M. (2011). Impenetrability of Aharonov–Bohm solenoids: Proof of norm resolvent convergence. Letters in Mathematical Physics, 95, 41–51.

    Article  Google Scholar 

  • Earman, J. (2004). Curie’s principle and spontaneous symmetry breaking. International Studies in the Philosophy of Science, 18(2–3), 173–198.

    Article  Google Scholar 

  • Earman, J. (2008). Superselection rules for philosophers. Erkenntnis, 69, 377–414.

    Article  Google Scholar 

  • Earman, J. (2009). Essential self-adjointness: Implications for determinism and the classical-quantum correspondence. Synthese, 169, 2750.

    Google Scholar 

  • Earman, J. (2010). Understanding permutation invariance in quantum mechanics. https://www.youtube.com/watch?v=xciuUhnsx1k (unpublished preprint).

  • Earman, J. (2016). The role of idealization in the Aharonov–Bohm effect. http://philsci-archive.pitt.edu/12696/.

  • Ehrenberg, W., & Siday, R. W. (1949). The refractive index in electron optics and the principles of dynamics. Proceedings of the Physical Society London: Section B, 62(1), 8–21.

    Article  Google Scholar 

  • Ellis, B. (1992). Idealizations in science. In C. Dilworth (Ed.), Idealization IV: Intelligibility in science. Amsterdam: Rodopi.

    Google Scholar 

  • Emch, G. (2006). Quantum statistical physics. In J. Butterfield, & J. Earman (Eds.), Philosophy of physics, part B, a volume of the handbook of the philosophy of science (pp. 1075–1182). North Holland.

  • Eskin, G. (2013). A simple proof of magnetic and electric Aharonov–Bohm effects. Communications in Mathematical Physics, 321(3), 747–767.

    Article  Google Scholar 

  • Ezawa, Z. F. (2013). Quantum Hall effects: Recent theoretical and experimental developments. Singapore: World Scientific.

    Book  Google Scholar 

  • Fisher, R. A. (1930). The genetical theory of natural selection. Oxford: Clarendon.

    Book  Google Scholar 

  • Fodor, J. (1974). Special sciences, or the disunity of sciences as a working hypothesis. Synthese, 28, 97–115.

    Article  Google Scholar 

  • Franzosi, R., & Pettini, M. (2004). Theorem on the origin of phase transitions. Physical Review Letters, 92, 060601.

    Article  Google Scholar 

  • Franzosi, R., Pettini, M., & Spinelli, L. (2000). Topology and phase transitions: Paradigmatic evidence. Physical Review Letters, 84, 2774–2777.

    Article  Google Scholar 

  • Fraser, J. D. (2016). Spontaneous symmetry breaking in finite systems. Philosophy of Science, 83(4), 585–605.

    Article  Google Scholar 

  • Gelfert, A. (2016). How to do science with models: A philosophical primer. Cham: Springer.

    Book  Google Scholar 

  • Gross, D. H. E., & Votyakov, E. V. (2000). Phase transitions in “small” systems. The European Physical Journal B-Condensed Matter and Complex Systems, 15, 115–126.

    Article  Google Scholar 

  • Healey, R. (1997). Nonlocality and the Aharonov–Bohm effect. Philosophy of Science, 64, 18–41.

    Article  Google Scholar 

  • Healey, R. (1999). Quantum analogies: A reply to Maudlin. Philosophy of Science, 66, 440–447.

    Article  Google Scholar 

  • Healey, R. A. (2007). Gauging what’s real: The conceptual foundations of contemporary gauge theories. New York: Oxford University Press.

    Book  Google Scholar 

  • Hiley, B. J. (2013). The early history of the AB effect. arXiv:1304.4736.

  • Kadanoff, L. P. (2000). Statistical physics: Statics, dynamics and renormalization. Singapore: World Scientific.

    Book  Google Scholar 

  • Landsman, N. P. (2013). Spontaneous symmetry breaking in quantum systems: Emergence or reduction? Studies in History and Philosophy of Modern Physics, 44, 379–394.

    Article  Google Scholar 

  • Landsman, N. P. (2016). Quantization and superselection III: Mutliply connected spaces and indistinguishable particles. Reviews in Mathematical Physics, 28, 1650019.

    Article  Google Scholar 

  • Lebowitz, J. L. (1999). Statistical mechanics: A selective review of two central issues. Reviews of Modern Physics, 71(2), S346–S357.

    Article  Google Scholar 

  • Liu, C., & Emch, G. G. (2005). Explaining quantum spontaneous symmetry breaking. Studies in History and Philosophy of Modern Physics, 36, 137–163.

    Article  Google Scholar 

  • Lui, C. (1999). Explaining the emergence of cooperative phenomena. Philosophy of Science, 66, S92–S106.

    Article  Google Scholar 

  • Lyre, H. (2001). The principles of gauging. Philosophy of Science, 68(3), S371–S381.

    Article  Google Scholar 

  • Lyre, H. (2009). Aharonov–Bohm effect. In D. Greengerger, C. Hentschel, & F. Weinert (Eds.), Compendium of quantum mechanics (pp. 1–3). Berlin: Springer.

    Google Scholar 

  • Magni, C., & Valz-Gris, F. (1995). Can elementary quantum mechanics explain the Aharonov–Bohm effect? Journal of Mathematical Physics, 36(1), 177–186.

    Article  Google Scholar 

  • Maudlin, T. (1998). Healey on the Aharonov–Bohm effect. Philosophy of Science, 65, 361–368.

    Article  Google Scholar 

  • Menon, T., & Callender, C. (2013). Turn and face the strange.. ch-ch-changes: Philosophical questions raised by phase transitions. In R. W. Batterman (Ed.), The Oxford handbook of philosophy of physics. Oxford: Oxford University Press.

    Google Scholar 

  • Möllenstedt, G., & Bayh, W. (1962). Kontinuierliche Phasenschiebung von Elektronenwellen im kraftfeldfreien Raum durch das magnetische Vektorpotential eines Solenoids. Zeitschrift für Physik, 169, 263.

    Article  Google Scholar 

  • Morrison, M. (2012). Emergent physics and micro-ontology. Philosophy of Science, 79, 141–166.

    Article  Google Scholar 

  • Morrison, M. (2015). Why is more different? In B. Falkenburg & M. Morrison (Eds.), Why more is different: Philosophical issues in condensed matter physics and complex systems (pp. 91–114). Heidelberg: Springer.

    Google Scholar 

  • Norton, J. D. (2012). Approximations and idealizations: Why the difference matters. Philosophy of Science, 79, 207–232.

    Article  Google Scholar 

  • Nounou, A. M. (2003). A fourth way to the Aharonov–Bohm effect. In K. Bradind & E. Castellani (Eds.), Symmetries in physics: Philosophical reflections. Cambridge: Cambridge University Press.

    Google Scholar 

  • Peshkin, M., & Tonomura, A. (1989). The Aharonov–Bohm effect. Berlin: Springer.

    Book  Google Scholar 

  • Prigogine, I. (1997). The end of certainty. New York: The Free Press.

    Google Scholar 

  • Reed, M., & Simon, B. (1980). Methods of modern mathematical physics (Vol. I-IV). San Diego: Academic Press Inc.

    Google Scholar 

  • Roberts, B. (2016). Observables, disassembled. http://philsci-archive.pitt.edu/12478/.

  • Rueger, A. (2000). Physical emergence, diachronic and synchronic. Synthese, 124, 297–322.

    Article  Google Scholar 

  • Rueger, A. (2006). Functional reduction and emergence in the physical sciences. Synthese, 151, 335–346.

    Article  Google Scholar 

  • Ruetsche, L. (2003). A matter of degree: Putting unitary inequivalence to work. Philosophy of Science, 70(5), 1329–1342.

    Article  Google Scholar 

  • Ruetsche, L. (2011). Interpreting quantum theories. Oxford: Oxford University Press.

    Book  Google Scholar 

  • Ruijsenaars, S. N. M. (1983). The Aharonov–Bohm effect and scattering theory. Annals of Physics, 146, 1–34.

    Article  Google Scholar 

  • Ryder, L. H. (1996). Quantum field theory. Cambridge: Cambridge University Press.

    Book  Google Scholar 

  • Schulman, L. (1971). A path integral for spin. Physical Review, 176, 1558–1569.

    Article  Google Scholar 

  • Shech, E. (2013). What is the ‘paradox of phase transitions? Philosophy of Science, 80, 1170–1181.

    Article  Google Scholar 

  • Shech, E. (2014). Scientific misrepresentation and guides to ontology: The need for representational code and contents. Synthese, 192(11), 3463–3485.

    Article  Google Scholar 

  • Shech, E. (2015). Two approaches to fractional statistics in the quantum Hall effect: Idealizations and the curious case of the anyon. Foundations of Physics, 45(9), 1063–1110.

    Article  Google Scholar 

  • Shech, E. (2016). Fiction, depiction, and the complementarity thesis in art and science. The Monist, 99(3), 311–332.

    Article  Google Scholar 

  • Shech, E.,  & Gelfert, A. (2016). The exploratory role of idealizations and limiting cases in models (preprint).

  • Tonomura, A. (1999). Electron holography. Berlin: Springer.

    Book  Google Scholar 

  • Tonomura, A. (2010). The AB effect and its expanding applications. Journal of Physics A, 43(35), 1–13.

    Article  Google Scholar 

  • Tonomura, A., Matsuda, T., Suzuki, R., Fukuhara, A., Osakabe, N., Umezaki, H., Endo, J., Shinagawa, K., Sugita, Y., & Fujiwara, H. (1982). Observation of the Aharonov-Bohm effect by electron holography. Physical Review Letters, 48, 1443.

  • Tonomura, A., Osakabe, N., Matsuda, T., Kawasaki, T., Endo, J., Yano, S., & Yamada, H. (1986). Evidence for Aharonov-Bohm effect with magnetic field completely shielded from electron wave. Physical Review Letter, 56, 792–795.

  • Wales, D. J., & Berry, R. S. (1994). Coexistence in finite systems. Physical Review Letters, 73, 2875–2878.

    Article  Google Scholar 

  • Wallace, D. (2014). Deflating the Aharonov–Bohm effect. https://arxiv.org/abs/1407.5073.

  • Wayne, A. (2009). Emergence and singular limits. Synthese, 184(3), 341–356.

    Article  Google Scholar 

  • Weisskopf, V. F. (1961). Selected topics in theoretical physics. In W. Brittin (Ed.), Lectures in theoretical physics (Vol. III, pp. 67–70). New York: Interscience.

    Google Scholar 

  • Wilczek, F. (1982). Quantum mechanics of fractional-spin particles. Physical Review Letters, 49(14), 957–959.

  • Wilczek, F. (Ed.). (1990). Fractional statistics and anyon superconductivity. Singapore: World Scientific.

    Google Scholar 

  • Wu, T. T., & Yang, C. N. (1975). Concept of nonintegrable phase factors and global formulation of gauge fields. Physical Review D, 12, 3845.

    Article  Google Scholar 

  • Yang, N. C., & Lee, T. D. (1952). Statistical theory of equations of state and phase transitions. I. Theory of condensation. Physical Review, 97, 404.

    Article  Google Scholar 

Download references

Acknowledgements

I am extremely grateful to John Earman, John D. Norton, Laura Ruetsche, Robert W. Batterman, James Woodward, Mark Wilson, Giovanni Valente, Nicholaos Jones, Bryan W. Roberts, Aaron Novick, and Samuel C. Fletcher, as well as audiences in numerous workshops and conferences, for insightful conversations and many constructive comments on earlier versions of this paper going back to 2014 under the title of “Topological Idealization, Asymptotic-Minimal Model Explanation, and the Aharonov–Bohm Effect.” Also thanks to Narin Shech for help with figures, and to Michel Smith and Cesar R. de Oliveira for assistance with technical issues with this version of the paper. Special thanks to John Earman for his guidance and mentorship regarding the details and issues discussed in this paper and over the years. Needless to say, my mistakes are my own, and I refer the reader to John Earman’s own more recent and excellent contribution in his “The Role of Idealization in the Aharonov–Bohm Effect” (http://philsci-archive.pitt.edu/12696/).

Author information

Authors and Affiliations

  1. Department of Philosophy, 6080 Haley Center, Auburn University, Auburn, AL, 36849-3715, USA

    Elay Shech

Authors
  1. Elay Shech
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Elay Shech.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Shech, E. Idealizations, essential self-adjointness, and minimal model explanation in the Aharonov–Bohm effect. Synthese 195, 4839–4863 (2018). https://doi.org/10.1007/s11229-017-1428-6

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11229-017-1428-6

Keywords

Search

Navigation