The possibility of vagueness

Abstract

I present a new approach to the logic and semantics of vagueness.

Appendix

We deal with a propositional language in which formulas are formed from an infinite set SL of sentence letters $\hbox {p}_\mathrm{1}$, $\hbox {p}_\mathrm{2}$, ...by means of the connectives $\vee$, $\wedge , \lnot ,$ and $\supset$ We shall also find it convenient to use the falsum constant $\bot$ in place of $\lnot$, defining $\lnot$A as A $\supset \bot$.

1.1 Intuitionistic Semantics

We begin by reminding the reader of the Kripke semantics for intuitionistic logic. This will then serve as a foil for our own compatibilist-style semantics.

Under the Kripke semantics, a model M is a triple ($U$, $\le$, $\upvarphi )$, where:

I(i):

$U$ (‘points’ or ‘uses’) is a non-empty set;

I(ii):

$\le$ (extension) is a reflexive and transitive relation on U;

I(iii):

$\upvarphi$ (valuation) is a function from SL into  

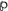
(U) subject to the Hereditary Condition:

$$\begin{aligned} u\in \upvarphi \left( \hbox {p} \right) \; \mathrm{{and}} \; u \le { {v}} \hbox { implies } { {v}}\in \upvarphi \left( \hbox {p} \right) \hbox { for } \hbox { all } u,{ {v}}\in U \hbox { and } \hbox { p} \in \hbox { SL}. \end{aligned}$$

Intuitively, we may think of $U$ as consisting of hypothetical uses of the predicates of the language; $\le$ is the extension relation, where one use extends another when anything taken to be true under the second use is taken to be true under the first; and $\upvarphi$ tells us which sentence letters are true under any given use.

Relative to a model M, truth of a formula A under the use $u$ ($u~{\vert }\!= \hbox {A}$) is defined by the following clauses:

T(i):

$u\,|{=\hbox { p iff }} u \in \upvarphi \left( \hbox {p} \right)$

T(ii):

$u\,|{=\hbox { B}}\wedge \hbox {C} \hbox { iff }\, u\,\left| \!\,{=\hbox { B} \hbox { and}}\, u\, \right| \!\,{=\,\hbox { C}}$

T(iii):

$u\,|{=\hbox { B}}\vee \hbox {C iff }\, u\,\left| \!\,{=\hbox { B or}\, u}\, \right| \!\,{=\hbox { C}}$

T(iv):

$u\,|{=\lnot \hbox {B iff not }} { {v}}\,|{=\hbox { B for any }} v \hbox { for which}\, u\le { {v}}$

T(v):

$u\,|{=\hbox { B}}\supset \hbox {C iff } { {v}}\,\left| {={\hbox { C whenever}}\, { {v}}} \,\right| {=\hbox { B} \hbox { and}}\, u\,\le { {v}}.$

We may also employ the following clause for $\bot$ in place of the clause for $\lnot$:

T(vi):

$\hbox {never}\, u\,|\!=\bot .$

We adopt some standard logical terminology. A is said to be a consequence of $\Delta$—in symbols, $\Delta {\vert }\!=\hbox { A}$—if, for any model M and point $u$ of M, A is true at $u$ in M whenever $\Delta$ (i.e. each formula of $\Delta )$ is true at $u$ in M. We say that A is valid—in symbols, ${\vert }\!= \hbox {A}$—if A is a consequence of the empty set of formulas $\oslash$, i.e. if A is true at any point in any model; and we say that A is equivalent to B—in symbols,$\hbox { A}=\!{\vert }{\vert }\!=\hbox { B}$—if $\hbox {A}{\vert }\!=\hbox { B}$ and $\hbox {B }{\vert }\!=\hbox { A}$. The formulas of $\Delta$ are said to satisfiable if for some model M and point $u$ of M, $\Delta$ is true at $u$ in M; and the formulas of $\Delta$ are said to be compatible with the formula A (or with the set of formulas $\Gamma )$ if $\Delta \quad \cup$ {A} (or $\Delta \quad \cup \quad \Gamma )$ is satisfiable (and similarly for unsatisfiability). Clearly, the formulas $\Delta$ are satisfiable iff not $\Delta {\vert }\!= \bot$.

1.2 The Compatibilist Semantics

Under the compatibilist semantics, we take a model M to be a triple ($U$, $\circ$, $\upvarphi )$, where:

C(i):

$U$ (‘points’ or ‘uses’) is a non-empty set;

C(ii):

$\circ$ (compatibility) is a reflexive and symmetric relation on $U$;

C(iii):

$\upvarphi$ (valuation) is a function from SL into

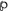
(U).

Our intuitive understanding of $U$ and of $\upvarphi$ is as before.

Relative to a model M, truth of a formula A under a use $u\, (u\, {\vert }=\hbox { A}$) is defined by the following clauses:

T(i):

$u\,|{=\hbox { p iff }} u\in \upvarphi \left( \hbox { p} \right)$

T(ii):

$u\,|{=\hbox { B}}\wedge \hbox {C iff }\, u\,\left| {=\hbox { B and}\, u} \,\right| {=\hbox { C}}$

T(iii):

$u\,|{=\hbox { B}}\vee \hbox {C iff } u\,\left| {=\hbox { B or}\, u}\, \right| {=\hbox { C}}$

T(iv):

$u\,\left| {=\lnot \hbox {B iff not}\, { {v}}}\, \right| {=\hbox { B for any}}\, { {v}}\, \hbox {for which }\, u\circ { {v}}$

T*(v):

$u\,|{=\hbox { B}}\supset \hbox {C iff either }\left( \hbox {a} \right) \,u\,\left| {=\hbox { B and}\, u} \,\right| {=\hbox { C or }}\left( \hbox {b} \right) { {v}}\left| {=\hbox { C whenever }} { {v}}\, \right| {=\hbox { B and }} u\circ { {v}}$

T(vi):

$\hbox {never}\, u\,|{=\bot } .$

There are two main changes from the semantics for intuitionist logic. The first is in the substitution of the reflexive and symmetric relation $\circ$ for the reflexive and transitive relation $\le$ (along with the elimination of the hereditary condition); and the second is in the modification of the clause for the conditional (T*(v) in place of T(v)). The new clause might be regarded as a form of the ‘closest world’ semantics; for either the closest B-world is the actual world $u$, in which case C must also be true in $u$, or else the closest B-worlds are the worlds compatible with the actual world, in which case C must also be true in those worlds. Thus there are two ways in which the conditional B $\supset$ C can be true at a point—either ‘truth-functionally’ with both B and C true or ‘strictly’ with C true whenever B is true.¹⁷

Logical notions such as validity and consequence transfer in the obvious way to the present semantics. When there is a need to distinguish the semantics in question, we shall talk of compatibilitist or C-validity versus intuitionistic or I-validity; and similarly when other semantical frameworks are in question. We may also define a natural notion of disagreement, where two sets of formulas $\Delta$ and $\Gamma$ are said to be in disagreement—$\Delta \bullet \Gamma$—if in any model, never $u\, {\vert }\!= \Delta$, ${ {v}}\ {\vert }\!= \Gamma$ and $u \circ { {v}}$.

We present three central results—Completeness, Inclusion and Possibility—which we state without proof (further details will be provided elsewhere). Let CL (compatibilist logic) be the system defined by the following axioms and rules of inference (with $\bot$ as primitive):

1.3 Axioms

• A1. $\hbox {A}\wedge (\hbox {A}\supset \hbox {B})\supset \hbox {B}$

• A2. $\hbox {A}\supset ((\hbox {A}\supset \hbox {B})\supset \hbox {B})$

• A3. $\hbox {A}\supset (\hbox {B}\supset \hbox {B})$

• A4. $\hbox {A}\wedge \hbox {B}\supset (\hbox {A}\supset \hbox {B})$

• A5. $(\hbox {A}\supset \hbox {B}\wedge \hbox {B}\supset \hbox {C})\supset \hbox {B}\vee (\hbox {A}\supset \hbox {C})$

• A6. $\hbox {A}\wedge \hbox {B}\supset \hbox {A}$

• A7. $\hbox {A}\wedge \hbox {B}\supset \hbox {B}$

• A8. $(\hbox {A}\supset \hbox {B}\wedge \hbox {A}\supset \hbox {C})\supset (\hbox {A}\supset \hbox {B}\wedge \hbox {C})$

• A9. $\hbox {A}\supset \hbox {A}\vee \hbox {B}$

• A10. $\hbox {B}\supset \hbox {A}\vee \hbox {B}$

• A11. $((\hbox {A}\supset \hbox {C})\wedge (\hbox {B}\supset \hbox {C}))\supset ((\hbox {A}\vee \hbox {B})\supset \hbox {C})$

• A12. $\hbox {A}\wedge (\hbox {B}\vee \hbox {C})\supset (\hbox {A}\wedge \hbox {B})\vee (\hbox {A}\wedge \hbox {C}).$

• A13. $\bot \supset \hbox {A}.$

1.4 Rules of inference

• R1. $\hbox {A},\hbox { A}\supset \hbox {B}/\hbox { B}$

• R2. $\hbox {A},\hbox { B }/\hbox { A}\wedge \hbox {B}$

• R3. $\hbox {A}\supset \hbox {B},\hbox { B}\supset \hbox {C }/\hbox { A}\supset \hbox {C}$

Theorem 1

(Completeness) A formula A is a theorem of the system CL iff it is valid under the compatiblist semantics.

The above axiom system is perhaps a little unnatural, but I believe a much more natural system can be obtained using disagreement—$\Delta \, {\bullet } \,\Gamma$—as the basic meta-logical primitive in place of theoremhood; and, indeed, the resulting system may well be regarded as a basic way to formalize the logic of disagreement.

We also have:

Theorem 2

(Inclusion) Any theorem of compatibilist logic is a theorem of intuitionistic logic.

This inclusion is proper, since $\hbox {p}\wedge \lnot (\hbox {p}\wedge \hbox {q})\supset \lnot \hbox {q}$ is a theorem of intuitionistic logic but not of compatibility logic.

To state the third result, we need some terminology from Fine (2008). A (collective) response is a sequence A(p), A(p),..., A(p) of formulas constructed from the sentence letter p; and $\hbox {A}_{1}, \hbox {A}_{2}, \ldots , \hbox {A}_\mathrm{n}$ is said to be a (collective)response to $\hbox {B}_{1}, \hbox {B}_{2}, \ldots , \hbox {B}_\mathrm{n}$ if $\hbox {A}_{1}, \hbox {A}_{2}, \ldots , \hbox {A}_\mathrm{n}$ are respectively of the form $\hbox {A}_{1}(\hbox {B}_{1}),\hbox { A}_{2}(\hbox {B}_{2}), \ldots ,\hbox { A}_\mathrm{n}(\hbox {B}_\mathrm{n})$, where $\hbox {A}_{1}\hbox {(p)},\hbox { A}_{2}\hbox {(p)}, \ldots ,\hbox { A}_\mathrm{n}\hbox {(p)}$ is a collective response. We say that the collective response $\hbox {A}_{1}, \hbox {A}_{2}, \ldots ,\hbox { A}_\mathrm{n}$ is sharp if:

• (i) $\hbox {A}_\mathrm{i} \ne \hbox {A}_\mathrm{j}\ \hbox {for some i},\hbox { j}\le \hbox {n};$

• (ii) $\hbox {A}_\mathrm{i}\ \hbox {is incompatible with A}_\mathrm{j}\ \hbox {or A}_\mathrm{i} =\hbox { A}_\mathrm{j}\ \hbox {whenever 1}\le \hbox {i }<\hbox { j}\le \hbox {n}.$

We may similarly talk of a sharp response to $\hbox {B}_\mathrm{1}$, $\hbox {B}_\mathrm{2}$, ..., $\hbox {B}_\mathrm{n}$. We use:

$$\begin{aligned} \hbox {I}^{*}\left( {\hbox {A}_\mathrm{1} ,\hbox { A}_\mathrm{2} ,\ldots ,\hbox { A}_\mathrm{n} } \right) \hbox { for }\lnot \bigwedge _{\hbox {1}\le \hbox {i}\le \hbox {n}} (\lnot \hbox {A}_\mathrm{i} \vee \lnot \lnot \hbox {A}_\mathrm{i} ). \end{aligned}$$

The indeterminacy operator I* can then be employed to evade the impossibility result of Fine (2008).

Theorem 3

(Possibility) For n $\ge$ 2, I*(p$_{1}$, p$_{2}$, ..., p$_{\mathrm{{n}}+1}$) is compatible with $\{ \mathrm{{p}}_1, \lnot \mathrm {p}_{\mathrm{{n}}+1}\}$ and incompatible with any sharp response to p$_{1}$, p$_{2}$, ..., p$_{\mathrm{{n}}+1}$.

This is a fundamental result and shows how vagueness, as we naturally conceive it, is indeed possible.