Abstract
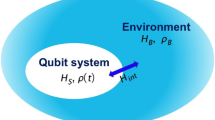
There is a rich variety of physics underlying the fundamental gating operations for quantum information processing (QIP). A key aspect of a QIP system is how noise may enter during quantum operations and how suppressing or correcting its effects can best be addressed. Quantum control techniques have been developed to specifically address this effort, although a detailed classification of the compatibility of controls schemes with noise sources found in common quantum systems has not yet been performed. This work numerically examines the performance of modern control methods for suppressing decoherence in the presence of noise forms found in viable quantum systems. The noise-averaged process matrix for controlled one-qubit and two-qubit operations are calculated across noise found in systems driven by Markovian open quantum dynamics. Rather than aiming to describe the absolute best control scheme for a given physical circumstance, this work serves instead to classify quantum control behavior across a large class of noise forms so that opportunities for improving QIP performance may be identified.



Similar content being viewed by others
References
Clark, C.R., Metodi, T.S., Gasster, S.D., Brown, K.R.: Resource requirements for fault-tolerant quantum simulation: the ground state of the transverse ising model. Phys. Rev. A 79, 062314–062323 (2009)
Jones, N.C., Van Meter, R., Fowler, A.G., McMahon, P.L., Kim, J., Ladd, T.D., Yamamoto, Y.: Layered architecture for quantum computing. Phys. Rev. X 2, 031007–031034 (2012)
Devitt, S.J., Stephens, A.M., Munro, W.J., Nemoto, K.: Requirements for fault-tolerant factoring on an atom-optics quantum computer. Nat. Commun. (2013). doi:10.1038/ncomms3524
Nielsen, M.A., Chuang, I.L.: Quantum Computation and Quantum Information. Cambridge Univeristy Press, Cambridge (2000)
Brif, C., Chakrabarti, R., Rabitz, H.: Control of quantum phenomena: past, present and future. N. J. Phys. 12(7), 075008–75076 (2010)
Glaser, J.S., Boscain, U., Calarco, T., Koch, P.C., Köckenberger, W., Kosloff, R., Kuprov, I., Luy, B., Schirmer, S., Schulte-Herbrüggen, T., Sugny, D., Frank Wilhelm, K.: Training schrödinger’s cat: quantum optimal control. Eur. Phys. J. D 69(12), 1–24 (2015)
Siegel, R., Nakashima, T.T., Wasylishen, R.E.: Signal-to-noise enhancement of NMR spectra of solids using multiple-pulse spin-echo experiments. Concepts Magn. Reson. Part A 26A(2), 62–77 (2005)
Wimperis, S.: Broadband, narrowband, and passband composite pulses for use in advanced NMR experiments. J. Magn. Reson. Ser. A 109(2), 221–231 (1994)
Rego, L.G.C., Santos, L.F., Batista, V.S.: Coherent control of quantum dynamics with sequences of unitary phase-kick pulses. Ann. Rev. Phys. Chem. 60(1), 293–320 (2009)
Viola, L., Knill, E., Lloyd, S.: Dynamical decoupling of open quantum systems. Phys. Rev. Lett. 82, 2417–2421 (1999)
Khodjasteh, K., Viola, L.: Dynamically error-corrected gates for universal quantum computation. Phys. Rev. Lett. 102, 080501–080505 (2009)
Viola, L., Lloyd, S.: Dynamical suppression of decoherence in two-state quantum systems. Phys. Rev. A 58, 2733–2744 (1998)
Khodjasteh, K., Viola, L.: Dynamical quantum error correction of unitary operations with bounded controls. Phys. Rev. A 80, 032314–032333 (2009)
Kabytayev, C., Green, T.J., Khodjasteh, K., Biercuk, M.J., Viola, L., Brown, K.R.: Robustness of composite pulses to time-dependent control noise. Phys. Rev. A 90, 012316–012325 (2014)
Khodjasteh, K., Bluhm, H., Viola, L.: Automated synthesis of dynamically corrected quantum gates. Phys. Rev. A 86, 042329–042336 (2012)
Merrill, J.T., Brown, K.R.: Progress in Compensating Pulse Sequences for Quantum Computation. Wiley, NewYork (2014)
Suzuki, M.: General theory of higher-order decomposition of exponential operators and symplectic integrators. Phys. Lett. A 165, 387–395 (1992)
Dawson, C.M., Nielsen, M.A.: The solovay–kitaev algorithm. Quant. Inf. Comp. 6, 81–95 (2006)
Brown, K.R., Harrow, A.W., Chuang, I.L.: Arbitrarily accurate composite pulse sequences. Phys. Rev. A 70, 052318–052322 (2004)
Brown, K.R., Harrow, A.W., Chuang, I.L.: Erratum: Arbitrarily accurate composite pulse sequences [Phys. Rev. A 70, 052318 (2004)]. Phys. Rev. A 72, 039905 (2005)
Ho, T.-S., Dominy, J., Rabitz, H.: Landscape of unitary transformations in controlled quantum dynamics. Phys. Rev. A 79, 013422–013438 (2009)
Rabitz, H., Hsieh, M., Rosenthal, C.: Landscape for optimal control of quantum-mechanical unitary transformations. Phys. Rev. A 72, 052337–052342 (2005)
Hsieh, M., Rabitz, H.: Optimal control landscape for the generation of unitary transformations. Phys. Rev. A 77, 042306–042311 (2008)
Hsieh, M., Rebing, W., Rabitz, H.: Topology of the quantum control landscape for observables. J. Chem. Phys. 130(10), 10410–10416 (2009)
Rabitz, H., Ho, T.-S., Hsieh, M., Kosut, R., Demiralp, M.: Topology of optimally controlled quantum mechanical transition probability landscapes. Phys. Rev. A 74, 012721–012730 (2006)
Bruer, H.-P.: The Theory of Open Quantum Systems. Oxford Univ. Press, Oxford (2002)
Hocker, D., Brif, C., Grace, M.D., Donovan, A., Ho, T.-S., Tibbetts, K.M., Wu, R., Rabitz, H.: Characterization of control noise effects in optimal quantum unitary dynamics. Phys. Rev. A 90, 062309–062318 (2014)
Brockwell, P.J., Davis, R.J.: Introduction to Time Series and Forecasting, 2nd edn. Springer, NewYork (2002)
Stark, H., Woods, J.W.: Probability, Random Processes, and Estimation Theory for Engineers. Prentice Hall, Upper Saddle River (1986)
Khaneja, N., Brockett, R., Glaser, S.J.: Time optimal control in spin systems. Phys. Rev. A 63, 032308–032321 (2001)
Boscain, U., Chitour, Y.: Time-optimal synthesis for left-invariant control systems on so(3). SIAM J. Control Optim. 44(1), 111–139 (2005)
Beltrani, V., Dominy, J., Ho, T.-S., Rabitz, H.: Exploring the top and bottom of the quantum control landscape. J. Chem. Phys. 134(19), 194106 (2011)
Rothman, A., Ho, T.-S., Rabitz, H.: Exploring the level sets of quantum control landscapes. Phys. Rev. A 73, 053401–053410 (2006)
David, H., Herschel R.: Meeting a high-performance quantum technology primary objective in the presence of secondary objectives. Phys. Rev. A (2016, submitted)
Uhrig, G.S.: Keeping a quantum bit alive by optimized \(\pi \)-pulse sequences. Phys. Rev. Lett. 98, 100504–100508 (2007)
Khodjasteh, K., Lidar, D.A., Viola, L.: Arbitrarily accurate dynamical control in open quantum systems. Phys. Rev. Lett. 104, 090501–090505 (2010)
Hocker, D., Kosut, R., Rabitz, H.: Peet: a matlab tool for estimating physical gate errors in quantum information processing systems. Quant. Inf. Process. (2016). doi:10.1007/s11128-016-1337-5
Martinis, J.M., Nam, S., Aumentado, J., Lang, K.M., Urbina, C.: Decoherence of a superconducting qubit due to bias noise. Phys. Rev. B 67, 094510–094520 (2003)
Martinis, J.M.: Superconducting phase qubits. Quant. Inf. Process. 8(2–3), 81–103 (2009)
Strauch, F.W., Johnson, P.R., Dragt, A.J., Lobb, C.J., Anderson, J.R., Wellstood, F.C.: Quantum logic gates for coupled superconducting phase qubits. Phys. Rev. Lett. 91, 167005–167009 (2003)
DiCarlo, L., Chow, J.M., Gambetta, J.M., Bishop, L.S., Johnson, B.R., Schuster, D.I., Majer, J., Blais, A., Frunzio, L., Girvin, S.M., Schoelkopf, R.J.: Demonstration of two-qubit algorithms with a superconducting quantum processor. Nature 460(7252), 240–244, 07 (2009)
Bialczak, R.C., McDermott, R., Ansmann, M., Hofheinz, M., Katz, N., Lucero, E., Neeley, M., O’Connell, A.D., Wang, H., Cleland, A.N., Martinis, J.M.: 1/f Flux noise in Josephson phase qubits. Phys. Rev. Lett. 99, 187006–187010 (2007)
Martinis, J.M., Cooper, K.B., McDermott, R., Steffen, M., Ansmann, M., Osborn, K.D., Cicak, K., Oh, S., Pappas, D.P., Simmonds, R.W., Yu, C.C.: Decoherence in Josephson qubits from dielectric loss. Phys. Rev. Lett. 95, 210503–210507 (2005)
Wineland, D.J., Monroe, C., Itano, W.M., Leibfried, D., King, B.E., Meekhof, D.M.: Experimentalissuesincoherent quantum-state manipulation of trapped atomic ions. J. Res. Natl. Inst. Stand. Technol. 103(3), 259–328 (1998)
Leibfried, D., DeMarco, B., Meyer, V., Lucas, D., Barrett, M., Britton, J., Itano, W.M., Jelenkovic, B., Langer, C., Rosen-band, T., Wineland, D.J.: Experimental demonstration of a robust, high-fidelity geometric two ion-qubit phase gate. Nature 422(6930), 412–415, 03 (2003)
Schneider, S., Milburn, G.J.: Decoherence in ion traps due to laser intensity and phase fluctuations. Phys. Rev. A 57, 3748–3752 (1998)
Saffman, M., Walker, T.G., Mølmer, K.: Quantum information with Rydberg atoms. Rev. Mod. Phys. 82, 2313–2363 (2010)
Saffman, M., Walker, T.G.: Analysis of a quantum logic device based on dipole-dipole interactions of optically trapped Rydberg atoms. Phys. Rev. A 72, 022347–022368 (2005)
Taylor, J.M., Petta, J.R., Johnson, A.C., Yacoby, A., Marcus, C.M., Lukin, M.D.: Relaxation, dephasing, and quantum control of electron spins in double quantum dots. Phys. Rev. B 76, 035315–035332 (2007)
Coish, W.A., Loss, D.: Singlet-triplet decoherence due to nuclear spins in a double quantum dot. Phys. Rev. B 72, 125337–125349 (2005)
Hu, X., Sarma, S.D.: Charge-fluctuation-induced dephasing of exchange-coupled spin qubits. Phys. Rev. Lett. 96, 100501–100505 (2006)
Tibbetts, K.W.M., Brif, C., Grace, M.D., Donovan, A., Hocker, D.L., Ho, T.-S., Wu, R.-B., Rabitz, H.: Exploring the tradeoff between fidelity and time optimal control of quantum unitary transformations. Phys. Rev. A 86, 062309–062325 (2012)
Acknowledgments
This material is based upon work supported by the (D.H.) National Science Foundation Graduate Research Fellowship Program under Grant No. (DGE 1148900), (H.R.for the basic concepts) National Science Foundation (CHE-1058644) and (Y.Z., R.K., T.B) ARO-MURI (W911NF-11-1-2068). This work was also supported by the (Y.Z., R.K., T.B) (H.R. for the illustrations) Intelligence Advanced Research Projects Activity (IARPA) via Department of Interior National Business Center Contract No. D11PC20165. The US Government is authorized to reproduce and distribute reprints for governmental purposes notwithstanding any copyright annotation thereon. The views and conclusions contained herein are those of the authors and should not be interpreted as necessarily representing the official policies or endorsements, either expressed or implied, of IARPA, DoI/NBC, or the US Government.
Author information
Authors and Affiliations
Corresponding author
Appendix
Appendix
1.1 Trotter–Suzuki and Solovay–Kitaev control
Both TS and SK methods utilize the identity that imperfect rotations by integer units of \(\pi \) can accumulate and eventually cancel out the error through [17]
For TS control, sets of expansion coefficients \(p_{jn}\) are chosen such that
for sets of non-commuting matrices \(\{A_i\}\). With individual qubit rotation in the X-Y plane, it can be shown that a even-ordered correction sequences \(R_{TS2k}\) can be constructed through the identities
The recursive formula for the sequence in Eq. (47) uses only imperfect rotations along special angles \(\phi _j\), and relies on the initial mapping \(\hat{S}_1\) and the concatenated formula in Eq. (49). The angles of rotation are chosen to satisfy the decomposition in (46) and are given by
The identities in Eq. (47) and (49) alongside angle choices from Eq. (50) yield the second sequence N2 of Eq. (39). Due to the long length of higher-order sequences, only N2 is simulated in this work. For example, the fourth-order sequence is given by
SK methods utilize sets of \(2\pi \) rotations that rely on suppressing errors related to commutators of lower-order commutators [18]. The first-order sequence SK1 of Eq. (40) can be shown to cancel first-order errors to \( M(\theta ,\phi )\) for rotation angle choice of \(\phi _1 = \cos ^{-1}\left( -\frac{\theta }{4\pi }\right) \):
The higher-order SK sequences concatenate lower-order sequences and cancel subsequently higher-order errors related to commutators of lower orders. This suppression relies on an identity from the proof of the Solovay–Kitaev theorem that gives a relation between commutators of the lower-order rotation operators,
Equation (54) yields the second-order terms \(SK_{2} (\phi _2) \) of Eq. (43), and leads to the full error-correction sequence SK2 of Eq. (42).
1.2 Optimal control
Unconstrained primitive control utilizes an optimization to locate controls beyond the amplitude constraints of each system. Here we calculate the necessary gradient \(\delta \chi _{ {\mathcal {E}}}/ \delta c(t)\) for a given control c(t) . The gradient of J with respect to c(t) can first be expressed as
where the explicit time dependence upon \(\chi _{{\mathcal {E}}}\) has now been shown for clarity. Calculation of \(\delta \chi _{{\mathcal {E}}}(T) / \delta c(t)\) can be performed by examining the time evolution of a linear disturbance to \(\chi _{{\mathcal {E}}}(T)\). First note that analogous to the unitary evolution operator in a closed quantum system, the time evolution of \(\chi _{{\mathcal {E}}}(t)\) follows a similar equation of motion:
Inserting a variation \(\delta \chi _{{\mathcal {E}}}(t)\) into Eq. (56) and keeping first-order terms yield an equation for evolution of dynamical disturbances to the process matrix as
where the \(\delta L(t)\) corresponds to a variation of the control in the superoperator.
For example, in the case of an X rotation in the RWA (i.e., Eq. (6), \(\phi =0\), \(\delta \omega (t) = 0\)), this term is expressed as
Equation (57) is an inhomogeneous differential equation and has a homogenous solution with Green’s function \(G(t,t')\),
The above equation can be identified as the time evolution of the process matrix in Eq. (56), and \(G(t,t')\) represents evolution of the process matrix from time point \(t'\) to time t. Expressing this Green’s function in a separable form \(G(t,t')\) relates it to the process matrix,
The inhomogeneous solution to \(\delta \chi _{{\mathcal {E}}}(T)\) can now be expressed as
The gradient \(\delta \chi _{{\mathcal {E}}}(T) / \delta c(t)\) can then be identified from the total first-order variation in Eq. (62) as
and the entire gradient of J can then be explicitly written from Eqs. (63) and (55) as
Optimal control pulses were found through the D-MORPH algorithm of Eqs. (34) and (35) to minimize J. As the quantum dot qubit system was excluded from optimal control, all systems were simulated under the RWA, in which controls were either a bias pulse in the Z direction, or the amplitudes for an on-resonance field in the XY plane. To explore the performance beyond primitive gate operations with constant amplitudes, a Gaussian temporal envelope amplitude was instead used for initial guesses \(c_i(t)\). For rotation through angle \(\theta \), the initial guess is expressed as
Rights and permissions
About this article
Cite this article
Hocker, D., Zheng, Y., Kosut, R. et al. Survey of control performance in quantum information processing. Quantum Inf Process 15, 4361–4390 (2016). https://doi.org/10.1007/s11128-016-1406-9
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11128-016-1406-9