Skip to main content
SpringerLink
Log in

Periodically kicked feedforward chains of simple excitable FitzHugh–Nagumo neurons

  • Original Paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

Abstract

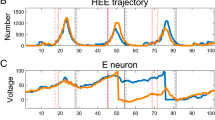
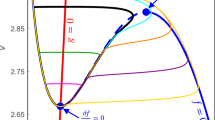
This article communicates results on regular depolarization cascades in periodically kicked feedforward chains of excitable two-dimensional FitzHugh–Nagumo systems driven by sufficiently strong excitatory forcing at the front node. The study documents a parameter exploration by way of changes to the forcing period, upon which the dynamics undergoes a transition from simple depolarization to more complex behavior, including the emergence of mixed-mode oscillations. Both rigorous studies and careful numerical observations are presented. In particular, we provide rigorous proofs for existence and stability of periodic traveling waves of depolarization, as well as the existence and propagation of a simple mixed-mode oscillation that features depolarization and refraction in alternating fashion. Detailed numerical investigation reveals a mechanism for the emergence of complex mixed-mode oscillations featuring a potentially high number of large amplitude voltage spikes interspersed by an occasional small amplitude reset that fails to cross threshold. Further careful numerical investigation provides insights into the propagation of this complex phenomenology in the downstream, where we see an effective filtration property of the network; the latter amounts to a successive reduction in the complexity of mixed-mode oscillations down the chain.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17
Fig. 18
Fig. 19
Fig. 20
Fig. 21
Fig. 22
Fig. 23
Fig. 24

Similar content being viewed by others

Availability of data and materials

Not Applicable.

Notes

  1. Of interest for the feedforward chain network studied here, how do MMOs propagate downstream (do they regularize or become more irregular?), and are there regimes in which wave propagation/signal transmission downstream is altogether blocked?

  2. See our discussion of the state of affairs for \(\alpha \) only slightly smaller than \(\alpha _2\) in Remark 11.

References

  1. Hodgkin, A.L., Huxley, A.F.: A quantitative description of membrane current and its application to conduction and excitation in nerve. J. Physiol. 117(4), 500–544 (1952). https://doi.org/10.1113/jphysiol.1952.sp004764

    Article  Google Scholar 

  2. FitzHugh, R.: Impulses and physiological states in theoretical models of nerve membrane. Biophys. J . 1(6), 445–466 (1961). https://doi.org/10.1016/s0006-3495(61)86902-6

    Article  Google Scholar 

  3. Nagumo, J., Arimoto, S., Yoshizawa, S.: An active pulse transmission line simulating nerve axon. Proc. IRE 50(10), 2061–2070 (1962). https://doi.org/10.1109/jrproc.1962.288235

    Article  Google Scholar 

  4. Fenton, F., Cherry, E.: Models of cardiac cell. Scholarpedia 3(8), 1868 (2008). https://doi.org/10.4249/scholarpedia.1868

    Article  Google Scholar 

  5. Hastings, S.P.: Some mathematical problems from neurobiology. Am. Math. Mon. 82(9), 881–895 (1975). https://doi.org/10.1080/00029890.1975.11993972

    Article  MathSciNet  MATH  Google Scholar 

  6. Hastings, S.P.: On the existence of of homoclinic and periodic orbits for the Fitzhugh–Nagumo equations. Q. J. Math. 27(1), 123–134 (1976). https://doi.org/10.1093/qmath/27.1.123

    Article  MathSciNet  MATH  Google Scholar 

  7. McKean, H.P.: Nagumo’s equation. Adv. Math. 4(3), 209–223 (1970). https://doi.org/10.1016/0001-8708(70)90023-x

  8. Jones, C.K.R.T.: Stability of the travelling wave solution of the FitzHugh–Nagumo system. Trans. Am. Math. Soc. 286(2), 431–431 (1984). https://doi.org/10.1090/s0002-9947-1984-0760971-6

    Article  MathSciNet  MATH  Google Scholar 

  9. Jones, C., Kopell, N., Langer, R.: Construction of the Fitzhugh–Nagumo pulse using differential forms. In: Aris, R., Aronson, D.G., Swinney, H.L. (eds.) Patterns and Dynamics in Reactive Media, pp. 101–115. Springer, New York (1991)

    Chapter  Google Scholar 

  10. Rauch, J., Smoller, J.: Qualitative theory of the FitzHugh–Nagumo equations. Adv. Math. 27(1), 12–44 (1978). https://doi.org/10.1016/0001-8708(78)90075-0

    Article  MathSciNet  MATH  Google Scholar 

  11. Rinzel, J., Keaner, J.P.: Hopf bifurcation to repetitive activity in nerve. SIAM J. Appl. Math. 43(4), 907–922 (1983). https://doi.org/10.1137/0143058

    Article  MathSciNet  MATH  Google Scholar 

  12. Ambrosio, B.: Hopf bifurcation in an oscillatory-excitable reaction–diffusion model with spatial heterogeneity. Int. J. Bifurc. Chaos 27(05), 1750065 (2017). https://doi.org/10.1142/s0218127417500651

    Article  MathSciNet  MATH  Google Scholar 

  13. Ambrosio, B., Francoise, J.-P.: Propagation of bursting oscillations. Philosophical Transactions of the Royal Society A: Mathematical, Physical and Engineering Sciences 367(1908), 4863–4875 (2009). https://doi.org/10.1098/rsta.2009.0143

    Article  MathSciNet  MATH  Google Scholar 

  14. Maia, P.D., Kutz, J.N.: Identifying critical regions for spike propagation in axon segments. J. Comput. Neurosci. 36(2), 141–155 (2013). https://doi.org/10.1007/s10827-013-0459-3

    Article  MathSciNet  Google Scholar 

  15. Jean-René, C., Fernandez, B.: Dynamics of Coupled Map Lattices and of Related Spatially Extended Systems. Springer, Berlin (2005). https://doi.org/10.1007/b103930

    Book  MATH  Google Scholar 

  16. Afraimovich, V.: Some Topological Properties of Lattice Dynamical Systems, pp. 153–179. Springer, Berlin, Heidelberg (2005). https://doi.org/10.1007/11360810_7

    Book  Google Scholar 

  17. Baesens, C.: Spatially extended systems with monotone dynamics: continuous time. In: Lecture Notes in Physics, pp. 241–263. Springer, Berlin, Heidelberg (2005)

  18. Floría, L.M., Baesens, C., Gómez-Gardeñes, J.: The Frenkel–Kontorova model. In: Lecture Notes in Physics, pp. 209–240. Springer, Berlin, Heidelberg (2005). https://doi.org/10.1007/11360810_9

  19. Ermentrout, G.B., Terman, D.H.: Mathematical Foundations of Neuroscience. Springer, New York, London (2010). https://doi.org/10.1007/978-0-387-87708-2

    Book  MATH  Google Scholar 

  20. Izhikevich, E.M.: Dynamical Systems in Neuroscience. Computational neuroscience Dynamical systems in neuroscience. MIT Press, Cambridge (2007). https://books.google.com/books?id=kVjM6DFk-twC

  21. Chariker, L., Shapley, R., Young, L.-S.: Orientation selectivity from very sparse LGN inputs in a comprehensive model of macaque v1 cortex. J. Neurosci. 36(49), 12368–12384 (2016). https://doi.org/10.1523/jneurosci.2603-16.2016

    Article  Google Scholar 

  22. Chariker, L., Young, L.-S.: Emergent spike patterns in neuronal populations. J. Comput. Neurosci. 38(1), 203–220 (2014). https://doi.org/10.1007/s10827-014-0534-4

    Article  MATH  Google Scholar 

  23. Gerstner, W., Kistler, W.M.: Spiking Neuron Models: Single Neurons, Populations, Plasticity. Cambridge University Press, Cambridge, New York (2002). https://books.google.com/books?id=Rs4oc7HfxIUC

  24. Keener, J., Sneyd, J.: Mathematical Physiology. Springer, New York (2009). https://doi.org/10.1007/978-0-387-75847-3

    Book  MATH  Google Scholar 

  25. Fenichel, N.: Geometric singular perturbation theory for ordinary differential equations. J. Differ. Equ. 31(1), 53–98 (1979). https://doi.org/10.1016/0022-0396(79)90152-9

    Article  MathSciNet  MATH  Google Scholar 

  26. Jones, C.K.R.T.: Geometric singular perturbation theory. In: Dynamical Systems, pp. 44–118. Springer, Berlin, Heidelberg (1995). https://doi.org/10.1007/bfb0095239

  27. Krupa, M., Szmolyan, P.: Extending geometric singular perturbation theory to nonhyperbolic points–fold and canard points in two dimensions. SIAM J. Math. Anal. 33(2), 286–314 (2001). https://doi.org/10.1137/s0036141099360919

    Article  MathSciNet  MATH  Google Scholar 

  28. Broens, M., Bar-Eli, K.: Canard explosion and excitation in a model of the Belousov–Zhabotinskii reaction. J. Phys. Chem. 95(22), 8706–8713 (1991). https://doi.org/10.1021/j100175a053

    Article  Google Scholar 

  29. Rotstein, H.G., Kopell, N., Zhabotinsky, A.M., Epstein, I.R.: Canard phenomenon and localization of oscillations in the Belousov–Zhabotinsky reaction with global feedback. J. Chem. Phys. 119(17), 8824–8832 (2003). https://doi.org/10.1063/1.1614752

    Article  Google Scholar 

  30. Rotstein, H.G., Wechselberger, M., Kopell, N.: Canard induced mixed-mode oscillations in a medial entorhinal cortex layer II stellate cell model. SIAM J. Appl. Dyn. Syst. 7(4), 1582–1611 (2008). https://doi.org/10.1137/070699093

    Article  MathSciNet  MATH  Google Scholar 

  31. V-Ghaffari, B., Kouhnavard, M., Elbasiouny, S.M.: Mixed-mode oscillations in pyramidal neurons under antiepileptic drug conditions. PLOS ONE 12(6), 0178244 (2017). https://doi.org/10.1371/journal.pone.0178244

  32. Desroches, M., Guckenheimer, J., Krauskopf, B., Kuehn, C., Osinga, H.M., Wechselberger, M.: Mixed-mode oscillations with multiple time scales. SIAM Rev. 54(2), 211–288 (2012). https://doi.org/10.1137/100791233

    Article  MathSciNet  MATH  Google Scholar 

  33. Wechselberger, M.: Canards. Scholarpedia 2(4), 1356 (2007). https://doi.org/10.4249/scholarpedia.1356

    Article  MathSciNet  Google Scholar 

  34. Benoît, E., Callot, J.F., Diener, F., Diener, M.: Chasse au canard. Collect. Math. 32, 37–119 (1981)

    MathSciNet  MATH  Google Scholar 

  35. Ambrosio, B., Aziz-Alaoui, M.A., Yafia, R.: Canard phenomenon in a slow-fast modified Leslie–Gower model. Math. Biosci. 295, 48–54 (2018). https://doi.org/10.1016/j.mbs.2017.11.003

    Article  MathSciNet  MATH  Google Scholar 

  36. Dumortier, F., Roussarie, R.: Canard cycles and center manifolds. Mem. Am. Math. Soc. 121, 577 (1996). https://doi.org/10.1090/memo/0577

    Article  MathSciNet  MATH  Google Scholar 

  37. Eckhaus, W.: Relaxation oscillations including a standard chase on french ducks. In: Lecture Notes in Mathematics, pp. 449–497. Springer, Berlin, Heidelberg (1983). https://doi.org/10.1007/bfb0062381

  38. Brøns, M., Krupa, M., Wechselberger, M.: Mixed mode oscillations due to the generalized Canard phenomenon. Am. Math. Soc. (2006). https://doi.org/10.1371/journal.pone.0178244

    Article  MATH  Google Scholar 

  39. Krupa, M., Ambrosio, B., Aziz-Alaoui, M.A.: Weakly coupled two-slow-two-fast systems, folded singularities and mixed mode oscillations. Nonlinearity 27(7), 1555–1574 (2014). https://doi.org/10.1088/0951-7715/27/7/1555

    Article  MathSciNet  MATH  Google Scholar 

  40. Fernandez, B., Mintchev, S.M.: Wave generation in unidirectional chains of idealized neural oscillators. J. Math. Neurosci. (2016). https://doi.org/10.1186/s13408-016-0037-x

    Article  MathSciNet  MATH  Google Scholar 

  41. Lanford, O.E., Mintchev, S.M.: Stability of a family of travelling wave solutions in a feedforward chain of phase oscillators. Nonlinearity 28(1), 237–261 (2014). https://doi.org/10.1088/0951-7715/28/1/237

    Article  MathSciNet  MATH  Google Scholar 

  42. Perko, L.: Differential Equations and Dynamical Systems. Springer, New York (2001). https://doi.org/10.1007/978-1-4613-0003-8

    Book  MATH  Google Scholar 

  43. Andronov, A.A.: Theory of Bifurcations of Dynamic Systems on a Plane. Wiley, New York (1973)

    Google Scholar 

  44. Krupa, M., Popović, N., Kopell, N.: Mixed-mode oscillations in three time-scale systems: A prototypical example 7(2), 361–420 (2008). https://doi.org/10.1137/070688912

  45. Krupa, M., Popović, N., Kopell, N., Rotstein, H.G.: Mixed-mode oscillations in a three time-scale model for the dopaminergic neuron 18(1), 015106 (2008). https://doi.org/10.1063/1.2779859

  46. Maesschalck, P.D., Kutafina, E., Popović, N.: Three time-scales in an extended bonhoeffer-van der pol oscillator 26(4), 955–987 (2014). https://doi.org/10.1007/s10884-014-9356-3

  47. Ambrosio, B., Aziz-Alaoui, M.A., Mondal, A., Mondal, A., Sanjeev, Sharma, K., Upadhyay, R.K.: Non trivial dynamics dynamics in the fizhugh-rinzel model and in non-homogeneous oscillatory-excitable reaction-diffusion systems: a few highlights. https://doi.org/10.48550/arXiv.2205.04311

Download references

Acknowledgements

The research of Benjamin Ambrosio (BA) on Neuroscience inspired modeling is partially funded by CNRS (IEA00134). More generally BA wants to thank Le Havre Normandie University, LMAH, Région Normandie, ISCN, the Courant Institute of Mathematical Sciences and New York University, the Hudson School of Mathematics, New York, USA, for material and/or financial support for research in Dynamical Systems, Complex Systems and its applications.

Funding

The research of BA has been partially funded by CNRS (IEA00134), LMAH, Région Normandie and the Hudson School of Mathematics.

Author information

Author notes

  1. Stanislav M. Mintchev contributed equally to this work.

Authors and Affiliations

  1. Department of Mathematics, UNIHAVRE, LMAH, FR CNRS 3335, ISCN, Normandie Univ, 25 Rue Philippe Lebon, 76600, Le Havre, Normandie, France

    Benjamin Ambrosio

  2. The Hudson School of Mathematics, 244 Fifth Avenue, Suite Q224, New York, NY, 10001, USA

    Benjamin Ambrosio

  3. Department of Mathematics, The Cooper Union for the Advancement of Science and Art, 41 Cooper Sq., New York, NY, 10003, USA

    Stanislav M. Mintchev

Authors
  1. Benjamin Ambrosio
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Stanislav M. Mintchev
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Benjamin Ambrosio.

Ethics declarations

Conflict of interest

Authors declare no competing interests

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Springer Nature or its licensor holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Ambrosio, B., Mintchev, S.M. Periodically kicked feedforward chains of simple excitable FitzHugh–Nagumo neurons. Nonlinear Dyn 110, 2805–2829 (2022). https://doi.org/10.1007/s11071-022-07757-0

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-022-07757-0

Keywords

Search

Navigation