Abstract
We obtain analytical approximations for period-1 rotations of both vertically and horizontally excited pendulum using Galerkin projections with elliptic functions (GP), elliptic averaging (EA), method of multiple scales (MMS) and harmonic balance (HB). The application of GP and EA has been extended to parametrically excited pendulum for the first time in this paper, while the results from MMS and HB have been adapted from the existing literature. We compare these approximations with the numerical solution to ascertain their accuracy and identify the correct approximate solution to be used for determining other properties like stability of the solution. This comparison has been made for two different forcing frequencies: one closer to the natural frequency of the pendulum while the other at a higher frequency. We find that the appearance of the period-1 rotation at smaller amplitudes of forcing as a saddle-node bifurcation is best captured by GP and EA, but the approximation for the initial angular displacement and velocity as well as the root- mean-square error over the entire time period using GP and EA deteriorates at larger forcing amplitudes. In the large amplitude regime, HB with one-term approximation gives the best result for lower forcing frequency, while MMS results in the best approximation for the higher frequency. Inclusion of more terms in the harmonic balance approximation leads to an increased accuracy in the entire frequency and amplitude range. This observation holds for both vertical and horizontal excitation. We have also used these approximations to ascertain the stability of period-1 rotation and find that the HB approximation results in the most consistent prediction of stable parameter regimes. Hence, a multi-term HB analysis, which is the simplest and straight forward technique among the methods studied in this paper, leads to the best approximation for period-1 rotation of parametric pendula.





















Similar content being viewed by others
Notes
Averaged Lagrangian gives similar result if the unknowns in the HB approximation are chosen as the generalized coordinates.
Detailed derivations of these equations for general right side function (f) can be found in reference [27].
Largest between the two in terms of absolute value.
Abbreviations
- \(A,{\bar{\omega }}\) :
-
Amplitude and frequency of the excitation
- C :
-
Torsional viscous damping
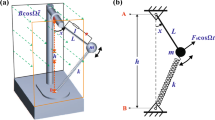
- M, L :
-
Mass of the pendulum bob and length of the pendulum rod
- \(\omega _n\) :
-
Natural frequency of simple undamped pendulum
- \(\beta , p, \omega \) :
-
Non-dimensional system parameters (damping, forcing amplitude and forcing frequency, respectively) with non-dimensionalization using the timescale corresponding to \(\omega _n\)
- c, a, b :
-
Non-dimensional system parameters (damping, stiffness and forcing amplitude, respectively) with non-dimensionalization using the timescale corresponding to \({\bar{\omega }}\)
- \(M_0\) :
-
Initial energy of simple undamped pendulum
- K, E :
-
Elliptic integrals of first and second kind
- \(K^{\prime }\) :
-
Complimentary elliptic integral of the first kind
- k :
-
Elliptic modulus, \(0<k<1\)
- \(\phi \) :
-
Initial phase
- \(\text {am}\) :
-
Jacobi amplitude
- \(\text {sn}, \text {cn}, \text {dn}\) :
-
Jacobi elliptic functions
- Z :
-
Jacobi zeta function
- J :
-
Bessel function of the first kind
- N :
-
Number of harmonics chosen for carrying out harmonic balance
- \(\epsilon \) :
-
Small book-keeping parameter, \(\epsilon \ll 1\)
References
Koch, B.P., Levan, R.W.: Subharmonic and homoclinic bifurcations in parametrically forced pendulum. Phys. D: Nonlinear Phenom. 16, 1–13 (1985)
Bryant, P.J., Miles, J.W.: On a periodically forced, weakly damped pendulum. Part 2: horizontal forcing. J. Aust. Math. Soc. Ser. B Appl. Math. 32(01), 23–41 (1990)
Bryant, P.J., Miles, J.W.: On a periodically forced, weakly damped pendulum. Part 3: vertical forcing. J. Aust. Math. Soc. Ser. B Appl. Math. 32(01), 42–60 (1990)
Capecchi, D., Bishop, S.R.: Periodic oscillations and attracting basins for a parametrically excited pendulum. Dyn. Stab. Syst. 9, 123–143 (1994)
Clifford, M.J., Bishop, S.R.: Rotating periodic orbits of the parametrically excited pendulum. Phys. Lett. A 201, 191–196 (1995)
Xu, X., Wiercigroch, M., Cartmell, M.P.: Rotating orbits of a parametrically-excited pendulum. Chaos Solitons Fract. 23, 1537–1548 (2005)
Lenci, S., Pavlovskaia, E., Rega, G., Wiercigroch, M.: Rotating solutions and stability of parametric pendulum by perturbation method. J. Sound Vib. 310, 243–259 (2008)
Nandakumar, K., Wiercigroch, M., Chatterjee, A.: Optimum energy extraction from rotational motion in a parametrically excited pendulum. Mech. Res. Commun. 43, 7–14 (2012)
Lenci, S., Brocchini, M., Lorenzoni, C.: Experimental rotations of a pendulum on water waves. J. Comput. Nonlinear Dyn. 7(1), 011007 (2012)
Xu, X.: Nonlinear Dynamics of Parametric Pendulum for Wave Energy Extraction. PhD Thesis, University of Aberdeen, UK (2005)
Najdecka, A.: Rotating Dynamics of Pendula Systems for Energy Harvesting from Ambient Vibrations. PhD Thesis, University of Aberdeen, UK (2013)
Wiercigroch, M.: A New Concept of Energy Extraction from Waves via Parametric Pendulor. UK Patent Application, Pending (2010)
Miles, J.: Resonance and symmetry breaking for the pendulum. Phys. D: Nonlinear Phenom. 31(2), 252–268 (1988)
Ganji, D.D., Houman, B.R., Sfahani, M.G., Ganji, S.S.: Approximate traveling wave solutions for coupled Whitham–Broer–Kaup shallow water. Adv. Eng. Softw. 41, 956–961 (2010)
Ganji, D.D., Malvandi, A.: Preparation and theoretical modeling of nanofluids. In: Ganji, D.D., Malvandi, A. (eds.) Heat Transfer Enhancement Using Nanofluid Flow in Microchannels, chapter 3, pp. 71–182, Micro and Nano Technologies, William Andrew Publishing (2016)
He, J.H.: Homotopy perturbation method: a new nonlinear analytical technique. Appl. Math. Comput. 135, 73–79 (2003)
Nayfeh, A.H.: Perturbation Methods. Wiley, New York (2000)
Nayfeh, A.H., Mook, D.T.: Nonlinear Oscillations. Wiley, New York (1979)
Kevorkian, J., Cole, J.D.: Multiple Scales and Singular Perturbation Methods. Springer, New York (1996)
Sanders, J.A., Verhulst, F.: Averaging Methods in Nonlinear Dynamical Systems. Springer, New York (1985)
Mickens, R.E.: Truly Nonlinear Oscillations: Harmonic Balance, Parameter Expansions, Iteration, and Averaging Methods. World Scientific, Chennai (2010)
Mickens, R.E.: Oscillations in Planar Dynamic Systems. World Scientific, New York (1996)
Newland, D.E.: On the methods of Galerkin, Ritz and Krylov–Bogoliubov in the theory of nonlinear vibrations. Int. J. Mech. Sci. 7, 159–172 (1965)
Marinca, V., Herisanu, N.: Nonlinear Dynamical Systems in Engineering. In: Marinca, V., Herisanu, N. (eds.) The Method of Harmonic Balance, Chapter 3, pp. 31–45. Springer, Berlin (2011)
Miles, J.: On resonant rotation of a weakly damped pendulum. J. Sound Vib. 280, 401–406 (2005)
Bryant, P.J., Miles, J.W.: On a periodically forced, weakly damped pendulum. Part 1: applied torque. J. Aust. Math. Soc. Ser. B Appl. Math. 32(01), 1–22 (1990)
Valér Roy, R.: Averaging method for strongly non-linear oscillators with periodic excitations. Int. J. Nonlinear Mech. 29, 737–753 (1994)
Coppola, V.T., Rand, R.H.: Averaging using elliptic functions: approximation of limit cycles. Acta Mech. 81, 125–142 (1990)
Waluya, S.B., van Horssen, W.T.: On approximations of first integrals for a system of weakly nonlinear. Coupled Harmonic Oscil. Nonlinear Dyn. 30, 243–266 (2002)
Waluya, S.B., van Horssen, W.T.: On approximations of first integrals for a strongly nonlinear forced oscillator. Nonlinear Dyn. 33, 225–252 (2003)
Kovacic, I., Cveticanin, L., Zukovic, M., Rakaric, Z.: Jacobi elliptic functions: a review of nonlinear oscillatory application problems. J. Sound Vib. 380, 1–36 (2016)
Xu, X., Wiercigroch, M.: Approximate analytical solutions for oscillatory and rotational motion of a parametric pendulum. Nonlinear Dyn. 47, 311–320 (2007)
Pavlovskaia, E., Horton, B., Wiercigroch, M.: Approximate rotational solutions of pendulum under combined vertical and horizontal excitation. Int. J. Bifurc. Chaos 22, 1250100 (2012)
Zhang, H., Ma, T.W.: Period-one rotating solutions of horizontally excited pendulum based on iterative harmonic balance. Adv. Pure Math. 5(08), 413 (2015)
Ablowitz, M.J., Fokas, A.S.: Complex Variables: Introduction and Applications. Cambridge University Press, Cambridge (2003)
Clifford, M., Bishop, S.R.: Approximating the escape zone for the parametrically excited pendulum. J. Sound Vib. 172(4), 572–576 (1994)
Stewart, I.W., Faulkner, T.R.: Estimating the escape zone for a parametrically excited pendulum-type equation. Phys. Rev. E 62(4), 485661 (2000)
Sofroniou, A., Bishop, S.R.: Breaking the symmetry of the parametrically excited pendulum. Chaos Solitons Fract. 28(3), 673–681 (2006)
Abramowitz, M., Stegun, I.A.: Handbook of Mathematical Functions: With Formulas, Graphs, and Mathematical Tables. Dover, New York (1964)
Farkas, M.: Periodic Motions. Springer, New York (1994)
Jordan, D.W., Smith, P.: Nonlinear Ordinary Differential Equations: An Introduction to Dynamical Systems. Oxford University Press, Oxford (1999)
Byrd, P.F., Friedman, M.D.: Handbook of Elliptic Integrals for Engineer and Physicists. Springer, New York (1954)
Lawden, D.F.: Elliptic Functions and Applications. Springer, New York (1989)
Acknowledgements
We thank the anonymous reviewer for critical comments which helped in improving the clarity of presentation in this paper.
Author information
Authors and Affiliations
Corresponding author
Appendices
Appendix 1: Evaluation of integrals arising in Galerkin projection using elliptic functions
We have outlined here the procedure for carrying out the Galerkin projection using elliptic functions and have also performed the evaluation of a representative integral using contour integration in detail.
1.1 Vertical excitation
To get Eq. (4.8), we multiply both sides of Eq. (4.7) with \( \text {sn}\, \tau \, \text {cn}\, \tau \) and integrate w.r.t. \(\tau \) from \(\tau =0\) to 2K. This yields
There are four integrals in Eq. (8.1). The first integral can be directly taken from the handbook [43] and is
Using the integration identity \(\displaystyle \int _0^{2a_1}h(x) \mathrm{d}x=\int _0^{a_1} h(x) \mathrm{d}x + \int _0^{a_1} h(2a_1-x) \mathrm{d}x\) for a real function h(x) with \(a_1\) as a real number, and the properties of the Jacobi elliptic functions [43, 44], one can easily show that
We are left with evaluating the third integral in Eq. (8.1), i.e., \(\displaystyle I=\int _0^{2K} (\text {sn}\, \tau \, \text {cn}\, \tau )^2 \cos \dfrac{\pi \tau }{K} \mathrm{d}\tau \) which is not straightforward. We will use the method of contour integral for the same. Toward this end, we introduce a complex variable z in place of the real variable \(\tau \). With the complex variable z, our integrand has some poles in the complex plane which can be exploited to evaluate the desired integral on the real axis. The contour we use for integration is shown in Fig. 22.
Our integrand \((\text {sn}\, z \, \text {cn}\, z)^2 \cos \dfrac{\pi z}{K}\) has two simple poles at \(z=iK^{\prime }\) (which lies on \(C_1\)) and \(z=2K+i2K^{\prime }\) (which lies on \(C_3\)), where \(i=\sqrt{-1}\). These two simple poles will contribute half of their respective residues to the evaluation of the integral over the contour. The residuesFootnote 4 at the two poles are the same and are given by
Using the residue theorem [35], we have
where P.V. stands for “Principal Value.” Using the periodicity of Jacobi elliptic functions, one can easily show that
By definition, we have \(\displaystyle \int _{C_2}(\text {sn}\, z \, \text {cn}\, z)^2 \cos \dfrac{\pi z}{K} \mathrm{d}z = I\). To evaluate the integral along \(C_4\), we make a transformation \(z=u+2iK^{\prime }\) with u varying from 2K to 0. With this transformation and again using the periodicity of the Jacobi elliptic functions, we can evaluate

Substituting the above integrals into Eq. (8.5) gives us
Equation (4.8) is obtained by substituting Eqs. (8.2)–(8.4) and (8.6) in Eq. (8.1).
Similarly for obtaining Eq. (4.9), we multiply both sides of Eq. (4.7) with \( \text {dn}\, \tau \) and integrate w.r.t. \(\tau \) from 0 to 2K. This results in
The first integral of Eq. (8.7) has already been shown to be zero in Eq. (8.3). The second integral of Eq. (8.7) can be found in the handbook [43].
Using the same integral identity as before and the properties of the Jacobi elliptic functions [43, 44], one can easily show that
i.e., the third integral vanishes. For the evaluation of the fourth integral, we again use contour integration with the same contour as shown in Fig. 22 to get
1.2 Horizontal excitation
The relevant equation for getting Eq. (5.5) is
The first and second integrals of Eq. (8.11) have already been evaluated in Eqs. (8.2) and (8.3), respectively. The third and fourth integrals can be evaluated using the method of contour integral by taking the same contour as shown in Fig. 22. The respective results are
Similarly, to get Eq. (5.6), we need to consider the equation
Again, the first and second integrals of Eq. (8.14) are given in Eqs. (8.3) and (8.8), respectively. The third and fourth integrals evaluated using contour integral are
Appendix 2: Evaluation of integrals arising in elliptic averaging
We have outlined here the procedure for evaluating the integrals for carrying out the elliptic averaging. The subscript “av” has been omitted to simplify the presentation.
1.1 Vertical excitation
Integrating the r.h.s. of Eq. (4.12) w.r.t. t from 0 to \(T=\dfrac{2\pi }{\omega }\) and dividing it by T leads to
where \(\Delta =\dfrac{K \phi }{\pi }\). Since the integrands are periodic with period \(=2K\), changing the limits of integration as done in Eq. (9.1) does not change the value of the integrals.
The first integral of Eq. (9.1) has already been evaluated in Eq. (8.8). The second integral can be evaluated using contour integral with the same contour as shown in Fig. 22. The result is
Similarly, integrating the r.h.s. of Eq. (4.13) w.r.t. t from 0 to T and dividing by T yields
where \(\Delta =\dfrac{K \phi }{\pi }\). The first integral is given in Eq. (8.3). For the second integral, we use the integral identity \(\displaystyle \int _0^{2a_1}h(x) \mathrm{d}x=\int _0^{a_1} h(x) \mathrm{d}x + \int _0^{a_1} h(2a_1-x) \mathrm{d}x\) and the properties of the Jacobi elliptic and zeta functions [43, 44] to show that
The third and fourth integrals can be evaluated using the method of contour integration as
1.2 Horizontal excitation
The relevant equations for the case of horizontal excitation are
The values of the first, second and third integrals of Eq. (9.7) are available in Eqs. (8.8), (8.16) and (8.15), respectively. There were more integrals involved in Eq. (9.8) which vanish as per Eqs. (8.3) and (9.4). The first and second integrals of Eq. (9.8) are evaluated in Eqs. (8.12) and (8.13), respectively. Finally, the third and the fourth integrals in Eq. (9.8) can be evaluated using the method of contour integration as
Rights and permissions
About this article
Cite this article
Das, S., Wahi, P. Approximations for period-1 rotation of vertically and horizontally excited parametric pendulum. Nonlinear Dyn 88, 2171–2201 (2017). https://doi.org/10.1007/s11071-017-3370-z
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11071-017-3370-z