Abstract
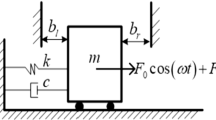
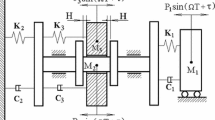
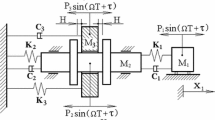
Based on the method of Poincaré mapping under cell reference, we describe basins of attraction for coexisting multi-dimensional tori attractors in a three-degree-of-freedom vibro-impact system. Because the multi-dimensional tori attractors are very rare in the low-dimensional systems, we find that these coexisting tori attractors have positive measure basins in the sense of Milnor. The coexisting multi-dimensional tori attractors can be distinguished by the exactly Poincaré mapping and Lyapunov dimension. The basin of attraction can be estimated by the limit cycle in the Poincaré section from the viewpoint of engineering.





Similar content being viewed by others
References
Thompson, J.M.T., Stewart, H.B.: Nonlinear Dynamics and Chaos, 2nd edn. Wiley, New York (2002)
Grebogi, C., Ott, E., Yorke, J.A.: Are three-frequency quasiperiodic orbits to be expected in typical nonlinear dynamical systems? Phys. Rev. Lett. 51, 339–342 (1983)
Lorenz, E.N.: Deterministic nonperiodic flow. J. Atmos. Sci. 20, 130–141 (1963)
Grebogi, C., Ott, E., Pelikan, S., Yorke, J.A.: Strange attractors that are not chaotic. Phys. D 13, 261–268 (1984)
Timme, M., Wolf, F., Geisel, T.: Prevalence of unstable attractors in networks of pulse-coupled oscillators. Phys. Rev. Lett. 89, 154105 (2002)
Milnor, J.: On the concept of attractor. Commun. Math. Phys. 99, 177–195 (1985)
Wen, G.L., Xie, J.H., Xu, D.: Onset of degenerate Hopf bifurcation of a vibro-impact oscillator. J. Appl. Mech. 71, 579–581 (2004)
Wen, G., Xu, H., Xie, J.: Controlling Hopf-Hopf interaction bifurcations of a two-degree-of-freedom self-excited system with dry friction. Nonlinear Dyn. 64, 49–57 (2011)
Luo, G.W., Chu, Y.D., Zhang, Y.L., Zhang, J.G.: Double Neimark-Sacker bifurcation and torus bifurcation of a class of vibratory systems with symmetrical rigid stops. J. Sound Vib. 298, 154–179 (2006)
Xie, J.H., Ding, W.C.: Hopf-Hopf bifurcation and invariant torus \(\text{ T }^{2}\) of a vibro-impact system. Int. J. Non-Linear Mech. 40, 531–543 (2005)
Yue, Y., Xie, J.H.: Lyapunov exponents and coexistence of attractors in vibro-impact systems with symmetric two-sided rigid constraints. Phys. Lett. A 373, 2041–2046 (2009)
Feudel, U.: Complex dynamics in multistable systems. Int. J. Bifurc. Chaos 18, 1607–1626 (2008)
Hsu, C.S.: Cell-to-Cell Mapping: A Method of Global Analysis for Nonlinear Systems. Springer, New York (1987)
Tongue, B.H.: On obtaining global nonlinear system characteristics through interpolated cell mapping. Phys. D 28, 401–408 (1987)
Tongue, B.H., Gu, K.: Interpolated cell mapping of dynamical systems. J. Appl. Mech. 55, 461–466 (1988)
Virgin, L.N., Begley, C.J.: Grazing bifurcations and basins of attraction in an impact-friction. Phys. D 130, 43–57 (1999)
Ge, Z.M., Lee, S.C.: A modified interpolated cell mapping method. J. Sound. Vib. 199, 189–206 (1997)
Hong, L., Xu, J.: Chaotic saddles in Wada basin boundaries and their bifurcations by the generalized cell-mapping digraph (GCMD) method. Nonlinear Dyn. 32, 371–385 (2003)
Hong, L., Sun, J.Q.: Codimension two bifurcations of nonlinear systems driven by fuzzy nose. Phys. D 213, 181–189 (2006)
Eason, R.P., Dick, A.J.: A parallelized multi-degrees-of-freedom cell mapping method. Nonlinear Dyn. 77, 467–479 (2014)
van der Spek, J.A.W., de Hoon, C.A.L., de Kraker, A., van Campen, D.H.: Application of cell mapping methods to a discontinuous dynamic system. Nonlinear Dyn. 6, 87–99 (1994)
Zhang, Y.: Strange nonchaotic attractors with Wada basins. Phys. D 259, 26–36 (2013)
Zhang, Y., Luo, W.: Torus-doubling bifurcations and strange nonchaotic attractors in a vibro-impact system. J. Sound Vib. 332, 5462–5475 (2013)
Yue, Y., Xie, J., Cao, X.: Determining Lyapunov spectrum and Lyapunov dimension based on the Poincaré map in a vibro-impact system. Nonlinear Dyn. 69, 743–753 (2012)
De Souza, S.L.T., Caldas, I.L.: Calculation of Lyapunov exponts in systems with impact. Chaos Solitons Fractals 19, 569–579 (2004)
Jiang, J., Xu, J.X.: A method of point mapping under cell reference for global analysis of non-linear dynamical systems. Phys. Lett. A 188, 137–145 (1994)
Jiang, J., Xu, J.X.: An iterative method of point mapping under cell reference for the global analysis: theory and a multiscale reference technique. Nonlinear Dyn. 15, 103–114 (1998)
Jiang, J., Xu, J.X.: A iterative method of point mapping under cell reference for global analysis of non-linear dynamical systems. J Sound. Vib. 194, 605–621 (1996)
Grassberger, P., Procaccia, I.: Measuring the strangeness of strange attractors. Phys. D 9, 189–208 (1983)
Hsu, C.S.: Global analysis by cell mapping. Int. J. Bifurc. Chaos 2, 727–771 (1992)
Acknowledgments
The authors are deeply indebted to all anonymous reviewers for their careful reading of the manuscript, as well as for their fruitful comments and advice which led to an improvement of this Letter. We would like to thank you very much for helping us to correct some minor points in the manuscript. This work was supported by the National Natural Science Foundation of China (Nos. 61034005 and 61433004) and the National High Technology Research and Development Program of China (2012AA040104) and IAPI Fundamental Research Funds 2013ZCX14. This work was supported also by the development project of key laboratory of Liaoning province and supported by the NSFC (Nos. 11172119 and 11362008).
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Zhang, H., Zhang, Y. & Luo, G. Basins of coexisting multi-dimensional tori in a vibro-impact system. Nonlinear Dyn 79, 2177–2185 (2015). https://doi.org/10.1007/s11071-014-1803-5
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11071-014-1803-5