Principal resonance bifurcation of bending–torsion coupling of aero-engine compressor blade with assembled clearance under lateral displacement excitation of rotor shaft

Abstract

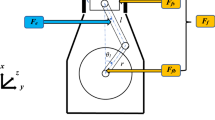
The principal resonance of bending–torsion coupling of a compressor blade with an assembled clearance and a cubic structural nonlinearity, subjected to the lateral displacement excitation of rotor shaft and aerodynamic loads, is analyzed to explore the topology transformation of amplitude–frequency response along with the changes of the physical parameters. The bifurcation equation of the first-order principal resonance response is derived from using the averaging method. The transition set and the bifurcation figures of the response solution are obtained by the singularity theory. The effects of the main physical parameters of the system on the topology transformation of amplitude–frequency response are discussed.

Appendix

The coefficient expressions of Eqs. (5) and (6) are

$$\begin{aligned}&a_{11} =\frac{\bar{{\omega }}^{2}r_\alpha ^2 }{r_\alpha ^2 -x_a^2 }, \quad a_{12} =\frac{\left[ {2V^{*{2}}}r_\alpha ^2 +x_a V^{^{{*2}}(1+2a_h )-\mu x_a r_\alpha ^2 } \right] }{\mu \left( {r_\alpha ^2 -x_a ^{2}} \right) }, \\&a_{13} =\frac{2\zeta _\lambda r_\alpha ^2 \bar{{\omega }}}{r_\alpha ^2 -x_a^2 }, \quad a_{{14}} -\frac{2\zeta _\alpha x_a r_\alpha ^2 }{r_\alpha ^2 -x_a^2 }, \quad a_{15} = -\frac{x_a r_\alpha ^2 \beta }{r_\alpha ^2 -x_a^2 }, \\&a_{16} =\frac{r_\alpha ^2 D_1 \omega ^{2}\sin \gamma }{r_\alpha ^2 -x_a^2 }, a_{21} = -\frac{x_a }{r_\alpha ^2 }a_{11} ,\\&a_{22} =\frac{\left( {2V^{*{2}}x_a +V^{*{2}}(1+2a_h ) -\mu r_\alpha ^2 } \right) }{\mu \left( {x_a^2 -r_\alpha ^2 } \right) },\\&a_{23} =-\frac{x_a }{r_\alpha ^2 }a_{13} , \quad a_{24} =-\frac{1}{x_a }a_{{14}} ,\\&a_{25} =-\frac{1}{x_a }a_{15} , \quad a_{26} =-\frac{x_a }{r_\alpha ^2 }a_{16} . \end{aligned}$$

The matrix ${{\varvec{A}}}$ and vector $F(X,\tau )$ of Eq. (7) are

$$\begin{aligned}&\mathbf{A}=\left[ {{\begin{array}{cccc} 0&{} 1&{} 0&{} 0 \\ {-a_{11} }&{} 0&{} {-a_{12} }&{} 0 \\ 0&{} 0&{} 0&{} 1 \\ {-a_{21} }&{} 0&{} {-a_{22} }&{} 0 \\ \end{array} }} \right] , \quad F(X,\tau )=\left\{ {{\begin{array}{l} 0 \\ {F_1 } \\ 0 \\ {F_2 } \\ \end{array} }} \right\} , \end{aligned}$$

$$\begin{aligned} \left\{ {\begin{array}{l} \displaystyle F_1 \!=\!-a_{13} x_2 -a_{{14}} x_4 -\;a_{11} S(x_1 )-a_{15} x_3^3 +a_{16} \cos \omega \tau \\ \displaystyle F_2 \!=\!-a_{23} x_2 -\;a_{24} x_4 -a_{21} S(x_1 )-a_{25} x_3^3 +a_{26} \cos \omega \tau \\ \end{array}} \right. . \end{aligned}$$

The variables of Eq. (9) are

$$\begin{aligned} \overline{F_1 } =0, \quad \overline{F_2 } =F_1 , \quad \overline{F_3 } =0, \quad \overline{F_4 } =F_2 . \end{aligned}$$

The modal frequencies of derivation equation $\dot{X}=AX$ of Eq. (7) are

$$\begin{aligned}&\lambda _k =\sqrt{\frac{1}{2}\left[ {\left( {a_{22} +a_{11} } \right) \mp \sqrt{\left( {a_{22} +a_{11} } \right) ^{2}-4\left( {a_{22} a_{11} -a_{21} a_{12} } \right) }} \right] }\\&\quad (k=1,2), \end{aligned}$$

where the conditions of two different positive $\lambda _k$ are

$$\begin{aligned} \left\{ {\begin{array}{l} a_{22} +a_{11} >0 \\ \left( {a_{22} +a_{11} } \right) ^{2}-4\left( {a_{22} a_{11} -a_{21} a_{12} } \right) >0 \\ \end{array}} \right. . \end{aligned}$$

The fundamental solution sets $\phi _{sk} (\theta _k )$ and $\varphi _{sk}^*(\theta _k )$ of the derivation equation $\dot{X}=AX$ of Eq. (7) are

$$\begin{aligned} \left. {\begin{array}{ll} \varphi _{1k} =\cos \lambda _k \tau ,\\ \varphi _{1k}^*=\sin \lambda _k \tau \\ \varphi _{2k} =-\lambda _k \sin \lambda _k \tau ,\\ \varphi _{2k}^*=\lambda _k \cos \lambda _k \tau \\ \varphi _{3k} =\frac{1}{a_{12} }\left( {\lambda _k^2 -a_{11} } \right) \cos \lambda _k \tau ,\\ \varphi _{3k}^*=\frac{1}{a_{12} }\left( {\lambda _k^2 -a_{11} } \right) \sin \lambda _k \tau \\ \varphi _{4k} =-\frac{\lambda _k }{a_{12} }\left( {\lambda _k^2 -a_{11} } \right) \sin \lambda _k \tau ,\\ \varphi _{4k}^*=\frac{\lambda _k }{a_{12} }\left( {\lambda _k^2 -a_{11} } \right) \cos \lambda _k \tau \\ \end{array}} \right\} \quad (k=1,2). \end{aligned}$$

The fundamental solution sets $\phi _{sj} (\theta _j )$ and $\phi _{sj}^*(\theta _j )$ of the adjoint equation of the derivation equation $\dot{X}={{\varvec{A}}}\cdot X$of Eq. (7) are

$$\begin{aligned} \left. {\begin{array}{l} \phi _{1k} =\cos \lambda _k \tau ,\\ \phi _{1k}^*=\sin \lambda _k \tau \\ \phi _{2k} =-\frac{1}{\lambda _k }\sin \lambda _k \tau ,\\ \phi _{2k}^*=\frac{1}{\lambda _k }\cos \lambda _k \tau \\ \phi _{3k} =\frac{1}{a_{21} }\left( {\lambda _k ^{2}-a_{11} } \right) \cos \lambda _k \tau ,\\ \phi _{3k}^*=\frac{1}{a_{21} }\left( {\lambda _k ^{2}-a_{11} } \right) \sin \lambda _k \tau \\ \phi _{4k} =-\frac{1}{a_{21} \lambda _k }\left( {\lambda _k ^{2}-a_{11} } \right) \sin \lambda _k \tau ,\\ \phi _{4k}^*=\frac{1}{a_{21} \lambda _k }\left( {\lambda _k ^{2}-a_{11} } \right) \cos \lambda _k \tau \\ \end{array}} \right\} \quad (k=1,2). \end{aligned}$$

The expression of $\Delta _k^{\prime }$ in formula (10) is

$$\begin{aligned} \Delta _k^{\prime } =1+\frac{1}{a_{12} a_{21} }\left( {\lambda _k ^{2}-a_{11} } \right) ^{2}. \end{aligned}$$