Thermo-elastic solutions for multilayered wide plates and beams with interfacial imperfections through the transfer matrix method

Abstract

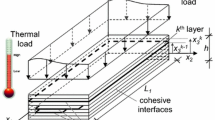
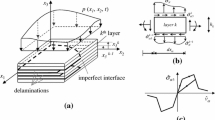
A matrix technique is formulated to efficiently solve stationary two-dimensional thermo-elasticity problems in simply supported multilayered beams and plates with an arbitrary number of layers which may be in imperfect mechanical and thermal contact. The method uses local transfer matrices and continuity conditions at the layer interfaces to establish explicit relationships between the unknown integration constants in the solution of a generic layer and those of the first layer. Explicit expressions are then derived for temperature, displacements and stresses through the imposition of the boundary conditions at the top and bottom surfaces of the plate. The dimensionless expressions allow to easily generate exact solutions, also for plates with many layers and interfacial thermal and mechanical imperfections. The solutions can be used for parametric analyses, to investigate the influence of the inhomogeneous material structure and interfacial imperfections on local fields or to verify the accuracy of approximate theories and numerical models.

Appendices

Appendix 1: Dimensionless forms

Dimensional quantities and groups which appear throughout the model are listed below along with their dimensionless counterparts. If the quantities which appear in the equations in the paper are interpreted as their dimensionless counterparts, the equations are dimensionless (see Table 2).

Table 2 Dimensionless quantities (with ${}^{(1)}\alpha_{2}$, $h$ and ${}^{(1)}C_{22}$ as fundamental units)

Appendix 2: Derivation of the unknown constants of the heat conduction problem

Equation (21) is expanded as:

$$\begin{aligned} {}^{(k)}c_{1} = {}^{(k)}F_{11} {}^{(1)}c_{1} + {}^{(k)}F_{12} {}^{(1)}c_{2} \hfill \\ {}^{(k)}c_{2} = {}^{(k)}F_{21} {}^{(1)}c_{1} + {}^{(k)}F_{22} {}^{(1)}c_{2} \hfill \\ \end{aligned} ,$$

(41)

where:

$${}^{(k)}F_{ij} = \sum\limits_{t = 1}^{2} {\left\{ {\sum\limits_{r = 1}^{2} {{}^{(k)}Z_{ir} {}^{(k)}N_{rt} } } \right\}} {}^{(1)}D_{tj} \left( {x_{3}^{0} } \right) .$$

(42)

The terms ${}^{(k)}Z_{ir}$, for i, r = 1, 2 are:

$$\begin{aligned} {}^{(k)}Z_{11} = \frac{{e^{{ - {}^{(k)}sx_{3}^{k} }} }}{2} , { }{}^{(k)}Z_{21} = \frac{{e^{{{}^{(k)}sx_{3}^{k} }} }}{2} \, \hfill \\ {}^{(k)}Z_{12} = \frac{{{}^{(k + 1)}K_{3} e^{{ - {}^{(k)}sx_{3}^{k} }} }}{2}\left[ {\frac{1}{{{}^{(k)}K_{3} {}^{(k)}s}} - R^{k} } \right] \hfill \\ {}^{(k)}Z_{22} = - \frac{{{}^{(k + 1)}K_{3} e^{{{}^{(k)}sx_{3}^{k} }} }}{2}\left[ {\frac{1}{{{}^{(k)}K_{3} {}^{(k)}s}} + R^{k} } \right] \hfill \\ \end{aligned} ,$$

(43)

the ${}^{(1)}D_{tj} \left( {x_{3}^{0} } \right)$ are given in Eq. (16) and the ${}^{(k)}N_{rt}$, for r, t = 1, 2, are defined by the recursive formula:

$$\begin{aligned} & {}^{(k)}N_{rt} = \sum\limits_{l = 1}^{2} {{}^{(k)}A_{rl} {}^{(k - 1)}N_{lt} } {\text{ for }}k{ = 2, } \ldots , { }n \, \\ & {}^{(1)}N_{rt} { = }{}^{(1)}A_{rt} \\ \end{aligned} ,$$

(44)

where:

$$\begin{aligned} {}^{(k)}A_{11} = \frac{{\left( {1 + {}^{(k)}K_{3} {}^{(k)}sR^{k} } \right)\left( {1 + e^{{2{}^{(k)}s{}^{(k)}h}} } \right) - 2{}^{(k)}K_{3} {}^{(k)}sR^{k} }}{{2e^{{{}^{(k)}s{}^{(k)}h}} }} \hfill \\ {}^{(k)}A_{12} = \frac{{\left( {1 + {}^{(k)}K_{3} {}^{(k)}sR^{k} } \right)\left( {1 + e^{{2{}^{(k)}s{}^{(k)}h}} } \right) - 2}}{{2{}^{(k)}se^{{{}^{(k)}s{}^{(k)}h}} }} \hfill \\ {}^{(k)}A_{21} = \frac{{{}^{(k)}K_{3} }}{{{}^{(k + 1)}K_{3} }}\frac{{{}^{(k)}s\left( {e^{{2{}^{(k)}s{}^{(k)}h}} - 1} \right)}}{{2e^{{{}^{(k)}s{}^{(k)}h}} }} \hfill \\ {}^{(k)}A_{22} = \frac{{{}^{(k)}K_{3} }}{{{}^{(k + 1)}K_{3} }}\frac{{e^{{2{}^{(k)}s{}^{(k)}h}} + 1}}{{2e^{{{}^{(k)}s{}^{(k)}h}} }} \hfill \\ \end{aligned}$$

(45)

For perfect thermal contact, the $R^{k}$ terms in Eq. (45) vanish. The terms given in Eqs. (43)–(45) are the coefficients of the matrices ${}^{(k)}Z = {}^{(k)}D^{ - 1} (x_{3}^{k} )\left( {J^{k} } \right)^{ - 1}$, ${}^{(k)}N = \prod\nolimits_{i = k}^{1} {{}^{(i)}A}$ and ${}^{(i)}A = \left( {J^{i} } \right){}^{(i)}D(x_{3}^{i} ){}^{(i)}D^{ - 1} (x_{3}^{i - 1} )$. Application of the boundary conditions at the top and bottom surfaces of the plate and using Eq. (41) for $k = n$, result in the following explicit expressions for the unknown constants of the first layer:

$$\begin{aligned} &{}^{(1)}c_{1} = \frac{{T_{u} - T_{l} {}^{(n)}\left( {F_{12} e^{{sx_{3}^{n} }} + F_{22} e^{{ - sx_{3}^{n} }} } \right)e^{{{}^{(1)}sx_{3}^{0} }}}}{{{}^{(n)}\left( {F_{11} e^{{sx_{3}^{n} }} + F_{21} e^{{ - sx_{3}^{n} }} } \right) -} {}^{(n)}\left( {F_{12} e^{{sx_{3}^{n} }} + F_{22} e^{{ - sx_{3}^{n} }} } \right)e^{{2{}^{(1)}sx_{3}^{0} }}} \hfill \\ &{}^{(1)}c_{2} = \frac{{T_{l} - {}^{(1)}c_{1} e^{{{}^{(1)}sx_{3}^{0} }} }}{{e^{{ - {}^{(1)}sx_{3}^{0} }} }} \hfill \\ \end{aligned}$$

(46)

Appendix 3: Unknown constants of the particular solution of layer k

$$\begin{aligned} {}^{(k)}B_{1} = - {}^{(k)}\left\{ {\frac{{pc_{1} \left[ {(C_{33} s^{2} - C_{55} p^{2} )(C_{12} \alpha_{1} + C_{22} \alpha_{2} ) - s^{2} (C_{23} + C_{55} )(C_{13} \alpha_{1} + C_{23} \alpha_{2} ) - C_{55} \alpha_{3} (C_{23} p^{2} + C_{33} s^{2} )} \right]}}{{C_{22} p^{2} (C_{33} s^{2} - C_{55} p^{2} ) - C_{23} p^{2} s^{2} (C_{23} + 2C_{55} ) - C_{33} C_{55} s^{4} }}} \right\} \hfill \\ {}^{(k)}B_{2} = {}^{(k)}\left( {\frac{{c_{2} }}{{c_{1} }}} \right){}^{(k)}B_{1} \hfill \\ {}^{(k)}D_{1} = - {}^{(k)}\left\{ {\frac{{sc_{1} \left[ {(C_{55} s^{2} - C_{22} p^{2} )(C_{13} \alpha_{1} + C_{33} \alpha_{3} ) + p^{2} (C_{23} + C_{55} )(C_{12} \alpha_{1} + C_{23} \alpha_{3} ) + C_{55} \alpha_{2} (C_{22} p^{2} + C_{23} s^{2} )} \right]}}{{C_{22} p^{2} (C_{33} s^{2} - C_{55} p^{2} ) - C_{23} p^{2} s^{2} (C_{23} + 2C_{55} ) - C_{33} C_{55} s^{4} }}} \right\} \hfill \\ {}^{(k)}D_{2} = - {}^{(k)}\left( {\frac{{c_{2} }}{{c_{1} }}} \right){}^{(k)}D_{1} \hfill \\ \end{aligned}$$

(47)

Appendix 4: Matrix ${}^{({\varvec{k}})}{\varvec{E}}$

Positive discriminant:

$$\begin{aligned} &{}^{(k)}E_{11} (x_{3} ) = {}^{(k)}X_{1} (x_{3} ) , { }{}^{(k)}E_{12} (x_{3} ) = {}^{(k)}Y_{1} (x_{3} ){ }\\ &{}^{(k)}E_{13} (x_{3} ) = {}^{(k)}X_{2} (x_{3} ),{}^{(k)}E_{14} (x_{3} ) = {}^{(k)}Y_{2} (x_{3} ) \hfill \\ &{}^{(k)}E_{21} (x_{3} ) = {}^{(k)}\beta_{1} {}^{(k)}Y_{1} (x_{3} )\\ &{}^{(k)}E_{22} (x_{3} ) = {}^{(k)}\lambda {}^{(k)}\beta_{1} {}^{(k)}X_{1} (x_{3} )\\ &{}^{(k)}E_{23} (x_{3} ) = {}^{(k)}\beta_{2} {}^{(k)}Y_{2} (x_{3} ) \hfill \\ &{}^{(k)}E_{24} (x_{3} ) = {}^{(k)}\lambda {}^{(k)}\beta_{2} {}^{(k)}X_{2} (x_{3} )\\ &{}^{(k)}E_{31} (x_{3} ) = {}^{(k)}\left( {C_{33} \beta_{1} m_{1} - C_{23} p} \right){}^{(k)}X_{1} (x_{3} ) \hfill \\ &{}^{(k)}E_{32} (x_{3} ) = {}^{(k)}\left( {C_{33} \beta_{1} m_{1} - C_{23} p} \right){}^{(k)}Y_{1} (x_{3} )\\ &{}^{(k)}E_{33} (x_{3} ) = {}^{(k)}\left( {C_{33} \beta_{2} m_{2} - C_{23} p} \right){}^{(k)}X_{2} (x_{3} ) \hfill \\ &{}^{(k)}E_{34} (x_{3} ) = {}^{(k)}\left( {C_{33} \beta_{2} m_{2} - C_{23} p} \right){}^{(k)}Y_{2} (x_{3} )\\ &{}^{(k)}E_{41} (x_{3} ) = {}^{(k)}C_{55} {}^{(k)}\left( {\beta_{1} p + \lambda m_{1} } \right){}^{(k)}Y_{1} (x_{3} ) \hfill \\ &{}^{(k)}E_{42} (x_{3} ) = {}^{(k)}C_{55} {}^{(k)}\left( {\lambda \beta_{1} p + m_{1} } \right){}^{(k)}X_{1} (x_{3} )\\ &{}^{(k)}E_{43} (x_{3} ) = {}^{(k)}C_{55} {}^{(k)}\left( {\beta_{2} p + \lambda m_{2} } \right){}^{(k)}Y_{2} (x_{3} ) \hfill \\ &{}^{(k)}E_{44} (x_{3} ) = {}^{(k)}C_{55} {}^{(k)}\left( {\lambda \beta_{2} p + m_{2} } \right){}^{(k)}X_{2} (x_{3} ) \hfill \\ \end{aligned}$$

(48)

Zero discriminant:

This case occurs when the layer is isotropic.

$$\begin{aligned} {}^{(k)}E_{11} (x_{3} ) = e^{{px_{3} }} , { }{}^{(k)}E_{12} (x_{3} ) = x_{3} e^{{px_{3} }} , { }{}^{(k)}E_{13} (x_{3} ) = e^{{ - px_{3} }} ,{}^{(k)}E_{14} (x_{3} ) = x_{3} e^{{ - px_{3} }} , { }{}^{(k)}E_{21} (x_{3} ) = e^{{px_{3} }} \, \hfill \\ {}^{(k)}E_{22} (x_{3} ) = \left(\frac{{4{}^{(k)}\nu - 3}}{p} + x_{3} \right)e^{{px_{3} }} ,{}^{(k)}E_{23} (x_{3} ) = - e^{{ - px_{3} }} ,{}^{(k)}E_{24} (x_{3} ) = \left(\frac{{4{}^{(k)}\nu - 3}}{p} - x_{3}\right)e^{{ - px_{3} }} \hfill \\ {}^{(k)}E_{31} (x_{3}) = \frac{{p{}^{(k)}E}}{{1 + {}^{(k)}\nu }}e^{{px_{3} }} \, ,{}^{(k)}E_{32} (x_{3} ) = \frac{{px_{3} + 2({}^{(k)}\nu - 1)}}{{1 + {}^{(k)}\nu }}{}^{(k)}Ee^{{px_{3} }} ,{}^{(k)}E_{33} (x_{3} ) = \frac{{p{}^{(k)}E}}{{1 + {}^{(k)}\nu }}e^{{ - px_{3} }} \hfill \\ {}^{(k)}E_{34} (x_{3} ) = \frac{{px_{3} + 2(1 - {}^{(k)}\nu )}}{{1 + {}^{(k)}\nu }}{}^{(k)}Ee^{{ - px_{3} }} ,{}^{(k)}E_{41} (x_{3} ) = \frac{{p{}^{(k)}E}}{{1 + {}^{(k)}\nu }}e^{{px_{3} }} ,{}^{(k)}E_{42} (x_{3} ) = \frac{{px_{3} + 2{}^{(k)}\nu - 1}}{{1 + {}^{(k)}\nu }}{}^{(k)}Ee^{{px_{3} }} \hfill \\ {}^{(k)}E_{43} (x_{3} ) = - \frac{{p{}^{(k)}E}}{{1 + {}^{(k)}\nu }}e^{{ - px_{3} }} ,{}^{(k)}E_{44} (x_{3} ) = - \frac{{px_{3} - 2{}^{(k)}\nu + 1}}{{1 + {}^{(k)}\nu }}{}^{(k)}Ee^{{ - px_{3} }} \hfill \\ \end{aligned}$$

(49)

where ${}^{(k)}\nu$ and ${}^{(k)}E$ are Poisson ratio and Young’s modulus of the layer k and $p = {{m\pi } \mathord{\left/ {\vphantom {{m\pi } L}} \right. \kern-0pt} L}$ with $m \in {\mathbb{N}}$.

Negative discriminant:

This case occurs when the transverse stiffness of the layer is much higher than the in-plane stiffnesses.

$$\begin{aligned} {}^{{(k)}}E_{{11}} (x_{3} ) & = e^{{{}^{{(k)}}\rho _{1} x_{3} }} \cos ({}^{{(k)}}\rho _{2} x_{3} )\,{}^{{(k)}}E_{{12}} (x_{3} ) = e^{{{}^{{(k)}}\rho _{1} x_{3} }} \sin ({}^{{(k)}}\rho _{2} x_{3} ){\text{ }}\,{}^{{(k)}}E_{{13}} (x_{3} ) = e^{{ - {}^{{(k)}}\rho _{1} x_{3} }} \cos ({}^{{(k)}}\rho _{2} x_{3} ) \\ {}^{{(k)}}E_{{14}} (x_{3} ) & = e^{{ - {}^{{(k)}}\rho _{1} x_{3} }} \sin ({}^{{(k)}}\rho _{2} x_{3} ){\text{ }}\,{}^{{(k)}}E_{{21}} (x_{3} ) = e^{{{}^{{(k)}}\rho _{1} x_{3} }} {}^{{(k)}}\left[ {r_{1} \cos (\rho _{2} x_{3} ) + r_{2} \sin (\rho _{2} x_{3} )} \right] \\ {}^{{(k)}}E_{{22}} (x_{3} ) & = e^{{{}^{{(k)}}\rho _{1} x_{3} }} {}^{{(k)}}\left[ {r_{1} \sin (\rho _{2} x_{3} ) - r_{2} \cos (\rho _{2} x_{3} )} \right]\,{}^{{(k)}}E_{{23}} (x_{3} ) = e^{{ - {}^{{(k)}}\rho _{1} x_{3} }} {}^{{(k)}}\left[ {r_{2} \sin (\rho _{2} x_{3} ) - r_{1} \cos (\rho _{2} x_{3} )} \right] \\ {}^{{(k)}}E_{{24}} (x_{3} ) & = - e^{{ - {}^{{(k)}}\rho _{1} x_{3} }} {}^{{(k)}}\left[ {r_{2} \cos (\rho _{2} x_{3} ) + r_{1} \sin (\rho _{2} x_{3} )} \right] \\ {}^{{(k)}}E_{{31}} (x_{3} ) & = e^{{{}^{{(k)}}\rho _{1} x_{3} }} {}^{{(k)}}\left[ { - pC_{{23}} \cos (\rho _{2} x_{3} )} \right. + C_{{33}} \left( {\rho _{1} r_{1} \cos (\rho _{2} x_{3} )} \right. - \rho _{2} r_{1} \sin (\rho _{2} x_{3} ) + \left. {\left. {\rho _{1} r_{2} \sin (\rho _{2} x_{3} ) + \rho _{2} r_{2} \cos (\rho _{2} x_{3} )} \right)} \right] \\ {}^{{(k)}}E_{{32}} (x_{3} ) & = e^{{{}^{{(k)}}\rho _{1} x_{3} }} {}^{{(k)}}\left[ { - pC_{{23}} \sin (\rho _{2} x_{3} ) + C_{{33}} } \right.\left( { - \rho _{1} r_{2} \cos (\rho _{2} x_{3} )} \right.\left. {\left. { + \rho _{2} r_{2} \sin (\rho _{2} x_{3} ) + \rho _{1} r_{1} \sin (\rho _{2} x_{3} ) + \rho _{2} r_{1} \cos (\rho _{2} x_{3} )} \right)} \right] \\ {}^{{(k)}}E_{{33}} (x_{3} ) & = e^{{ - {}^{{(k)}}\rho _{1} x_{3} }} {}^{{(k)}}\left[ { - pC_{{23}} \cos (\rho _{2} x_{3} ) + C_{{33}} \left( {\rho _{1} r_{1} \cos (\rho _{2} x_{3} )} \right.} \right.\left. {\left. { + \rho _{2} r_{1} \sin (\rho _{2} x_{3} ) - \rho _{1} r_{2} \sin (\rho _{2} x_{3} ) + \rho _{2} r_{2} \cos (\rho _{2} x_{3} )} \right)} \right] \\ {}^{{(k)}}E_{{34}} (x_{3} ) & = e^{{ - {}^{{(k)}}\rho _{1} x_{3} }} {}^{{(k)}}\left[ { - pC_{{23}} \sin (\rho _{2} x_{3} ) + C_{{33}} \left( {\rho _{1} r_{2} \cos (\rho _{2} x_{3} )} \right.} \right.\left. {\left. { + \rho _{2} r_{2} \sin (\rho _{2} x_{3} ) + \rho _{1} r_{1} \sin (\rho _{2} x_{3} ) - \rho _{2} r_{1} \cos (\rho _{2} x_{3} )} \right)} \right] \\ {}^{{(k)}}E_{{41}} (x_{3} ) & = e^{{{}^{{(k)}}\rho _{1} x_{3} }} {}^{{(k)}}C_{{55}} {}^{{(k)}}\left[ {\rho _{1} \cos (\rho _{2} x_{3} ) - \rho _{2} \sin (\rho _{2} x_{3} )} \right.\left. { + p\left( {r_{1} \cos (\rho _{2} x_{3} ) + r_{2} \sin (\rho _{2} x_{3} )} \right)} \right] \\ {}^{{(k)}}E_{{42}} (x_{3} ) & = e^{{{}^{{(k)}}\rho _{1} x_{3} }} {}^{{(k)}}C_{{55}} {}^{{(k)}}\left[ {\rho _{1} \sin (\rho _{2} x_{3} ) + \rho _{2} \cos (\rho _{2} x_{3} )} \right.\left. { + p\left( {r_{1} \sin (\rho _{2} x_{3} ) - r_{2} \cos (\rho _{2} x_{3} )} \right)} \right] \\ {}^{{(k)}}E_{{43}} (x_{3} ) & = e^{{ - {}^{{(k)}}\rho _{1} x_{3} }} {}^{{(k)}}C_{{55}} {}^{{(k)}}\left[ { - \rho _{1} \cos (\rho _{2} x_{3} ) - \rho _{2} \sin (\rho _{2} x_{3} )} \right.\left. { + p\left( { - r_{1} \cos (\rho _{2} x_{3} ) + r_{2} \sin (\rho _{2} x_{3} )} \right)} \right] \\ {}^{{(k)}}E_{{44}} (x_{3} ) & = e^{{ - {}^{{(k)}}\rho _{1} x_{3} }} {}^{{(k)}}C_{{55}} {}^{{(k)}}\left[ { - \rho _{1} \sin (\rho _{2} x_{3} ) + \rho _{2} \cos (\rho _{2} x_{3} )} \right. - \left. {p\left( {r_{1} \sin (\rho _{2} x_{3} ) + r_{2} \cos (\rho _{2} x_{3} )} \right)} \right] \\ \end{aligned}$$

(50)

with:

$$\begin{aligned} {}^{(k)}\rho_{1} = {}^{(k)}\left[ {\sqrt {\frac{{\sqrt {A_{1}^{2} + \left| \Delta \right|} }}{{2A_{0} }}} \cos \left( {\frac{{\arctan \left( {{{ - \sqrt {\left| \Delta \right|} } \mathord{\left/ {\vphantom {{ - \sqrt {\left| \Delta \right|} } {A_{1} }}} \right. \kern-0pt} {A_{1} }}} \right)}}{2}} \right)} \right] \hfill \\ {}^{(k)}\rho_{2} = {}^{(k)}\left[ {\sqrt {\frac{{\sqrt {A_{1}^{2} + \left| \Delta \right|} }}{{2A_{0} }}} \sin \left( {\frac{{\arctan \left( {{{ - \sqrt {\left| \Delta \right|} } \mathord{\left/ {\vphantom {{ - \sqrt {\left| \Delta \right|} } {A_{1} }}} \right. \kern-0pt} {A_{1} }}} \right)}}{2}} \right)} \right] \hfill \\ {}^{(k)}r_{1} = {}^{(k)}\left( {\frac{{\rho_{1} \left[ {C_{22} p^{2} - C_{55} \left( {\rho_{1}^{2} + \rho_{2}^{2} } \right)} \right]}}{{p\left( {C_{23} + C_{55} } \right)\left( {\rho_{1}^{2} + \rho_{2}^{2} } \right)}}} \right) \hfill \\ {}^{(k)}r_{2} = {}^{(k)}\left( {\frac{{\rho_{2} \left[ {C_{22} p^{2} + C_{55} \left( {\rho_{1}^{2} + \rho_{2}^{2} } \right)} \right]}}{{p\left( {C_{23} + C_{55} } \right)\left( {\rho_{1}^{2} + \rho_{2}^{2} } \right)}}} \right) \hfill \\ \end{aligned} ,$$

(51)

where ${}^{(k)}\Delta = {}^{(k)}(A_{1}^{2} - 4A_{0} A_{2} )$ and ${}^{(k)}A_{0}$, ${}^{(k)}A_{1}$ and ${}^{(k)}A_{2}$ are defined in Eq. (26).

Appendix 5: Derivation of displacements and stresses

An explicit relationship between ${}^{(k)}M(x_{3}^{k} )$ and ${}^{(1)}M(x_{3}^{0} )$ is obtained using Eqs. (34) and (35) and following the procedure presented in the main text for the heat conduction problem:

$$\begin{aligned} {}^{(k)}M(x_{3}^{k} ) = \left( {B^{k} } \right)^{ - 1} \left\{ {\prod\limits_{i = k}^{1} {\left\{ {\left( {B^{i} } \right){}^{(i)}E(x_{3}^{i} ){}^{(i)}E^{ - 1} (x_{3}^{i - 1} )} \right\}} \times \left\{ {{}^{(1)}M(x_{3}^{0} ) - {}^{(1)}Q(x_{3}^{0} )} \right\}} \right. \hfill \\ + \sum\limits_{i = 2}^{k} {\left( {\prod\limits_{j = k}^{i} {\left\{ {\left( {B^{j} } \right){}^{(j)}E(x_{3}^{j} ){}^{(j)}E^{ - 1} (x_{3}^{j - 1} )} \right\}} } \right.} \left. {\left. \times {\left\{ {\left( {B^{i - 1} } \right){}^{(i - 1)}Q(x_{3}^{i - 1} ) - {}^{(i)}Q(x_{3}^{i - 1} )} \right\}} \right)} \right\} + \, {}^{(k)}Q(x_{3}^{k} ) \hfill \\ \end{aligned}$$

(52)

Expressions relating the four unknown constants, ${}^{(k)}a_{11}$, ${}^{(k)}a_{21}$, ${}^{(k)}a_{12}$ and ${}^{(k)}a_{22}$, to ${}^{(1)}M(x_{3}^{0} )$ are derived by substituting ${}^{(k)}M(x_{3}^{k} )$ on the left hand side of (52) with Eq. (32) and multiplying both sides by ${}^{(k)}E^{ - 1} (x_{3}^{k} )$:

$$\begin{aligned} {}^{(k)}\left[ {\begin{array}{*{20}c} {a_{11} } \\ {a_{21} } \\ {a_{12} } \\ {a_{22} } \\ \end{array} } \right] = {}^{(k)}E^{ - 1} (x_{3}^{k} )\left( {B^{k} } \right)^{ - 1} \times \left\{ {\prod\limits_{i = k}^{1} {\left\{ {\left( {B^{i} } \right){}^{(i)}E(x_{3}^{i} ){}^{(i)}E^{ - 1} (x_{3}^{i - 1} )} \right\}} } \right.\left\{ {{}^{(1)}M(x_{3}^{0} ) - {}^{(1)}Q(x_{3}^{0} )} \right\} \hfill \\ + \sum\limits_{i = 2}^{k} {\left( {\prod\limits_{j = k}^{i} {\left\{ {\left( {B^{j} } \right){}^{(j)}E(x_{3}^{j} ){}^{(j)}E^{ - 1} (x_{3}^{j - 1} )} \right\}} } \right.} \left. {\left. \times {\left\{ {\left( {B^{i - 1} } \right){}^{(i - 1)}Q(x_{3}^{i - 1} ) - {}^{(i)}Q(x_{3}^{i - 1} )} \right\}} \right)} \right\} \hfill \\ \end{aligned}$$

(53)

for k = 2, …, n. Inserting the expressions of the unknown in (53) into (32) yields:

$$\begin{aligned} {}^{(k)}M(x_{3} ) = {}^{(k)}Q(x_{3} ) + {}^{(k)}E(x_{3} ){}^{(k)}E^{ - 1} (x_{3}^{k} )\left( {B^{k} } \right)^{ - 1} \times \left\{ {\prod\limits_{i = k}^{1} {\left\{ {\left( {B^{i} } \right){}^{(i)}E(x_{3}^{i} ){}^{(i)}E^{ - 1} (x_{3}^{i - 1} )} \right\}} \left\{ {{}^{(1)}M(x_{3}^{0} ) - {}^{(1)}Q(x_{3}^{0} )} \right\}} \right. \hfill \\ + \sum\limits_{i = 2}^{k} {\left( {\prod\limits_{j = k}^{i} {\left\{ {\left( {B^{j} } \right){}^{(j)}E(x_{3}^{j} ){}^{(j)}E^{ - 1} (x_{3}^{j - 1} )} \right\}} } \right.} \left. \times {\left. {\left\{ {\left( {B^{i - 1} } \right){}^{(i - 1)}Q(x_{3}^{i - 1} ) - {}^{(i)}Q(x_{3}^{i - 1} )} \right\}} \right)} \right\} \hfill \\ \end{aligned}$$

(54)

Equation (30) defines displacements and transverse stresses in the layer k in terms of ${}^{(k)}M(x_{3} )$, which is given in Eq. (54). The elements of the vector ${}^{(1)}M(x_{3}^{0} )$ in Eq. (54) are defined as follows. The third and fourth elements are obtained using Eq. (30) for $x_{3} = x_{3}^{0}$ and k = 1 and the boundary conditions (10); the first and second elements are obtained using Eqs. (52) for k = n and the boundary conditions (10):

$$\begin{aligned} &{}^{(1)}M_{1} (x_{3}^{0} ) = {}^{(n)}\left( {\frac{{\varOmega_{ \, 42} }}{{\varOmega_{ \, 42} \varOmega_{ 31} - \varOmega_{ \, 41} \varOmega_{ 32} }}} \right)\left[ {f_{u} + \mu_{3} - \frac{{{}^{(n)}\varOmega_{{{ 3}2}} }}{{{}^{(n)}\varOmega_{{{ 4}2}} }}\mu_{4} } \right] \hfill \\ &{}^{(1)}M_{2} (x_{3}^{0} ) = \frac{1}{{{}^{(n)}\varOmega_{{{ 4}2}} }}\left[ { - {}^{(n)}\varOmega_{ 41} {}^{(1)}M_{1} (x_{3}^{0} ) + \mu_{4} } \right] \hfill \\ &{}^{(1)}M_{3} (x_{3}^{0} ) = - f_{l} \hfill \\ &{}^{(1)}M_{4} (x_{3}^{0} ) = 0 \hfill \\ \end{aligned}$$

(55)

where $\mu_{i}$ for i = 3, 4 are:

$$\mu_{i} = \sum\limits_{g = 1}^{4} {{}^{(n)}\varOmega_{ \, ig} } {}^{(1)}Q_{g} (x_{3}^{0} ) + {}^{(n)}\varOmega_{ \, i3} f_{l} - {}^{(n)}S_{i} - {}^{(n)}Q_{i} (x_{3}^{n} )$$

(56)

and ${}^{(k)}\varOmega_{ \, ig}$ and ${}^{(k)}S_{i}$ are defined by the recursive formulas:

$$\begin{aligned} & {}^{(k)}\varOmega_{ \, ig} = \sum\limits_{m = 1}^{4} {{}^{(k)}U_{im} } {}^{(k - 1)}\varOmega_{ \, mg} {\text{ for }}k{ = 2,} \ldots , { }n{ ; }{}^{(1)}\varOmega_{ \, ig} { = }{}^{(1)}U_{ig} \\ & {}^{(k)}U_{im} = \sum\limits_{n = 1}^{4} {\left\{ {\sum\limits_{p = 1}^{4} {B_{ip}^{k} {}^{(k)}E_{pn} (x_{3}^{k} )} } \right\}} {}^{(k)}E^{ - 1}_{nm} (x_{3}^{k - 1} ) \\ & {}^{(k)}S_{i} = \sum\limits_{q = 1}^{4} {{}^{(k)}U_{iq} \left[ {{}^{(k - 1)}S_{q} - {}^{(k)}Q_{q} (x_{3}^{k - 1} ) + \sum\limits_{b = 1}^{4} {\left( {B^{k - 1} } \right)_{qb} {}^{(k - 1)}Q_{b} (x_{3}^{k - 1} )} } \right]} \, \;{\text{for}}\;k = 2, \ldots ,n;\;{}^{(1)}S_{i} = 0 \, \\ \end{aligned}$$

(57)

where the elements of ${}^{(k)}Q(x_{3} )$ and $B^{k}$ are given in Eqs. (33) and (36) and those of ${}^{(k)}E(x_{3} )$ in Appendix 4. For fully bonded layers Eq. (57) simplifies as:

$$\begin{aligned} & {}^{(k)}\varOmega_{ \, ig} = \sum\limits_{m = 1}^{4} {{}^{(k)}U_{im} } {}^{(k - 1)}\varOmega_{ \, mg} \, {\text{for}} \, k \, = \, 2, \, \ldots , \, n \, ; \, {}^{(1)}\varOmega_{ \, ig} = {}^{(1)}U_{ig} \\ & {}^{(k)}U_{im} = \sum\limits_{n = 1}^{4} {{}^{(k)}E_{in} (x_{3}^{k} )} {}^{(k)}E^{ - 1}_{nm} (x_{3}^{k - 1} ) \\ & {}^{(k)}S_{i} = \sum\limits_{q = 1}^{4} {{}^{(k)}U_{iq} \left[ {{}^{(k - 1)}S_{q} - {}^{(k)}Q_{q} (x_{3}^{k - 1} ) + {}^{(k - 1)}Q_{q} (x_{3}^{k - 1} )} \right]} \, {\hbox{for}} \, k \, = \, 2, \, \ldots , \, n \, ;{}^{(1)}S_{i} = 0 \, \\ \end{aligned}$$

(58)

Explicit expressions for displacements and stresses in the generic layer k are given in Eqs. (38) in the main text.