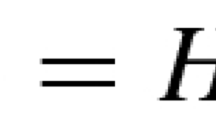Abstract
Consider a weakly nonlinear CGL equation on the torus \(\mathbb {T}^d\):
Here \(u=u(t,x)\), \(x\in \mathbb {T}^d\), \(0<\epsilon <<1\), \(\mu \geqslant 0\), \(b,c\in \mathbb {R}\) and \(m,p,q\in \mathbb {N}\). Define \(I(u)=(I_{\mathbf {k}},\mathbf {k}\in \mathbb {Z}^d)\), where \(I_{\mathbf {k}}=v_{\mathbf {k}}\bar{v}_{\mathbf {k}}/2\) and \(v_{\mathbf {k}}\), \(\mathbf {k}\in \mathbb {Z}^d\), are the Fourier coefficients of the function \(u\) we give. Assume that the equation \((*)\) is well posed on time intervals of order \(\epsilon ^{-1}\) and its solutions have there a-priori bounds, independent of the small parameter. Let \(u(t,x)\) solve the equation \((*)\). If \(\epsilon \) is small enough, then for \(t\lesssim {\epsilon ^{-1}}\), the quantity \(I(u(t,x))\) can be well described by solutions of an effective equation:
where the term \(F(u)\) can be constructed through a kind of resonant averaging of the nonlinearity \(b|u|^{2p}+ ic|u|^{2q}u\).
Similar content being viewed by others
Notes
The result of the present paper is part of the Ph.D works of the author ([8]) at École Polytechnique, France.
References
Bambusi, D.: Galerkin averaging method and Poincaré normal form for some quasilinear PDEs. Ann. Sc. Norm. Super. Pisa Cl. Sci 5(4), 669–702 (2005)
Bourgain, J.: Fourier transform restriction phenomena for certain lattice subsets and applications to nonlinear evolution equations. Geometric Funct Anal 3(2), 107–156 (1993)
Brézis, H., Gallouet, T.: Nonlinear Schrödinger evolution equations. Nonlinear Anal 4(4), 677–681 (1980)
I.D. Chueshov. Introduction to the Theory of Infinite-Dimensional Dissipative Systems. ACTA Scientific Publishing House, 2002.
Faou, E., Germain, P., Hani, Z.: The weakly nonlinear large box limit of the 2d cubic nonlinear Schrödinger equation. preprint, 2013. arXiv:1308.6267.
Gérard, P., Grellier, S.: Effective integrable dynamics for a certain nonlinear wave equation. Anal PDE 5, 1139–1154 (2012)
Herr, S., Tataru, D., Tzvetkov, N.: Global well-posedness of the energy-critical nonlinear Schrödinger equation with small initial data in \( {H^1}(\mathbb{T}^3)\). Duke Math J 159(2), 329–349 (2011)
Huang, G.: Une théorie de la moyenne pour les équations aux dérivées partielles nonlinéaires. PhD thesis, École Polytechnique, Frane, 2014.
Kuksin, S., Maiocchi, A.: Resonant averaging for weakly nonlinear stochastic Schrödinger equations. preprint, 2013. arXiv:1309.5022.
Nazarenko, S.: Wave Turbulence. Springer (2011)
Nirenberg, L.: On elliptic partial differential equations. Ann. Sci. Norm. Sup. Pisa 13, 115–162 (1959)
Taylor, M.: Partial differential equations, Vol. III. Springer, 1996.
Acknowledgments
The author wants to thank his Ph.D supervisor professor Sergei Kuksin for formulation of the problem and guidance. He is grateful to professor Dario Bambusi for his useful comments. Finally, he wants to thank the staff and faculty at C.M.L.S of École Polytechnique for their support.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Huang, G. Long-time Dynamics of Resonant Weakly Nonlinear CGL Equations. J Dyn Diff Equat 28, 375–387 (2016). https://doi.org/10.1007/s10884-014-9391-0
Received:
Revised:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10884-014-9391-0