Entanglement Dynamics of Two Spins in Initially Correlated Wheel-Shaped Spin Baths

Abstract

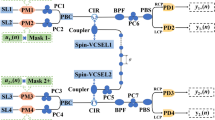
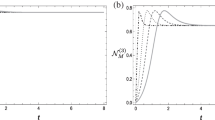
We study the effects of the initial correlations in environment on the entanglement dynamics of spin system. The correlated environment is novelly simulated by two correlated wheel-shaped spin baths, each consisting of an intermediate spin interacting with a spin-ring. The correlations in environment are achieved by the entanglement between two intermediate spins. The spin system includes two system-spins, and the interaction between the spin system and the environment is implemented by the coupling between the system-spin and the intermediate spin. Firstly, we analyze the influences of the initial entanglement between the two intermediate spins, the coupling parameters and the temperature of the baths on the entanglement dynamics of the two system-spins in equivalent subsystems. It is demonstrated that the initial entanglement between the baths can act as a resource for the generation and the revivals of the entanglement of the system-spins. Moreover, the amount of the generation and the revivals of the entanglement of the system-spins can be enhanced by regulating the coupling constants and the temperature of the baths. In addition, we also investigate the influences of different coupling ratios in non-equivalent subsystems, it is found that changing the coupling ratios of two subsystems has a significant effect on the generation and revivals of entanglement of system-spins.

Appendix

In the Appendix we provide the method to obtain the exact time evolution operator and the reduced density matrix of the system-spins. Note that the environment is constituted by the intermediate spins and the spin-rings, it is difficult to trace over them together directly. Here, we adopt a skillful method to handle this problem. Firstly, we take the two system-spins and the two intermediate spins as a whole, and their reduced density matrix can be written as

$$ {\rho_{AaBb}}(t) = t{r_{{R_{1}}{R_{2}}}}[{U_{1}}(t) \otimes {U_{2}}(t){\rho_{AB}}(0)\otimes {\rho_{ab}}(0){\otimes\rho_{{R_{1}}}} \otimes {\rho_{{R_{2}}}}U_{1}^{\dag} (t) \otimes U_{2}^{\dag} (t)]. $$

(17)

Since there is no interaction between the subsystems 1 and 2 , we first concentrate on the time evolution operator of a single subsystem only. We use U _{i j} to denote the components of the single time evolution operator U _l acting on the basis $\{ \left | {00} \right \rangle$, $\left | {01} \right \rangle$, $\left | {10} \right \rangle$, $\left | {11} \right \rangle \}$, here |m n〉 corresponds to the states of the system-spin and the intermediate spin [20]. From the Schrodinger equation we obtain

$$\begin{array}{@{}rcl@{}} \frac{d}{{dt}}{U_{1j}} &=& - i{\kern 1pt} {\kern 1pt} \beta_{l} {b^ + }{U_{2j}}, \end{array} $$

(18)

$$\begin{array}{@{}rcl@{}} \frac{d}{{dt}}{U_{2j}} &=& - i{\kern 1pt} {\kern 1pt} [ - \beta_{l} b{U_{1j}} + \alpha_{l} {U_{3j}}], \end{array} $$

(19)

$$\begin{array}{@{}rcl@{}} \frac{d}{{dt}}{U_{3j}} &=& - i{\kern 1pt} {\kern 1pt} [\alpha_{l} {U_{2j}} + \beta_{l} {b^ + }{U_{4j}}], \end{array} $$

(20)

$$\begin{array}{@{}rcl@{}} \frac{d}{{dt}}{U_{4j}} &=& - i{\kern 1pt} {\kern 1pt} \beta_{l} b{U_{3j}} . \end{array} $$

(21)

Here, j=1,2,3,4 is the number of the column of the evolution operator U _l in the chosen basis. Differentiating the equations (19) and (20), we obtain

$$\begin{array}{@{}rcl@{}} \frac{d}{{d{t^{2}}}}{U_{2j}} &=& - i{{\beta_{l}^{2}}}(1 + \hat n){U_{2j}} - i\alpha_{l} \frac{d}{{dt}}{U_{3j}} \end{array} $$

(22)

$$\begin{array}{@{}rcl@{}} \frac{d}{{d{t^{2}}}}{U_{3j}} &=& - i{{\beta_{l}^{2}}}\hat n{U_{3j}} - i\alpha_{l} \frac{d}{{dt}}{U_{2j}} \end{array} $$

(23)

where $\hat n=b^ + b$. By combining with the equations (18) and (21), we can get the explicit expressions of the evolution operator

$$\begin{array}{@{}rcl@{}} {U_{11}}\! &=&\! \frac{{({A_{1}} + {{\alpha_{l}^{2}}} - {{\beta_{l}^{2}}})\cosh (\frac{{{B_{1}}_ + t}}{{\sqrt{2}}})+({A_{1}} - {{\alpha_{l}^{2}}} + {{\beta_{l}^{2}}})\cosh (\frac{{{B_{1 - }}t}}{{\sqrt{2}}})}}{{2{A_{1}}}}, \end{array} $$

(24)

$$\begin{array}{@{}rcl@{}} {U_{21}}\! &=&\! \frac{{ - ib\beta_{l} [({A_{1}} + {{\alpha_{l}^{2}}} + {{\beta_{l}^{2}}}){B_{1 + }}\sinh (\frac{{{B_{1 - }}t}}{{\sqrt{2}}}) + ({A_{1}} - {{\alpha_{l}^{2}}} - {{\beta_{l}^{2}}}){B_{1 - }}\sinh (\frac{{{B_{1 + }}t}}{{\sqrt{2}}})]}}{{\sqrt{2}{A_{1}}{B_{1 - }}{B_{1 + }}}}, \end{array} $$

(25)

$$\begin{array}{@{}rcl@{}} {U_{31}}\! &=&\! \frac{{\alpha_{l} \beta_{l} b [\cosh (\frac{{{B_{1 - }}t}}{{\sqrt{2}}}) - \cosh (\frac{{{B_{1 + }}t}}{{\sqrt{2}}})]}}{{{A_{1}}}}, \end{array} $$

(26)

$$\begin{array}{@{}rcl@{}} {U_{41}}\! &=&\! \frac{{ - i\sqrt{2}\alpha_{l} {{\beta_{l}^{2}}}bb[{B_{1 + }}\sinh (\frac{{{B_{1 - }}t}}{{\sqrt{2}}}) - {B_{1 - }}\sinh (\frac{{{B_{1 + }}t}}{{\sqrt{2}}})]}}{{{A_{1}}{B_{1 - }}{B_{1 + }}}}, \end{array} $$

(27)

$$\begin{array}{@{}rcl@{}} {U_{12}} \!&=&\!\! \frac{{ - i\beta_{l}{b^ + }[({A_{1}}\! +\! {{\alpha_{l}^{2}}}\! +\! {{\beta_{l}^{2}}}){B_{1 + }}\sinh (\frac{{{B_{1 - }}t}}{{\sqrt{2}}})\! +\! ({A_{1}} - {{\alpha_{l}^{2}}} - {{\beta_{l}^{2}}}){B_{1 - }}\sinh (\frac{{{B_{1 + }}t}}{{\sqrt{2}}})]}}{{\sqrt{2}{A_{1}}{B_{1 - }}{B_{1 + }}}}, \end{array} $$

(28)

$$\begin{array}{@{}rcl@{}} {U_{22}}\! &=&\! \frac{{({A_{1}} + {{\alpha_{l}^{2}}} + {{\beta_{l}^{2}}})\cosh (\frac{{{B_{1 - }}t}}{{\sqrt{2}}}) + ({A_{1}} - {{\alpha_{l}^{2}}} - {{\beta_{l}^{2}}})\cosh (\frac{{{B_{1 + }}t}}{{\sqrt{2}}})}}{{2{A_{1}}}}, \end{array} $$

(29)

$$\begin{array}{@{}rcl@{}} {U_{32}}\! &=&\! {U_{23}} = \frac{{i\alpha_{l} [{B_{1 - }}\sinh (\frac{{{B_{1 - }}t}}{{\sqrt{2}}}) - {B_{1 + }}\sinh (\frac{{{B_{1 + }}t}}{{\sqrt{2}}})]}}{{\sqrt{2}{A_{1}}}}, \end{array} $$

(30)

$$\begin{array}{@{}rcl@{}} {U_{42}} &=& \frac{{\alpha_{l} \beta_{l} b [\cosh (\frac{{{B_{1 - }}t}}{{\sqrt{2}}}) - \cosh (\frac{{{B_{1 + }}t}}{{\sqrt{2}}})]}}{{{A_{1}}}}, \end{array} $$

(31)

$$\begin{array}{@{}rcl@{}} {U_{13}}\! &=&\! \frac{{\alpha_{l} \beta_{l} {b^ + } [\cosh (\frac{{{B_{1 - }}t}}{{\sqrt{2}}}) - \cosh (\frac{{{B_{1 + }}t}}{{\sqrt{2}}})]}}{{{A_{1}}}}, \end{array} $$

(32)

$$\begin{array}{@{}rcl@{}} {U_{33}}\! &=&\! \frac{{ ({A_{1}} + {{\alpha_{l}^{2}}} - {{\beta_{l}^{2}}})\cosh (\frac{{{B_{1 - }}t}}{{\sqrt{2}}}) + ({A_{1}} - {{\alpha_{l}^{2}}} + {{\beta_{l}^{2}}})\cosh (\frac{{{B_{1 + }}t}}{{\sqrt{2}}})}}{{2{A_{1}}}}, \end{array} $$

(33)

$$\begin{array}{@{}rcl@{}} {U_{43}}\! &=&\! \frac{{ - i\beta_{l} b [({A_{1}} + {{\alpha_{l}^{2}}} - {{\beta_{l}^{2}}}){B_{1 + }}\sinh (\frac{{{B_{1 - }}t}}{{\sqrt{2}}}) + ({A_{1}} - {{\alpha_{l}^{2}}} + {{\beta_{l}^{2}}}){B_{1 - }}\sinh (\frac{{{B_{1 + }}t}}{{\sqrt{2}}})]}}{{\sqrt{2}{A_{1}}{B_{1 - }}{B_{1 + }}}}, \end{array} $$

(34)

$$\begin{array}{@{}rcl@{}} {U_{14}}\! &=&\! \frac{{ - i\sqrt{2}\alpha_{l} {{\beta_{l}^{2}}}{b^ + }{b^ + }[{B_{1 + }}\sinh (\frac{{{B_{1 - }}t}}{{\sqrt{2}}}) - {B_{1 - }}\sinh (\frac{{{B_{1 + }}t}}{{\sqrt{2}}})]}}{{{A_{1}}{B_{1 - }}{B_{1 + }}}}, \end{array} $$

(35)

$$\begin{array}{@{}rcl@{}} {U_{24}}\! &=&\! \frac{{\alpha_{l} \beta_{l} {b^ + }[\cosh (\frac{{{B_{1 - }}t}}{{\sqrt{2}}}) - \cosh (\frac{{{B_{1 + }}t}}{{\sqrt{2}}})]}}{{{A_{1}}}}, \end{array} $$

(36)

$$\begin{array}{@{}rcl@{}} {U_{34}}\! &=&\! \frac{{ - i\beta_{l} {b^ + } [({A_{1}} + {{\alpha_{l}^{2}}}\! -\! {{\beta_{l}^{2}}}){B_{1 + }}\sinh (\frac{{{B_{1 - }}t}}{{\sqrt{2}}})\! +\! ({A_{1}}\! -\! {{\alpha_{l}^{2}}} + {{\beta_{l}^{2}}}){B_{1 - }}\sinh (\frac{{{B_{1 + }}t}}{{\sqrt{2}}})]}}{{\sqrt{2}{A_{1}}{B_{1 - }}{B_{1 + }}}}, \end{array} $$

(37)

$$\begin{array}{@{}rcl@{}} {U_{44}}\! &=&\! \frac{{({A_{1}} - {{\alpha_{l}^{2}}} - {{\beta_{l}^{2}}})\cosh (\frac{{{B_{1 - }}t}}{{\sqrt{2}}}) + ({A_{1}} + {{\alpha_{l}^{2}}} + {{\beta_{l}^{2}}})\cosh (\frac{{{B_{1 + }}t}}{{\sqrt{2}}})}}{{2{A_{1}}}}. \end{array} $$

(38)

In the above equations we have used the notation

$${A_{1}} = \sqrt{{{({{\alpha_{l}^{2}}} + {{\beta_{l}^{2}}})}^{2}} + 4{{\alpha_{l}^{2}}}{{\beta_{l}^{2}}}\hat n},{\kern 10pt}{B_{1, \pm }} = \sqrt{ - {{\alpha_{l}^{2}}} - {{\beta_{l}^{2}}}(1 + 2\hat n) \pm {A_{1}}} $$

Having determined the exact analytical form of the evolution coefficients U _{i j} of the single subsystem, we could get the total components of the time evolution operator U _{t o t} (t). And after the trace over the intermediate spins, we can find the explicit reduced density matrix of the two system-spins as

$$\begin{array}{l} {\rho_{AB}}(t) = \left( {\begin{array}{*{20}{c}} {{\rho_{11}}(t)}&0&0&{{\rho_{14}}(t)}\\ 0&{{\rho_{22}}(t)}&{{\rho_{23}}(t)}&0\\ 0&{\rho_{23}^ *(t)}&{{\rho_{33}}(t)}&0\\ {\rho_{14}^ * (t)}&0&0&{{\rho_{44}}(t)} \end{array}} \right) \end{array}$$

where

$$\begin{array}{l} {\rho_{11}}(t) = {|{c_{1}}|^{2}}{f_{1}}(t) {f_{2}}(t) + {|{c_{2}}|^{2}}{f_{3}}(t) {f_{4}}(t), \end{array}$$

$$\begin{array}{l} {\rho_{44}}(t) = {|{c_{1}}|^{2}}{g_{1}}(t) {g_{2}}(t) + {|{c_{2}}|^{2}}{g_{3}}(t) {g_{4}}(t), \end{array}$$

$$\begin{array}{l} {\rho_{22}}(t) = {|{c_{1}}{d_{1}}|^{2}}{f_{1}}(t) {g_{2}}(t) + {|{c_{1}}{d_{2}}|^{2}}{f_{2}}(t) {g_{1}}(t)\\ {\kern 20pt}+{|{c_{2}}{d_{1}}|^{2}}{f_{3}}(t) {g_{4}}(t) + {|{c_{2}}{d_{2}}|^{2}}{f_{4}}(t) {g_{3}}(t), \end{array}$$

$$\begin{array}{l} {\rho_{33}}(t) = {| {{c_{1}}{d_{2}}} |^{2}}{f_{1}}(t) {g_{2}}(t) + {| {{c_{1}}{d_{1}}}|^{2}}{f_{2}}(t) {g_{1}}(t) \\ {\kern 20pt}+{|{c_{2}}{d_{2}}|^{2}}{f_{3}}(t) {g_{4}}(t) + {|{c_{2}}{d_{1}}|^{2}}{f_{4}}(t) {g_{3}}(t), \end{array}$$

$$\begin{array}{l} {\rho_{23}}(t) = {|{c_{1}}|^{2}}{d_{1}}d_{2}^ * {h_{3}^{2}}(t) + {|{c_{2}}|^{2}}{d_{1}}d_{2}^ * {h_{4}^{2}}(t), \end{array}$$

$$\begin{array}{l} {\rho_{14}}(t) = \rho_{41}^ * (t) = {c_{1}}c_{2}^ * {h_{1}}(t){h_{2}}(t), \end{array}$$

and

$$\begin{array}{l} {f_{1}}(t) = \frac{1}{Z}(\sum\limits_{n = 0}^{\infty} {{U_{11}}} U_{11}^{\dag} {e^{- 2\gamma n/T}} +\sum\limits_{n = 1}^{\infty} {{U_{21}}} U_{21}^{\dag} {e^{- 2\gamma n/T}}), \end{array}$$

$$\begin{array}{l} {f_{2}}(t) = \frac{1}{Z}(\sum\limits_{n = 0}^{\infty} {{U_{12}}} U_{12}^{\dag} {e^{- 2\gamma n/T}}+ \sum\limits_{n = 0}^{\infty} {{U_{22}}} U_{22}^{\dag} {e^{- 2\gamma n/T}}), \end{array}$$

$$\begin{array}{l} {f_{3}}(t) = \frac{1}{Z}(\sum\limits_{n = 0}^{\infty} {{U_{13}}} U_{13}^{\dag} {e^{- 2\gamma n/T}} + \sum\limits_{n = 0}^{\infty} {{U_{23}}} U_{23}^{\dag} {e^{- 2\gamma n/T}}), \end{array}$$

$$\begin{array}{l} {f_{4}}(t) = \frac{1}{Z}(\sum\limits_{n = 0}^{\infty} {{U_{14}}} U_{14}^{\dag} {e^{- 2\gamma n/T}} + \sum\limits_{n = 0}^{\infty} {{U_{24}}} U_{24}^{\dag} {e^{- 2\gamma n/T}}), \end{array}$$

$$\begin{array}{l} {g_{1}}(t) = \frac{1}{Z}(\sum\limits_{n = 1}^{\infty} {{U_{31}}} U_{31}^{\dag} {e^{- 2\gamma n/T}} + \sum\limits_{n = 2}^{\infty} {{U_{41}}} U_{41}^{\dag} {e^{- 2\gamma n/T}}), \end{array}$$

$$\begin{array}{l} {g_{2}}(t) = \frac{1}{Z}(\sum\limits_{n = 0}^{\infty} {{U_{32}}} U_{32}^{\dag} {e^{- 2\gamma n/T}} + \sum\limits_{n = 1}^{\infty} {{U_{42}}} U_{42}^{\dag} {e^{- 2\gamma n/T}}), \end{array}$$

$$\begin{array}{l} {g_{3}}(t) = \frac{1}{Z}(\sum\limits_{n = 0}^{\infty} {{U_{33}}} U_{33}^{\dag} {e^{- 2\gamma n/T}} + \sum\limits_{n = 1}^{\infty} {{U_{43}}} U_{43}^{\dag} {e^{- 2\gamma n/T}}), \end{array}$$

$$\begin{array}{l} {g_{4}}(t) = \frac{1}{Z}(\sum\limits_{n = 0}^{\infty} {{U_{34}}} U_{34}^{\dag} {e^{- 2\gamma n/T}} + \sum\limits_{n = 0}^{\infty} {{U_{44}}} U_{44}^{\dag} {e^{- 2\gamma n/T}}), \end{array}$$

$$\begin{array}{l} {h_{1}}(t) = \frac{1}{Z}(\sum\limits_{n = 0}^{\infty} {{U_{11}}} U_{33}^{\dag} {e^{- 2\gamma n/T}} + \sum\limits_{n = 1}^{\infty} {{U_{21}}} U_{43}^{\dag} {e^{- 2\gamma n/T}}), \end{array}$$

$${h_{2}}(t) = \frac{1}{Z}(\sum\limits_{n = 0}^{\infty} {{U_{12}}} U_{34}^{\dag} {e^{- 2\gamma n/T}} + \sum\limits_{n = 0}^{\infty} {{U_{22}}} U_{44}^{\dag} {e^{- 2\gamma n/T}}), $$

$$\begin{array}{l} {h_{3}}(t) = \frac{1}{Z}(\sum\limits_{n = 0}^{\infty} {{U_{11}}} U_{32}^{\dag} {e^{- 2\gamma n/T}} + \sum\limits_{n = 1}^{\infty} {{U_{21}}} U_{42}^{\dag} {e^{- 2\gamma n/T}}), \end{array}$$

$$\begin{array}{l} {h_{4}}(t) = \frac{1}{Z}(\sum\limits_{n = 0}^{\infty} {{U_{13}}} U_{34}^{\dag} {e^{- 2\gamma n/T}} + \sum\limits_{n = 0}^{\infty} {{U_{23}}} U_{44}^{\dag} {e^{- 2\gamma n/T}}). \end{array}$$

In the above expressions, $Z = \frac {1}{{1 - {e^{- 2\gamma /T}}}}$.