On thermal resistance in concentric residential geothermal heat exchangers

Abstract

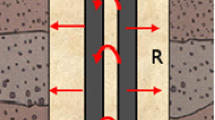
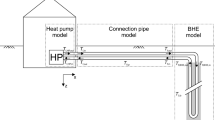
Residential geothermal ground-source heat pumps have been used for nearly 30 years as a low-cost, environmentally friendly alternative to traditional fossil-fuel systems. However, the limitation on a wider range of acceptance of the technology is the cost of the installation of a piping network through which the energy is transferred between the soil and the coolant. This cost is proportional to the piping length. We formulate a new mathematical modeling framework that calculates a characteristic streamwise length based on the geometry of the system, the operating conditions, and the material properties of the system materials and effective properties of the surrounding soil using a vertical concentric geothermal heat exchanger as an example. These concentric systems consist of a core flow (from the residence), which flows from the ground surface to the base of the well, and an annular return region in which the heat exchange between the fluid and the soil is expected to take place. Two modeling scenarios are considered: steady-state temperature profiles in the annular fluid region if the radial thermal resistance between the fluid and soil is fixed; a quasi-steady fluid temperature that captures the radial heat transfer from the fluid to the soil. For the first case, we find that the characteristic length is determined by the smallest eigenvalue of the separable thermal problem, where the velocity profile is laminar and there is no thermal transport between the core and the fluid. When this core-annular heat transfer is possible, the eigenvalue problem no longer satisfies the conditions for Sturm–Liouville theory, and through direct computation we find that energy transferred from the annular flow to the core reduces the temperature change. In the second case, we find that the temperature change is reduced over time, as the soil temperature near the exchanger responds to the energy transport. In both cases, the best thermal transport takes place when the annular gap is small. The impact of these results on system design considerations is discussed.

Appendix: Finite difference computational model

To find the steady-state temperature in the core and in the annular region for $k \ne 0$ with Nu constant, we computationally model system (45) and (46), subject to boundary conditions (47)–(50). Our approach is to use finite difference methods in the radial variable $r$ and the axial variable $z$. Note that (45) is computationally stable when solving the problem backward in $z$. We prescribe a specific temperature profile along $z = 1, 0 < r < 1-\epsilon$, and a well-posed computational solution can be found. However, the temperature profile in the annular region is computationally stable when solving the problem forward in $z$. Hence, any direct computational approach to find the temperature profiles for both regions simultaneously is likely to be computationally unstable.

Instead, we adopt an iterative approach to find the steady-state temperature profiles. To do this, we introduce a heat flux within the inner tube region $1-\epsilon < r < 1$, which depends on $z$. If this flux is denoted by $q_j(z)$ during the $j\mathrm{th}$ iteration of the scheme, then we solve the following initial-value problems for each iteration:

$$\begin{aligned}&-\mathrm{Pe}\,\left( \frac{R^2-1}{(1-\epsilon )^2}\right) \,w_1(r)\frac{\partial \theta ^{(1)}}{\partial z} = \frac{1}{r} \frac{\partial }{\partial r} \left( r\frac{\partial \theta ^{(1)}}{\partial r}\right) , \quad 0 < r < 1-\epsilon , \quad 0 < z < 1 , \end{aligned}$$

(72)

$$\begin{aligned}&\lim _{r\rightarrow 0} r \frac{\partial \theta ^{(1)}}{\partial r} = 0, \quad \left. \frac{\partial \theta ^{(1)}}{\partial r}\right| _{r = 1-\epsilon } = \frac{k\,q_j(z)}{1-\epsilon } , \end{aligned}$$

(73)

$$\begin{aligned}&\theta ^{(1)}(r,1) = 1, \end{aligned}$$

(74)

$$\begin{aligned}&\mathrm{Pe}\,w_2(r)\frac{\partial \theta ^{(2)}}{\partial z} = \frac{1}{r} \frac{\partial }{\partial r} \left( r\frac{\partial \theta ^{(2)}}{\partial r}\right) \!, \quad 1 < r < R, \quad 0 < z < 1 , \end{aligned}$$

(75)

$$\begin{aligned}&\left. \frac{\partial \theta ^{(2)}}{\partial r}\right| _{r = 1} = {k\,q_j(z)}, \quad \left. \frac{\partial \theta ^{(1)}}{\partial r} + \mathrm{Nu} \theta ^{(2)}\right| _{r = R} = 0, \end{aligned}$$

(76)

$$\begin{aligned}&\theta ^{(2)}(r,0) = A_2\left\{ 1-\frac{\mathrm{Nu}(1-r^2)}{2(R-1)+\mathrm{Nu}(R-1)^2}\right\} , \end{aligned}$$

(77)

where $A_2 = \theta ^{(1)}(1-\epsilon ,0)+q_j(0)$ allows continuity of temperature at the inner tube wall for $z = 0$ and satisfies boundary condition (30). Note that this formulation satisfies jump condition (43).

The goal is to form a converging sequence of functions $q_j$ such that the last condition (44) is satisfied. To do this, we set

$$\begin{aligned} \hat{q} = \frac{1}{\log {\left( \frac{1}{1-\epsilon }\right) }} \left[ \theta ^{(2)}(1,z)-\theta ^{(1)}(1-\epsilon ,z)\right] \end{aligned}$$

(78)

and use a fixed-point iteration to determine the next approximation to the sequence $q_j$:

$$\begin{aligned} q_{j+1}(z) = q_j(z) + \tanh {\frac{\hat{q}(z)-q_j(z)}{10}}. \end{aligned}$$

(79)

The iteration is said to converge when a quadrature approximation to the error,

$$\begin{aligned} \tau = \sqrt{\int _0^1\left( q_{j+1}-q_{j}\right) ^2\,\mathrm{d}z}, \end{aligned}$$

(80)

is below a specified tolerance $\tau < 10^{-5}$.

To determine the solution of (72) subject to (73), and analogously the solution of (75) subject to (76), with $q_j(z)$ given, we discretize the spatial domain with a uniform grid interior to the domain boundary in $r$ and use a Crank–Nicolson scheme, where the time variable is given by $z$ oriented in the same direction as the velocity field, and a nonstandard Mickens-type scheme is used for the radial differential operator [29]. That is, for the core fluid we march the solution from $z = 1$ to $z = 0$, and for the annular fluid we march from $z = 0$ to $z = 1$. For clarity in describing the Crank–Nicolson method, let us consider the canonical heat equation

$$\begin{aligned}&\frac{\partial u}{\partial t} = \mathcal L \left\{ u\right\} = \frac{1}{r}\frac{\partial }{\partial r} \left( r\frac{\partial u}{\partial r}\right) \!, \quad 0 < r < 1\!, \quad t > 0 \end{aligned}$$

(81)

$$\begin{aligned}&u(r,0) = u_o(r), \quad \lim _{r\rightarrow 0} \frac{\partial u}{\partial r} = 0, \quad \frac{\partial u}{\partial r}(1,t) = q. \end{aligned}$$

(82)

We approximate $u(r_m,t_n) \approx U_m^n$, and we let $\Delta r = r_{m+1}-r_m$, $\Delta t = t_{n+1}-t_n$ be a constant for positive integers $m,n \le N$, resulting in a uniform grid on $0 < r < 1$, $0 < t < T$. We then implement Crank–Nicolson for (81)

$$\begin{aligned} \mathbf{U}^{n+1}-\mathbf{U}^n = \frac{\Delta t}{2} \left\{ \mathbf{L} \mathbf{U}^{n+1} + \mathbf{L} \mathbf{U}^n\right\} + (\Delta t)\mathbf{B}, \end{aligned}$$

(83)

where $\mathbf{U}^n = (U_1^n, U_2^n, \ldots , U_N^n)$ is the spatial vector of the solution at time $t = t_n$, and the $m\mathrm{th}$ element of the nonstandard discretization for $\mathcal L$ is given by

$$\begin{aligned} \left( \mathbf{L} \mathbf{U}\right) _m = \frac{1}{r_m}\left( \frac{u_{m+1}-u_m}{\log {(r_{m+1}/r_m)}} - \frac{u_m - u_{m-1}}{\log {(r_m/r_{m-1})}}\right) . \end{aligned}$$

(84)

The vector $\mathbf{B}$ corresponds to the inhomogeneous boundary conditions that result from points not on the computational grid (e.g., $r = r_o = 0, r = r_{N+1} = 1$). In the simulations shown in this paper, $N = 200$ for each radial domain $0 < r < 1-\epsilon$ and $1 < r < R$, along with $N = 200$ in the axial domain.