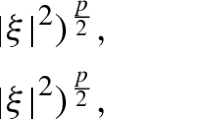Skip to main content
SpringerLink
Log in

Regularity for free interface variational problems in a general class of gradients

  • Published:
Calculus of Variations and Partial Differential Equations Aims and scope Submit manuscript

Abstract

We present a way to study a wide class of optimal design problems with a perimeter penalization. More precisely, we address existence and regularity properties of saddle points of energies of the form

$$\begin{aligned} (u,A) \quad \mapsto \quad \int _\Omega 2fu \,\mathrm {d}x \; - \int _{\Omega \cap A} \sigma _1\mathscr {A}u\cdot \mathscr {A}u \, \,\mathrm {d}x \; - \int _{\Omega {\setminus } A} \sigma _2\mathscr {A}u\cdot \mathscr {A}u \, \,\mathrm {d}x \; + \; \text {Per }(A;\overline{\Omega }), \end{aligned}$$

where \(\Omega \) is a bounded Lipschitz domain, \(A\subset \mathbb {R}^N\) is a Borel set, \(u:\Omega \subset \mathbb {R}^N \rightarrow \mathbb {R}^d\), \(\mathscr {A}\) is an operator of gradient form, and \(\sigma _1, \sigma _2\) are two not necessarily well-ordered symmetric tensors. The class of operators of gradient form includes scalar- and vector-valued gradients, symmetrized gradients, and higher order gradients. Therefore, our results may be applied to a wide range of problems in elasticity, conductivity or plasticity models. In this context and under mild assumptions on f, we show for a solution (w, A), that the topological boundary of \(A \cap \Omega \) is locally a \(\mathrm {C}^1\)-hypersurface up to a closed set of zero \(\mathscr {H}^{N-1}\)-measure.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

Notes

  1. Here, \(\mathrm {Per}(A;\overline{\Omega }) = |\mu _A|(\overline{\Omega })\), where \(\mu _A\) is the Gauss–Green measure of A; see Sect. 2.4.

  2. Due to the nature of the problem, we cannot replace \(\mathrm {Per}(A;\overline{\Omega })\) with \(\mathrm {Per}(A;\Omega )\) in E(A) because it possible that minimizing sequences tend to accumulate perimeter in \(\partial \Omega \).

  3. Here, \(\mathrm {W}^\mathscr {A}(\Omega ) = \big \{u \in \mathrm {L}^2(\Omega ;\mathbb {R}^d) : \mathscr {A}u \in \mathrm {L}^2(\Omega ;\mathbb {R}^{dN^k}) \big \}\) is the \(\mathscr {A}\)-Sobolev space of \(\Omega \).

  4. Possibly abusing the notation, we will denote by \(C(\Omega )\) the Poincaré constants from Definition 2.1 and Remark 2.4.

  5. Here, \(\mathscr {B}\) is a second order operator expressing the Saint-Venant compatibility conditions.

  6. As stated in Sect. 2.4, we write \(A \in \mathrm {BV}_\mathrm{{loc }}(\mathbb {R}^N)\) to express that A is a Borel set of locally finite perimeter in \(\mathbb {R}^N\).

  7. The convergence of the total energy is not covered by Lemma 2.9; however, this can be deduced using integration by parts and the fact that \(w_h\) has zero boundary values for every \(h \in \mathbb N\).

  8. This scaling has the property that \(s^{N-1}J_{B_1}(\mathscr {A}w^s,A^s) = J_{B_s}(\mathscr {A}w,A)\).

  9. As it can be seen from the proof of Lemma 5.1, the constant \(c_1\) does not depend on K.

  10. The constant \(c_2\) is independent of the compact set K; indeed, this is the result of universal comparison estimates in \(\Omega \).

  11. The notation \(B_r^\pm \) stands for the upper and lower half ball of radius r: \(B_r \cap H\) and \(B_r \cap - H\) respectively.

  12. Recall that, for a 1-st order operator as in (7), the coefficients \(A_\alpha \) can be simply denoted by \(A_i\) with \(i = 1,\dots ,N\).

  13. \(\mathrm {L}^{2^*}(\Omega )\)-integrability of \(\mathscr {A}w\), for some exponent \(2^* > 2\), can be established by standard methods through the use of the Caccioppoli inequality in Lemma 2.5.

  14. Here, \(\nu _r\) is the \(\mathscr {A}\)-free corrector function for w in \(B_r\), see Definition 2.1.

References

  1. Hashin, Z., Shtrikman, S.: A variational approach to the theory of the elastic behaviour of multiphase materials. J. Mech. Phys. Solids 11, 127–140 (1963)

    Article  MathSciNet  MATH  Google Scholar 

  2. Kohn, R.V., Strang, G.: Optimal design and relaxation of variational problems. I. Commun. Pure Appl. Math. 39(1), 113–137 (1986)

    Article  MathSciNet  MATH  Google Scholar 

  3. Kohn, R.V., Strang, G.: Optimal design and relaxation of variational problems. II. Commun. Pure Appl. Math. 39(2), 139–182 (1986)

    Article  MathSciNet  MATH  Google Scholar 

  4. Kohn, R.V., Strang, G.: Optimal design and relaxation of variational problems. III. Commun. Pure Appl. Math. 39(3), 353–377 (1986)

    Article  MathSciNet  MATH  Google Scholar 

  5. Murat, F., Tartar, L.: Optimality conditions and homogenization. In: Nonlinear variational problems (Isola d’Elba, 1983), Res. Notes in Math., vol. 127, pp. 1–8. Pitman, Boston (1985)

  6. Murat, F., Tartar, L.: Calcul des variations et homogénéisation. In: Homogenization methods: theory and applications in physics (Bréau-sans-Nappe, 1983), Collect. Dir. Études Rech. Élec. France, vol. 57, pp. 319–369. Eyrolles, Paris (1985)

  7. Ambrosio, L., Buttazzo, G.: An optimal design problem with perimeter penalization. Calc. Var. Partial Differ. Equ. 1(1), 55–69 (1993)

    Article  MathSciNet  MATH  Google Scholar 

  8. Lin, F.H.: Variational problems with free interfaces. Calc. Var. Partial Differ. Equ. 1(2), 149–168 (1993)

    Article  MathSciNet  MATH  Google Scholar 

  9. Kohn, R.V., Lin, F.H.: Partial regularity for optimal design problems involving both bulk and surface energies. Chin. Ann. Math. Ser. B 20(2), 137–158 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  10. Larsen, C.J.: Regularity of components in optimal design problems with perimeter penalization. Calc. Var. Partial Differ. Equ. 16(1), 17–29 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  11. Fusco, N., Julin, V.: On the regularity of critical and minimal sets of a free interface problem. Interfaces Free Bound 17(1), 117–142 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  12. De Philippis, G., Figalli, A.: A note on the dimension of the singular set in free interface problems. Differ. Integr. Equ. 28(5–6), 523–536 (2015)

    MathSciNet  MATH  Google Scholar 

  13. Carozza, M., Fonseca, I., Di Napoli, A.P.: Regularity results for an optimal design problem with a volume constraint. ESAIM Control Optim. Calc. Var. 20(2), 460–487 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  14. De Giorgi, E.: Frontiere orientate di misura minima. Seminario di Matematica della Scuola Normale Superiore di Pisa, 1960–61. Editrice Tecnico Scientifica, Pisa (1961)

  15. Almgren, F.: Existence and regularity almost everywhere of solutions to elliptic variational problems among surfaces of varying topological type and singularity structure. Ann. Math. 2(87), 321–391 (1968)

    Article  MathSciNet  MATH  Google Scholar 

  16. Fonseca, I., Müller, S.: \({\cal{A}}\)-quasiconvexity, lower semicontinuity, and Young measures. SIAM J. Math. Anal. 30(6), 1355–1390 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  17. Carstensen, C., Müller, S.: Local stress regularity in scalar nonconvex variational problems. SIAM J. Math. Anal. 34(2), 495–509 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  18. Brézis, H.: Analyse fonctionnelle. Collection Mathématiques Appliquées pour la Maîtrise, Masson, Paris

  19. Barton, A.: Gradient estimates and the fundamental solution for higher-order elliptic systems with rough coefficients. Manuscripta Mathematica 151(3–4), 375–418 (2016)

    Article  MathSciNet  Google Scholar 

  20. Milton, G.W.: The Theory of Composites, Cambridge Monographs on Applied and Computational Mathematics, vol. 6. Cambridge University Press, Cambridge (2002)

    Google Scholar 

  21. Ball, J.: A version of the fundamental theorem for Young measures. In: PDEs and continuum models of phase transitions. Proceedings of an NSF-CNRS joint seminar held in Nice, France, January 18–22, 1988, pp. 207–215. Springer, Berlin (1989)

  22. Maggi, F.: Sets of Finite Perimeter and Geometric Variational Problems, Cambridge Studies in Advanced Mathematics, vol. 135. Cambridge University Press, Cambridge (2012). (An introduction to geometric measure theory)

  23. Ambrosio, L., Fusco, N., Pallara, D.: Functions of Bounded Variation and Free Discontinuity Problems. Oxford Mathematical Monographs. The Clarendon Press, Oxford University Press, New York (2000)

    MATH  Google Scholar 

  24. Federer, H.: Geometric measure theory. Die Grundlehren der mathematischen Wissenschaften, Band 153. Springer-Verlag New York Inc., New York (1969)

  25. Tamanini, I.: Regularity Results for Almost Minimal Oriented Hypersurfaces in \(\mathbb{R}^n\). Dipartimento di Matematica dell’Università di Lecce, Lecce (1984)

    MATH  Google Scholar 

  26. Allaire, G., Kohn, R.V.: Optimal bounds on the effective behavior of a mixture of two well-ordered elastic materials. Q. Appl. Math. 51(4), 643–674 (1993)

    MathSciNet  MATH  Google Scholar 

  27. Allaire, G., Kohn, R.V.: Optimal lower bounds on the elastic energy of a composite made from two non-well-ordered isotropic materials. Q. Appl. Math. 52(2), 311–333 (1994)

    MathSciNet  MATH  Google Scholar 

  28. Evans, L.C.: Quasiconvexity and partial regularity in the calculus of variations. Arch. Rational Mech. Anal. 95(3), 227–252 (1986)

    Article  MathSciNet  MATH  Google Scholar 

  29. Sverák, V., Yan, X.: Non-Lipschitz minimizers of smooth uniformly convex functionals. Proc. Natl. Acad. Sci. USA 99(24), 15269–15276 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  30. Giaquinta, M.: Multiple Integrals In the Calculus of Variations and Nonlinear Elliptic Systems, Annals of Mathematics Studies, vol. 105. Princeton University Press, Princeton (1983)

    MATH  Google Scholar 

  31. Agmon, S., Douglis, A., Nirenberg, L.: Estimates near the boundary for solutions of elliptic partial differential equations satisfying general boundary conditions. I. Commun. Pure Appl. Math. 12, 623–727 (1959)

    Article  MathSciNet  MATH  Google Scholar 

  32. Agmon, S., Douglis, A., Nirenberg, L.: Estimates near the boundary for solutions of elliptic partial differential equations satisfying general boundary conditions. II. Commun. Pure Appl. Math. 17, 35–92 (1964)

    Article  MathSciNet  MATH  Google Scholar 

  33. Simon, L.: Schauder estimates by scaling. Calc. Var. Partial Differ. Equ. 5(5), 391–407 (1997)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

I wish to extend many thanks to Prof. Stefan Müller for his advice and fruitful discussions in this beautiful subject. I would also like to thank the reviewer and the editor for their patience and care which derived in the correct formulation of Theorem 1.5. The support of the University of Bonn and the Hausdorff Institute for Mathematics is gratefully acknowledged. The research conducted in this paper forms part of the author’s Ph.D. thesis at the University of Bonn.

Author information

Authors and Affiliations

  1. Institut für Angewandte Mathematik, Endenicher Allee 60, 53115, Bonn, Germany

    Adolfo Arroyo-Rabasa

Authors
  1. Adolfo Arroyo-Rabasa
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Adolfo Arroyo-Rabasa.

Additional information

Communicated by L. Ambrosio.

Glossary of constants

Glossary of constants

  • N spatial dimension

  • M coercivity and bounding constant for the tensors \(\sigma _1\) and \(\sigma _2\) (as quadratic forms)

  • K an arbitrary compact set in \(\Omega \)

  • \(\lambda _K\) local upper bound constant

Other constants Groups of constants are numbered in non-increasing order, e.g., \(c_1^* \ge c_2^* \ge c_3^*\). The following constants play an important role in our calculations:

Constant

Dependence

Description

\(\theta _1\)

arbitrary in (0, 1 / 2)

Ratio constant

\(c_1\)

\(\theta _1,N,M\)

Universal constant

\(\varepsilon _1\)

\(\theta _1, N, M\)

Smallness of perimeter density

\(c_2\)

N, M

Universal constant

\(\gamma \)

N, M

Universal constant

\(\theta _2\)

N, M

Universal constant

\(\varepsilon _2\)

N, M

Smallness of excess energy

\(\theta _0(\varepsilon )\)

N, M, K

Smallness of perimeter density

\(c^*_1\)

\(\lambda _K, N\)

Constant in the Height bound Lemma

\(\theta _1^*\)

arbitrary in (0, 1 / 2)

Ratio constant

\(c_2^*\)

\(\theta _1^*, N,M\)

Universal constant

\(\epsilon _2^*\)

\(\theta ^*_1,N, M\)

Smallness of flatness excess

\(c_3^*\)

K, N, M, f

Flatness excess improvement scaling constant

\(\varepsilon _3^*\)

K, N, M, f

Smallness of flatness excess

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Arroyo-Rabasa, A. Regularity for free interface variational problems in a general class of gradients. Calc. Var. 55, 154 (2016). https://doi.org/10.1007/s00526-016-1085-5

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s00526-016-1085-5

Mathematics Subject Classification

Search

Navigation