Geometry of the random walk range conditioned on survival among Bernoulli obstacles

Abstract

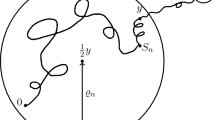
We consider a discrete time simple symmetric random walk among Bernoulli obstacles on ${{\mathbb {Z}}}^d$, $d\ge 2$, where the walk is killed when it hits an obstacle. It is known that conditioned on survival up to time N, the random walk range is asymptotically contained in a ball of radius $\varrho _N=C N^{1/(d+2)}$ for any $d\ge 2$. For $d=2$, it is also known that the range asymptotically contains a ball of radius $(1-\epsilon )\varrho _N$ for any $\epsilon >0$, while the case $d\ge 3$ remains open. We complete the picture by showing that for any $d\ge 2$, the random walk range asymptotically contains a ball of radius $\varrho _N-\varrho _N^\epsilon$ for some $\epsilon \in (0,1)$. Furthermore, we show that its boundary is of size at most $\varrho _N^{d-1}(\log \varrho _N)^a$ for some $a>0$.

Appendices

Estimates for eigenvalues and eigenfunctions

In this section, we collect some estimates on eigenvalues and eigenfunctions, including Lemma 5.1, and then prove (EV), (EF) (used in the proof of Lemma 4.5) and Lemma 5.2. Recall that $\lambda ^{(k)}_{T}$ and $\phi ^{(k)}_{T}$ are the k-th smallest Dirichlet eigenvalue and corresponding eigenfunction with $\Vert \phi ^{(k)}_{T}\Vert _2=1$ for $-\frac{1}{2d}\Delta$ in $T\subset {{\mathbb {R}}}^d$ and $\lambda ^{\mathrm{RW},(k)}_{T}$ and $\phi ^{\mathrm{RW},(k)}_{T}$ are their discrete space counterparts.

We begin with the following comparison lemma which includes Lemma 5.1.

Lemma A.1

For any $d\ge 1$ and $k\ge 1$, there exists $\gamma _k>0$ such that for all sufficiently large $R>0$, the following bounds hold:

$$\begin{aligned} \left| \lambda ^{\mathrm{RW},(k)}_{B(0;R)}-\lambda ^{(k)}_{B(0;R)}\right|&\le \gamma _k R^{-3}, \end{aligned}$$

(A.1)

$$\begin{aligned} {\min _{|y|\le 1}}\phi _{B(0;R)}^{\mathrm{RW},(1)}({y})&\ge \gamma _1^{-1} R^{-d/2}, \end{aligned}$$

(A.2)

and for any $x\in {{\mathbb {Z}}}^d$ and $T\supset B(x;\varrho _N/2)$,

$$\begin{aligned} \lambda ^{\mathrm{RW},(1)}_T\ge \lambda _{\widetilde{T}}^{(1)}-{\gamma _1} \varrho _N^{-4}. \end{aligned}$$

(A.3)

Proof of Lemma A.1

The first assertion can be found in [22, (3.27) and (6.11)]. The second assertion follows from [5, Lemma 2.1(b)], which states that

$$\begin{aligned} {\sup _{y\in B(0;R)}}\left| \phi _{B(0;R)}^{(1)}({y})-\phi _{B(0;R)}^{\mathrm{RW},(1)}({y})\right| \le cR^{-d/2-1}, \end{aligned}$$

(A.4)

and the fact that ${\min _{y\in {{\mathbb {Z}}}^d:|y|\le 1}}\phi _{B(0;R)}^{(1)}({y})\ge c^{-1}R^{-d/2}$. The third assertion follows from [22, (6.9)], which states that

$$\begin{aligned} \lambda _{\widetilde{T}}^{(1)}\le \lambda ^{\mathrm{RW},(1)}_T(1+c\varrho _N^{-2}), \end{aligned}$$

(A.5)

and the bound $\lambda ^{\mathrm{RW},(1)}_T\le c\varrho _N^{-2}$ that follows from the assumption $T\supset B(x;\varrho _N/2)$. $\square$

Next we restate and prove (EV) and (EF).

Lemma A.2

There exist $c_7,c_8>0$ such that if $B(x;(1-\epsilon )\varrho _N) \subset \mathcal{B}\subset B(x;\varrho _N+\varrho _N^{\epsilon _1})$ for some $x\in {{\mathbb {Z}}}^d$ and $\epsilon >0$ sufficiently small depending only on the dimension d, then the following bounds hold:

$$\begin{aligned} \lambda ^{\mathrm{RW},(2)}_{\mathcal{B}}-\lambda ^{\mathrm{RW},(1)}_{\mathcal{B}}&\ge c_7\varrho _N^{-2}, \end{aligned}$$

(EV)

$$\begin{aligned} \left\| \phi ^{\mathrm{RW},(1)}_{\mathcal{B}}\right\| _\infty&\le c_8\varrho _N^{-d/2}. \end{aligned}$$

(EF)

Proof of Lemma A.2

In order to show the first assertion (EV), recall first that the continuum eigenvalue satisfies the scaling relation $\lambda ^{(k)}_{B(0;R)}=R^{-2}\lambda ^{(k)}_{B(0;1)}$. Combining this with (A.1), we get the following bounds:

$$\begin{aligned} \begin{aligned} \lambda ^{\mathrm{RW},(1)}_{\mathcal{B}}&\le \lambda ^{\mathrm{RW},(1)}_{B(0;\varrho _N+\varrho _N^{\epsilon _1})}\\&\le \lambda ^{(1)}_{B(0;\varrho _N+\varrho _N^{\epsilon _1})}+{\gamma _1}\varrho _N^{-3}\\&\le \varrho _N^{-2}\lambda ^{(1)}_{B(0;1)}+c\varrho _N^{-3+\epsilon _1},\\ \end{aligned} \end{aligned}$$

(A.6)

and

$$\begin{aligned} \begin{aligned} \lambda ^{\mathrm{RW},(2)}_{\mathcal{B}}&\ge \lambda ^{\mathrm{RW}, (2)}_{B(0;(1-\epsilon )\varrho _N)}\\&\ge \lambda ^{(2)}_{B(0;(1-\epsilon )\varrho _N)}-{\gamma _2}\varrho _N^{-3}\\&\ge \varrho _N^{-2}\lambda ^{(2)}_{B(0;1)}-c\epsilon \varrho _N^{-2}. \end{aligned} \end{aligned}$$

(A.7)

Since $\lambda ^{(1)}_{B(0;1)}<\lambda ^{(2)}_{B(0;1)}$, the desired bound (EV) follows for sufficiently small $\epsilon >0$.

Next, we show the second assertion (EF). By the eigenvalue equation for the semigroup, it follows for any $x\in \mathcal{B}$ that

$$\begin{aligned} \begin{aligned} \phi ^{\mathrm{RW},(1)}_{\mathcal{B}}(x)&=\left( 1-\lambda ^{\mathrm{RW},(1)}_{\mathcal{B}}\right) ^{-\lfloor 1/\lambda ^{\mathrm{RW},(1)}_{\mathcal{B}}\rfloor }\sum _{y\in \mathcal{B}}p^\mathcal{B}_{\lfloor 1/\lambda ^{\mathrm{RW},(1)}_{\mathcal{B}}\rfloor }(x,y)\phi ^{\mathrm{RW},(1)}_{\mathcal{B}}(y)\\&\le \left( 1-\lambda ^{\mathrm{RW},(1)}_{\mathcal{B}}\right) ^{-\lfloor 1/\lambda ^{\mathrm{RW},(1)}_{\mathcal{B}}\rfloor }\left\| p^\mathcal{B}_{\lfloor 1/\lambda ^{\mathrm{RW},(1)}_{\mathcal{B}}\rfloor }(x,\cdot )\right\| _2 \left\| \phi ^{\mathrm{RW},(1)}_{\mathcal{B}}\right\| _2, \end{aligned} \end{aligned}$$

(A.8)

where we have used the Schwarz inequality in the second line. Then by the symmetry of the transition kernel $p^\mathcal{B}$, the Chapman–Kolmogorov identity and the normalization $\Vert \phi _\mathcal{B}^{(1)}\Vert _2=1$, we can further rewrite (A.8) as

$$\begin{aligned} \begin{aligned} |\phi ^{\mathrm{RW},(1)}_{\mathcal{B}}(x)|&\le \left( 1-\lambda ^{\mathrm{RW},(1)}_{\mathcal{B}}\right) ^{-\lfloor 1/\lambda ^{\mathrm{RW},(1)}_{\mathcal{B}}\rfloor }p^\mathcal{B}_{2\lfloor 1/\lambda ^{\mathrm{RW},(1)}_{\mathcal{B}}\rfloor }(x,x)^{1/2}\\&\le c\left( \lambda ^{\mathrm{RW},(1)}_{\mathcal{B}}\right) ^{d/4}, \end{aligned} \end{aligned}$$

(A.9)

where we used the local limit theorem as an upper bound. Since we have $\lambda ^{\mathrm{RW},(1)}_{\mathcal{B}}\ge c\varrho _N^{-2}$ similarly to (A.6), the last line is bounded by $c\varrho _N^{-d/2}$. $\square$

In order to prove Lemma 5.2, we use the eigenfunction expansion for the semigroup generated by the random walk killed upon exiting $B(0;\varrho _N)$, whose generator we denote by $Q_{\varrho _N}$. Due to the periodicity of the random walk, it is convenient to consider the semigroup generated by $Q_{\varrho _N}^2$. Let ${{\mathbb {Z}}}^d_{\mathrm{e}}$ (${{\mathbb {Z}}}^d_{\mathrm{o}}$) and $1_{\mathrm{e}}$ ($1_{\mathrm{o}}$) denote the set of even (odd) sites and its indicator function, respectively.

Lemma A.3

For any positive integer $k\le |B(0;\varrho _N)|/2$, the k-th largest eigenvalues of $Q_{\varrho _N}^2$ is $(1-\lambda _{B(0;\varrho _N)}^{\mathrm{RW},(k)})^2$. Moreover the eigenspace corresponding to $(1-\lambda _{B(0;\varrho _N)}^{\mathrm{RW},(1)})^2$ is spanned by $\phi _{B(0;\varrho _N)}^{\mathrm{RW},(1)}1_{\mathrm{e}}$ and $\phi _{B(0;\varrho _N)}^{\mathrm{RW},(1)}1_{\mathrm{o}}$.

Proof

For any eigenvalue $\zeta$ and corresponding eigenfunction $\phi _\zeta$ of $Q_{\varrho _N}$, we have

$$\begin{aligned} Q_{\varrho _N}1_{\mathrm{e}} \phi _\zeta =\zeta 1_{\mathrm{o}}\phi _\zeta \end{aligned}$$

(A.10)

and the same holds with $1_{\mathrm{e}}$ and $1_{\mathrm{o}}$ interchanged. It follows that

$$\begin{aligned} Q_{\varrho _N}(1_{\mathrm{e}} \phi _\zeta -1_{\mathrm{o}} \phi _\zeta ) =-\zeta (1_{\mathrm{e}} \phi _\zeta -1_{\mathrm{o}} \phi _\zeta ), \end{aligned}$$

(A.11)

that is, $-\zeta$ is also an eigenvalue. Since $Q_{\varrho _N}$ has $|B(0;\varrho _N)|$ eigenvalues counting multiplicity, the first assertion about the eigenvalues follows.

The second assertion about the eigenfunction is a consequence of the following two facts: $\lambda _{B(0;\varrho _N)}^{\mathrm{RW},(1)}$ is a simple eigenvalue and $Q_{\varrho _N}^2$ leaves $\ell ^2({{\mathbb {Z}}}^d_{\mathrm{e}})$ and $\ell ^2({{\mathbb {Z}}}^d_{\mathrm{o}})$ invariant. $\square$

Proof of Lemma 5.2

Let us start with the case that N is an even integer. In this case, $S_N\in {{\mathbb {Z}}}^d_{\mathrm{e}}$ and hence

$$\begin{aligned} \begin{aligned}&{{\mathbb {P}}}\otimes \varvec{\mathrm {P}}\left( \tau _\mathcal{O}>N\right) \\&\quad \ge {{\mathbb {P}}}(\mathcal{O}\cap B(0;\varrho _N)=\emptyset )\varvec{\mathrm {P}}\left( \tau _{B(0;\varrho _N)^c}>N, S_N\in {{\mathbb {Z}}}^d_{\mathrm{e}}\right) \\&\quad =p^{|B(0;\varrho _N)|}Q_{\varrho _N}^{N/2}1_{\mathrm{e}}(0). \end{aligned} \end{aligned}$$

(A.12)

Let us denote by P the orthogonal projection onto the first eigenspace of $Q_{\varrho _N}^2$. Then

$$\begin{aligned} Q_{\varrho _N}^{N/2}1_{\mathrm{e}}(0) =\left( 1-\lambda _{B(0;\varrho _N)}^{\mathrm{RW},(1)}\right) ^NP1_{\mathrm{e}}(0) +Q_{\varrho _N}^{N/2}(\mathrm{id}-P)1_{\mathrm{e}}(0). \end{aligned}$$

(A.13)

Using $1-\lambda \ge \exp \{-\lambda -\lambda ^2\}$ for small $\lambda >0$, $\phi ^{\mathrm{RW},(1)}_{B(0;\varrho _N)}\ge 0$, (A.2) and Lemma A.3, we can bound the first term below by

$$\begin{aligned} \begin{aligned}&\left( 1-\lambda ^{\mathrm{RW},(1)}_{B(0;\varrho _N)}\right) ^{N} \left\langle \phi ^{\mathrm{RW},(1)}_{B(0;\varrho _N)}, 1_{\mathrm{e}}\right\rangle \phi ^{\mathrm{RW},(1)}_{B(0;\varrho _N)}(0)\\&\quad \ge \left( 1-\lambda ^{\mathrm{RW},(1)}_{B(0;\varrho _N)}\right) ^N\phi ^{\mathrm{RW},(1)}_{B(0;\varrho _N)}(0)^2\\&\quad \ge \exp \left\{ -N\lambda ^{\mathrm{RW},(1)}_{B(0;\varrho _N)}-cN\varrho _N^{-3}\right\} . \end{aligned} \end{aligned}$$

(A.14)

On the other hand, it also follows from Lemma A.3 that the operator norm of $Q_{\varrho _N}^{N} (\mathrm{id}-P)$ is bounded by $(1-\lambda _{B(0;\varrho _N)}^{\mathrm{RW},(2)})^N$. Combining this with (EV) and $1-\lambda \le \exp \{-\lambda \}$, we can bound the second term in (A.13) by

$$\begin{aligned} \begin{aligned} \left| Q_{\varrho _N}^{N}(\mathrm{id}-P)1_{\mathrm{e}}(0)\right|&\le \left( 1-\lambda _{B(0;\varrho _N)}^{\mathrm{RW},(2)}\right) ^N\Vert 1_{\mathrm{e}}\Vert _{\ell ^2(B(0;\varrho _N))}\\&\le \exp \left\{ -N\lambda ^{\mathrm{RW},(1)}_{B(0;\varrho _N)}-cN\varrho _N^{-2}\right\} . \end{aligned} \end{aligned}$$

(A.15)

Since (A.15) is negligible compared with (A.14) for large N, we obtain

$$\begin{aligned} {{\mathbb {P}}}\otimes \varvec{\mathrm {P}}\left( \tau _\mathcal{O}>N\right) \ge \exp \left\{ |B(0;\varrho _N)|\log (1/p)-N\lambda ^{\mathrm{RW},(1)}_{B(0;\varrho _N)}-cN\varrho _N^{-3}\right\} . \end{aligned}$$

(A.16)

Substituting the bound $\left| |B(0;\varrho _N)|-\mathrm{vol}(B(0;\varrho _N))\right| \le c\varrho _N^{d-1}$ and (A.1) into the above, we arrive at the desired bound.

Finally when N is an odd integer, we start with

$$\begin{aligned} \begin{aligned}&{{\mathbb {P}}}(\mathcal{O}\cap B(0;\varrho _N)=\emptyset )\varvec{\mathrm {P}}\left( \tau _{B(0;\varrho _N)^c}>N\right) \\&\quad =\sum _{|y|=1}{{\mathbb {P}}}(\mathcal{O}\cap B(0;\varrho _N)=\emptyset )\varvec{\mathrm {P}}_y\left( \tau _{B(0;\varrho _N)^c}>N-1\right) . \end{aligned} \end{aligned}$$

(A.17)

Then the rest of the argument is the same as before. We have the sum of $\frac{1}{2d}\phi ^{\mathrm{RW},(1)}_{B(0;\varrho _N)}(y)$ over $\{|y|=1\}$ instead of $\phi ^{\mathrm{RW},(1)}_{B(0;\varrho _N)}(0)$ in (A.14), but (A.2) gives us the same lower bound. $\square$

On the proof of Theorem A

In this section, we briefly explain how to prove Theorem A by adapting the argument in [19]. Roughly speaking, it consists of the following five steps:

• 1. use the method of enlargement of obstacles in [21] to define a clearing set$\mathscr {U}_{\mathrm{cl}}$,

• 2. prove volume and eigenvalue constraints for $\mathscr {U}_{\mathrm{cl}}$,

• 3. apply a quantitative Faber–Krahn inequality to show that $\mathscr {U}_{\mathrm{cl}}$ is close to a ball with radius $\varrho _N$,

• 4. prove a sharp lower bound on the partition function in terms of a random eigenvalue,

• 5. use the fact that the region outside $\mathscr {U}_{\mathrm{cl}}$ has much larger eigenvalue to deduce that it is too costly for the random walk to get away from the ball.

In what follows, we explain these steps in more detail using the same parameters as in [19] as much as possible. It turns out that it is only Step 3 that requires an extra argument in the discrete setting.

Steps 1 and 2 can be carried out exactly as in [19] since the method of enlargement of obstacles used in that paper has been translated to the discrete setting in [3]. This method allows us to define the clearing set $\mathscr {U}_{\mathrm{cl}}$ as a union of large boxes (lattice animal) that are almost free of obstacles (cf. [19, (51)–(52)]). Since $\mathscr {U}_{\mathrm{cl}}^c$ has rather high density of obstacles, we can effectively discard it when we consider the eigenvalue (cf. [19, (23), (55)]):

$$\begin{aligned} \left| \lambda ^{\mathrm{RW}, (1)}_{\mathscr {U}_{\mathrm{cl}}}-\lambda ^{\mathrm{RW}, (1)}_{B(0;2N){\setminus }\mathcal{O}}\right| \le \varrho _N^{-\rho } \end{aligned}$$

(B.1)

for some $\rho >0$. Combining the above two properties, in the same way as [19, Proposition 1], we can prove that for some $\alpha _1>0$, the $\mu _N$-probability of

$$\begin{aligned} |\mathscr {U}_{\mathrm{cl}}|\log \tfrac{1}{p}+N\lambda ^{\mathrm{RW},(1)}_{\mathscr {U}_{\mathrm{cl}}}\le N^{\frac{d}{d+2}}\left( c(d,p)+N^{-\frac{\alpha _1}{d+2}}\right) \end{aligned}$$

(B.2)

tends to one as $N\rightarrow \infty$.

As for Step 3, if $\mathscr {U}_{\mathrm{cl}}$ were a subset of ${{\mathbb {R}}}^d$, then the quantitative Faber–Krahn inequality [19, Theorem A] would imply that $\mathscr {U}_{\mathrm{cl}}$ satisfying (B.2) must be close to a ball with radius $\varrho _N$ in the symmetric difference. But we are in the discrete setting and hence we need to find a set in ${{\mathbb {R}}}^d$ whose volume and (continuum) eigenvalue are almost the same as $|\mathscr {U}_{\mathrm{cl}}|$ and $\lambda ^{\mathrm{RW},(1)}_{\mathscr {U}_{\mathrm{cl}}}$, respectively. By the results in [22], the set $\mathscr {U}_{\mathrm{cl}}^+=\left\{ x\in {{\mathbb {R}}}^d:\mathrm{dist}_{\ell ^\infty }(x,\mathscr {U}_{\mathrm{cl}})\le 2\right\}$ has the eigenvalue $\lambda ^{(1)}_{\mathscr {U}_{\mathrm{cl}}^+}$ almost the same as $\lambda ^{\mathrm{RW},(1)}_{\mathscr {U}_{\mathrm{cl}}}$. Also, since $\mathscr {U}_{\mathrm{cl}}$ is a union of large boxes, the volume of $\mathscr {U}_{\mathrm{cl}}^+$ is close to $|\mathscr {U}_{\mathrm{cl}}|$. In this way, we can conclude that there exists a ball $B({\fancyscript{x}}_N;\varrho _N)$ that almost coincides with $\mathscr {U}_{\mathrm{cl}}$. The outside of this ball has rather high density of obstacles and hence just as in [19, Lemma 1], we have

$$\begin{aligned} \lambda ^{\mathrm{RW}, (1)}_{B(0;2N){\setminus }B({\fancyscript{x}}_N;\varrho _N)} \ge \varrho _N^{-2+\alpha _3} \end{aligned}$$

(B.3)

for some $\alpha _3>0$.

Step 4 relies on a simple functional analytic argument and there is no difficulty in adapting it to the discrete setting. It corresponds to [19, Lemma 2] and provides the following lower bound on the partition function:

$$\begin{aligned} {{\mathbb {P}}}\otimes \varvec{\mathrm {P}}\left( \tau _\mathcal{O}>N\right) \ge N^{-c}{{\mathbb {E}}}\left[ \exp \left\{ -N\lambda ^{\mathrm{RW}, (1)}_{B(0;2N){\setminus }\mathcal{O}}\right\} \right] . \end{aligned}$$

(B.4)

See also [15, Lemma 2] for a slightly simplified argument.

Finally, Step 5 roughly goes as follows. For $0\le k< l\le N$, consider the event that $S_k$ is away from the confinement ball $B({\fancyscript{x}}_N;\varrho _N)$ and returns to it at time l for the first time after k. In this situation, the survival probability between k and l decays like $\exp \{-(l-k)\lambda ^{\mathrm{RW}, (1)}_{B(0;2N){\setminus }B({\fancyscript{x}}_N;\varrho _N)}\}$ and hence using Steps 3 and 4, we obtain

$$\begin{aligned} \begin{aligned}&\mu _N\left( S_k\not \in B({\fancyscript{x}}_N;\varrho _N+\varrho _N^{\epsilon _1}), k+\tau _{B({\fancyscript{x}}_N;\varrho _N)}\circ \theta _k=l\right) \\&\quad \lesssim N^c \frac{{{\mathbb {E}}}\left[ \exp \left\{ -(N-l+k)\lambda ^{\mathrm{RW}, (1)}_{B(0;2N){\setminus }\mathcal{O}}+(l-k)\varrho _N^{-2+\alpha _3}\right\} \right] }{{{\mathbb {E}}}\left[ \exp \left\{ -N\lambda ^{\mathrm{RW}, (1)}_{B(0;2N){\setminus }\mathcal{O}}\right\} \right] }. \end{aligned} \end{aligned}$$

(B.5)

Note that we may impose $\lambda ^{\mathrm{RW}, (1)}_{B(0;2N){\setminus }\mathcal{O}}\le 2c(d,p)\varrho _N^{-2}$ both in the numerator and denominator, in view of (1.5). Now if $l-k\ge \varrho _N^{-2+2\alpha _3}$, then the term $(l-k)\varrho _N^{-2+\alpha _3}$ in the numerator causes a large additional cost and hence the right-hand side decays stretched exponentially in N. If $l-k< \varrho _N^{-2+2\alpha _3}$, then we choose $\epsilon _1>1-\alpha _3$ (so that $|S_l-S_k|\gg (l-k)^{1/2}$) and use the Gaussian heat kernel bound for the random walk, instead of the eigenvalue bound, to derive a stretched exponential decay. Summing over k and l, we conclude that the random walk does not make a crossing from $B({\fancyscript{x}}_N;\varrho _N+\varrho _N^{\epsilon _1})^c$ to $B({\fancyscript{x}}_N;\varrho _N)$. The case that the random walk does not return to $B({\fancyscript{x}}_N;\varrho _N)$ after visiting $B({\fancyscript{x}}_N;\varrho _N+\varrho _N^{\epsilon _1})^c$ but this can be dealt with in a similar way, by changing l to the last visit to $B({\fancyscript{x}}_N;\varrho _N)$ before k.

Index of notation

$\tau _A$ :

(1.1)

$\mu _N$ :

(1.2)

c(d, p):

(1.5)

$\varrho _N$ :

(1.6)

$\fancyscript{x}_N$ :

Theorem A

$B(x;R)$ :

Theorem A

$\partial A$ :

(1.10)

$|A|, \mathrm{vol}(A)$ :

End of Sect. 1

$E_l^\delta$ :

(2.2)

A(x; r, R):

(2.12)

$\mathcal{T}$ :

Definition 2.3

$\mathcal{X}_l$ :

(3.13) and (3.14)

$p^D_n(u,v)$ :

(3.16)

$\Gamma (k)$ :

(3.17)

$E_l^{\delta ,\rho }$ :

(3.18)

$\mathcal{X}_{l}^{\circ }$ :

(3.62)

$G_{\mathcal{O}}$ :

(4.8)

$\mathcal{L}(l$):

(4.9)

$\lambda ^{\mathrm{RW},(k)}_{A}$ and $\phi ^{\mathrm{RW},(k)}_{A}$:

(4.30)

$\lambda _{A}^{(k)}$ and $\phi _{A}^{(k)}$:

Below (5.1)