Abstract
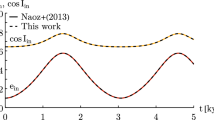
In the mathematical framework of a restricted, slightly dissipative spin–orbit model, we prove the existence of periodic orbits for astronomical parameter values corresponding to all satellites of the Solar System observed in exact spin–orbit resonance.

Similar content being viewed by others
Notes
The largest relative inclination (of the spin axis to the orbital plane) is that of Iapetus (\(8.298^{\circ }\)) followed by Mercury (\(7^{\circ }\)), Moon (\(5.145^{\circ }\)), and Miranda (\(4.338^{\circ }\)); all the other moons have inclination on the order of \(1^{\circ }\) or less.
The analytic expression of the true anomaly in terms of the eccentric anomaly is given by \(\mathrm{f}_\mathbf{e}(t)= 2 \arctan \left( \sqrt{\frac{1+\mathbf{e}}{1-\mathbf{e}}} \tan \left( \frac{u_\mathbf{e}(t)}{2}\right) \right) \).
As is well known (see Wintner 1941), \(\mathbf{e}\rightarrow u_\mathbf{e}(t)\) is, for every \(t \in \mathbb R\), holomorphic for \(|\mathbf{e}|< r_{\star }\), with \( r_\star := \max \limits _{y \in \mathbb R} \frac{y}{\cosh (y)} = \frac{y_\star }{\cosh (y_\star )} = 0.6627434\ldots \mathrm and y_\star = 1.1996786\ldots \).
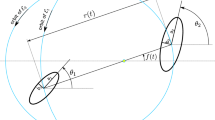
\(a\ge b\) denote the maximal and minimal observed equatorial radii, which, in our model, are assumed to be the axes of the ellipse modeling the equatorial section of the satellite. The dimensions of the polar radius are not relevant in our model; however, for all the cases considered in this paper it turns out to be always smaller than or equal to the smallest equatorial radius.
Of course, in physical space, \(x\) and \(t\), being angles, are defined modulus \(2{\pi }\), but to keep track of the topology (windings and rotations) one needs to consider them in the universal cover \({\mathbb {R}}\) of \({\mathbb {R}}/(2{\pi }{\mathbb {Z}})\).
The procedure consists in reducing the problem to a fixed point problem containing parameters: The question is then solved by a Lyapunov–Schmidt or “range-bifurcation” decomposition. The “range equation” is solved by standard contraction mapping methods, but in order for the fixed point to correspond to a true solution of the original problem, a compatibility (zero-mean) condition has to be satisfied (“the bifurcation equation”), and this is done by exploiting a free parameter by means of a topological argument.
The parameter \({\xi }\) is given by \((1/2{\pi }) \int _0^{2{\pi }} \left( x(qt)- pt\right) \mathrm{d}t\) and will be our “bifurcation parameter.”
\( \Vert v\Vert _{C^k}:= \sup \limits _{0\le j\le k} \sup \limits _{t\in {\mathbb {R}}}|D^j v(t)|\).
\( \Vert {\mathcal G}\Vert _{L({\mathbb {B}},{\mathbb {B}})}=\sup \limits _{u: \Vert u\Vert _{C^0}=1} \Vert {\mathcal G}(u)\Vert _{C^0}\).
It is easy to see that the estimates in Lemma 2.2 are sharp.
We shall choose \(h=4\) for the 1:1 resonances and \(h=21\) for the 3:2 case of Mercury.
Use \( e^{2iz}=\frac{i- w }{w+i}=-\frac{(w-i)^2}{w^2+1}\) and \(\tan ^2 (\alpha /2)= (1-\cos \alpha )/(1+\cos \alpha )\).
The values for \(b\) are rather arbitrary (as long as \(0<b<1\)); our choice is made for optimizing the estimates.
Thus, the inequality is satisfied if the numerical value in the column is positive; the same applies to the fifth column.
A factor \(-1/2\) is missing in the definition of \(G(t)\) given in Biasco and Chierchia (2009), (iii) p. 4366 and, consequently, it has to be included at p. 4367 in line 6 (from above, counting also lines with formulas) in front of “Re”; in line 12, 17, and 18 the factor \(1/(2{\pi })\) has to be replaced by \(-1/(4{\pi })\).
For pictures, see: http://photojournal.jpl.nasa.gov/catalog/PIA10369 (Phobos), http://photojournal.jpl.nasa.gov/catalog/PIA11826 (Deimos), http://photojournal.jpl.nasa.gov/catalog/PIA02532 (Amalthea), http://photojournal.jpl.nasa.gov/catalog/PIA12714 (Janus), http://photojournal.jpl.nasa.gov/catalog/PIA12700 (Epimetheus).
Positive values in the third and fourth column and values less than 0.008 in the fifth column imply that the assumptions of Proposition 1 hold.
References
Bambusi, D., Haus, E.: Asymptotic stability of synchronous orbits for a gravitating viscoelastic sphere. Celest. Mech. Dyn. Astron. 114(3), 255–277 (2012)
Biasco, L., Chierchia, L.: Low-order resonances in weakly dissipative spin–orbit models. J. Differ. Equ. 246, 4345–4370 (2009)
Castillo-Rogez, J.C., Efroimsky, M., Lainey, V.: The tidal history of Iapetus: spin dynamics in the light of a refined dissipation mode. J. Geophys. Res. 116, E09008 (2011). doi:10.1029/2010JE003664
Celletti, A.: Analysis of resonances in the spin-orbit problem in celestial mechanics: the synchronous resonance (part I). J. Appl. Math. Phys. 41, 174–204 (1990)
Celletti, A.: Stability and Chaos in Celestial Mechanics. Springer-Praxis, Providence (2010)
Celletti, A., Chierchia, L.: Quasi-periodic attractors in celestial mechanics. Arch. Ration. Mech. Anal. 191(2), 311–345 (2009)
Correia, A.C.M., Laskar, J.: Mercury’s capture into the 3/2 spin–orbit resonance as a result of its chaotic dynamics. Nature 429, 848–850 (2004)
Danby, J.M.A.: Fundamentals of Celestial Mechanics. Macmillan, New York (1962)
Dougherty, M.K., et al. (eds.): Saturn from Cassini–Huygens. Springer, Netherlands (2009). doi:10.1007/978-1-4020-9217-6
Goldreich, P., Peale, S.: Spin–orbit coupling in the solar system. Astron. J. 71, 425 (1967)
Hussmann, H., Sohl, F., Spohn, T.: Subsurface oceans and deep interiors of medium-sized outer planet satellites and large trans-neptunian objects. Icarus 185, 258–273 (2006)
Iess, L., et al.: Gravity field, shape, and moment of inertia of Titan. Science 327, 1367–1369 (2010)
Iess, L., et al.: The tides of Titan. Science 337(6093), 457–459 (2012)
Lainey, V., et al.: Strong tidal dissipation in Saturn and constraints on Enceladus’ thermal state from astrometry. Astrophys. J. 752(1), 14–19 (2012)
MacDonald, G.J.F.: Tidal friction. Rev. Geophys. 2, 467–541 (1964)
Peale, S.J.: The free precession and libration of Mercury. Icarus 178, 4–18 (2005)
Porco, C.C., et al.: Saturn’s small inner satellites: clues to their origins. Science 318, 1602–1607 (2007)
Runcorn, S.K., Hofmann, S.: The Moon. In: Proceedings from IAU Symposium no. 47. Reidel, Dordrecht (1972)
Sicardy, B., et al.: Charon’s size and an upper limit on its atmosphere from a stellar occultation. Nature 439, 52–54 (2006)
Thomas, P.C.: Radii, shapes, and topography of the satellites of Uranus from limb coordinates. Icarus 73, 427–441 (1988)
Thomas, P.C.: The shapes of small satellites. Icarus 77, 248–274 (1989)
Thomas, P.C., et al.: The small inner satellites of Jupiter. Icarus 135, 360–371 (1998)
Wintner, A.: The Analytic Foundations of Celestial Mechanics. Princeton University Press, Princeton (1941)
Wisdom, J.: Rotational dynamics of irregularly shaped natural satellites. Astron. J. 94(5), 1350–1360 (1987)
Acknowledgments
We thank J. Castillo-Rogez, A. Celletti, M. Efroimsky, and F. Nimmo for useful discussions. Partially supported by the MIUR grant “Critical Point Theory and Perturbative Methods for Nonlinear Differential Equations” (PRIN2009).
Author information
Authors and Affiliations
Corresponding author
Additional information
Communicated by Amadeu Delshams.
Appendices
Appendix 1: Proof of Lemma 2.2
Proof
We first prove (14). Up to a rescaling we can prove (14) assuming \(\Vert v'\Vert _{C^0}=1\). Assume by contradiction that
Note that it is obvious that \(c\le \pi \), since v has zero average and, therefore, must vanish at some point. Since |v| is a continuous periodic function it attains a maximum at some point; up to a translation we can assume that |v| attains its maximum in \(-c\). In that case, multiplying by \(-1\), we can also assume that \(-c\) is a minimum, namely
Since \(\Vert v'\Vert _{C^0}=1\), we get
and, therefore,
Since \(\Vert v'\Vert _{C^0}=1\), we also get
Then,
Combining with the last inequality in (46), we get
which contradicts the fact that \(v\) has zero average, proving (14).
We now prove (15). Up to a rescaling we can prove (15) assuming \(\Vert v''\Vert _{C^0}=1\). Assume by contradiction that
Up to a translation we can assume that \(|v|\) attains maximum at \(0\). In that case, multiplying by \(-1\), we can also assume that \(-c\) is a minimum, namely
Since \(\Vert v''\Vert _{C^0}=1\), we get
Since v has zero average must exist \(t_1 < 0 < t_2\)
Moreover,
Since \(v\) has zero average and is \(2\pi \)-periodic,
Set
and note that
Note that \(u\in \mathbb B\cap C^2\) and, by (48),
Consider now the even function
Note that \(w\in \mathbb B\cap C^2\) and
Set
We claim that
Then,
which is a contradiction.
Let us prove the claim in (52). Note that \(z(-a)=w(-a)=0\). Assume by contradiction that there exists \( \bar{t}\in [-a,0)\) such that
Then, since \(\Vert w''\Vert _{C^0}\le 1\),
Then,
which contradicts the first inequality in (51). This completes the proof of (15). \(\square \)
Appendix 2: Fourier Coefficients of the Newtonian Potential
Properties of the Fourier coefficients \(\alpha _j\) of the Newtonian potential \(f\), including Eq. (32), have been discussed, e.g., in Appendix 1 ofFootnote 17 Biasco and Chierchia (2009).
Here we provide a simple formula for the Fourier coefficients \(\alpha _j\) of the Newtonian potential \(f\) in (3) [compare (d) of §1, and (31)–(32)]; namely we prove that
where \(w=w(u;\mathbf{e}) :=\sqrt{\frac{1+\mathbf{e}}{1-\mathbf{e}}} \tan \frac{u}{2}\), \(\rho =1-\mathbf{e}\cos u\), and
Proof
If \(z=\arctan w \), then
so that if \(w_\mathbf{e}(t):=w(u_{\mathbf{e}}(t),\mathbf{e})\) one has \( f_\mathbf{e}= 2 \arctan w_\mathbf{e}\) and
By parity properties, it is easy to see that the \(G_j\)’s are real, namely \(G_j=\bar{G}_j\), so that
Making the change of variable given by the Kepler equation (5), i.e., integrating from \(t\) to \(u=u_\mathbf{e}\) and setting \(u_\mathbf{e}(t)' = \frac{1}{\rho _\mathbf{e}(t)}\), one gets (53). \(\square \)
Appendix 3: Small Bodies
In the Solar System, besides the 18 moons listed in Table 1 and Mercury, there are five other minor bodies with mean radius smaller than 100 km observed in 1:1 spin–orbit resonance around their planet: Phobos and Deimos (Mars), Amalthea (Jupiter), and Janus and Epimetheus (Saturn), as listed in Table 4.
Besides being small, such bodies have also a quite irregular shape and only Janus and Epimetheus have good equatorial symmetry.Footnote 18 Indeed, for these two small moons (and only for them among the minor bodies), our theorem holds as shown by the data reported in Table 5.Footnote 19
Rights and permissions
About this article
Cite this article
Antognini, F., Biasco, L. & Chierchia, L. The Spin–Orbit Resonances of the Solar System: A Mathematical Treatment Matching Physical Data. J Nonlinear Sci 24, 473–492 (2014). https://doi.org/10.1007/s00332-014-9196-7
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00332-014-9196-7
Keywords
- Periodic orbits
- Celestial mechanics
- Spin–orbit resonances
- Moons in the Solar System
- Mercury
- Dissipative systems