Abstract
In production economies with incomplete markets, shareholders disagree about optimal production plans, and there is no natural objective of the firm. From a normative perspective, the firm should choose plans that lead to a constrained Pareto efficient allocation. From a positive perspective, all decisions of the firm should be supported by a majority of shareholders. This paper asks whether one can design objectives for the firm that meet both normative and positive criteria. The answer is negative: Constrained efficient production plans would generically be turned down by a majority of shareholders. This finding is related to the generic nonexistence of Makowski equilibria.
Similar content being viewed by others
Notes
Throughout generic shall mean: for an open, dense set of economies whose complement has Lebesgue measure zero.
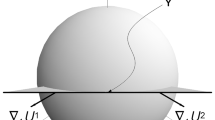
Note that \(\text {dim}({\mathcal {Y}}^k)=|\varvec{{\varOmega }}|+1\), i.e., the smallest linear subspace containing \({\mathcal {Y}}^k\) is the full consumption space. One could relax this assumption to \(\text {dim}({\mathcal {Y}}^k)>2\), but Jacobians would need to be replaced by generalized, set-valued derivatives. This would complicate the notation and inflate most proofs without adding much insight.
As pointed out by Dierker and Dierker (2012), this creates a conflict between initial and final shareholders. If the firm acts in the interest of initial shareholders, it will increase their rent by charging prices above marginal costs. On the other hand, if the firm acts in the interest of final shareholders, it must extract the rent by enforcing prices below marginal costs.
Known nonexistence problems include the production analogue of the Hart (1975) problem, a discontinuity of demand when the rank of Y drops, as well as the Momi (2001) problem, a discrete change of the Drèze objective function at a rank drop when consumers with negative shareholdings receive zero weight.
The same holds true for weaker efficiency criteria such as minimal efficiency, which allows the planner to make pre-market choices of production plans and post-market compensation payments of date 0 consumption only; see Dierker et al. (2005).
A proof can be found in Magill and Quinzii (1996), p. 87, Theorem 10.5.
A proof can be found in Magill and Quinzii (1996), p. 104, Theorem 12.3.
References
Bisin, A., Gottardi, P., Ruta, G.: Equilibrium corporate finance and intermediation. NBER working paper, 20345 (2014)
DeMarzo, P.M.: Majority voting and corporate control: the rule of the dominant shareholder. Rev. Econ. Stud. 60, 713–734 (1993)
Diamond, P.A.: The role of a stock market in a general equilibrium model with technological uncertainty. Am. Econ. Rev. 57, 759–776 (1967)
Dierker, E., Dierker, H.: Ownership structure and control in incomplete market economies with transferable utility. Econ. Theory 51, 713–728 (2012)
Dierker, E., Dierker, H., Grodal, B.: Nonexistence of constrained efficient equilibria when markets are incomplete. Econometrica 70, 1245–1251 (2002)
Dierker, E., Dierker, H., Grodal, B.: Are incomplete markets able to achieve minimal efficiency? Econ. Theory 25, 75–87 (2005)
Drèze, J.: Investment under private ownership: optimality, equilibrium and stability. In: Drèze, J. (ed.) Allocation Under Uncertainty, Equilibrium and Optimality, pp. 129–165. Wiley, New York (1974)
Drèze, J.: (Uncertainty and) the firm in general equilibrium theory. Econ. J. 95, 1–20 (1985)
Ekern, S., Wilson, R.: On the theory of the firm in an economy with incomplete markets. Bell J. Econ. Manag. Sci. 5, 171–180 (1974)
Gevers, L.: Competitive equilibrium of the stock exchange and Pareto efficiency. In: Drèze, J. (ed.) Allocation Under Uncertainty, Equilibrium and Optimality, pp. 167–191. Wiley, New York (1974)
Guillemin, V., Pollack, A.: Differential Topology. Prentice-Hall, Englewood Cliffs (1974)
Hart, O.D.: On the optimality of equilibrium when the market structure is incomplete. J. Econ. Theory 11, 418–443 (1975)
Hirsch, M.W.: Differential Topology, 5th edn. Springer, Berlin (1994)
Magill, M., Quinzii, M.: Theory of Incomplete Markets. MIT Press, Cambridge (1996)
Makowski, L.: A characterization of perfectly competitive economies with production. J. Econ. Theory 22, 208–221 (1980)
Makowski, L.: Competition and unanimity revisited. Am. Econ. Rev. 73, 329–339 (1983)
Makowski, L.: Competitive stock markets. Rev. Econ. Stud. 20, 305–330 (1983)
Makowski, L., Pepall, L.: Easy proofs of unanimity and optimality without spanning: a pedagogical note. J. Finance 40, 1245–1250 (1985)
Momi, T.: Non-existence of equilibrium in an incomplete stock market economy. J. Math. Econ. 35, 41–70 (2001)
Ostroy, J.M.: The no-surplus condition as a characterization of perfectly competitive equilibrium. J. Econ. Theory 22, 183–207 (1980)
Radner, R.: A note on unanimity of stockholders’ preferences among alternative production plans: a reformulation of the Ekern–Wilson model. Bell J. Econ. Manag. Sci. 5, 181–184 (1974)
Villanacci, A., Carosi, L., Benevieri, P., Battinelli, A.: Differential Topology and General Equilibrium with Complete and Incomplete Markets. Kluwer Academic Publishers, Boston (2002)
Author information
Authors and Affiliations
Corresponding author
Additional information
This paper has benefited from the valuable comments of Egbert Dierker, Herakles Polemarchakis, Julia Reynolds, Klaus Ritzberger, Jan Werner, two anonymous referees, and seminar participants at Humboldt University and the University of Innsbruck. Large parts of this research were funded by the Austrian Science Fund (FWF) under project I 1242-G16.
Appendix
Appendix
The appendix contains detailed proofs. It starts with a brief formal discussion of exchange equilibria. Fix the subset of parameters \(({\mathcal {Y}},U,\delta ,X_{\varvec{1}})\) and consider variations of (e, Y). Let \(\phi ^{i*}(e,Y,p)\) and \(\psi ^{i*}(e,Y,p)\) be consumer i’s demand functions for assets and shares respectively with the usual notation \(\bar{\phi }^*\) and \(\bar{\psi }^*\) for aggregate demand. Denote by \(\partial {\mathcal {Y}}^k\) the smooth boundary of \({\mathcal {Y}}^k\). The following lemma shows that for each firm k, the social planner would only choose a production plan \(Y^k \in \partial {\mathcal {Y}}^k\).
Lemma 1
In any constrained efficient plan \((Y,c,\phi ,\psi )\), for each firm k, \(Y^k \in \partial {\mathcal {Y}}^k\).
Proof
Suppose not. Then \(Y^k\) would lie in the interior of \({\mathcal {Y}}^k\) for some firm k. As a consequence, this firm could realize an alternative plan \(\hat{Y}^k\) with identical costs \(\hat{Y}_0^k = Y_0^k\) but proportionally greater output \(\hat{Y}_{\varvec{1}}^k = \beta Y_{\varvec{1}}^k\) for some scalar \(\beta > 1\). Note that the asset span does not change if the alternative plan is realized. If the planner implements \(\hat{Y}^k\) and simultaneously subtracts \(\psi _k^i(1-\frac{1}{\beta })\) from the shareholdings of each consumer i (for short sellers this means an addition), then everybody consumes the same amounts as under the original plan. However, the number of shares outstanding after the portfolio readjustments would be
and therefore a number \((1-\frac{1}{\beta })\) of unassigned shares could be distributed to the consumers. Each consumer who receives such shares is strictly better off than under the original plan. Thus, the original plan cannot be constrained efficient. \(\square \)
As a consequence of the lemma, the further analysis can be restricted to the smooth manifold  . Denote by \({\varPi } \subset {\mathbb {R}}^{J+K}_{++}\) the set of possible equilibrium prices, which is under Assumptions 1, 2, and 4 open and bounded. Define the mapping \(F:{\mathbb {E}}\times \partial {\mathcal {Y}}\times {\varPi } \rightarrow {\mathbb {R}}^{J+K}\) as
. Denote by \({\varPi } \subset {\mathbb {R}}^{J+K}_{++}\) the set of possible equilibrium prices, which is under Assumptions 1, 2, and 4 open and bounded. Define the mapping \(F:{\mathbb {E}}\times \partial {\mathcal {Y}}\times {\varPi } \rightarrow {\mathbb {R}}^{J+K}\) as

An exchange equilibrium is any vector \(p \in {\mathbb {R}}^{J+K}\) that solves the equation \(F(e,Y,p)=0\). Such a solution vector exists for any choice of (e, Y).Footnote 8 However, all types of production equilibria based on firm value maximization (Drèze, Makowski, agent dictatorship) fail to exist at points in the space of economies at which the rank of Y drops below K. To study production equilibria, it therefore makes sense to restrict attention to \(\tilde{{\mathcal {Y}}} = \left\{ Y \in {\mathcal {Y}}\,\left| \;\text {rank}\left( Y\right) =K\right. \right\} \), which is an open, dense subset of \({\mathcal {Y}}\), whose complement has zero measure. Denote by \(\tilde{F}\) the domain restriction of F to \({\mathbb {E}}\times \partial \tilde{{\mathcal {Y}}}\times {\varPi }\). It should be easy to see that \(D\tilde{F}\) has full rank: One can perturb the aggregate demand for any asset or share simply by decreasing (increasing) \(e^i_{\varvec{1}}\) proportionally to its payoff and increasing (decreasing) \(e^i_0\) by its price for some consumer i. It follows directly from the regular value theorem (see Villanacci et al. (2002), p. 84, Theorem 9) that \({\varXi } = \tilde{F}^{-1}(0)\) is a smooth submanifold of \({\mathbb {E}}\times \partial \tilde{{\mathcal {Y}}}\times {\varPi }\). It shall be referred to as the exchange equilibrium manifold.
Proof of Proposition 1
The two set inclusions are proven separately.
Proof of \({\mathcal {E}} \subseteq {\mathcal {D}}\): The tools of the constrained efficient planner in a production economy are the choice of Y as well as redistribution of \((e_0,\delta )\) before the market opens and redistribution of \((c_0,\phi ,\psi )\) after the market has closed. However, it is a well-known result that in two-date finance economies the planner need not make any redistribution after the market has closed: The first-order conditions for \((c,\phi ,\psi )\) of the consumers in an exchange equilibrium imply the first-order conditions of the constrained efficient planner in an exchange economy. Since the constrained feasible set in an exchange economy is convex, these conditions are necessary and sufficient for optimality.Footnote 9 Furthermore, pre-market transfers of \(\delta \) are redundant: They affect only date 0 budget constraints, which can equivalently achieved by transfers of date 0 endowment \(e_0\).
Therefore, the problem of the constrained efficient planner can be viewed as choosing points \(\xi = (\hat{e},\hat{Y},\hat{p})\) on the exchange equilibrium manifold. He must, however, satisfy the restrictions on endowment changes from Definition 3 for some given endowment vector e. Let \(U = (U^i)_{i=1}^I\) be the vector valued utility mapping and let \(c^*:{\varXi }\rightarrow {\mathbb {R}}^{I(|\varvec{{\varOmega }}|+1)}_{++}\) represent equilibrium consumption. Each constrained efficient equilibrium is a solution to the Pareto problem
for some nonzero vector of multipliers \(\alpha \in {\mathbb {R}}^I_+\). Problem (5) is defined in the Euclidean ambient space of \({\mathbb {E}}\times \partial {\mathcal {Y}}\times {\varPi }\). The choice set is extended to the closure of the exchange equilibrium manifold \(\text {cl}({\varXi })\) in that space. By Assumptions 1, 2, and 4, the relevant subset of \({\mathbb {Y}}\) as well as the sets \({\mathbb {E}}\) and \({\varPi }\) are bounded. Therefore, \(\text {cl}({\varXi })\) is compact and the existence of a solution \(\xi ^*\) to (5) is guaranteed. If \(\xi ^* \in \text {cl}({\varXi })\backslash {\varXi }\), the definition of exchange equilibrium is violated. In that case, \({\mathcal {E}}\) is empty and \({\mathcal {E}} \subseteq {\mathcal {D}}\) holds trivially. Thus, in the remainder of the proof one can concentrate on the case \(\xi ^* \in {\varXi }\).
In order to formulate a Lagrangian for (5), the inclusion \(\xi \in {\varXi }\) must be expressed in terms of equality constraints. The constraints on \(\hat{e}_0\) and \(\hat{e}_{\varvec{1}}\) are already given above. Let \(\lambda \in {\mathbb {R}}_+\) and \(\kappa \in {\mathbb {R}}^{I|\varvec{{\varOmega }}|}_+\) be the associated Lagrange multipliers. The constraint on \(\hat{Y}\) is \(P(\hat{Y})=0\) in which \(P(\hat{Y})=(P^1(\hat{Y}^1),\dots ,P^K(\hat{Y}^K))\). Let \(\mu \in {\mathbb {R}}^K_+\) be the associated vector of Lagrange multipliers. For the constraint on p, note that \({\varXi }\) is a smooth manifold of \({\mathbb {E}}\times \partial {\mathcal {Y}}\times {\varPi }\). Therefore, there exists a local parameterization \(f_\xi \) around every \(\xi \). One would like to have a function \(p^*:{\mathbb {E}}\times \partial {\mathcal {Y}} \rightarrow {\varPi }\) that maps parameters \((\hat{e},\hat{Y})\) in a neighborhood of the optimum \(\xi ^*\) to corresponding equilibrium prices. The local parameterization around \(\xi ^*\) can be used to specify such a function. It is implicitly defined by \((\hat{e},\hat{Y},p^*(\hat{e},\hat{Y})) \in \text {Im}(f_{\xi ^*})\). The resulting constraint is \(\hat{p} = p^*(\hat{e},\hat{Y})\). Let \(\nu \in {\mathbb {R}}_+\) be the associated Lagrange multiplier.
is the Lagrangian for problem (5) with the system of first-order conditions
in which \(Dc^*\) can be expanded by means of equation (2):
Observe that hats are used to highlight vectors and matrices that contain choice variables of the current problem, e.g., \(\hat{X}\) which contains \(\hat{p}_X\). The equation system can be simplified in two steps. First, \(\phi ^{i*}\) and \(\psi ^{i*}\) are maximizers of problem (1). It follows from the envelope theorem that \(DU\cdot \hat{X}\cdot D\phi ^* = 0\) and \(DU\cdot (\hat{Y}-\hat{Z})\cdot D\psi ^* = 0\). Second, the constraints associated with \(\kappa \) and \(\nu \) define \(\hat{e}_{\varvec{1}}\) and \(\hat{p}\) and eliminate those variables de facto as maximizers. One can substitute the constraints into (8) and remove all first-order conditions with respect to those variables. After some rearrangement the simplified system of first-order conditions assumes the form

in which \(I^{|\varvec{{\varOmega }}|}\) represents the \(|\varvec{{\varOmega }}|\)-dimensional identity matrix. The equation system is homogeneous of degree 1 in the multipliers \((\alpha ,\lambda ,\mu )\), and thus it can be normalized such that \(\lambda = 1\). One can conjecture that \(\alpha ^i = 1/D_{c_0^i}U^i\;\forall i\), which makes the left summations disappear both in (9) and (10) when \(\bar{\delta } = 1\), since aggregate excess demand is zero by the market clearing conditions. In the case of a partnership, \(\bar{\delta } = 0\) and \(p_Y^* = -Y_0\) such that the summation in (9) disappears because \(D_{e_0^i}p_Y^* = 0\). Additionally, the summation in (10) simpifies to the row vector \((1,0,\dots ,0)\). Either way, (9) reduces to \(1 = 1\) and is therefore indeed satisfied. Summing up (10), one derives the reduced first-order conditions at the optimum \(\xi ^*\) in the form
Note that since \({\varXi }\) is not convex, (11) is a necessary but not a sufficient condition for optimality. It will also hold for suboptimal values \(e_0 \ne e_0^*\). Finally, observe that the first-order conditions of firms in Drèze equilibria, which are necessary and sufficient for optimality given any \(e \in {\mathbb {E}}\), are
Since \(e^* \in {\mathbb {E}}\), (11) implies (12). This proves the first inclusion.
Proof of \({\mathcal {M}} \subseteq {\mathcal {E}}\): Suppose the plan \((Y,c,\phi ,\psi )\) induced by a Makowski equilibrium were constrained inefficient. Then, there would be an alternative constrained feasible plan \((\hat{Y},\hat{c},\hat{\phi },\hat{\psi })\) such that \(U^i(\hat{c}^i) \ge U^i(c^i)\) with strict inequality for at least one consumer i, which implies
By a well-known argument (see for example Magill and Quinzii (1996), p. 359, Proposition 31.2), the above inequality implies
which can be expanded to
It should be noted that \(\nabla U^i\cdot X = 0\;\forall i\) by the first-order conditions of an exchange equilibrium, which eliminates the second summand. Moreover, note that the date 0 component of \(\nabla U^i\) is 1 for all consumers. Therefore, the first condition in Definition 3 guarantees that the first summand is the same on both sides of the inequality, while the fourth condition implies that the fourth summand is zero. As a consequence, the inequality can only hold if
Let \(m(k) \in \hbox {arg max}_i \nabla U^i\cdot Y^k\). Note that the objective of the firm from Definition 7 implies
Combining (13) and (14) implies that a Makowski equilibrium can only be constrained inefficient if
However, the first-order conditions of an exchange equilibrium imply that \(\nabla U^{m(k)}\cdot Y^k = \nabla U^i\cdot Y^k\;\forall i\,\forall k\). Thus, the inequality could only hold if the fourth condition in Definition 3 were violated – if a plan is Pareto superior to a Makowski equilibrium, it cannot be constrained feasible. This proves the second inclusion. \(\square \)
Proof of Proposition 2
The proof makes use of the following theorem.
Theorem 2
(DeMarzo (1993), Theorem 6) At any majority stable equilibrium, for each firm k, there exists some shareholder d(k) such that \(\nabla U^{d(k)} = \mu ^k DP^k\), so that production is optimal with respect to the state prices of shareholder d(k).
The set inclusions are proven one by one.
-
\({\mathcal {S}} \subseteq {\mathcal {A}}\): the first-order conditions for firms in agent dictatorship equilibria, necessary and sufficient for optimality, are \(\nabla U^{d(k)} = \mu ^k DP^k\;\forall k\). If follows from Theorem 2 that these conditions are fulfilled by a majority stable equilibrium for some dictator appointment mapping d.
-
\({\mathcal {U}} \subseteq {\mathcal {S}}\): Definition 8 implies Definition 9 trivially.
-
\({\mathcal {M}} \subseteq {\mathcal {U}}\): since \(\max _{h \in \{1,\ldots ,I\}} \nabla U^h \cdot y \ge \nabla U^i \cdot y \; \forall i\; \forall y \in {\mathcal {Y}}^k\), Definition 7 implies Definition 8.
\(\square \)
Proof of Theorem 1
Under Assumption 1, value maximization with respect to different state price systems leads to different maxima: Let \(q \in {\mathbb {R}}^{|\varvec{{\varOmega }}|+1}\) be some state price vector. Then the first-order condition for value maximization is \(q = \mu ^k DP^k[y]\), which implies that a production choice \(y \in {\mathcal {Y}}^k\) can only maximize value with respect to state price vector q or multiples thereof. Since marginal rates of substitution vectors are normalized state price vectors, distinctness of an objective function implies distinctness of the problem’s solution.
To prove the theorem, it must therefore be shown that in equilibrium generically \(\left( \nabla U^1,\dots ,\nabla U^I\right) \cdot \psi _k \ne \nabla U^i\;\forall k\), i.e., the share-weighted sum of vectors is not equal to a single vector. A sufficient condition is that for some state \(\omega \) the weighted sum of components \(\left( \nabla _\omega U^1,\ldots ,\nabla _\omega U^I\right) \cdot \psi _k = \nabla _\omega U \cdot \psi _k\) is not equal to the single component \(\nabla _\omega U^i\). This requirement can be expressed by means of a mapping \(G:{\mathbb {E}}\times \partial {\mathcal {Y}}\times {\varPi } \rightarrow {\mathbb {R}}^{K}\) defined as
in which d is an arbitrary dictator assignment mapping. It must be shown that \(F^{-1}(0) \cap G^{-1}(0)\) is a closed, zero-measure set. Since Drèze equilibria do not exist at points where the rank of Y drops, attention can be restricted to \(\tilde{{\mathcal {Y}}}\) once again. Denote by \(\tilde{G}\) the domain restriction of G to \({\mathbb {E}}\times \partial \tilde{{\mathcal {Y}}}\times {\varPi }\). The theorem shall hold for arbitrary \(Y \in \tilde{{\mathcal {Y}}}\). Pick one and let \(\tilde{F}_Y:{\mathbb {E}}\times {\mathbb {R}}^{J+K} \rightarrow {\mathbb {R}}^{J+K}\) and \(\tilde{G}_Y:{\mathbb {E}}\times {\mathbb {R}}^{J+K} \rightarrow {\mathbb {R}}^{K}\) be smooth extensions of \(\tilde{F}\) and \(\tilde{G}\) with fixed Y. Define \(H:{\mathbb {E}}\times {\mathbb {R}}^{J+K} \rightarrow {\mathbb {R}}^{J+2K}\) as

Note that \(\psi ^*\) and \(\nabla _\omega U\) can be perturbed independently via endowments. As discussed in the proof of Proposition 1, for arbitrary changes of \(\psi ^{i*}\) one needs a change \(de^i\) in which \(de_{\varvec{1}}^i \in \text {Im}(X_{\varvec{1}},Y_{\varvec{1}})\), i.e., the future change lies within the asset span, and \(de_0^i = -\nabla _{\varvec{1}} U^i\cdot de_{\varvec{1}}^i\). Such changes leave \(c^i\) unaltered and thus do not affect \(\nabla U^i\). For arbitrary changes of \(\nabla _\omega U^i\) one needs a change \(de^i\) in which \(de_{\varvec{1}}^i \,\bot \, \text {Im}(X_{\varvec{1}},Y_{\varvec{1}})\), i.e., the future change is orthogonal to the asset span, and \(de_0^i = 0\). Such changes affect consumption \(dc^i = de^i\) directly and thus modify \(\nabla _\omega U^i\) but leave portfolios unchanged. Given \(K < I\), one can verify that DH has full rank. Assume for the moment that all consumers hold a nonzero share in all firms. Then one can use the endowment of consumer 1 to perturb the row corresponding to production of firm 1, the endowment of consumer 2 to perturb the row of firm 2, etc. Such future endowment changes must be orthogonal to the asset span. This covers the last K rows of DH. To perturb the first \(J+K\) rows, one can use the endowment of consumer I. The future endowment changes must lie in the asset span. Since share holdings are present in all rows of DH, such an endowment perturbation also has an effect on the last K rows. This effect can, however, be offset by orthogonal perturbation of other consumers’ endowments. One can use consumer 1 to offset the effect on firm 1, consumer 2 to offset the effect on firm 2, etc. Finally, consider the unlikely but possible case that some consumer \(h < K\) holds zero shares of firm h. In that case one needs an additional step before one can perturb the row of firm h: Perturb \(e^h\) within-span to increase consumer h’s demand for shares of firm h to a positive number and perturb \(e^I\) in the opposite direction within-span to keep aggregate demand constant.
Since DH has full rank at any point in \(H^{-1}(0)\), it follows that H is transverse to \(\{0\}\), written \(H \pitchfork \{0\}\). Denote \(H_e(p) = H(e,p)\) for some fixed \(e \in {\mathbb {E}}\) such that \(H_e:{\mathbb {R}}^{J+K} \rightarrow {\mathbb {R}}^{J+2K}\). It follows from the transversality theorem (Guillemin and Pollack (1974), p. 68 and Hirsch (1994), p. 74) that there is a full-measure subset \(E \subset {\mathbb {E}}\) such that \(H_e \pitchfork \{0\}\) for all \(e \in E\). Moreover, since \(H^{-1}(0) \subset {\mathbb {E}}\times \text {cl}({\varPi })\) and \(\text {cl}({\varPi })\) is compact, the set E is open and dense in \({\mathbb {E}}\). Eventually, note that \(H_e\) maps from \((J+K)\)-dimensional space to \((J+2K)\)-dimensional space. As in the above discussion of the exchange equilibrium manifold, one can apply the regular value theorem to deduce that \(H_e^{-1}(0)\) is empty for all \(e \in E\). Thus, generically the equation \(H(e,p) = 0\) has no solution. This concludes the proof of the theorem. \(\square \)
Rights and permissions
About this article
Cite this article
Zierhut, M. Constrained efficiency versus unanimity in incomplete markets. Econ Theory 64, 23–45 (2017). https://doi.org/10.1007/s00199-016-0968-1
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00199-016-0968-1
Keywords
- Incomplete markets with production
- Firm objectives
- Constrained efficiency
- Shareholder unanimity
- Majority stability