On the existence and uniqueness of the maximum likelihood estimates of parameters of Laplace Birnbaum–Saunders distribution based on Type-I, Type-II and hybrid censored samples

Abstract

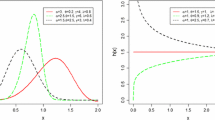
In this paper, we discuss the existence and uniqueness of the maximum likelihood estimates (MLEs) of the parameters of Laplace Birnbaum–Saunders distribution based on Type-I, Type-II and hybrid censored samples. We first derive the relationship between the MLEs of the two parameters and then discuss the monotonicity property of the profile likelihood function. Numerical iterative procedure is then discussed for determining the MLEs of the parameters. Finally, for illustrative purpose, we analyze one real data from the literature and present some graphical illustrations of the approach.

Appendix

Proof of Remark 1

It is clear that $\alpha (\beta ,t_{k:n},k)$ in (12), for $\beta \le t_{k:n}$, is not differentiable at $\beta =t_{i:n}$, for $i=1,\ldots ,k$. However, it is continuous, and so we can consider $\alpha (\beta ,t_{k:n},k)$ reaches the local maximum or local minimum at t if $\lim \nolimits _{x\rightarrow t^+} \alpha '(x,t_{k:n},k)\lim \nolimits _{x\rightarrow t^-}\alpha '(x,t_{k:n},k)<0$. For the sake of convenience, we define $\alpha '(x,t_{k:n},k)=0$ if $\lim \nolimits _{x\rightarrow t^+} \alpha '(x,t_{k:n},k)\lim \nolimits _{x\rightarrow t^-}\alpha '(x,t_{k:n},k)<0$. Now, if $t_{j:n}<\beta <t_{j+1:n}$, $j=0,\ldots ,k-1,$ with $t_{0:n}\equiv 0$, then the first derivative of $\alpha$ with respect to $\beta$ is given by

$$\begin{aligned} \alpha '(\beta ,t_{k:n},k)&=\frac{1}{2k\beta }\left[ \sum _{i=1}^j y_i(\beta ) -\sum _{i=j+1}^k y_i(\beta )- (n-k)S(\beta ,t_{k:n})\right] . \end{aligned}$$

It is clear that when $\beta \rightarrow 0$, we have $j=0$ and so

$$\begin{aligned}\lim \limits _{\beta \rightarrow 0} \alpha '(\beta ,t_{k:n},k)<0.\end{aligned}$$

We also have

$$\begin{aligned}\lim \limits _{\beta \rightarrow t_{k:n}^{-1}} \alpha '(\beta , t_{k:n}, k)= \frac{1}{2kt_{k:n}}\left\{ \sum _{i=1}^{k-1}\left[ \left( \frac{t_{k:n}}{t_{i:n}}\right) ^{\frac{1}{2}} +\left( \frac{t_{i:n}}{t_{k:n}}\right) ^{\frac{1}{2}}\right] - 2(n-k+1)\right\} \end{aligned}$$

and

$$\begin{aligned} {[}\beta \alpha '(\beta ,t_{k:n},k)]'=\frac{\alpha (\beta ,t_{k:n},k)}{4\beta }>0, \end{aligned}$$

which suggests that there exists at most one root for $[\beta \alpha '(\beta ,t_{k:n},k)]=0$, thus showing that there exists at most one root for $\alpha '(\beta ,t_{k:n},k)=0$ in turn. We can thus conclude the lemma, as required. $\square$

Proof of Lemma 1

Let us consider the first derivative of $\ln L_{profile}$ with respect to $\beta$ in each interval $(t_{j:n} , t_{j+1:n})$, for $j=0,\ldots , k-1$, with $t_0\equiv 0$, given by

$$\begin{aligned} \ln L'_{pro}=-\frac{k \alpha '(\beta ,t_{k:n},k)}{\alpha (\beta ,t_{k:n},k)} +\sum \limits _{i=1}^k\frac{\beta -t_{i:n}}{2\beta (\beta +t_{i:n})}=\frac{\gamma (\beta )}{\beta ^2\alpha (\beta ,t_{k:n},k)}, \end{aligned}$$

where $\gamma (\beta )=-\beta ^2 k\alpha '(\beta ,t_{k:n},k)+\beta \alpha (\beta ,t_{k:n},k)\sum \nolimits _{i=1}^k\frac{\beta -t_{i:n}}{2(\beta +t_{i:n})}$.

Moreover, we have

$$\begin{aligned} \lim _{\beta \rightarrow 0}\ln L'_{pro}&= \lim _{\beta \rightarrow 0}\left\{ \frac{k}{\beta }\frac{\sum \nolimits _{i=1}^k\left( \frac{\beta }{t_{i:n}}\right) ^\frac{1}{2}+(n-k)\left( \frac{\beta }{t_{k:n}}\right) ^\frac{1}{2}}{\sum \nolimits _{i=1}^k\left[ \left( \frac{t_{i:n}}{\beta }\right) ^{\frac{1}{2}}-\left( \frac{\beta }{t_{i:n}}\right) ^{\frac{1}{2}}\right] +(n-k)\left[ \left( \frac{t_{k:n}}{\beta }\right) ^{\frac{1}{2}}-\left( \frac{\beta }{t_{k:n}}\right) ^{\frac{1}{2}}\right] }\right. \\&\quad \left. +\sum _{i=1}^k\frac{1}{\beta +t_{i:n}}\right\} >0,\\ \lim _{\beta \rightarrow t_{k:n}^-}\ln L'_{pro}&= - \frac{k}{t_{k:n}}\frac{\sum \nolimits _{i=1}^{k-1}\left( \frac{t_{i:n}}{t_{k:n}}\right) ^\frac{1}{2}-(n-k+1)}{\sum \nolimits _{i=1}^{k-1}\left[ \left( \frac{t_{k:n}}{t_{i:n}}\right) ^{\frac{1}{2}}-\left( \frac{t_{i:n}}{t_{k:n}}\right) ^{\frac{1}{2}}\right] } -\frac{1}{t_{k:n}}\sum _{i=1}^k\frac{t_{i:n}}{t_{k:n}+t_{i:n}}\\&=-\frac{C_1(t_{k:n},k)}{t_{k:n}}. \end{aligned}$$

Thus, if $\lim _{\beta \rightarrow t_{k:n}^-}\ln L'_{pro}<0$, then there must exist a $\beta _0$ such that $\gamma (\beta )>0$ for $\beta <\beta _0$ and $\gamma (\beta )<0$ for $\beta >\beta _0$. We show in the following once $\gamma (\beta )<0$, then $\gamma (\beta )$ will be a decreasing function of $\beta$, which in turn suggests that there will be no root for $\gamma (\beta )=0$ for $\beta >\beta _0$. The first derivative of $\gamma (\beta )$ is

$$\begin{aligned} \gamma '(\beta )=&-\left[ k\beta \alpha '(\beta ,t_{k:n},k)+\frac{k\alpha (\beta ,t_{k:n},k)}{4}\right] \\&+\left[ \left( \alpha (\beta ,t_{k:n},k)+\beta \alpha '(\beta ,t_{k:n},k)\right) \sum \limits _{i=1}^k\frac{\beta -t_{i:n}}{2(\beta +t_{i:n})}\right. \\&\left. +\beta \alpha (\beta ,t_{k:n},k)\sum _{i=1}^k\frac{t_{i:n}}{(t_{i:n}+\beta )^2}\right] \\ =&-\alpha '(\beta ,t_{k:n},k)\beta \sum _{i=1}^k\frac{\beta +3t_{i:n}}{2(\beta +t_{i:n})}+ \alpha (\beta ,t_{k:n},k)\sum _{i=1}^k\frac{(\beta -t_{i:n})(\beta +3t_{i:n})}{4(t_{i:n}+\beta )^2}\\ =&-\alpha '(\beta ,t_{k:n},k)\beta \sum _{i=1}^k\frac{\beta +3t_{i:n}}{2(\beta +t_{i:n})} +\alpha (\beta ,t_{k:n},k)\\&\times \left[ \frac{1}{k}\sum _{i=1}^k\frac{\beta +3t_{i:n}}{2(\beta +t_{i:n})}\sum _{i=1}^k\frac{\beta -t_{i:n}}{2(\beta +t_{i:n})} \right. \\&\left. -\sum _{i=1}^k\left( \frac{t_{i:n}}{t_{i:n}+\beta }\right) ^2 +\frac{1}{k}\left( \sum _{i=1}^k\frac{t_{i:n}}{t_{i:n}+\beta }\right) ^2\right] \\ =&\frac{\gamma (\beta )}{k\beta }\sum _{i=1}^k\frac{\beta +3t_i}{2(\beta +t_i)}\\&-\frac{\alpha (\beta ,t_{k:n},k)}{k}\left[ k\sum _{i=1}^k\left( \frac{t_i}{t_i+\beta }\right) ^2-\left( \sum _{i=1}^k\frac{t_i}{t_i+\beta }\right) ^2\right] <0.\\ \end{aligned}$$

Moreover, for any non-differentiable point $t_{j:n}$ for $j=1,\ldots ,k-1$, we have

$$\begin{aligned}&\lim \limits _{\beta \rightarrow t_{j:n}^-}\ln L'_{pro}-\lim \limits _{\beta \rightarrow t_{j:n}^+}\ln L'_{pro}\\&\quad =\frac{k\left( \lim \limits _{\beta \rightarrow t_{j:n}^+}\alpha '(\beta ,t_{k:n},k)-\lim \limits _{\beta \rightarrow t_{j:n}^-}\alpha '(\beta ,t_{k:n},k)\right) }{\alpha (t_{j:n},t_{k:n},k)}>0. \end{aligned}$$

We can thus conclude that if $\lim \nolimits _{\beta \rightarrow t_{k:n}}\ln L'_{pro}<0$, then there exists only one root for the equation $\ln L'_{pro}(\beta )=0$ for $\beta \le t_{k:n}$, and if $\lim \nolimits _{\beta \rightarrow t_{k:n}}\ln L'_{pro}>0$, then there will be no root for the equation $\ln L'_{pro}(\beta )=0$ for $\beta \le t_{k:n}$. $\square$

Proof of Lemma 3

We will first prove that $H(\eta (\beta ,t_{k:n}))$ is a decreasing function of $\beta$ for $\beta >t_{k:n}$. It is well-known that the hazard function of the standard Laplace distribution is an increasing function of x for $x<0$ and a constant for $x\ge 0$. Studying the monotonicity of $H(\eta (\beta ,t_{k:n}))$ is equivalent to studying the property of $\eta (\beta ,t_{k:n})$, where

$$\begin{aligned} \eta (\beta ,t_{k:n})=-\frac{S(\beta ,t_{k:n})}{\alpha (\beta ,t_{k:n},k)} = -\frac{\left( \sqrt{\frac{\beta ^2}{t_{k:n}}}-\sqrt{t_{k:n}}\right) \left( {\sum \limits _{i=1}^k\frac{t_{k:n}+t_{i:n}}{t_{i:n}+\beta }}\right) }{\sum \limits _{i=1}^k\left( \sqrt{\frac{t_{k:n}^2}{t_{i:n}}}-\sqrt{t_{i:n}}\right) }. \end{aligned}$$

Let us denote $g_1(\beta )=\left( \sqrt{\frac{\beta ^2}{t_{k:n}}}-\sqrt{t_{k:n}}\right) \left( {\sum \limits _{i=1}^k\frac{t_{k:n}+t_{i:n}}{t_{i:n}+\beta }}\right)$; we then have

$$\begin{aligned} g_1'(\beta )= & {} \sqrt{\frac{1}{t_{k:n}}} \left( {\sum \limits _{i=1}^k\frac{t_{k:n}+t_{i:n}}{t_{i:n}+\beta }}\right) -\left( \sqrt{\frac{\beta ^2}{t_{k:n}}}-\sqrt{t_{k:n}}\right) \left( {\sum \limits _{i=1}^k\frac{t_{k:n}+t_{i:n}}{(t_{i:n}+\beta )^2}}\right) \\> & {} \sqrt{\frac{1}{t_{k:n}}} \left( {\sum \limits _{i=1}^k\frac{t_{k:n}+t_{i:n}}{t_{i:n}+\beta }}\right) -\left( \sqrt{\frac{\beta ^2}{t_{k:n}}}-\sqrt{t_{k:n}}\right) \left( {\sum \limits _{i=1}^k\frac{t_{k:n}+t_{i:n}}{(t_{i:n}+\beta )\beta }}\right) >0. \end{aligned}$$

Hence, $\eta (\beta ,t_{k:n})$ is a decreasing function of $\beta$.

Next, we will prove that $\alpha (\beta ,t_{k:n},k)\sqrt{\beta }\sum \nolimits _{i=1}^k\frac{t_{i:n}}{t_{i:n}+\beta }=g_2(\beta )$ is an increasing function of $\beta$ for $\beta >t_{k:n}$, where

$$\begin{aligned} g_2(\beta )=\sum \limits _{i=1}^k\left( \sqrt{\frac{t_{k:n}^2}{t_{i:n}}}-\sqrt{t_{i:n}}\right) \frac{\sum _{i=1}^k\frac{t_{i:n}}{t_{i:n}+\beta }}{\sum _{i=1}^k\frac{t_{k:n}+t_{i:n}}{t_{i:n}+\beta }}. \end{aligned}$$

Let us consider

$$\begin{aligned} g_3(\beta )=\frac{\sum _{i=1}^k\frac{t_{i:n}}{t_{i:n}+\beta }}{\sum _{i=1}^k\frac{t_{k:n}+t_{i:n}}{t_{i:n}+\beta }}; \end{aligned}$$

then, we have

$$\begin{aligned} g_3'(\beta )= & {} \frac{-\sum _{i=1}^k\frac{t_{i:n}}{(t_{i:n}+\beta )^2}\sum _{i=1}^k\frac{t_{k:n}}{(t_{i:n}+\beta )} +\sum _{i=1}^k\frac{t_{k:n}}{(t_{i:n}+\beta )^2}\sum _{i=1}^k\frac{t_{i:n}}{(t_{i:n}+\beta )}}{\left( \sum _{i=1}^k\frac{t_{k:n}+t_{i:n}}{t_{i:n}+\beta }\right) ^2}\\= & {} \frac{t_{k:n}\left[ k\sum _{i=1}^k\frac{1}{(t_{i:n}+\beta )^2}-\left( \sum _{i=1}^k\frac{1}{t_{i:n}+\beta }\right) ^2\right] }{\left( \sum _{i=1}^k\frac{t_{k:n}+t_{i:n}}{t_{i:n}+\beta }\right) ^2}>0, \end{aligned}$$

showing the monotonicity of $g_2(\beta )$ and then $G(\beta )$ in turn.

Moreover, we have

$$\begin{aligned} \lim \limits _{\beta \rightarrow t_{k:n}^+}G(\beta )= & {} - \frac{\sqrt{t_{k:n}}}{k} \sum \limits _{i=1}^k\left( \sqrt{\frac{t_{k:n}}{t_{i:n}}}-\sqrt{\frac{t_{i:n}}{t_{k:n}}}\right) \sum \limits _{i=1}^k\frac{t_{i:n}}{t_{i:n}+t_{k:n}}\\&-\sum \limits _{i=1}^k\sqrt{t_{i:n}}+(n-k)\sqrt{t_{k:n}}\\= & {} - \frac{\sqrt{t_{k:n}}}{k} \sum \limits _{i=1}^k\left( \sqrt{\frac{t_{k:n}}{t_{i:n}}}-\sqrt{\frac{t_{i:n}}{t_{k:n}}}\right) \\&\times \left[ \sum \limits _{i=1}^k\frac{t_{i:n}}{t_{i:n}+t_{k:n}} +k\frac{\sum \limits _{i=1}^k\sqrt{\frac{t_{i:n}}{t_{k:n}}}-(n-k)}{\sum \limits _{i=1}^k\left( \sqrt{\frac{t_{k:n}}{t_{i:n}}}-\sqrt{\frac{t_{i:n}}{t_{k:n}}}\right) }\right] \\= & {} - \frac{\sqrt{t_{k:n}}}{k} \sum \limits _{i=1}^k\left( \sqrt{\frac{t_{k:n}}{t_{i:n}}}-\sqrt{\frac{t_{i:n}}{t_{k:n}}}\right) C_2(t_{k:n},k),\\ \lim \limits _{\beta \rightarrow \infty }G(\beta )= & {} -\sqrt{t_{k:n}} \sum \limits _{i=1}^k\left( \sqrt{\frac{t_{k:n}}{t_{i:n}}}-\sqrt{\frac{t_{i:n}}{t_{k:n}}}\right) \\&\times \left[ \frac{\sum \limits _{i=1}^kt_{i:n}}{\sum \limits _{i=1}^k(t_{i:n}+t_{k:n})} +\frac{\sum \limits _{i=1}^k\sqrt{\frac{t_{i:n}}{t_{k:n}}}-(n-k)(\eta (\infty ,t_{k:n}))}{\sum \limits _{i=1}^k\left( \sqrt{\frac{t_{k:n}}{t_{i:n}}}-\sqrt{\frac{t_{i:n}}{t_{k:n}}}\right) }\right] \\= & {} -\sqrt{t_{k:n}} \sum \limits _{i=1}^k\left( \sqrt{\frac{t_{k:n}}{t_{i:n}}}-\sqrt{\frac{t_{i:n}}{t_{k:n}}}\right) C_3(t_{k:n},k). \end{aligned}$$

$\square$