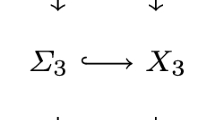Abstract
We define and investigate a class of compact homogeneous CR manifolds, that we call \( \mathfrak{n} \)-reductive. They are orbits of minimal dimension of a compact Lie group K 0 in algebraic affine homogeneous spaces of its complexification K. For these manifolds we obtain canonical equivariant fibrations onto complex flag manifolds, generalizing the Hopf fibration \( {S^3}\to \mathbb{C}{{\mathbb{P}}^1} \). These fibrations are not, in general, CR submersions, but satisfy the weaker condition of being CR-deployments; to obtain CR submersions we need to strengthen their CR structure by lifting the complex stucture of the base.
Similar content being viewed by others
References
D. V. Alekseevsky, A. Spiro, Compact homogeneous CR manifolds, J. Geom. Anal. 12 (2002), no. 2, 183–201. MR 1888514 (2003e:32062).
D. V. Alekseevsky, A. Spiro, Invariant CR structures on compact homogeneous manifolds, Hokkaido Math. J. 32 (2003), no. 2, 209–276. MR 1996278(2004f:32047).
A. Altomani, C. Medori, M. Nacinovich, The CR structure of minimal orbits in complex flag manifolds, J. Lie Theory 16 (2006), no. 3, 483–530. MR 2248142 (2007c:32043).
A. Altomani, C. Medori, M. Nacinovich, On homogeneous and symmetric CR manifolds, Boll. Unione Mat. Ital. (9) 3 (2010), no. 2, 221–265. MR 2666357 (2011e:32045).
A. Altomani, C. Medori, M. Nacinovich, Orbits of real forms in complex flag manifolds, Ann. Scuola Norm. Sup. Pisa Cl. Sci. (5) 9 (2010), no. 1, 69–109. MR 2668874.
A. Andreotti, G. A. Fredricks, Embeddability of real analytic Cauchy−Riemann manifolds, Ann. Scuola Norm. Sup. Pisa Cl. Sci. (4) 6 (1979), no. 2, 285–304. MR 541450 (80h:32019).
W. M. Boothby, H. C. Wang, On contact manifolds, Ann. of Math. (2) 68 (1958), 721–734. MR 0112160 (22 #3015).
A. Borel, J. Tits, Éléments unipotents et sous-groupes paraboliques de groupes réductifs. I, Invent. Math. 12 (1971), 95–104. MR 0294349 (45 #3419).
N. Bourbaki, Éléments de Mathématique. Fasc. XXXVIII: Groupes et Algèbres de Lie. Chap. VII: Sous-Algèbres de Cartan, Éléments Réguliers. Chap. VIII: Algèbres de Lie Semi-Simples Déployées, Actualités Scientifiques et Industrielles, No. 1364, Hermann, Paris, 1975. MR 0453824 (56 #12077). Russian transl.: Н. Бурбаки, Группы и алгебры Ли. Подалгебры Картана, pегуляpные злeмeнты, paсщeпляeмые полупpocтые aлгeбpы Ли, Миp, M., 1978.
J.-Y. Charbonnel, H. O. Khalgui, Classification des structures CR invariantes pour les groupes de Lie compacts, J. Lie Theory 14 (2004), no. 1, 165–198. MR 2040175 (2005b:22012).
A. A. George Michael, On the conjugacy theorem of Cartan subalgebras, Hiroshima Math. J. 32 (2002), no. 2, 155–163. MR 1925894(2004f:17011).
Б. Гиллиган, А. Т. Xaклберри, Слоения и глобализации компактных однородныx CR-многообразий, Изв. PAH, cep. мат 73 (2009), no. 3, 67–126. Engl. transl.: B. Gilligan, A. T. Huckleberry, Fibrations and globalizations of compact homogeneous CR-manifolds, Izvestiya: Math. 73 (2009), no. 3, 501–553. MR 2553089 (2011c:32056).
J. E. Humphreys, Linear Algebraic Groups, Graduate Texts in Mathematics, Vol. 21, Springer-Verlag, New York, 1975. MR 0396773 (53 #633). Russian transl.: Дж. Хамфри, Линейные алгебраические группы, Наука, M., 1980.
A. W. Knapp, Lie Groups Beyond an Introduction, 2nd ed., Progress in Mathematics, Vol. 140, Birkhäuser Boston, Boston, MA, 2002. MR 1920389 (2003c:22001).
B. Kostant, Root systems for Levi factors and Borel−de Siebenthal theory, in: Symmetry and Spaces, Progress in Mathematics, Vol. 278, Birkhäuser Boston, Boston, MA, 2010, pp. 129–152. MR 2562626 (2011b:17023).
T. Matsuki, Closure relations for orbits on affine symmetric spaces under the action of parabolic subgroups. Intersections of associated orbits, Hiroshima Math. J. 18 (1988), no. 1, 59–67. MR 935882(89f:53073).
Y. Matsushima, Espaces homogènes de Stein des groupes de Lie complexes, Nagoya Math. J 16 (1960), 205–218. MR 0109854 (22#739).
Y. Matsushima, A. Morimoto, Sur certains espaces fibrés holomorphes sur une variété de Stein, Bull. Soc. Math. France 88 (1960), 137–155. MR 0123739 (23 #A1061).
C. Medori, M. Nacinovich, Algebras of infinitesimal CR automorphisms, J. Algebra 287 (2005), no. 1, 234–274. MR 2134266 (2006a:32043).
В.В. Морозов, Доказательство теоремы регулярности, УMН XI (1956), №5, 191–194 (Russian). [V. V. Morozov, Proof of the theorem of regularity, Usp. Mat. Nauk XI (1956), №5, 191–194. MR 0088488 (19,527c)].
G. D. Mostow, On covariant fiberings of Klein spaces, Amer. J. Math. 77 (1955), 247–278. MR 0067901 (16,795d).
G. D. Mostow, Covariant fiberings of Klein spaces. II, Amer. J. Math. 84 (1962), 466–474. MR 0142688 (26 #257).
A. L. Onishchik, È. B. Vinberg, Lie Groups and Algebraic Groups, Springer Series in Soviet Mathematics, Springer-Verlag, Berlin, 1990.
В. П. Платонов, Доказательство гипотезы конечности для разрешимых подгрупп алгебраических групп, Сиб. мат. жypн. 10 (1969), 1084–1090. Engl. transl.: V. P. Platonov, A proof of the finiteness hypothesis for solvable subgroups of algebraic groups, Sib. Math. J. 10 (1969), 800–804. MR 0254145 (40 #7355).
B. Ю. Вейсфейлер, Об одном классе унипотентных подгрупп полупростых алгебраических групп, УМН XXI (1966), №2, 222–223. Engl. transl.: B. Weisfeiler, On one class of unipotent subgroups of semisimple algebraic groups, arXiv:math/0005149v1 [math.AG].
J. A. Wolf, The action of a real semisimple group on a complex flag manifold. I. Orbit structure and holomorphic arc components, Bull. Amer. Math. Soc. 75 (1969), 1121–1237.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Altomani, A., Medori, C. & Nacinovich, M. REDUCTIVE COMPACT HOMOGENEOUS CR MANIFOLDS. Transformation Groups 18, 289–328 (2013). https://doi.org/10.1007/s00031-013-9218-9
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00031-013-9218-9