Abstract
We analyse the process of energy exchanges generated by the elastic collisions between a point-particle, confined to a two-dimensional cell with convex boundaries, and a ‘piston’, i.e. a line-segment, which moves back and forth along a one-dimensional interval partially intersecting the cell. This model can be considered as the elementary building block of a spatially extended high-dimensional billiard modeling heat transport in a class of hybrid materials exhibiting the kinetics of gases and spatial structure of solids. Using heuristic arguments and numerical analysis, we argue that, in a regime of rare interactions, the billiard process converges to a Markov jump process for the energy exchanges and obtain the expression of its generator.





Similar content being viewed by others
Notes
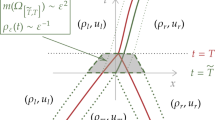
The upper bound on \(\delta \) is imposed so as to prevent overlap between the piston and a similar hypothetical vertical piston centered on either of the top and bottom edges of the cell, such as in the cells depicted in Fig. 1.
For \(\rho = \tfrac{1}{2}\), however, we have \(\lambda = 0\) so that, in the small \(\delta \) regime, \(|\Gamma | \simeq 2 \delta \left( 1 - \tfrac{\pi }{4}\right) \) and \(|\partial \Gamma _{\textsc {bp}}| \simeq \tfrac{2\sqrt{2}}{3} \delta ^3\). The two limits \(\rho \rightarrow \tfrac{1}{2}\) and \(\delta \rightarrow 0\) are therefore not interchangeable:
$$\begin{aligned} \lim _{\rho \rightarrow {1}/{2}} \lim _{\delta \rightarrow 0} \delta ^2 \frac{|\Gamma |}{|\partial \Gamma _{\textsc {bp}}|} = \frac{1}{4 \sqrt{2}} (4 - \pi ) \ne \lim _{\delta \rightarrow 0} \lim _{\rho \rightarrow {1}/{2}} \delta ^2 \frac{|\Gamma |}{|\partial \Gamma _{\textsc {bp}}|} = \frac{3}{ 4 \sqrt{2}} ( 4 - \pi )\,. \end{aligned}$$The integral of the surface element on \(\mathbb {S}^{2}\) along the two horizontal circles at heights \(\pm \sqrt{2\epsilon _{\textsc {p}} }\) is \(1/\sqrt{2\epsilon _{\textsc {p}} }\), which is the density of the Beta distribution of shape parameters \(\tfrac{1}{2}\) and 1, properly normalised.
The energy values include \(\epsilon _{\textsc {p}} = 1/100\), 2 / 100, \(\dots \), 49 / 100, to which are added \(\epsilon _{\textsc {p}} = 1/200\), 1 / 400, \(\dots \), 1 / 3200 and \(\epsilon _{\textsc {p}} = {1}/{2}-1/200\), \({1}/{2}-1/400\), \(\dots \), \({1}/{2}-1/3200\).
References
Ruelle, D.: General linear response formula in statistical mechanics, and the fluctuation-dissipation theorem far from equilibrium. Phys. Lett. A 245(3—-4), 220–224 (1998). doi:10.1016/S0375-9601(98)00419-8
Ruelle, D.: In: Nicolis, G. (ed.) Probabilistic and thermodynamic aspects of nonlinear dynamics. Satellite Meeting to STATPHYS 20. Université Libre de Bruxelles, Brussels (1998)
Fourier, J.: Theorie analytique de la chaleur, par M. Fourier (Firmin Didot, père et fils, 1822) (Reprinted, J Gabay, Paris, 1988). http://www.gabay-editeur.com/FOURIER-Theorie-analytique-de-la-chaleur-1822
Bonetto, F., Lebowitz, J.L., Rey-Bellet, L.: Fourier’s Law: A Challenge to Theorists, chap. 8. Imperial College Press, Distributed by World Scientific Publishing Co, London, pp. 128–150 (2000). http://www.worldscientific.com/doi/abs/10.1142/9781848160224_0008
Narasimhan, T.N.: Fourier’s heat conduction equation: history, influence, and connections. Rev. Geophys. 37(1), 151–172 (1999). doi:10.1029/1998RG900006
Lepri, S., Livi, R., Politi, A.: Thermal conduction in classical low-dimensional lattices. Phys. Rep. 377(1), 1–80 (2003). doi:10.1016/S0370-1573(02)00558-6
Dhar, A.: Heat transport in low-dimensional systems. Adv. Phys. 57(5), 457–537 (2008). doi:10.1080/00018730802538522
Bunimovich, L.A., Liverani, C., Pellegrinotti, A., Suhov, Y.M.: Ergodic systems of n balls in a billiard table. Commun. Math. Phys. 146, 357–396 (1992). doi:10.1007/BF02102633
Gaspard, P., Gilbert, T.: Heat conduction and Fourier’s law by consecutive local mixing and thermalization. Phys. Rev. Lett. 101(2), 20601 (2008). doi:10.1103/PhysRevLett.101.020601
Sasada, M.: Heat conductivity for stochastic exchange model with mechanical origin. Preprint (2015)
Gaspard, P., Gilbert, T.: On the derivation of Fourier’s law in stochastic energy exchange systems. J. Stat. Mech. 11(1), 021 (2008). doi:10.1088/1742-5468/2008/11/P11021
Sasada, M.: Spectral gap for stochastic energy exchange model with non-uniformly positive rate function. Ann. Probab. 43(4), 1663–1711 (2015). doi:10.1214/14-AOP916
Grigo, A., Khanin, K., Szász, D.: Mixing rates of particle systems with energy exchange. Nonlinearity 25(8), 2349–2376 (2012). doi:10.1088/0951-7715/25/8/2349
Liverani, C., Olla, S.: Toward the Fourier law for a weakly interacting anharmonic crystal. J. Am. Math. Soc. 25(2), 555–583 (2012). doi:10.1090/S0894-0347-2011-00724-8
Bricmont, J., Kupiainen, A.: Towards a derivation of Fourier’s law for coupled anharmonic oscillators. Commun. Math. Phys. 274(3), 555–626 (2007). doi:10.1007/s00220-007-0284-5
Bernardin, C., Olla, S.: Fourier’s law for a microscopic model of heat conduction. J. Stat. Phys. 121(3), 271–289 (2005). doi:10.1007/s10955-005-7578-9
Keller, G., Liverani, C.: Map lattices coupled by collisions. Commun. Math. Phys. 291(2), 591–597 (2009). doi:10.1007/s00220-009-0835-z
Huveneers, F.: Energy transport through rare collisions. J. Stat. Phys. 146(1), 73–97 (2012). doi:10.1007/s10955-011-0374-9
Eckmann, J.P., Young, L.S.: Nonequilibrium energy profiles for a class of 1-D Models. Commun. Math. Phys. 262(1), 237–267 (2006). doi:10.1007/s00220-005-1462-y
Li, Y., Nándori, P., Young, L.S.: Local thermal equilibrium for certain stochastic models of heat transport. J. Stat. Phys. 163(1), 61–91 (2016). doi:10.1007/s10955-016-1466-3
Kipnis, C., Marchioro, C., Presutti, E.: Heat flow in an exactly solvable model. J. Stat. Phys. 27(1), 65–74 (1982). doi:10.1007/BF01011740
Bonetto, F., Lebowitz, J.L., Lukkarinen, J.: Fourier’s law for a harmonic crystal with self-consistent stochastic reservoirs. J. Stat. Phys. 116, 783–813 (2004). doi:10.1023/B:JOSS.0000037232.14365.10
Gaspard, P., Gilbert, T.: Heat conduction and Fourier’s law in a class of many particle dispersing billiards. New J. Phys. 10(1), 3004 (2008). doi:10.1088/1367-2630/10/10/103004
Gaspard, P., Gilbert, T.: A two-stage approach to relaxation in billiard systems of locally confined hard spheres. Chaos 22, 026117 (2012). doi:10.1063/1.3697689
Bálint, P., Tóth, I.P.: Exponential decay of correlations in multi-dimensional dispersing billiards. Annales Henri Poincaré 9, 1309–1369 (2008). doi:10.1007/s00023-008-0389-1
Pajor-Gyulai, Z., Szász, D., Tóth, I.P.: Billiard models and energy transfer. In: Exner, P. (ed.) XVIth International Congress on Mathematical Physics. Prague. World Scientific, pp. 328–332 (2010). http://www.worldscientific.com/worldscibooks/10.1142/7727. Accessed 3–8 August 2009
Chernov, N., Dolgopyat, D.: Brownian Brownian Motion-I, Memoirs of the American Mathematical Society, vol. 198, American Mathematical Society, 2009. http://www.ams.org/bookstore-getitem/item=memo-198-927
Bálint, P., Nándori, P., Szász, D., Tóth, I.P.: Equidistribution for standard pairs in planar dispersing billiard flows. Work in progress (2015)
Dolgopyat, D., Liverani, C.: Energy transfer in a fast-slow Hamiltonian system. Commun. Math. Phys. 308(1), 201 (2011). doi:10.1007/s00220-011-1317-7
Dolgopyat, D., Nándori, P.: arXiv preprint. (2016). http://arxiv.org/abs/1603.07590
Kullback, S., Leibler, R.A.: On information and sufficiency. Ann. Math. Stat. 22(1), pp. 79–86 (1951). http://www.jstor.org/stable/2236703
Cornfeld, I.P., Fomin, S.V., Sinai, Y.G.: Ergodic Theory. Springer, New York (1982). http://link.springer.com/book/10.1007
Chernov, N.I.: Entropy, Lyapunov exponents, and mean free path for billiards. J. Stat. Phys. 88(1), 1–29 (1997). doi:10.1007/BF02508462
Chernov, N.I.: New proof of Sinai’s formula for the entropy of hyperbolic billiard systems. Application to Lorentz gases and Bunimovich stadiums. Funct. Anal. Appl. 25(3), 204–219 (1991). doi:10.1007/BF01085490
Gaspard, P., Gilbert, T.: Heat transport in stochastic energy exchange models of locally confined hardspheres. J. Stat. Mech. 08, 020 (2009). doi:10.1088/1742-5468/2009/08/P08020
Acknowledgments
The authors gratefully acknowledge fruitful discussions with Tamás Tasnádi. They are also grateful to Makiko Sasada for communicating her results prior to publishing. TG and PN wish to acknowledge the hospitality of the Institute of Mathematics at the Budapest University of Technology and Economics where part of this work was conducted. TG also wishes to acknowledge stimulating discussions with the DinAmicI community during their 2015 workshop, held in Corinaldo, Italy. The authors are grateful to the Erwin Schrödinger Institute, Vienna, for their hospitality on the occasion of the workshop Mixing Flows and Averaging Methods during which this work was finalized. Finally, they also express their gratitude to the referees for valuable comments. PB, DSz and IPT acknowledge the financial support of the Hungarian National Foundation for Scientific Research (OTKA): Grant T104745 and of Stiftung Aktion Österreich-Ungarn: Grants 87öu6 and 92öu6. TG is financially supported by the (Belgian) FRS-FNRS.
Author information
Authors and Affiliations
Corresponding author
Additional information
To David Ruelle and Yasha Sinai on the occasion of their 80th birthdays: We are deeply honored and privileged to dedicate this paper to David Ruelle and Yasha Sinai, great founding fathers of rigorous statistical physics. Their works were fundamental in creating the subject and have profoundly changed the way people think about it. In particular, beyond its wider and deeper impact, Ruelle’s 1998 work [1], as well as his Brussels lecture [2], were key to reinvigorating the general interest toward understanding Fourier’s law of heat conduction and raising hopes for a satisfactory answer to this difficult problem. The theory of hyperbolic billiards, initiated by Sinai, helped engineer models which offer the most promising perspectives in this direction. It is our hope this paper testifies to their influence.
Appendices
Appendix 1: Collision Volume
To determine the volume \(|\Gamma |\) of configuration space, note that when \(q_3 > (1 - \lambda )/2\), the volume of all possible positions \(q_1\) and \(q_2\) is
When \(q_3 < (1 - \lambda )/2\), we must subtract from the above area the quantity
We therefore obtain the total volume of configuration space (10) by multiplying Eq. (41) by \(\lambda + 2 \delta \) and subtracting the integral of Eq. (42) over \(q_3\) from \((1 - \lambda )/2 - \delta \) to \((1 - \lambda )/2\).
To compute the collision surface integral, note that the position coordinates on \(\partial \Gamma _{\textsc {bp}}\) are bounded according to
Its projection on the \((q_1, q_2)\) plane is the area (42) evaluated at \(q_3 = (1 - \lambda )/2 - \delta \). Since the surface itself makes an angle \(\pi /4\) with respect to the \((q_1, q_2)\) plane, we obtain for \(|\partial \Gamma _{\textsc {bp}}|\) the expression given by Eq. (11).
Appendix 2: Ball-Wall and Piston-Wall Return Times
Wall collision return times of the piston and ball can be computed in ways similar to Eq. (9),
where \(|\partial \Gamma _{\textsc {pw}}|\) and \(|\partial \Gamma _{\textsc {bw}}|\) are the areas of piston-wall and ball-wall collisions. The former corresponds to the area of all positions \(q_1\) and \(q_2\) such that \(q_3 = ( 1 \pm \lambda )/2 \pm \delta \), which is parallel to the \((q_1, q_2)\) plane and twice the area (41) minus the projection of the collision surface \(|\partial \Gamma _{\textsc {bp}}|\) (11) on this plane, and the latter to the positions \(q_1, q_2\) such that \((q_1 \pm \tfrac{1}{2})^2 + (q_2 \pm \tfrac{1}{2})^2 = \rho ^2\) while \(q_1 < q_3\), with \(q_3\) integrated over the interval (1). That is,
and
Appendix 3: Restriction of the Invariant Measure on \({M}_{\textsc {bp}}\) to Fixed Energy Configurations
Substituting the parametrisation (17) of the velocity vector \(\mathbf {v}\in \mathbb {S}^{2}\) and evaluating its scalar product with the normal vector (6), the velocity integral in Eq. (19) splits into two contributions, integrated over an arc-length proportional to the angle along the arcs:
see Fig. 6 for a graphical representation. Two separate regimes arise.
Equation (47) has either one (\(1/4 \le \epsilon _{\textsc {p}} \le \tfrac{1}{2}\)) or two (\(0 \le \epsilon _{\textsc {p}} < 1/4\)) contributions, given by the integrals along the arc-circles on the hemisphere of velocity coordinates whose projection along the normal to the collision surface (6) is positive. Here \(\epsilon _{\textsc {p}} = 1/8\) and the two arc-circles at \(v_3 = \pm \tfrac{1}{2}\) contributing to Eq. (47) are the portions (in green) of the corresponding full circles above the plane tangent to the collision surface (the excluded parts of those circles are shown in white) (Color figure online)
On the one hand, when the piston’s energy is less than the ball’s, the condition \(\sqrt{2\epsilon _{\textsc {p}} } \ge \sqrt{1 - 2\epsilon _{\textsc {p}} } \cos \alpha \) in the first of the two integrals on the right-hand side of Eq. (47) is equivalent to
Performing the integral, we obtain the contribution,
Likewise, the condition \(\sqrt{2\epsilon _{\textsc {p}} } \le - \sqrt{1 - 2\epsilon _{\textsc {p}} } \cos \alpha \) in the second of the two integrals on the right-hand side of Eq. (47) is equivalent to
Thus the second integral yields the contribution
The contribution to Eq. (19) from the velocity integral in the corresponding energy interval is thus given by the sum of Eqs. (49) and (51).
On the other hand, when the piston’s energy is larger than the ball’s, the condition \(\sqrt{2\epsilon _{\textsc {p}} } \ge \sqrt{1 - 2\epsilon _{\textsc {p}} } \cos \alpha \) in the first of the two integrals on the right-hand side of Eq. (47) holds true for all angles \(\alpha \). The result of the integration,
yields the only contribution to the velocity integral in Eq. (19).
Rights and permissions
About this article
Cite this article
Bálint, P., Gilbert, T., Nándori, P. et al. On the Limiting Markov Process of Energy Exchanges in a Rarely Interacting Ball-Piston Gas. J Stat Phys 166, 903–925 (2017). https://doi.org/10.1007/s10955-016-1598-5
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10955-016-1598-5