Skip to main content
SpringerLink
Log in

Diffusion in Porous Media: Phenomena and Mechanisms

  • Published:
Transport in Porous Media Aims and scope Submit manuscript

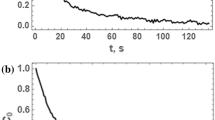
Abstract

Two distinct but interconnected approaches can be used to model diffusion in fluids; the first focuses on dynamics of an individual particle, while the second deals with collective (effective) motion of (infinitely many) particles. We review both modeling strategies, starting with Langevin’s approach to a mechanistic description of the Brownian motion in free fluid of a point-size inert particle and establishing its relation to Fick’s diffusion equation. Next, we discuss its generalizations which account for a finite number of finite-size particles, particle’s electric charge, and chemical interactions between diffusing particles. That is followed by introduction of models of molecular diffusion in the presence of geometric constraints (e.g., the Knudsen and Fick–Jacobs diffusion); when these constraints are imposed by the solid matrix of a porous medium, the resulting equations provide a pore-scale representation of diffusion. Next, we discuss phenomenological Darcy-scale descriptors of pore-scale diffusion and provide a few examples of other processes whose Darcy-scale models take the form of linear or nonlinear diffusion equations. Our review is concluded with a discussion of field-scale models of non-Fickian diffusion.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6

Similar content being viewed by others

References

  • Batchelor, G.K.: Brownian diffusion of particles with hydrodynamic interaction. J. Fluid Mech. 74(1), 1–29 (1976). https://doi.org/10.1017/S0022112076001663

    Article  Google Scholar 

  • Battiato, I., Ferrero V, P.T., O’ Malley, D., Miller, C.T., Takhar, P.S., Valdes-Parada, F.J., Wood, B.D.: Theory and applications of macroscale models. Transp. Porous Media (2019) (under review)

  • Bear, J.: Dynamics of Fluids in Porous Media. Elsevier, New York (1972)

    Google Scholar 

  • Benson, D.A., Wheatcraft, S.W., Meerschaert, M.M.: The fractional-order governing equation of Levy motion. Water Resour. Res. 36(6), 1413–1423 (2000)

    Article  Google Scholar 

  • Bijeljic, B., Blunt, M.J.: Pore-scale modeling and continuous time random walk analysis of dispersion in porous media. Water Resour. Res. 42, W01,202 (2006)

    Article  Google Scholar 

  • Bouchaud, J.P., Georges, A.: Anomalous diffusion in disordered media: statistical mechanisms, models and physical applications. Phys. Rep. 195(4,5), 127–293 (1990)

    Article  Google Scholar 

  • Bruna, M., Chapman, S.J.: Excluded-volume effects in the diffusion of hard spheres. Phys. Rev. E 85, 011–103 (2012). https://doi.org/10.1103/PhysRevE.85.011103

    Article  Google Scholar 

  • Bruna, M., Chapman, S.J.: Diffusion of finite-size particles in confined geometries. Bull. Math. Biol. 76, 947–982 (2014). https://doi.org/10.1007/s11538-013-9847-0

    Article  Google Scholar 

  • Burada, P.S., Hänggi, P., Marchesoni, F., Schmid, G., Talkner, P.: Diffusion in confined geometries. Chem. Phys. Chem. 10, 45–54 (2009). https://doi.org/10.1002/cphc.200800526

    Article  Google Scholar 

  • Carrera, J., Sanchez-Vila, X., Benet, I., Medina, A., Galarza, G., Guimera, J.: On matrix diffusion. Formulations, solutions methods and qualitative effects. Hydrogeol. J. 6(1), 178–190 (1998)

    Article  Google Scholar 

  • Cushman, J.H.: The Physics of Fluids in Hierarchical Porous Media: Angstroms to Miles. Kluwer Academic Publisher, New York (1997)

    Book  Google Scholar 

  • Cushman, J.H., Ginn, T.R.: Fractional advection–dispersion equation: a classical mass balance with convolution-Fickian flux. Water Resour. Res. 36(12), 3763–3766 (2000)

    Article  Google Scholar 

  • Cushman, J.H., Moroni, M.: Statistical mechanics with 3D-PTV experiments in the study of anomalous dispersion: part I. Theory. Phys. Fluids 13(1), 75–80 (2001)

    Article  Google Scholar 

  • Cushman, J.H., O’Malley, D.: Fickian dispersion is anomalous. J. Hydrol. 531, 161–167 (2015)

    Article  Google Scholar 

  • Cushman, J.H., O’Malley, D., Park, M.: Anomalous diffusion as modeled by a nonstationary extension of Brownian motion. Phys. Rev. E 79(3), 032–101 (2009). https://doi.org/10.1103/PhysRevE.79.032101

    Article  Google Scholar 

  • Cvetkovic, V., Cheng, H., Wen, X.H.: Analysis of nonlinear effects on tracer migration in heterogeneous aquifers using Lagrangian travel time statistics. Water Resour. Res. 32(6), 1671–1680 (1996)

    Article  Google Scholar 

  • Dagan, G.: Solute transport in heterogenous porous formations. J. Fluid Mech. 145, 151–177 (1984)

    Article  Google Scholar 

  • Dagan, G.: Flow and Transport in Porous Formations, 2nd edn. Springer, New York (2012)

    Google Scholar 

  • Dagan, G., Neuman, S.P. (eds.): Subsurface Flow and Transport: A Stochastic Approach. Cambridge, New York (1997)

    Google Scholar 

  • Delay, F., Bodin, J.: Time domain random walk method to simulate transport by advection–diffusion and matrix diffusion in fracture networks. Geophys. Res. Lett. 28, 4051–4054 (2001)

    Article  Google Scholar 

  • Dentz, M., Berkowitz, B.: Transport behavior of a passive solute in continuous time random walks and multirate mass transfer. Water Resour. Res. 39(5), 1111 (2003)

    Article  Google Scholar 

  • Dentz, M., Gouze, P., Russian, A., Dweik, J., Delay, F.: Diffusion and trapping in heterogeneous media: an inhomogeneous continuous time random walk approach. Adv. Water Resour. 49, 13–22 (2012)

    Article  Google Scholar 

  • Deshmukh, A., Elimelech, M.: Understanding the impact of membrane properties and transport phenomena on the energetic performance of membrane distillation desalination. J. Membr. Sci. 539, 458–474 (2017)

    Article  Google Scholar 

  • Dorfman, K.D., Yariv, E.: Assessing corrections to the Fick–Jacobs equation. J. Chem. Phys. 141, 044–118 (2014). https://doi.org/10.1063/1.4890740

    Article  Google Scholar 

  • Einstein, A.: Über die von der molekularkinetischen Theorie der Wärme geforderte Bewegung von in ruhenden Flüssigkeiten suspendierten Teilchen. Ann. Phys. 322, 549–560 (1905)

    Article  Google Scholar 

  • Fiori, A., Janković, I., Dagan, G., Cvetković, V.: Ergodic transport through aquifers of non? Gaussian log conductivity distribution and occurrence of anomalous behavior. Water Resour. Res. 43(9), W09,407 (2007). https://doi.org/10.1029/2007WR005976

    Article  Google Scholar 

  • Gardiner, C.: Stochastic Methods. Springer, Heidelberg (2010)

    Google Scholar 

  • Gouze, P., Melean, Z., Le Borgne, T., Dentz, M., Carrera, J.: Non-Fickian dispersion in porous media explained by heterogeneous microscale matrix diffusion. Water Resour. Res. 44, W11,416 (2008)

    Google Scholar 

  • Haggerty, R., Gorelick, S.M.: Multiple-rate mass transfer for modeling diffusion and surface reactions in media with pore-scale heterogeneity. Water Resour. Res. 31(10), 2383–2400 (1995)

    Article  Google Scholar 

  • Havlin, S., Ben-Avraham, D.: Diffusion in disordered media. Adv. Phys. 51, 187–292 (2002)

    Article  Google Scholar 

  • Jacobs, M.H.: Diffusion Processes. Springer, New York (1967)

    Book  Google Scholar 

  • Kane, J.J., Matthews, A.C., Orme, C.J., Contescu, C.I., Swank, W.D., Windes, W.E.: Effective gaseous diffusion coefficients of select ultra-fine, super-fine and medium grain nuclear graphite. Carbon 136, 369–379 (2018)

    Article  Google Scholar 

  • Kang, K., Redner, S.: Fluctuation-dominated kinetics in diffusion-controlled reactions. Phys. Rev. A 32, 435–447 (1985)

    Article  Google Scholar 

  • Kayser, R.F., Hubbard, J.B.: Diffusion in a random medium with a random distribution of static traps. Phys. Rev. Lett. 51, 79–82 (1983)

    Article  Google Scholar 

  • Kenkre, V.M., Montroll, E.W., Shlesinger, M.F.: Generalized master equations for continuous-time random walks. J. Stat. Phys. 9(1), 45–50 (1973)

    Article  Google Scholar 

  • Kennard, E.H.: Kinetic Theory of Gases. McGraw-Hill, New York (1938)

    Google Scholar 

  • Klinzing, G.R., Zavaliangos, A.: A simplified model of moisture transport in hydrophilic porous media with applications to pharmaceutical tablets. J. Pharamceutical Sci. 105(8), 2410–2418 (2016)

    Article  Google Scholar 

  • Koch, D.L., Brady, J.F.: Anomalous diffusion in heterogeneous porous media. Phys. Fluids 31(5), 965 (1988). https://doi.org/10.1063/1.866716

    Article  Google Scholar 

  • Kubo, R.: The fluctuation–dissipation theorem. Rep. Progr. Phys. 29(1), 255–284 (1966). https://doi.org/10.1088/0034-4885/29/1/306

    Article  Google Scholar 

  • Langevin, P.: Sur la théorie du mouvement brownien. C. R. Acad. Sci. (Paris) 146, 530–533 (1908)

    Google Scholar 

  • Metzler, R., Klafter, J.: The random walk’s guide to anomalous diffusion: a fractional dynamics approach. Phys. Rep. 339(1), 1–77 (2000)

    Article  Google Scholar 

  • Mitkov, I., Tartakovsky, D.M., Winter, C.L.: Dynamics of wetting fronts in porous media. Phys. Rev. E 58(5), 5245R–5248R (1998)

    Article  Google Scholar 

  • Montroll, E.W., Weiss, G.H.: Random walks on lattices. II. J. Math. Phys. 6(2), 167 (1965)

    Article  Google Scholar 

  • Morales-Casique, E., Neuman, S.P., Guadagnini, A.: Nonlocal and localized analyses of nonreactive solute transport in bounded randomly heterogeneous porous media: theoretical framework. Adv. Water Resour. 29(8), 1238–1255 (2006)

    Article  Google Scholar 

  • Moroni, M., Cushman, J.H.: Statistical mechanics with 3D-PTV experiments in the study of anomalous dispersion: part II. Experiment. Phys. Fluids 13(1), 81–91 (2001)

    Article  Google Scholar 

  • Narasimhan, T.N.: Fourier’s heat conduction equation: history, influence, and connections. Rev. Geophys. 37(1), 151–172 (1999)

    Article  Google Scholar 

  • Neuman, S.P.: Eulerian–Lagrangian theory of transport in space–time nonstationary velocity fields: exact nonlocal formalism by conditional moments and weak approximation. Water Resour. Res. 29(3), 633–645 (1993). https://doi.org/10.1029/92WR02306

    Article  Google Scholar 

  • Neuman, S.P., Tartakovsky, D.M.: Perspective on theories of anomalous transport in heterogeneous media. Adv. Water Resour. 32(5), 670–680 (2009). https://doi.org/10.1016/j.advwatres.2008.08.005

    Article  Google Scholar 

  • Neuman, S.P., Tartakovsky, D.M., Wallstrom, T.C., Winter, C.L.: Correction to the Neuman and Orr “Nonlocal theory of steady state flow in randomly heterogeneous media”. Water Resour. Res. 32(5), 1479–1480 (1996)

    Article  Google Scholar 

  • O’Malley, D., Cushman, J.H.: A renormalization group classification of nonstationary and/or infinite second moment diffusive processes. J. Stat. Phys. 146, 989–1000 (2012)

    Article  Google Scholar 

  • O’Malley, D., Vesselinov, V.V., Cushman, J.H.: A method for identifying diffusive trajectories with stochastic models. J. Stat. Phys. 156(5), 896–907 (2014)

    Article  Google Scholar 

  • Ovchinnikov, A.A., Zeldovich, Y.B.: Role of density fluctuations in bimolecular reaction kinetics. Chem. Phys. 28, 215–218 (1978)

    Article  Google Scholar 

  • Painter, S., Cvetkovic, V.: Upscaling discrete fracture network simulations: an alternative to continuum transport models. Water Resour. Res. 41(2) (2005). https://doi.org/10.1029/2004WR003682

  • Pfannkuch, H.O.: Contribution a l’étude des déplacements de fluides miscibles dans un milieux poreux. Rev. Inst. Fr. Petr. 18, 215–270 (1963)

    Google Scholar 

  • Picchi, D., Battiato, I.: The impact of pore-scale flow regimes on upscaling of immiscible two-phase flow in porous media. Water Resour. Res. 54(9), 6683–6707 (2018)

    Article  Google Scholar 

  • Picchi, D., Ullmann, A., Brauner, N.: Modelling of core-annular and plug flows of Newtonian/non-Newtonian shear-thinning fluids in pipes and capillary tubes. Int. J. Multiph. Flow 103, 43–60 (2018)

    Article  Google Scholar 

  • Qian, G., Morgan, N.T., Holmes, R.J., Cussler, E.L., Blaylock, D.W., Froese, R.D.J.: Sublimation as a function of diffusion. AIChE J. 62(3), 861–867 (2016)

    Article  Google Scholar 

  • Redner, S.: A Guide to First-Passage Processes. Cambridge University Press, Cambridge (2001)

    Book  Google Scholar 

  • Regner, B.M., Tartakovsky, D.M., Sejnowski, T.J.: Identifying transport behavior of single-molecule trajectories. Biophys. J. 107, 2345–2351 (2014). https://doi.org/10.1016/j.bpj.2014.10.005

    Article  Google Scholar 

  • Regner, B.M., Vucinic, D., Domnisoru, C., Bartol, T.M., Hetzer, M.W., Tartakovsky, D.M., Sejnowski, T.J.: Anomalous diffusion of single particles in cytoplasm. Biophys. J. 104, 1652–1660 (2013)

    Article  Google Scholar 

  • Risken, H.: The Fokker–Planck Equation. Springer, Heidelberg (1996)

    Book  Google Scholar 

  • Scher, H., Lax, M.: Stochastic transport in a disordered solid. I. Theory. Phys. Rev. B 7(1), 4491–4502 (1973)

    Article  Google Scholar 

  • Sutherland, W.: A dynamical theory of diffusion for non-electrolytes and the molecular mass of albumin. Phil. Mag. S. 9, 781–785 (1905)

    Article  Google Scholar 

  • Toussaint, D., Wilczek, F.: Particle–antiparticle annihilation in diffusive motion. J. Chem. Phys. 78, 2642–2647 (1983)

    Article  Google Scholar 

  • van der Pas, P.W.: The discovery of the Brownian motion. Scientiarum Historia 13, 27–35 (1971)

    Google Scholar 

  • von Smoluchowski, M.: Zur kinetischen Theorie der Brownschen Molekularbewegung und der Suspensionen. Ann. Phys. 21, 757–780 (1906)

    Google Scholar 

  • von Smoluchowski, M.: Versuch einer mathematischen Theorie der Koagulationskinetik kolloidaler Lösungen. Z. Phys. Chem. 92, 129–168 (1917)

    Google Scholar 

  • Zalc, J.M., Reyes, S.C., Iglesia, E.: The effects of diffusion mechanism and void structure on transport rates and tortuosity factors in complex porous structures. Chem. Eng. Sci. 59, 2947–2960 (2004). https://doi.org/10.1016/j.ces.2004.04.028

    Article  Google Scholar 

  • Zhang, X., Tartakovsky, D.M.: Effective ion diffusion in charged nanoporous materials. J. Electrochem. Soc. 164(4), E53–E61 (2017)

    Article  Google Scholar 

  • Zhang, X., Urita, K., Moriguchi, I., Tartakovsky, D.M.: Design of nanoporous materials with optimal sorption capacity. J. Appl. Phys. 117(24), 244–304 (2015). https://doi.org/10.1063/1.4923057

    Article  Google Scholar 

Download references

Acknowledgements

This research was supported in part by US National Science Foundation (NSF) under Award Number CBET-1606192 issued to DMT, and by the European Research Council (ERC) through the Project MHetScale (617511) issued to MD.

Author information

Authors and Affiliations

  1. Department of Energy Resources Engineering, Stanford University, 367 Panama Street, Stanford, CA, 94305, USA

    Daniel M. Tartakovsky

  2. Institute of Environmental Assessment and Water Research, Spanish National Research Council (CSIC), 08034, Barcelona, Spain

    Marco Dentz

Authors
  1. Daniel M. Tartakovsky
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Marco Dentz
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Daniel M. Tartakovsky.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Tartakovsky, D.M., Dentz, M. Diffusion in Porous Media: Phenomena and Mechanisms. Transp Porous Med 130, 105–127 (2019). https://doi.org/10.1007/s11242-019-01262-6

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11242-019-01262-6

Keywords

Mathematics Subject Classification

Search

Navigation