Weak quantum correlation quantifiers with generalized entropies

Abstract

We define a broad class of quantum correlation quantifiers (QCQs) based on generalized entropies and projective measurements and compare it with the case where the measurements are weak for a broad class of weak measurements (WMs) that operate in finite-dimensional systems. We also propose a general way to define a WM using a measure of disturbance and show that the QCQs by these measurements are majorized by those defined by the projective ones. Our result strengthens the hypothesis that WMs reveal fewer quantum correlations than projective measurements in a way that depends very little on the type of the QCQ. Finally, we prove that the quantifier is increasing with the force of the measurement and, as an example, we analytically calculate a particular case using the linear entropy.

Appendices

Proof of Theorem 1

For the proof, first we need the following lemma:

Lemma 1

If $\Arrowvert .\Arrowvert$ is a norm of $L({\mathcal {H}})$ invariant by unitary similarity transformations (UST), then $\sup _{\rho \in D_{P}}\Arrowvert \rho -P\Arrowvert =\sup _{\rho \in D_{Q}}\Arrowvert \rho -Q\Arrowvert$ for every rank-one projections P and Q, with $D_{P}\equiv \{\rho \in {\mathcal {D}}({\mathcal {H}}):{{\,\mathrm{Tr}\,}}(P\rho )\ne 0\}$ and $D_{Q}$ defined analogously.

Proof

Using the invariance by UST,

$$\begin{aligned} \Arrowvert \rho -P\Arrowvert =\Arrowvert U(\rho -P)U^{\dagger }\Arrowvert =\Arrowvert U\rho U^{\dagger }-UPU^{\dagger }\Arrowvert =\Arrowvert U\rho U^{\dagger }-Q\Arrowvert . \end{aligned}$$

(10)

The last equality follows from the fact that any rank-one projection has the form $P=|\psi \rangle \langle \psi |$ for some normalized state $|\psi \rangle$ and any normalized state defines a unique rank-one projection. Given any two state $|\phi \rangle$ and $|\psi \rangle$, there is a unitary operator U such that $|\phi \rangle =U|\psi \rangle$. So, given any two rank-one projections P and Q, there is a unitary operator U such that $Q=UPU^{\dagger }$.

Let ${\mathcal {R}}(P)\equiv \{\Arrowvert \rho -P\Arrowvert \in {\mathbb {R}}_{\ge 0}:\rho \in D_{P}\}$, so if $x\in {\mathcal {R}}(P)$, $\exists \rho :\Arrowvert \rho -P\Arrowvert =x$ and ${{\,\mathrm{Tr}\,}}(P\rho )\ne 0$, but $\Arrowvert \rho -P\Arrowvert =\Arrowvert U\rho U^{\dagger }-Q\Arrowvert$ for the unitary operator U and ${{\,\mathrm{Tr}\,}}(P\rho )={{\,\mathrm{Tr}\,}}(UPU^{\dagger }U\rho U^{\dagger })={{\,\mathrm{Tr}\,}}(QU\rho U^{\dagger })\ne 0\Rightarrow x\in {\mathcal {R}}(Q)\Rightarrow {\mathcal {R}}(P)\subseteq {\mathcal {R}}(Q)$. In the same way, if $y\in {\mathcal {R}}(Q)$, $\exists \rho :\Arrowvert \rho -Q\Arrowvert =y$ and ${{\,\mathrm{Tr}\,}}(Q\rho )\ne 0$, but $\Arrowvert \rho -Q\Arrowvert =\Arrowvert U^{\dagger }\rho U -U^{\dagger }QU\Arrowvert =\Arrowvert U^{\dagger }\rho U -P\Arrowvert$ and ${{\,\mathrm{Tr}\,}}(Q\rho )={{\,\mathrm{Tr}\,}}(U^{\dagger }QUU^{\dagger }\rho U)={{\,\mathrm{Tr}\,}}(PU^{\dagger }\rho U)\ne 0\Rightarrow y\in {\mathcal {R}}(P)\Rightarrow {\mathcal {R}}(Q)\subseteq {\mathcal {R}}(P)$, so ${\mathcal {R}}(P)={\mathcal {R}}(Q)\Rightarrow \sup _{\rho \in D_{P}}\Arrowvert \rho -P\Arrowvert =\sup _{\rho \in D_{Q}}\Arrowvert \rho -Q\Arrowvert$. $\square$

For Theorem 1, we will use the Frobenius norm, which is invariant by UST.

Proof of Theorem 1

With $\rho '\equiv M\rho M^{\dagger }/p_{M}$, by the Frobenius norm we have

$$\begin{aligned} \Arrowvert \rho -\rho '\Arrowvert ^{2}={{\,\mathrm{Tr}\,}}(\rho ^{2})+{{\,\mathrm{Tr}\,}}(\rho '^{2})-2{{\,\mathrm{Tr}\,}}(\rho \rho '). \end{aligned}$$

(11)

By Eq. (11), we have

$$\begin{aligned} \sup _{M}\sup _{\rho \in D_{M}}\Arrowvert \rho -M\rho M^{\dagger }/p_{M}\Arrowvert \le \sup _{\rho ,\rho ''\in D({\mathcal {H}})}\Arrowvert \rho -\rho ''\Arrowvert =\Arrowvert Q-P\Arrowvert =\sqrt{2},\langle Q,P\rangle =0, \end{aligned}$$

(12)

where P and Q are rank-one projections and $\langle Q,P\rangle \equiv {{\,\mathrm{Tr}\,}}(Q^{\dagger }P)={{\,\mathrm{Tr}\,}}(QP)$ is the trace inner product. For every value $\epsilon \in (0,1]$ it is possible to find a rank-one projection R such that $\langle R,P\rangle =\epsilon$. The number $\sqrt{2}$ is an upper bound for $\sup _{R:\langle R,P\rangle \ne 0}\Arrowvert R-P\Arrowvert$. Suppose there exists another upper bound $\delta <\sqrt{2}$. Then,

$$\begin{aligned} \Arrowvert R-P\Arrowvert = \sqrt{2-2\epsilon }\le \delta \Rightarrow \epsilon \ge 1-\delta ^2/2 >0 , \end{aligned}$$

but as $\epsilon \in (0,1]$, $\exists \epsilon \in \left( 0,1-\delta ^2 /2\right) :\sqrt{2-2\epsilon }>\delta ,$ what is an absurd. Thus, $\sqrt{2}$ is the least upper bound. Using Lemma 1, we conclude that

$$\begin{aligned} \sup _{M}\sup _{\rho \in D_{M}}\Arrowvert \rho -M\rho M^{\dagger }/p_{M}\Arrowvert =\sup _{\rho \in D_{P}}\Arrowvert \rho -P\Arrowvert =\sup _{R:\langle R,P\rangle \ne 0}\Arrowvert R-P\Arrowvert =\sqrt{2}. \end{aligned}$$

(13)

$\square$

Proof of Theorem 2

Proof

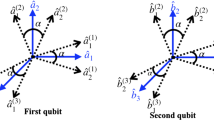
With $\varPhi _{B}(\rho )=\sum _{k=1}^{n}(\mathbb {1}_{A}\otimes P_{k})\rho (\mathbb {1}_{A}\otimes P_{k})$, $\dim ({\mathcal {H}}_{A})=m$ and $\dim ({\mathcal {H}}_{B})=n$, we have ${\mathfrak {M}}_{r,\varPhi _{B}}=\{M_{k}\}_{k=1}^{n}$ such that $M_{k}\equiv \sqrt{(1-r)/n}\mathbb {1}_{AB}+i\sqrt{r}\mathbb {1}_{A}\otimes P_{k}$ where $r\in (0,1)$. Then,

$$\begin{aligned} p_{k}\rho _{k}&=\frac{1-r}{n}\rho +i\sqrt{\frac{(1-r)r}{n}}[(\mathbb {1}_{A}\otimes P_{k}),\rho ]+r(\mathbb {1}_{A}\otimes P_{k})\rho (\mathbb {1}_{A}\otimes P_{k}),\nonumber \\ p_{k}&\equiv \frac{1-r}{n}+rq_{k},\quad q_{k}\equiv {{\,\mathrm{Tr}\,}}((\mathbb {1}_{A}\otimes P_{k})\rho ). \end{aligned}$$

(14)

Then,

$$\begin{aligned} \Vert \rho -\rho _{k}\Vert \le&\bigg \Vert \bigg (1-\frac{(1-r)}{np_{k}}\bigg )\rho +\frac{r}{p_{k}}(\mathbb {1}_{A}\otimes P_{k})\rho (\mathbb {1}_{A}\otimes P_{k})\bigg \Vert \nonumber \\&+\frac{1}{p_{k}}\sqrt{\frac{(1-r)r}{n}}\Vert (\mathbb {1}_{A}\otimes P_{k})\rho -\rho (\mathbb {1}_{A}\otimes P_{k})\Vert \end{aligned}$$

(15)

Let the first term in the right side of the inequality be Q and the second term be $Q^{\prime }$. We can write $\rho =\sum _{l}\lambda _{l}|l\rangle \langle l|$, with $|l\rangle =\sum _{i,j}\psi ^{(l)}_{i,j}|a_{i}\rangle |b_{j}\rangle$, where $\{|l\rangle \}$ is an orthonormal basis. Then,

$$\begin{aligned} Q&\le \bigg \arrowvert 1-\frac{1-r}{np_{k}}\bigg \arrowvert \Vert \rho \Vert +\frac{r}{p_{k}}\Vert (\mathbb {1}_{A}\otimes P_{k})\rho (\mathbb {1}_{A}\otimes P_{k})\Vert , \end{aligned}$$

(16)

$$\begin{aligned} \Vert (\mathbb {1}_{A}\otimes P_{k})\rho (\mathbb {1}_{A}\otimes P_{k})\Vert&\le \sum _{l}\sum _{i,i'}\lambda _{l}|\psi ^{(l)}_{i,k}||\psi ^{(l)}_{i',k}|\Vert |a_{i}\rangle \langle a_{i'}|\otimes |b_{k}\rangle \langle b_{k}|\Vert \nonumber \\&\le \sum _{l}\sum _{i,i'}\Vert |a_{i}\rangle \langle a_{i'}|\otimes |b_{k}\rangle \langle b_{k}|\Vert \nonumber \\&=\sum _{l}\sum _{i,i'}\Vert U_{A}|a_{i}\rangle \langle a_{i'}|U^{\dagger }_{A}\otimes U_{B}|b_{k}\rangle \langle b_{k}|U^{\dagger }_{B}\Vert \nonumber \\&\le n(m)^{3}\alpha \end{aligned}$$

(17)

where $P_{k}\equiv |b_{k}\rangle \langle b_{k}|$, $\alpha \equiv \max _{i,i',k}\{\Vert |a_{i}\rangle \langle a_{i'}|\otimes |b_{k}\rangle \langle b_{k}|\Vert \}$. The value of $\alpha$ is the same for any orthonormal basis of ${\mathcal {H}}_{A}$ and ${\mathcal {H}}_{B}$ by the UST invariance of the norm, which implies that it does not depend on the choice of $\varPhi _{B}$. Now, we treat another term in inequality (16):

$$\begin{aligned} \frac{1-r}{np_{k}}=\frac{1-r}{1-r+nrq_{k}}\le 1\Rightarrow \bigg \arrowvert 1-\frac{1-r}{np_{k}}\bigg \arrowvert \le \frac{nr}{1-r}. \end{aligned}$$

(18)

We also have $\Vert \rho \Vert \le \max \{\Vert |l\rangle \langle l|\Vert \}\equiv \beta$, which is valid for any $\rho$ by the UST invariance of the norm. By (17), (18) in (16),

$$\begin{aligned} Q\le \bigg (n\beta +n^2 m^3\alpha \bigg )\frac{r}{1-r}. \end{aligned}$$

(19)

We also are going to find an upper bound for $Q'$. Doing calculations similar to what was done in (17) for $\Vert (\mathbb {1}_{A}\otimes P_{k})\rho -\rho (\mathbb {1}_{A}\otimes P_{k})\Vert$, we conclude that this term has a nonzero finite upper bound $\gamma$ for any $\rho$ and $\varPhi _{B}$. Then,

$$\begin{aligned} Q'\le \sqrt{\frac{nr}{1-r}}\gamma . \end{aligned}$$

(20)

Considering arbitrary $\epsilon >0$ and the inequalities (19) and (20), we have

$$\begin{aligned}&r<\delta _{1}(\epsilon )\equiv \frac{\epsilon /3}{n\beta +\epsilon /3}\Rightarrow \frac{n\beta r}{1-r}<\epsilon /3.\nonumber \\&r<\delta _{2}(\epsilon )\equiv \frac{\epsilon /3}{n^2 m^3 \alpha +\epsilon /3}\Rightarrow \frac{n^2 m^3 \alpha r}{1-r}<\epsilon /3.\nonumber \\&r<\delta _{3}(\epsilon )\equiv \frac{(\epsilon /3)^2}{n\gamma ^2+(\epsilon /3)^2}\Rightarrow \sqrt{\frac{nr}{1-r}}\gamma <\epsilon /3. \end{aligned}$$

(21)

Using (19), (20) and (21) in (15), we have

$$\begin{aligned} \forall \epsilon >0, \exists \epsilon ''\in (0,\epsilon ),\delta '\equiv \min \{\delta _{k}(\epsilon '')\}_{k=1}^{3}: r\in (0,\delta ')\Rightarrow \sup _{\rho \in D_{k}}\Vert \rho -\rho _{k}\Vert \le \epsilon ''<\epsilon . \end{aligned}$$

(22)

As the result in (22) is valid for any k, we have $(1-\sum _{k}p_{k})=0$, which implies that for any $\delta >0$ and $\epsilon >0$, there exists a $(\epsilon ,\delta )$-WM set that belongs to the family. $\square$

Proof of Theorem 4

Lemma 2

The set $C_{\rho }^{B}\equiv \{\varPhi _{B}(\rho )\in D({\mathcal {H}}_{AB}):\varPhi _{B}\in \varGamma ^{P}_{B}\}$ is compact $\forall \rho \in D({\mathcal {H}}_{AB})$ as a topological subspace of $L({\mathcal {H}}_{AB})$.

Proof

Consider the topology of the normed vector spaces $L({\mathcal {H}}_{AB})$ and $L({\mathcal {H}}_{B})$ induced by the Frobenius norms $\Arrowvert . \Arrowvert _{AB}$ and $\Arrowvert . \Arrowvert _{B}$. With $\dim ({\mathcal {H}}_{B})=n$, we have $U(n)\subset L({\mathcal {H}}_{B})$ as the topological subspace of unitary operators. The set $C^{B}_{\rho }\subset L({\mathcal {H}}_{B})$ also has the subspace topology. The projection $P_{k}$ has the form $P_{k}=|k\rangle \langle k|$, where $\{|1\rangle ,\dots ,|n\rangle \}$ is an orthonormal basis of ${\mathcal {H}}_{B}$. Any other orthonormal basis is obtained applying an unitary operator U so, fixing the set of projections, any map $\varPhi _{B}\in \varGamma ^{P}_{B}$ has the form $\varPhi _{B}(X)=\sum _{k=1}^{n}(\mathbb {1}_{A}\otimes UP_{k}U^{\dagger })X(\mathbb {1}_{A}\otimes UP_{k}U^{\dagger })$ for some U. Let $F_{\rho }:U(n)\rightarrow D({\mathcal {H}}_{AB})$ be a function such that $F_{\rho }(U)=\sum _{k=1}^{n}(\mathbb {1}_{A}\otimes UP_{k}U^{\dagger })\rho (\mathbb {1}_{A}\otimes UP_{k}U^{\dagger })$ for $\rho \in D({\mathcal {H}}_{AB})$ and for a fixed set of projections. We prove that this function is continuous. Let $U, V\in U(n)$ and $Z\equiv U-V\Rightarrow U=Z+V$. Using the Frobenius norm, $\Arrowvert I\otimes Y\Arrowvert =\sqrt{n}\Arrowvert Y\Arrowvert$. Defining $\varDelta \equiv \Arrowvert F_{\rho }(U)-F_{\rho }(V)\Arrowvert$, we have

(23)

By the fact that $\Arrowvert V\Arrowvert =\sqrt{n}$, $\Arrowvert P_{k}\Arrowvert =1$, $\Arrowvert \rho \Arrowvert \le 1$ and $\Arrowvert Z\Arrowvert \le 2\sqrt{n}$, it is easy to see that the sum in the right side of the inequality has an upper bound $\alpha$ such that:

$$\begin{aligned} \forall \epsilon>0,\exists \delta \equiv \frac{\epsilon }{n\alpha }>0:\Arrowvert U-V\Arrowvert<\delta \Rightarrow \Arrowvert F_{\rho }(U)-F_{\rho }(V)\Arrowvert <\epsilon . \end{aligned}$$

(24)

By the proposition (24), we conclude that $F_{\rho }$ is continuous for any $\rho$ and at any point $V\in U(n)$. By the fact that U(n) is a compact space, $F_{\rho }(U(n))=C^{B}_{\rho }$ is also compact for any $\rho$. $\square$

Corollary 1

The topological subspace $C^{B}_{r, \rho }\equiv \{(1-r)\rho +r\varPhi _{B}(\rho ):\varPhi _{B}\in \varGamma ^{P}_{B}\}$ is compact $\forall r\in (0,1)$ and $\forall \rho \in D({\mathcal {H}}_{AB})$.

These results are necessary to guarantee that the infimum occurs at a minimum point inside the set ($C^{B}_{\rho }$ or $C^{B}_{w, \rho }$). Now, we show that our quantifiers are invariant by local UST:

Proof of Theorem 4

Using the property that S is invariant by UST,

$$\begin{aligned} S\circ \varPhi _{B}(U_{A}\otimes U_{B}\rho U^{\dagger }_{A}\otimes U^{\dagger }_{B})&=S((U_{A}\otimes \mathbb {1}_{B})\varPhi _{B}(\mathbb {1}_{A}\otimes U_{B}\rho \mathbb {1}_{A}\otimes U^{\dagger }_{B})(U^{\dagger }_{A}\otimes \mathbb {1}_{B}))\nonumber \\&=S\circ \varPhi _{B}(\mathbb {1}_{A}\otimes U_{B}\rho \mathbb {1}_{A}\otimes U^{\dagger }_{B})\nonumber \\&=S\left( \sum _{k}(\mathbb {1}_{A}\otimes U^{\dagger }_{B}P_{k}U_{B})\rho (\mathbb {1}_{A}\otimes U^{\dagger }_{B}P_{k}U_{B})\right) \nonumber \\&=S\left( \sum _{k}\mathbb {1}_{A}\otimes Q_{k}\rho \mathbb {1}_{A}\otimes Q_{k}\right) =S\circ \varPhi '_{B}(\rho ), \end{aligned}$$

(25)

where $\{Q_{K}\}$ is a set of rank-one orthogonal projections and $\varPhi '_{B}(\rho )$ is the result of the non-selective projective measurement. By Lemma 2, there is $\varPhi ''_{B}$ and $\varPhi '''_{B}$ such that $\inf _{\varPhi _{B}}S\circ \varPhi _{B}(\rho )=S\circ \varPhi ''_{B}(\rho )$ and $\inf _{\varPhi _{B}}S\circ \varPhi _{B}(U_{A}\otimes U_{B}\rho U^{\dagger }_{A}\otimes U^{\dagger }_{B})=S\circ \varPhi '''_{B}(U_{A}\otimes U_{B}\rho U^{\dagger }_{A}\otimes U^{\dagger }_{B})$. So, for $\varPhi ''_{B}$ and $\varPhi '''_{B}$ exist ${\tilde{\varPhi }}_{B}$ and ${\tilde{\varPhi }}'_{B}$ such that

$$\begin{aligned} \inf _{\varPhi _{B}}S\circ \varPhi _{B}(U_{A}\otimes U_{B}\rho U^{\dagger }_{A}\otimes U^{\dagger }_{B})&=S\circ \varPhi '''_{B}(U_{A}\otimes U_{B}\rho U^{\dagger }_{A}\otimes U^{\dagger }_{B})=S\circ {\tilde{\varPhi }}'_{B}(\rho )\nonumber \\&\ge \inf _{\varPhi _{B}}S\circ \varPhi _{B}(\rho ), \end{aligned}$$

(26)

and

$$\begin{aligned} \inf _{\varPhi _{B}}S\circ \varPhi _{B}(\rho )&=S\circ \varPhi ''_{B}(\rho )=S\circ {\tilde{\varPhi }}_{B}(U_{A}\otimes U_{B}\rho U^{\dagger }_{A}\otimes U^{\dagger }_{B})\nonumber \\&\ge \inf _{\varPhi _{B}}S\circ \varPhi _{B}(U_{A}\otimes U_{B}\rho U^{\dagger }_{A}\otimes U^{\dagger }_{B}). \end{aligned}$$

(27)

Now, using Eqs.(26) and (27), we conclude that

$$\begin{aligned} \inf _{\varPhi _{B}}S\circ \varPhi _{B}(U_{A}\otimes U_{B}\rho U^{\dagger }_{A}\otimes U^{\dagger }_{B})&=\inf _{\varPhi _{B}}S\circ \varPhi _{B}(\rho ) \Rightarrow \nonumber \\ S\circ \varPhi '''_{B}(U_{A}\otimes U_{B}\rho U^{\dagger }_{A}\otimes U^{\dagger }_{B})&=S\circ \varPhi ''_{B}(\rho ). \end{aligned}$$

(28)

From Eq. (28) and using the fact that S is invariant by UST, we have

$$\begin{aligned} I_{p}(U_{A}\otimes U_{B}\rho U^{\dagger }_{A}\otimes U^{\dagger }_{B})&=S\circ \varPhi '''_{B}(U_{A}\otimes U_{B}\rho U^{\dagger }_{A}\otimes U^{\dagger }_{B})-S(\rho )\nonumber \\&=S\circ \varPhi ''_{B}(\rho )-S(\rho )\nonumber \\&=I_{p}(\rho ). \end{aligned}$$

(29)

The proof for $I^{r}_{w}$ is similar. $\square$

Proofs of Theorems 5 and 6

Proof of Theorem 5

First, we prove a short result:

$$\begin{aligned} {{\,\mathrm{Tr}\,}}((\varPhi _{B}(\rho ))^{2})&={{\,\mathrm{Tr}\,}}\left( \sum _{k,l}(\mathbb {1}_{A}\otimes P_{k})\rho (\mathbb {1}_{A}\otimes P_{k}) (\mathbb {1}_{A}\otimes P_{l})\rho (\mathbb {1}_{A}\otimes P_{l})\right) \nonumber \\&={{\,\mathrm{Tr}\,}}\left( \sum _{k}(\mathbb {1}_{A}\otimes P_{k})\rho (\mathbb {1}_{A}\otimes P_{k})\rho \right) ={{\,\mathrm{Tr}\,}}(\varPhi _{B}(\rho )\rho ). \end{aligned}$$

(30)

By Corollary 1, if $I_{w}^{r}(\rho )=0$, $\exists {\mathcal {M}}^{r}_{B}:S\circ {\mathcal {M}}^{r}_{B}(\rho )=S(\rho )$. With $\rho ^{\prime }\equiv {\mathcal {M}}^{r}_{B}(\rho )$, we have $\rho ^{\prime }\prec \rho$. If $\rho ^{\prime }\ne U\rho U^{\dagger }$ for any unitary U, then at least one of the inequalities at (1) is strict, which implies that $S(\rho ^{\prime })>S(\rho )$, because S is strictly SC. This is an absurd, so $\rho ^{\prime }=U\rho U^{\dagger }$ for some unitary U. So, we have

$$\begin{aligned} (1-r)\rho +r\varPhi _{B}(\rho )&=U\rho U^{\dagger }\Rightarrow (1-r)^{2}\rho ^{2}+r^{2}(\varPhi _{B}(\rho ))^{2}+(1-r)r[\rho \varPhi _{B}(\rho )\nonumber \\&\quad +\varPhi _{B}(\rho )\rho ] =U\rho ^{2}U^{\dagger }. \end{aligned}$$

(31)

Using Eq. (30) in (31) and applying the trace function,

$$\begin{aligned} (-2r+r^{2}){{\,\mathrm{Tr}\,}}(\rho ^{2})-(-2r+r^{2}){{\,\mathrm{Tr}\,}}(\varPhi _{B}(\rho )\rho )&=0 \Rightarrow {{\,\mathrm{Tr}\,}}(\rho ^{2})={{\,\mathrm{Tr}\,}}(\varPhi _{B}(\rho )\rho )\nonumber \\&={{\,\mathrm{Tr}\,}}((\varPhi _{B}(\rho ))^{2}). \end{aligned}$$

(32)

By the Cauchy–Schwarz inequality applied to the trace inner product,

$$\begin{aligned} {{\,\mathrm{Tr}\,}}(\varPhi _{B}(\rho )\rho )^{2}&={{\,\mathrm{Tr}\,}}(\rho ^{2}){{\,\mathrm{Tr}\,}}(\varPhi _{B}(\rho )^{2})\Rightarrow \rho =\lambda \varPhi _{B}(\rho )\Rightarrow {{\,\mathrm{Tr}\,}}(\rho )=\lambda {{\,\mathrm{Tr}\,}}(\varPhi _{B}(\rho ))\nonumber \\&\Rightarrow \lambda =1. \end{aligned}$$

(33)

So, $\rho =\varPhi _{B}(\rho )$. The same result applies when $I_{p}(\rho )=0$. $\square$

Proof of Theorem 6

Let $\{P_{k}\}$ be a set of rank-one orthogonal projections that acts on ${\mathcal {H}}_{B}$, $\dim ({\mathcal {H}}_{B})=n$, such that $\sum _{k}P_{k}=\mathbb {1}_{B}$. Let $F:U(n)\times D({\mathcal {H}}_{AB})\rightarrow D({\mathcal {H}}_{AB})$ be a function such that $F(U,\rho )\equiv \sum _{k}(\mathbb {1}_{A}\otimes UP_{k}U^{\dagger })\rho (\mathbb {1}_{A}\otimes UP_{k}U^{\dagger })$. We can define a metric d on $U(n)\times D({\mathcal {H}}_{AB})$ as $d((U,\rho ),(V,\sigma ))=\Arrowvert U-V\Arrowvert +\Arrowvert \rho -\sigma \Arrowvert$. The norm used is the Frobenius norm. Set $W\equiv U-V$ and $\alpha \equiv \rho -\sigma$. We have

(34)

By the fact that $\Arrowvert V\Arrowvert =\sqrt{n}$, $\Arrowvert P_{k}\Arrowvert =1$, $\Arrowvert \sigma \Arrowvert \le 1$, $\Arrowvert \alpha \Arrowvert \le 2$ and $\Arrowvert W\Arrowvert \le 2\sqrt{n}$, it is easy to see that the sums in the right side of inequality have upper bound $\beta$ such that:

$$\begin{aligned} \forall \epsilon>0,\exists \delta \equiv \frac{\epsilon }{2n\beta }>0:d((U,\rho ),(V,\sigma ))<\delta&\Rightarrow \Arrowvert W\Arrowvert<\delta , \Arrowvert \alpha \Arrowvert<\delta \nonumber \\&\Rightarrow \Arrowvert F(U,\rho )-F(V,\sigma )\Arrowvert <\epsilon . \end{aligned}$$

(35)

So, F is a continuous function. Let $S':U(n)\times D({\mathcal {H}}_{AB})\rightarrow {\mathbb {R}}$ be a function such that $S'(U,\rho )=S(\rho )\quad \forall U\in U(n)$. The function S is continuous, so $S'$ is a continuous function and, therefore, $J\equiv S\circ F-S'$ is continuous. The set U(n) is compact, so the function $I_{p}(\rho )=\inf _{U\in U(n)}J(U,\rho )$ is also continuous. The proof that $I_{w}^{r}$ is continuous is similar and it will be omitted. $\square$

Proof of Theorem 8

Lemma 3

$\forall r^{\prime }<r$, with $r^{\prime },r\in (0,1), \exists r''\in (0,1):{\mathcal {M}}^{r}_{B}={\mathcal {M}}^{r''}_{B}\circ {\mathcal {M}}^{r'}_{B}$.

Proof

We have

$$\begin{aligned} {\mathcal {M}}^{r}_{B}&={\mathcal {M}}^{r''}_{B}\circ {\mathcal {M}}^{r'}_{B}(\rho )=(1-r')(1-r'')\rho +[(1-r'')r'+(1-r')r''\nonumber \\&\quad +r'r'']\varPhi _{B}(\rho ). \end{aligned}$$

(36)

We want to find $r''$ such that ${\mathcal {M}}^{r}_{B}={\mathcal {M}}^{r''}_{B}\circ {\mathcal {M}}^{r'}_{B}$. We can do it by finding a solution for the equations:

$$\begin{aligned}&1-r=(1-r')(1-r'')\nonumber \\&r=(1-r'')r'+(1-r')r''+r'r''. \end{aligned}$$

(37)

Considering $r^{\prime }<r$ and $r^{\prime },r\in (0,1)$, the solution is

$$\begin{aligned} r''=1-(1-r)/(1-r'), r''\in (0,1). \end{aligned}$$

(38)

$\square$

Now, the proof of Theorem 8:

Proof

From Lemma 3 and by the non-decreasing property of S by the application of a CPTP unital map,

$$\begin{aligned} S\circ {\mathcal {M}}^{r''}_{B}\circ {\mathcal {M}}^{r'}_{B}(\rho )=S\circ {\mathcal {M}}^{r}_{B}(\rho )\ge S\circ {\mathcal {M}}^{r'}_{B}(\rho )\Rightarrow I_{w}^{r}(\rho )\ge I_{w}^{r^{\prime }}(\rho ). \end{aligned}$$

(39)

$\square$