Skip to main content
SpringerLink
Log in

A minimal three-term chaotic flow with coexisting routes to chaos, multiple solutions, and its analog circuit realization

  • Published:
Analog Integrated Circuits and Signal Processing Aims and scope Submit manuscript

Abstract

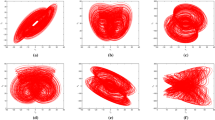
We introduce a unique chaotic flow with three terms expressed by \( \dddot x + abx = d \sinh \left( {\dot{x} + c\ddot{x}} \right) \). This minimal Jerk model is presented as a novel 3-D chaotic system consisting uniquely of two linear terms and single hyperbolic sine nonlinearity. The presence of coexisting routes to chaos in the numerical studies justifies the appearance of multiple solutions in some ranges of parameters. For instance, up to six different coexisting solutions (a pair of period-5 limit cycles and two pairs of chaotic attractors) have been tracked. Furthermore, the generalized form of the introduced Jerk system is synthesized with a parametric nonlinearity of the form \( \phi_{k} \left( X \right) = 0.5\left( {exp\left( {k\left( {\dot{x} + c\ddot{x}} \right)} \right) - exp\left( { - \left( {\dot{x} + c\ddot{x}} \right)} \right)} \right) \). Based on this generalized form, and judiciously adjusting the parameter \( k \), an in-depth description of the dynamics of the system at the symmetry boundaries is carried out. Hysteresis and parallel bifurcation behaviors reflect the presence of multiple asymmetric solutions (e.g. the coexistence of four asymmetric attractors) in the new model. It should be mention that the presence of six coexisting attractors reported in this work is rarely shown in third-order systems and therefore represents an enriching contribution to the study of dynamic systems in general. Finally, some PSpice simulations and laboratory tests of the proposed circuit are included.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16

Similar content being viewed by others

References

  1. Leonov, G. A., & Kuznetsov, N. V. (2013). Hidden attractors in dynamical systems. From hidden oscillations in Hilbert-Kolmogorov, Aizerman, and Kalman problems to hidden chaotic attractor in Chua circuits. International Journal of Bifurcation and Chaos,23(1), 1330002.

    Article  MathSciNet  MATH  Google Scholar 

  2. Pham, V. T., Volos, C., Kingni, S. T., Jafari, S., & Kapitaniak, T. (2016). Coexistence of hidden chaotic attractors in a novel no-equilibrium system. Nonlinear Dynamics,87(3), 2001–2010.

    Article  Google Scholar 

  3. Li, C., Sprott, J. C., & Xing, H. (2016). Crisis in amplitude control hides in multistability. International Journal of Bifurcation and Chaos,26(14), 1650233.

    Article  MathSciNet  MATH  Google Scholar 

  4. Li, C., Sprott, J. C., Akgul, A., Lu, H. H. C., & Zhao, Y. (2017). A new chaotic oscillator with free control. CHAOS,27, 083101.

    Article  MathSciNet  MATH  Google Scholar 

  5. Volos, C., Akgul, A., Pham, V.-T., Stouboulos, I., & Kyprianidis, I. (2017). A simple chaotic circuit with a hyperbolic sine function and its use in a sound encryption scheme. Nonlinear Dynamics,89, 1047–1061.

    Article  Google Scholar 

  6. Fozin, T., Srinivasan, K., Kengne, J., & Pelap, F. B. (2018). Coexisting bifurcations in a memristive hyperchaotic oscillator. International Journal of Electroninics and Communication,90, 110–122.

    Article  Google Scholar 

  7. Njitacke, Z. T., & Kengne, J. (2018). Complex dynamics of a 4D Hopfield neural networks (HNNs) with a nonlinear synaptic weight: Coexistence of multiple attractors and remerging Feigenbaum trees. International Journal of Electroninics and Communication.,93, 242–252.

    Article  Google Scholar 

  8. Kengne, R., Tchitnga, R., Mabekou, S., Wafo Tekam, B. R., Blondeau Soh, G. B., & Fomethe, A. (2018). On the relay coupling of three fractional-order oscillators with time-delay consideration: Global and cluster synchronizations. Chaos, Solitons & Fractals,111, 6–17.

    Article  MathSciNet  MATH  Google Scholar 

  9. Li, C., Sprott, J. C., Hu, W., & Xu, Y. (2017). Infinite multistability in a self-reproducing chaotic system. International Journal of Bifurcation and Chaos,27(10), 1750160.

    Article  MathSciNet  MATH  Google Scholar 

  10. Wang, Z., Abdolmohammadi, H. R., Alsaadi, F. E., Hayat, T., & Pham, V.-T. (2018). A new oscillator with infinite coexisting asymmetric attractors. Chaos, Solitons & Fractals,110, 252–258.

    Article  MathSciNet  MATH  Google Scholar 

  11. Njitacke, Z. T., Kengne, J., Wafo Tapche, R., & Pelap, F. B. (2018). Uncertain destination dynamics of a novel memristive 4D autonomous system. Chaos, Solitons & Fractals,107, 177–185.

    Article  MathSciNet  MATH  Google Scholar 

  12. Sprott, J. C. (2011). A proposed standard for the publication of new chaotic systems. International Journal of Bifurcation and Chaos,21(9), 2393–2394.

    Article  Google Scholar 

  13. Munmuangsaen, B., Srisuchinwong, B., & Sprott, J. C. (2011). Generalization of the simplest autonomous chaotic system. Physics Letters,375, 1445–1450.

    Article  MATH  Google Scholar 

  14. Rajagopal, K., Jafari, S., Akgul, A., Karthikeyan, A., Çiçek, S., & Shekofteh, Y. (2018). A simple snap oscillator with coexisting attractors, its time-delayed form, physical realization, and communication designs. A Journal of Physical Sciences, 73(5), 385–398.

    Google Scholar 

  15. Nguomkam Negou, A., & Kengne, J. (2018). Dynamic analysis of a unique jerk system with a smoothly adjustable symmetry and nonlinearity: Reversals of period doubling, offset boosting and coexisting bifurcations. International Journal of Electronics and Communications (AEÜ),90, 1–19.

    Article  Google Scholar 

  16. Sprott, J. C. (1997). Simplest dissipative chaotic flow. Physics Letters A,228, 271–274.

    Article  MathSciNet  MATH  Google Scholar 

  17. Wolf, A., Swift, J. B., Swinney, H. L., & Wastano, J. A. (1985). Determining Lyapunov exponents from time series. Physica D: Nonlinear Phenomena,16, 285–317.

    Article  MathSciNet  MATH  Google Scholar 

  18. Kyamakya, K., Mathis, W., Stoop, R., Chedjou, J. C., & Li, Z. (2018). Recent advantages in nonlinear dynamic and synchronization, studies in systems, decision and control (Vol. 109). New York: Springer.

    Book  MATH  Google Scholar 

  19. Germain, R. M., Mayfield, M. M., & Gilbert, B. (2018). The ‘filtering’ metaphor revisited: competition and environment jointly structure invasibility and coexistence. Biology Letters,14, 20180460.

    Article  Google Scholar 

  20. Nayfeh, A. H., & Balachandran, B. (1995). Applied nonlinear dynamics: Analytical, computational and experimental methods. New York: John Wiley & Sons.

    Book  MATH  Google Scholar 

  21. Broer Henk. W., Krauskopf, B., & Vegter, G. (2001). Global analysis of dynamical systems. IOP Publishing Ltd. ISBN 0 7503 0803 6.

  22. Strogatz, S. H. (1994). Nonlinear dynamics and chaos. Reading: Addison-Wesley.

    Google Scholar 

  23. Hogan, J., Champneys, A., Krauskopf, B., Bernardo, M. D., Osinga, H. M., & Homer, M. (2003). Nonlinear dynamics and chaos: Where do we go from here?. Bristol: IOP Publishing Ltd.

    Book  MATH  Google Scholar 

  24. Leutcho, G. D., Kengne, J., & Kengnou Telem, A. N. (2018). Reversals of period doubling, coexisting multiple attractors, and offset boosting in a novel memristive diode bridge-based hyperjerk circuit. Analog Integrated Circuits and Signal Processing. https://doi.org/10.1007/s10470-018-1372-5.

    Article  Google Scholar 

  25. Kengne, J., Tsafack, N., & Kamdjeu, K. L. (2018). Dynamical analysis of a novel single Opamp-based autonomous LC oscillator: Antimonotonicity, chaos, and multiple attractors. International Journal of Dynamics and Control. https://doi.org/10.1007/s40435-018-0414-92.

    Article  MathSciNet  Google Scholar 

  26. Sprott, J. C. (2015). Symmetric time-reversible flows with a strange attractor. International Journal of Bifurcation and Chaos,25(5), 1550078.

    Article  MathSciNet  MATH  Google Scholar 

  27. Prousalis, D. A., Volos, C. K., Stouboulos, L. N., & Kyprianidis, L. M. (2018). Extreme multi-stability in hyperjerk memristive system with hidden attractors and its adaptive synchronisation scheme. International Journal of Simulation and Process Modelling. https://doi.org/10.1504/IJSPM.2018.094737.

    Article  Google Scholar 

  28. Vaidyanathan, S., Jafari, S., Pham, V.-T., Azar, A. T., & Alsaadi, F. E. (2018). A 4-D chaotic hyperjerk system with a hidden attractor, adaptive backstepping control and circuit design. Archives of Control Sciences,28(2), 239–254.

    Google Scholar 

  29. Vaidyanathan, S., Akgul, A., Kaçar, S., et al. (2018). A new 4-D chaotic hyperjerk system, its synchronization, circuit design and applications in RNG, image encryption and chaos-based steganography. The European Physical Journal Plus,133, 46. https://doi.org/10.1140/epjp/i2018-11872-8.

    Article  Google Scholar 

  30. Wang, X., Akgul, A., Kacar, S., & Pham, V.-T. (2017). Multimedia security application of a ten-term chaotic system without equilibrium. Complexity, 8412093.

  31. Wang, Z., Cang, S., Ochola, E. O., & Sun, Y. (2012). A hyperchaotic system without equilibrium. Nonlinear Dynamics,69, 531–537.

    Article  MathSciNet  Google Scholar 

  32. Zhao, H., Lin, Y., & Dai, Y. (2014). Attractors and dynamics of a general autonomous van der Pol-Duffing Oscillator. International Journal of Bifurcation and Chaos,24(6), 1450080.

    Article  MathSciNet  MATH  Google Scholar 

  33. Wang, X., & Chen, G. (2012). A chaotic system with only one stable equilibrium. Communications in Nonlinear Science and Numerical Simulation,17, 1264–1272.

    Article  MathSciNet  Google Scholar 

  34. Jafari, S., & Sprott, J. C. (2013). Simple chaotic flows with a line equilibrium. Chaos, Solitons & Fractals,57(79), 84.

    MathSciNet  MATH  Google Scholar 

  35. Wei, Z. (2011). Dynamical behaviors of a chaotic system with no equilibria. Physics Letters A,376, 102–108.

    Article  MathSciNet  MATH  Google Scholar 

  36. Bragin, V. O., Vagaitsev, V. I., Kuznetsov, N. V., & Leonov, G. A. (2011). Algorithms for finding hidden oscillations in nonlinear systems. The Aizerman and Kalman conjectures and Chua’s circuits. Journal of Computer and System Sciences International,50, 511–543.

    Article  MATH  Google Scholar 

  37. Ren, S., Panahi, S., Rajagopal, K., Akgul, A., Pham, V.-T., & Jafari, S. (2018). A new chaotic flow with hidden attractor: The first hyperjerk system with no equilibrium. Z. Naturforsch. https://doi.org/10.1515/zna-2017-0409.

    Article  Google Scholar 

  38. Nguomkam Negou, A., Kengne, J., & Tchiotsop, D. (2018). Periodicity, chaos and multiple coexisting attractors in a generalized Moore-Spiegel system. Chaos, Solitons & Fractals,107, 275–289.

    Article  MathSciNet  MATH  Google Scholar 

  39. Munmuangsaen, B., Sprott, J. C., Thio, W. J.-C., Buscarino, A., Fortuna, L., & Srisuchinwong, B. (2015). A simple chaotic flow with a continuously adjustable attractor dimension. International Journal of Bifurcation and Chaos,25(12), 1530036.

    Article  MathSciNet  MATH  Google Scholar 

  40. Sprott, J. C. (2000). Simple chaotic systems and circuits. American Journal of Physics,68, 758–763.

    Article  Google Scholar 

  41. Leutcho, G. D., Kengne, J., & Kamdjeu Kengne, L. (2018). Dynamic alanalysis of a novel autonomous 4-D hyperjerk circuit with hyperbolic sine nonlinearity: Chaos, antimonotonicity and a plethora of coexisting attractors. Chaos, Solutions & Fractals,107, 67–87.

    Article  MATH  Google Scholar 

  42. Mboupda Pone, J. R., Kamdoum Tamba, V., Kom, G. H., & Tiedeu, A. B. (2018). Period-doubling routes to chaos, bistability and antimononicity in a jerk circuit with quintinc nonlinearity. International Journal of Dynamics and Control,10, 12. https://doi.org/10.1007/s40435-018-0431-1.

    Article  Google Scholar 

  43. Manimehan, I., & Philominathan, P. (2012). Composite dynamical behaviors in a simple series-parallel LC circuit. Chaos, Solitons & Fractals,45, 1501–1509.

    Article  Google Scholar 

  44. Lai, Q., Tsafack, N., Kengne, J., & Zhao, X. W. (2018). Coexisting attractors and circuit implementation of a new 4D chaotic system with two equilibria. Chaos, Solitons & Fractals,107, 92–102.

    Article  MathSciNet  MATH  Google Scholar 

  45. Hamill, D. C. (1993). Learning about chaotic circuits with SPICE. IEEE Transactions on Educations,36, 28.

    Article  Google Scholar 

  46. Gaurav, G., & Tamas, R. (2009). MOS-integrable circuitry for multi-scroll chaotic grid realization: A SPICE-assisted proof. The International Journal of Circuit Theory and Applications,37, 473–483.

    Article  Google Scholar 

  47. Corinto, F., & Ascoli, A. (2012). Memristive diode bridge with LCR filter. Electronics Letters,48(14), 1.

    Article  Google Scholar 

  48. Daltzis, P. A., Volos, C. K., Nistazakis, H. E., Tzanakaki, A. A., & Tombras, G. S. (2017). Synchronization of Hyperchaotic Hyperjerk circuits with application in secure communications. In Proceedings of the 7th international conference on “Experiments/Process/System Modeling/Simulation/Optimization—IC-EPSMSO”, Athens, Greece (pp. 5–8).

  49. Fozin Fonzin, T., Kengne, J., & Pelap, F. B. (2018). Dynamical analysis and multistability in autonomous hyperchaotic oscillator with experimental verification. Nonlinear Dynamics,93, 653–669.

    Article  Google Scholar 

  50. Pham, V.-T., Kingni, S. T., Volos, C., Jafari, S., & Kapitaniake, T. A. (2018). Simple three-dimensional fractional-order chaotic system without equilibrium: Dynamics, circuit implementation and synchronization. International Journal of Electronics and Communications (AEÜ),86, 69–76.

    Article  Google Scholar 

  51. Jafari, S., Ahmadi, A., Khalaf, A. J. M., Reza Abdolmohammadi, H., Pham, V.-T., & Alsaadi, F. E. (2018). A new hidden chaotic attractor with extreme multi-stability. International Journal of Electronics and Communications. https://doi.org/10.1016/j.aeue.2018.03.037.

    Article  Google Scholar 

  52. Li, C.-L., Li, H.-M., Li, W., Tong, Y.-N., Zhang, J., Wei, D.-Q., et al. (2018). Dynamics, implementation and stability of a chaotic system with coexistence of hyperbolic and non-hyperbolic equilibria. International Journal of Electronics and Communications (AEÜ),84, 199–205.

    Article  Google Scholar 

  53. Rajagopal, K., Pham, V.-T., Tahir, F. R., Akgul, A., Abdolmohammadi, H. R., & Jafari, S. (2018). A chaotic jerk system with non-hyperbolic equilibrium: Dynamics, effect of time delay and circuit realization. Pramana Journal of Physics,90, 52.

    Article  Google Scholar 

  54. Pham, V.-T., Akgul, A., Volos, C., Jafari, S., & Kapitaniake, T. (2017). Dynamics and circuit realization of a no-equilibrium chaotic system with a boostable variable. International Journal of Electronics and Communications (AEÜ),8, 134–140.

    Article  Google Scholar 

  55. Wang, X., Akgul, A., Cicek, S., Pham, V.-T., & Hoang, D. V. (2017). A chaotic system with two stable equilibrium points: Dynamics, circuit realization and communication application. International Journal of Bifurcation and Chaos,27(8), 1750130.

    Article  MathSciNet  MATH  Google Scholar 

  56. Akgul, A., Li, C., & Pehlivan, I. (2017). Amplitude control analysis of a four-wing chaotic attractor, its electronic circuit designs and microcontroller-based random number generator. Journal of Circuits, Systems, and Computers,26(12), 1750190.

    Article  Google Scholar 

  57. Akgul, A., Li, C., & Pehlivan, I. (2017). Amplitude control analysis of a four-wing chaotic attractor, its electronic circuit designs and microcontroller-based random number generator. Journal of Circuits Systems and Computers,26, 1750190.

    Article  Google Scholar 

  58. Akgul, A., Shafqat, H., & Ihsan, P. (2016). A new three-dimensional chaotic system, its dynamical analysis and electronic circuit applications. Optik International, 127(18), 7062–7071.

    Article  Google Scholar 

  59. Jafari, S., Sprott, J. C., & Molaie, M. (2016). A simple chaotic flow with a plane of equilibria. International Journal of Bifurcation and Chaos,26(1650098), 52.

    MathSciNet  MATH  Google Scholar 

  60. Jafari, S., Sprott, J. C., Pham, V. T., Volos, K., & Li, C. (2016). Simple chaotic 3D flows with surfaces of equilibria. Nonlinear Dynamics,86(1349–1358), 53.

    Google Scholar 

  61. Leutcho, G. D., & Kengne, J. (2018). A unique chaotic snap system with a smoothly adjustable symmetry and nonlinearity: chaos, offset-boosting, antimonotonicity, and coexisting multiple attractors. Chaos, Solitons & Fractals,113(275–293), 54.

    MathSciNet  Google Scholar 

  62. Leutcho, G. D., Kengne, J., & Kengne, R. (2018). Remerging Feigenbaum trees, and multiple coexisting bifurcations in a novel hybrid diode-based hyperjerk circuit with offset boosting. International Journal of Dynamic Control. https://doi.org/10.1007/s40435-018-0438-7.

    Article  MATH  Google Scholar 

  63. Jafari, S., Ahmadi, A., Panahi, S., & Rajagopal, K. (2018). Extreme multi-stability: When imperfection changes quality. Chaos, Solitons & Fractals,108, 182–186.

    Article  Google Scholar 

  64. Njitacke, Z. T., & Kengne, J. (2018). Nonlinear dynamics of three-neurons-based Hopfield neural networks (HNNs): Remerging Feigenbaum trees, coexisting bifurcations and multiple attractors. Journal of Circuits, Systems and Computers. https://doi.org/10.1142/S0218126619501214.

    Article  Google Scholar 

  65. Jafari, S., Sprott, J. C., & Nazarimehr, F. (2015). Recent new examples of hidden attractors. European Physical Journal Special Topics,224, 1469–1476.

    Article  Google Scholar 

  66. Jafari, S., Pham, V. T., & Kapitaniak, T. (2016). Multi scroll chaotic sea obtained from a simple 3D system without equilibrium. International Journal of Bifurcation Chaos,26, 1650031.

    Article  MathSciNet  MATH  Google Scholar 

  67. Akgul, A., Calgan, H., Koyuncu, I., Pehlivan, I., & Istanbullu, A. (2015). Chaos-based engineering applications with a 3D chaotic system without equilibrium points. Nonlinear Dynamics. https://doi.org/10.1007/s11071-015-2501-7.

    Article  Google Scholar 

  68. Akgul, A. (2017). An electronic card for easy circuit realisation of complex nonlinear systems. Electronics World, December 2017.

  69. Leonov, G. A., Kuznetsov, N. V., & Vagaitsev, V. I. (2011). Localization of hidden Chua’s attractors. Physics Letters A,375, 2230–2233.

    Article  MathSciNet  MATH  Google Scholar 

  70. Li, C., & Sprott, J. C. (2014). Finding coexisting attractors using amplitude control. Nonlinear Dynamics,78, 2059–2064.

    Article  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Unité de Recherche d’Automatique et Informatique Appliquée (LAIA), Department of Electrical Engineering, IUT-FV Bandjoun, University of Dschang (Cameroon), P.O. Box: 134, Bandjoun, Cameroon

    Alexis Nguomkam Negou & Jacques Kengne

  2. Unité de Recherche de Matière Condensée, d’Electronique et de Traitement du Signal (URMACETS), Department of Physics, University of Dschang, P.O. Box 67, Dschang, Cameroon

    Alexis Nguomkam Negou & Jacques Kengne

Authors
  1. Alexis Nguomkam Negou
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Jacques Kengne
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Alexis Nguomkam Negou.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Nguomkam Negou, A., Kengne, J. A minimal three-term chaotic flow with coexisting routes to chaos, multiple solutions, and its analog circuit realization. Analog Integr Circ Sig Process 101, 415–429 (2019). https://doi.org/10.1007/s10470-019-01436-8

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10470-019-01436-8

Keywords

Search

Navigation