Concept for gradient-free MRI on twin natural slices

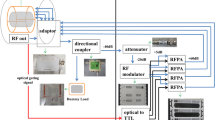
Abstract

Objective

The design of an MRI for use in space requires that the hardware be kept to an absolute minimum in terms of mass, complexity, and power. In addition, NASA requirements are that the external stray field needs to be less than 3.2 Gauss, 7 cm from the MRI enclosure.

Theory

RF encoding designs with Halbach magnets offer the best chance of meeting those requirements. Spatially non-uniform magnetic fields with foliations of isomagnetic surfaces, or natural slices, may be used to provide slice selection, and to reduce further the hardware complexity, for TRansmit Array Spatial Encoding (TRASE) Magnetic Resonance Imaging (MRI) or potentially for other radio frequency (RF) encoding methods. The design of such non-uniform magnetic fields in a Halbach configuration with built-in axial gradients leads to pairs of isomagnetic surfaces centered on either side of a central maximum field strength slice. If TRASE images from slices other than the central isomagnetic surface are desired, then the Nuclear Magnetic Resonance (NMR) signals originating from the twin natural slices must be separated during image reconstruction. Here, a design for simultaneously imaging on twin slices in such an inhomogeneous magnetic field using multiple receiver coils with spatially varying RF profiles is described mathematically and numerical simulation examples are given.

Design approach

To achieve RF encoding on the natural slices, at least three TRASE transmit coils are required. Here a solution with twisted solenoid coils is given. To achieve the twin slice separation at least two receive coils are required. Here a solution with two solenoids is given.

Discussion

The MRI design presented here uses a combination of RF encoding (TRASE), a spatial encoding magnetic field (SEM, pairs of natural slices) and receive coil spatial profiles to encode enough information into the NMR signal for image slice reconstruction. The design presented here enables using Halbach magnets with a built-in axial gradient to be used for MRI.

Conclusion

The result is a new gradient-free TRASE MRI design capable of imaging pairs of electronically selectable axial slices.

Appendix— Theorem 1

Theorem 1

Let the disentangling map ${{\mathcal {G}}}_{Q}: \mathbb {C}^{Q} \otimes \mathbb {C}^{2} \rightarrow \mathbb {C}^{Q} \otimes \mathbb {C}^{2}$ be defined by the matrices $[G]_{q}$, $q \in \{ 1, 2, \ldots , Q \}$ as

$$\begin{aligned}{}[G]_{q} \; \vec {V}_{q} = \left[ \begin{array}{cc} G_{11} &{} G_{12} \\ G_{12} &{} G_{22} \end{array} \right] \left[ \begin{array}{c} V_{1,q} \\ V_{2,q} \end{array} \right] = \left[ \begin{array}{c} W_{1,q} \\ W_{2,q} \end{array} \right] = \vec {W}_{q}, \end{aligned}$$

where ${{\mathcal {G}}}_{Q}$ acts on Q vectors $\vec {V}_{q} \in \mathbb {C}^{2}$ to give Q vectors $\vec {W}_{q} \in \mathbb {C}^{2}$. The two components of $\vec {V}_{q}$ represent the signal from the two receive channels when $Q = n_{k}$, the number of k-space points, and the two slices when $Q = n$, the number of image points. The two components of $\vec {W}_{q}$ respectively represent the disentangled Fourier transform of the signals from the two receive channels when $Q = n_{k}$ and the two slice images when $Q = n$. Note that $[G]_{q}$ is independent of q (i.e., of “pixel” location); this is an important hypothesis for this theorem.

Define the map (Fourier reconstruction)

$$\begin{aligned} {{\mathcal {P}}} = {P} \otimes {P}: \mathbb {C}^{2} \otimes \mathbb {C}^{n_{k}} \rightarrow \mathbb {C}^{2} \otimes \mathbb {C}^{n} \end{aligned}$$

via

$$\begin{aligned} (P \vec {{\mathcal {V}}}_{c})_{j} = \sum _{\ell =1}^{n_{k}} V_{c,\ell }\,\, e^{i \vec {\varphi }_{\ell ,j} \cdot \vec {\alpha }_{j} }, \end{aligned}$$

where $\vec {{\mathcal {V}}}_{c} \in \mathbb {C}^{n_{k}}$, $c \in \{ 1,2 \}$, indexes the channels, j indexes the $n = n_{x}n_{y}$ pixels for TRASE image reconstruction ($\vec {\alpha }_{j} \in \mathbb {R}^{2}$ is the coordinate of pixel j in coordinates that match the isophase lines produced by the TRASE coils) and, for TRASE signal reconstruction, $\vec {\varphi }_{\ell ,j} \in \mathbb {C}^{2}$ are defined as per Ref. [25] (they depend on the choice of k-space pattern and image pixel locations, for Cartesian coordinates, $\vec {\varphi }_{\ell ,j} = \vec {k}_{\ell ,j}$ is independent of j; explicitly, if $\vec {\alpha }_{j} = [x_{j},y_{j}]^{T}$ then $\vec {\varphi }_{\ell ,j} =[k_{x}^{\ell },k_{y}^{\ell }]^{T}$).

Finally, define ${{\mathcal {T}}}_{Q}: \mathbb {C}^{Q} \otimes \mathbb {C}^{2} \rightarrow \mathbb {C}^{2} \otimes \mathbb {C}^{Q}$ and ${\mathcal {T}}^{-1}_{Q}: \mathbb {C}^{2} \otimes \mathbb {C}^{Q} \rightarrow \mathbb {C}^{Q} \otimes \mathbb {R}^{2}$ by

$$\begin{aligned} {{\mathcal {T}}}_{Q} \left[ \begin{array}{c} \left[ \begin{array}{c} V_{1,1} \\ V_{2,1} \end{array} \right] \\ \vdots \\ \left[ \begin{array}{c} V_{1,q} \\ V_{2,q} \end{array} \right] \\ \vdots \\ \left[ \begin{array}{c} V_{1,Q} \\ V_{2,Q} \end{array} \right] \end{array} \right] = \left[ \begin{array}{c} \left[ \begin{array}{c} V_{1,1} \\ \vdots \\ V_{1,q} \\ \vdots \\ V_{1,Q} \end{array} \right] \\ \\ \left[ \begin{array}{c} V_{2,1} \\ \vdots \\ V_{2,q} \\ \vdots \\ V_{2,Q} \end{array} \right] \end{array} \right] \end{aligned}$$

and

$$\begin{aligned} {{\mathcal {T}}}^{-1}_{Q} \left[ \begin{array}{c} \left[ \begin{array}{c} V_{1,1} \\ \vdots \\ V_{1,q} \\ \vdots \\ V_{1,Q} \end{array} \right] \\ \\ \left[ \begin{array}{c} V_{2,1} \\ \vdots \\ V_{2,q} \\ \vdots \\ V_{2,Q} \end{array} \right] \end{array} \right] = \left[ \begin{array}{c} \left[ \begin{array}{c} V_{1,1} \\ V_{2,1} \end{array} \right] \\ \vdots \\ \left[ \begin{array}{c} V_{1,q} \\ V_{2,q} \end{array} \right] \\ \vdots \\ \left[ \begin{array}{c} V_{1,Q} \\ V_{2,Q} \end{array} \right] \end{array} \right] , \end{aligned}$$

where $V_{c,q}$ is component q of $\vec {V}_{c} \in \mathbb {C}^{Q}$.

Then, ${{\mathcal {G}}}_{Q}$ and ${{\mathcal {P}}}$ commute, in the sense that ${{\mathcal {T}}}_{n} {{\mathcal {G}}}_{n} {{\mathcal {T}}}^{-1}_{n} {{\mathcal {P}}} = {{\mathcal {P}}} {{\mathcal {T}}}_{n_{k}} {{\mathcal {G}}}_{n_{k}} {{\mathcal {T}}}^{-1}_{n_{k}}$.

Proof

By calculation. Let $\vec {{\mathcal {V}}}_{1} \otimes \vec {{\mathcal {V}}}_{2} \in \mathbb {C}^{2} \otimes \mathbb {C}^{n_{k}}$ (i.e. $\vec {{\mathcal {V}}}_{i} \in \mathbb {C}^{n_{k}}$), then

$$\begin{aligned}&{{\mathcal {G}}}_{n} {{\mathcal {T}}}^{-1}_{n} {{\mathcal {P}}} (\vec {{\mathcal {V}}}_{1} \otimes \vec {{\mathcal {V}}}_{2}) = {{\mathcal {G}}}_{n} \left[ \begin{array}{c} \sum _{\ell =1}^{n_{k}} V_{1,\ell }\,\, e^{i \vec {\varphi }_{\ell ,1} \cdot \vec {\alpha }_{1} } \\ \sum _{\ell =1}^{n_{k}} V_{2,\ell }\,\, e^{i \vec {\varphi }_{\ell ,1} \cdot \vec {\alpha }_{1} } \\ \vdots \\ \sum _{\ell =1}^{n_{k}} V_{1,\ell }\,\, e^{i \vec {\varphi }_{\ell ,j} \cdot \vec {\alpha }_{j} } \\ \sum _{\ell =1}^{n_{k}} V_{2,\ell }\,\, e^{i \vec {\varphi }_{\ell ,j} \cdot \vec {\alpha }_{j} } \\ \vdots \\ \sum _{\ell =1}^{n_{k}} V_{1,\ell }\,\, e^{i \vec {\varphi }_{\ell ,n} \cdot \vec {\alpha }_{n} } \\ \sum _{\ell =1}^{n_{k}} V_{2,\ell }\,\, e^{i \vec {\varphi }_{\ell ,n} \cdot \vec {\alpha }_{n} } \end{array} \right] \\&\quad = \left[ \begin{array}{cc} \left[ \begin{array}{cc} G_{11} &{} G_{12}\\ G_{21} &{} G_{22} \end{array} \right] &{} \left[ \begin{array}{c} \sum _{\ell =1}^{n_{k}} V_{1,\ell }\,\, e^{i \vec {\varphi }_{\ell ,1} \cdot \vec {\alpha }_{1} } \\ \sum _{\ell =1}^{n_{k}} V_{2,\ell }\,\, e^{i \vec {\varphi }_{\ell ,1} \cdot \vec {\alpha }_{1} } \end{array} \right] \\ \vdots \\ \left[ \begin{array}{cc} G_{11} &{} G_{12}\\ G_{21} &{} G_{22} \end{array} \right] &{} \left[ \begin{array}{c} \sum _{\ell =1}^{n_{k}} V_{1,\ell }\,\, e^{i \vec {\varphi }_{\ell ,j} \cdot \vec {\alpha }_{j} } \\ \sum _{\ell =1}^{n_{k}} V_{2,\ell }\,\, e^{i \vec {\varphi }_{\ell ,j} \cdot \vec {\alpha }_{j} } \end{array} \right] \\ \vdots \\ \left[ \begin{array}{cc} G_{11} &{} G_{12}\\ G_{21} &{} G_{22} \end{array} \right] &{} \left[ \begin{array}{c} \sum _{\ell =1}^{n_{k}} V_{1,\ell }\,\, e^{i \vec {\varphi }_{\ell ,n} \cdot \vec {\alpha }_{n} } \\ \sum _{\ell =1}^{n_{k}} V_{2,\ell }\,\, e^{i \vec {\varphi }_{\ell ,n} \cdot \vec {\alpha }_{n} } \end{array} \right] \end{array} \right] \\&= \left[ \begin{array}{c} \left[ \begin{array}{c} \sum _{\ell =1}^{n_{k}} G_{11} V_{1,\ell }\,\, e^{i \vec {\varphi }_{\ell ,1} \cdot \vec {\alpha }_{1} } + \sum _{\ell =1}^{n_{k}} G_{12} V_{2,\ell }\,\, e^{i \vec {\varphi }_{\ell ,1} \cdot \vec {\alpha }_{1} } \\ \sum _{\ell =1}^{n_{k}} G_{21} V_{1,\ell }\,\, e^{i \vec {\varphi }_{\ell ,1} \cdot \vec {\alpha }_{1} } + \sum _{\ell =1}^{n_{k}} G_{22} V_{2,\ell }\,\, e^{i \vec {\varphi }_{\ell ,1} \cdot \vec {\alpha }_{1} } \end{array} \right] \\ \vdots \\ \left[ \begin{array}{c} \sum _{\ell =1}^{n_{k}} G_{11} V_{1,\ell }\,\, e^{i \vec {\varphi }_{\ell ,j} \cdot \vec {\alpha }_{j} } + \sum _{\ell =1}^{n_{k}} G_{12} V_{2,\ell }\,\, e^{i \vec {\varphi }_{\ell ,j} \cdot \vec {\alpha }_{j} } \\ \sum _{\ell =1}^{n_{k}} G_{21} V_{1,\ell }\,\, e^{i \vec {\varphi }_{\ell ,j} \cdot \vec {\alpha }_{j} } + \sum _{\ell =1}^{n_{k}} G_{22} V_{2,\ell }\,\, e^{i \vec {\varphi }_{\ell ,1} \cdot \vec {\alpha }_{1} } \end{array} \right] \\ \vdots \\ \left[ \begin{array}{c} \sum _{\ell =1}^{n_{k}} G_{11} V_{1,\ell }\, e^{i \vec {\varphi }_{\ell ,n} \cdot \vec {\alpha }_{n} } + \sum _{\ell =1}^{n_{k}} G_{12} V_{2,\ell }\,\, e^{i \vec {\varphi }_{\ell ,n} \cdot \vec {\alpha }_{n} } \\ \sum _{\ell =1}^{n_{k}} G_{21} V_{1,\ell }\,\, e^{i \vec {\varphi }_{\ell ,n} \cdot \vec {\alpha }_{n} } + \sum _{\ell =1}^{n_{k}} G_{22} V_{2,\ell }\,\, e^{i \vec {\varphi }_{\ell ,n} \cdot \vec {\alpha }_{n} } \end{array} \right] \end{array} \right] \\&\quad = \left[ \begin{array}{c} \left[ \begin{array}{c} \sum _{\ell =1}^{n_{k}} (G_{11} V_{1,\ell } + G_{12} V_{2,\ell })\,\, e^{i \vec {\varphi }_{\ell ,1} \cdot \vec {\alpha }_{1} } \\ \sum _{\ell =1}^{n_{k}} (G_{21} V_{1,\ell } + G_{22} V_{2,\ell } )\,\, e^{i \vec {\varphi }_{\ell ,1} \cdot \vec {\alpha }_{1} } \end{array} \right] \\ \vdots \\ \left[ \begin{array}{c} \sum _{\ell =1}^{n_{k}} (G_{11} V_{1,\ell } + G_{12} V_{2,\ell } )\,\, e^{i \vec {\varphi }_{\ell ,j} \cdot \vec {\alpha }_{j} } \\ \sum _{\ell =1}^{n_{k}} (G_{21} V_{1,\ell } + G_{22} V_{2,\ell } )\,\, e^{i \vec {\varphi }_{\ell ,j} \cdot \vec {\alpha }_{j} } \end{array} \right] \\ \vdots \\ \left[ \begin{array}{c} \sum _{\ell =1}^{n_{k}} (G_{11} V_{1,\ell } + G_{12} V_{2,\ell } )\,\, e^{i \vec {\varphi }_{\ell ,n} \cdot \vec {\alpha }_{n} } \\ \sum _{\ell =1}^{n_{k}} (G_{21} V_{1,\ell } + G_{22} V_{2,\ell } )\,\, e^{i \vec {\varphi }_{\ell ,n} \cdot \vec {\alpha }_{n} } \end{array} \right] \end{array} \right] \end{aligned}$$

on one hand and

$$\begin{aligned}&{{\mathcal {T}}}^{-1}_{n} {{\mathcal {P}}} {{\mathcal {T}}}_{n_{k}} {{\mathcal {G}}} _{n_{k}} {{\mathcal {T}}}^{-1}_{n_{k}}(\vec {{\mathcal {V}}}_{1} \otimes \vec {{\mathcal {V}}}_{2}) = {{\mathcal {T}}}^{-1}_{n} {{\mathcal {P}}} {{\mathcal {T}}}_{n_{k}} {{\mathcal {G}}}_{n_{k}} \left[ \begin{array}{c} V_{1,\ell } \\ V_{2,\ell } \\ \vdots \\ V_{1,\ell } \\ V_{2,\ell } \\ \vdots \\ V_{1,\ell } \\ V_{2,\ell } \end{array} \right] \\&\quad = {{\mathcal {P}}} {{\mathcal {T}}}_{n_{k}} \left[ \begin{array}{cc} \left[ \begin{array}{cc} G_{11} &{} G_{12}\\ G_{21} &{} G_{22} \end{array} \right] &{} \left[ \begin{array}{c} V_{1,\ell } \\ V_{2,\ell } \end{array} \right] \\ \vdots \\ \left[ \begin{array}{cc} G_{11} &{} G_{12}\\ G_{21} &{} G_{22} \end{array} \right] &{} \left[ \begin{array}{c} V_{1,\ell } \\ V_{2,\ell } \end{array} \right] \\ \vdots \\ \left[ \begin{array}{cc} G_{11} &{} G_{12}\\ G_{21} &{} G_{22} \end{array} \right] &{} \left[ \begin{array}{c} V_{1,\ell } \\ V_{2,\ell } \end{array} \right] \end{array} \right] \\&= {{\mathcal {T}}}^{-1}_{n} {{\mathcal {P}}} {{\mathcal {T}}}_{n_{k}} \left[ \begin{array}{c} \left[ \begin{array}{c} (G_{11} V_{1,\ell } + G_{12} V_{2,\ell }) \\ (G_{21} V_{1,\ell } + G_{22} V_{2,\ell } ) \end{array} \right] \\ \vdots \\ \left[ \begin{array}{c} (G_{11} V_{1,\ell } + G_{12} V_{2,\ell } ) \\ (G_{21} V_{1,\ell } + G_{22} V_{2,\ell } ) \end{array} \right] \\ \vdots \\ \left[ \begin{array}{c} (G_{11} V_{1,\ell } + G_{12} V_{2,\ell } ) \\ (G_{21} V_{1,\ell } + G_{22} V_{2,\ell } ) \end{array} \right] \end{array} \right] \\&\quad = \left[ \begin{array}{c} \left[ \begin{array}{c} \sum _{\ell =1}^{n_{k}} (G_{11} V_{1,\ell } + G_{12} V_{2,\ell })\,\, e^{i \vec {\varphi }_{\ell ,1} \cdot \vec {\alpha }_{1} } \\ \sum _{\ell =1}^{n_{k}} (G_{21} V_{1,\ell } + G_{22} V_{2,\ell } )\,\, e^{i \vec {\varphi }_{\ell ,1} \cdot \vec {\alpha }_{1} } \end{array} \right] \\ \vdots \\ \left[ \begin{array}{c} \sum _{\ell =1}^{n_{k}} (G_{11} V_{1,\ell } + G_{12} V_{2,\ell } )\,\, e^{i \vec {\varphi }_{\ell ,j} \cdot \vec {\alpha }_{j} } \\ \sum _{\ell =1}^{n_{k}} (G_{21} V_{1,\ell } + G_{22} V_{2,\ell } )\,\, e^{i \vec {\varphi }_{\ell ,j} \cdot \vec {\alpha }_{j} } \end{array} \right] \\ \vdots \\ \left[ \begin{array}{c} \sum _{\ell =1}^{n_{k}} (G_{11} V_{1,\ell } + G_{12} V_{2,\ell } )\,\, e^{i \vec {\varphi }_{\ell ,n} \cdot \vec {\alpha }_{n} } \\ \sum _{\ell =1}^{n_{k}} (G_{21} V_{1,\ell } + G_{22} V_{2,\ell } )\,\, e^{i \vec {\varphi }_{\ell ,n} \cdot \vec {\alpha }_{n} } \end{array} \right] \end{array} \right] . \end{aligned}$$

So,

$$\begin{aligned} {{\mathcal {T}}}_{n} {{\mathcal {G}}}_{n} {{\mathcal {T}}}^{-1}_{n} {{\mathcal {P}}}_{n} (\vec {\mathcal {V}}_{1} \otimes \vec {{\mathcal {V}}}_{2}) = {{\mathcal {P}}} {{\mathcal {T}}}_{n_{k}} {{\mathcal {G}}} _{n_{k}} {{\mathcal {T}}}^{-1}_{n_{k}}(\vec {{\mathcal {V}}}_{1} \otimes \vec {{\mathcal {V}}}_{2}). \end{aligned}$$

$\square$

It is interesting to examine the theorem in a little detail. First, note that the map ${{\mathcal {G}}}_{n}$ acts on image space while ${\mathcal {G}}_{n_{k}}$ acts on k-space. So there is no reason to expect that the order of Fourier transformation and disentanglement can be swapped. In general, one has

$$\begin{aligned}{}[G]_{q} \; \vec {V}_{q} = \left[ \begin{array}{cc} G_{11,q} &{} G_{12,q} \\ G_{12,q} &{} G_{22,q} \end{array} \right] \left[ \begin{array}{c} V_{1,q} \\ V_{2,q} \end{array} \right] = \left[ \begin{array}{c} W_{1,q} \\ W_{2,q} \end{array} \right] = \vec {W}_{q}, \end{aligned}$$

(21)

where the values of $[G]_{q}$ are determined from the pixel-by-pixel values of the $B_{1}$ fields of the two receive coils on the excited natural slices. In general, it is not necessary that $n = n_{k}$ so the transformation from ${{\mathcal {G}}}_{n}$ to ${{\mathcal {G}}}_{n_{k}}$ will be somewhat complicated in general which would exclude the possibility of swapping the order of application. However, in the limit of a constant ${{\mathcal {G}}}_{n}$, its k-space version ${{\mathcal {G}}}_{n_{k}}$ will be the same even if $n \ne n_{k}$. That is, the two image functions will be entangled in the same way as their Fourier transforms.