Skip to main content
SpringerLink
Log in

Transient dynamic analysis of a fluid-saturated porous gradient elastic column

  • Published:
Acta Mechanica Aims and scope Submit manuscript

Abstract

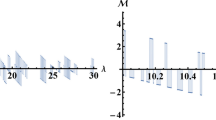
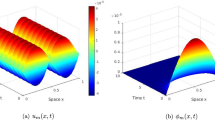
The dynamic response of a fluid-saturated porous gradient elastic column to a transient disturbance is determined analytically and numerically. The basic dynamic theory of a fluid-saturated poroelastic medium due to Biot is modified by replacing the classical linear elastic model of the solid skeleton by the simple gradient elastic model of Mindlin with just one elastic constant (internal length scale) in addition to the classical ones. Thus, the new theory, which is presently restricted to the one-dimensional case, can take into account the microstructural effects of the solid skeleton. After the establishment of appropriate boundary and initial conditions, the one-dimensional dynamic column problem is solved analytically with the aid of the Laplace transform with respect to time. The time domain response is finally obtained by a numerical inversion of the transformed solution. The effect of the solid microstructure on the response is assessed and discussed.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Biot M.A.: Theory of propagation of elastic waves in a fluid-saturated porous solid I. Low frequency range. J. Acoust. Soc. Am. 28, 168–178 (1956)

    Article  MathSciNet  Google Scholar 

  2. Bowen R.M., Lockett R.R.: Inertial effects in poroelasticity. J. Appl. Mech. ASME 50, 334–342 (1983)

    Article  MATH  Google Scholar 

  3. Vardoulakis I., Beskos D.E.: Dynamic behavior of nearly saturated porous media. Mech. Mater. 5, 87–108 (1986)

    Article  Google Scholar 

  4. Vgenopoulou I., Beskos D.E.: Dynamic poroelastic soil column and borehole problem analysis. Soil Dyn. Earthq. Eng. 11, 335–345 (1992)

    Article  Google Scholar 

  5. Vgenopoulou I., Beskos D.E.: Dynamic behavior of saturated poroviscoelastic media. Acta Mech. 95, 185–195 (1992)

    Article  MATH  Google Scholar 

  6. de Boer R., Ehlers W., Liu Z.: One-dimensional transient wave propagation in fluid-saturated incompressible porous media. Arch. Appl. Mech. 63, 59–72 (1993)

    Article  MATH  Google Scholar 

  7. Schanz M., Cheng A.H.D.: Transient wave propagation in a one-dimensional poroelastic column. Acta Mech. 145, 1–18 (2000)

    Article  MATH  Google Scholar 

  8. Schanz M., Cheng A.H.D.: Dynamic analysis of a one-dimensional poroviscoelastic column. J. Appl. Mech. ASME 68, 192–198 (2001)

    Article  MATH  Google Scholar 

  9. Cheng A.H.D., Badmus T., Beskos D.E.: Integral equation for dynamic poroelasticity in frequency domain with BEM solution. J. Eng. Mech. ASCE 117, 1136–1157 (1991)

    Article  Google Scholar 

  10. Dominguez J.: An integral formulation for dynamic poroelasticity. J. Appl. Mech. ASME 58, 588–591 (1991)

    Article  MATH  Google Scholar 

  11. Dominguez J.: Boundary element approach for dynamic poroelastic problems. Int. J. Numer. Method Eng. 35, 307–324 (1992)

    Article  MathSciNet  MATH  Google Scholar 

  12. Schanz M.: Application of 3D time domain boundary element formulation to wave propagation in poroelastic solids. Eng. Anal. Bound. Elem. 25, 363–376 (2001)

    Article  MATH  Google Scholar 

  13. Degrande G., de Roeck G., Vanden Broeck P., Smeulders D.: Wave propagation in layered dry, saturated and unsaturated poroelastic media. Int. J. Solid Struct. 35, 4753–4778 (1998)

    Article  MATH  Google Scholar 

  14. Schanz M.: Poroelastodynamics: linear models, analytical solutions and numerical methods. Appl. Mech. Rev. ASME 62, 1–15 (2009)

    Article  Google Scholar 

  15. Mindlin R.D.: Micro-structure in linear elasticity. Arch. Rat. Mech. Anal. 16, 51–78 (1964)

    Article  MathSciNet  MATH  Google Scholar 

  16. Vardoulakis I., Sulem J.: Bifurcation Analysis in Geomechanics. Blackie/Chapman and Hall, London (1995)

    Google Scholar 

  17. Exadaktylos G.E., Vardoulakis I.: Microstructure in linear elasticity and scale effects: a reconsideration of basic rock mechanics and rock fracture mechanics. Tectonophysics 335, 81–109 (2001)

    Article  Google Scholar 

  18. Ru C.Q., Aifantis E.C.: A simple approach to solve boundary value problems in gradient elasticity. Acta Mech. 101, 59–68 (1993)

    Article  MathSciNet  MATH  Google Scholar 

  19. Altan B.S., Evensen H., Aifantis E.C.: Longitudinal vibrations of a beam: a gradient elasticity approach. Mech. Res. Commun. 23, 35–40 (1996)

    Article  MATH  Google Scholar 

  20. Chang C.S., Gao J.: Wave propagation in granular rod using high-gradient theory. J. Eng. Mech. ASCE 123, 52–59 (1997)

    Article  Google Scholar 

  21. Georgiadis H.G., Vardoulakis I., Lykotrafitis G.: Torsional surface waves in a gradient elastic half-space. Wave Motion 31, 333–348 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  22. Georgiadis H.G.: The mode III crack problem in microstructured solids governed by dipolar gradient elasticity: static and dynamic analysis. J. Appl. Mech. ASME 70, 517–530 (2003)

    Article  MATH  Google Scholar 

  23. Amanatidou E., Aravas N.: Mixed finite element formulations of strain-gradient elasticity problems. Comput. Method Appl. Mech. Eng. 191, 1723–1751 (2002)

    Article  MATH  Google Scholar 

  24. Tsepoura K.G., Papargyri-Beskou S., Polyzos D., Beskos D.E.: Static and dynamic analysis of a gradient elastic bar in tension. Arch. Appl. Mech. 72, 483–497 (2002)

    Article  MATH  Google Scholar 

  25. Papargyri-Beskou S., Polyzos D., Beskos D.E.: Dynamic analysis of gradient elastic flexural beams. Struct. Eng. Mech. 15, 705–716 (2003)

    Google Scholar 

  26. Papargyri-Beskou S., Polyzos D., Beskos D.E.: Wave dispersion in gradient elastic solids and structures: a unified treatment. Int. J. Solids Struct. 46, 3751–3759 (2009)

    Article  MATH  Google Scholar 

  27. Papargyri-Beskou S., Giannakopoulos A.E., Beskos D.E.: Variational analysis of gradient elastic flexural plates under static loading. Int. J. Solid Struct. 47, 2755–2766 (2010)

    Article  MATH  Google Scholar 

  28. Polyzos D., Tsepoura K.G., Beskos D.E.: Transient dynamic analysis of 3-D gradient elastic solids by BEM. Comput. Struct. 83, 783–792 (2005)

    Article  Google Scholar 

  29. Giannakopoulos A.E., Stamoulis K.: Structural analysis of gradient elastic components. Int. J. Solid Struct. 44, 3440–3451 (2007)

    Article  MATH  Google Scholar 

  30. Karlis G.F., Tsinopoulos S.V., Polyzos D., Beskos D.E.: Boundary element analysis of mode I and mixed mode (I and II) crack problems in 2-D gradient elasticity. Comput. Method Appl. Mech. Eng. 196, 5092–5103 (2007)

    Article  MATH  Google Scholar 

  31. Papargyri-Beskou S., Beskos D.E.: Static, stability and dynamic analysis of gradient elastic flexural Kirchhoff plates. Arch. Appl. Mech. 78, 625–635 (2008)

    Article  MATH  Google Scholar 

  32. Zervos A.: Finite elements for elasticity with microstructure and gradient elasticity. Int. J. Numer. Method Eng. 73, 564–595 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  33. Askes H., Metrikine A.V., Pichugin A.V., Bennett T.: Four simplified gradient elasticity models for the simulation of dispersive wave propagation. Philos. Mag. 88, 3415–3443 (2008)

    Article  Google Scholar 

  34. Askes H., Wang B., Bennett T.: Element size and time step selection procedures for the numerical analysis of elasticity with higher-order inertia. J. Sound Vib. 314, 650–656 (2008)

    Article  Google Scholar 

  35. Tsamasphyros G.I., Vrettos C.D.: A mixed finite volume formulation for the solution of gradient elasticity problems. Arch. Appl. Mech. 80, 609–627 (2010)

    Article  Google Scholar 

  36. Bedford A., Drumheller D.S.: Theories of immiscible and structured mixtures. Int. J. Eng. Sci. 21, 863–960 (1983)

    Article  MathSciNet  MATH  Google Scholar 

  37. Berryman J.G., Thigpen L.: Nonlinear and semi-linear dynamic poroelasticity with microstructure. J. Mech. Phys. Solids 33, 97–116 (1985)

    Article  MATH  Google Scholar 

  38. Aifantis E.C.: Microscopic processes and macroscopic response. In: Mechanics of Engineering Materials, Desai, C.S., Gallagher, R.H. (Eds), John Wiley and Sons, Chichester, pp. 1–22 (1984)

  39. Vardoulakis I., Aifantis E.C.: On the role of microstructure in the behavior of soils: effects of higher order gradients and internal inertia. Mech. Mater. 18, 151–158 (1994)

    Article  Google Scholar 

  40. Beskos D.E.: Dynamics of saturated rocks. I: equations of motion. J. Eng. Mech. ASCE 115, 982–995 (1989)

    Article  Google Scholar 

  41. Taylor D.W.: Fundamentals of Soil Mechanics. Wiley, London (1948)

    Google Scholar 

  42. Durbin F.: Numerical inversion of Laplace transform: an efficient improvement to Dubner and Abate’s method. Comput. J. 17, 371–376 (1974)

    MathSciNet  MATH  Google Scholar 

  43. Narayanan G.V., Beskos D.E.: Numerical operational methods for time-dependent linear problems. Int. J. Numer. Method Eng. 18, 1829–1854 (1982)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Civil Engineering, Aristotle University of Thessaloniki, 54006, Thessaloniki, Greece

    Sofia Papargyri-Beskou

  2. Department of Mechanical Engineering, Technological Educational Institute of Patras, 26334, Patras, Greece

    Stefanos V. Tsinopoulos

  3. Department of Civil Engineering, University of Patras, 26500, Patras, Greece

    Dimitri E. Beskos

Authors
  1. Sofia Papargyri-Beskou
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Stefanos V. Tsinopoulos
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Dimitri E. Beskos
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Dimitri E. Beskos.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Papargyri-Beskou, S., Tsinopoulos, S.V. & Beskos, D.E. Transient dynamic analysis of a fluid-saturated porous gradient elastic column. Acta Mech 222, 351–362 (2011). https://doi.org/10.1007/s00707-011-0539-2

Download citation

  • Received:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00707-011-0539-2

Keywords

Search

Navigation