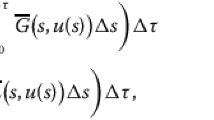1 Introduction

The theory of time scales was introduced and developed by Hilger [1] and Bohner and Peterson [2, 3] in order to unify continuous and discrete analysis. It has been applied to various fields of mathematics. In particular, many authors have extended some integral inequalities used in the theory of differential, difference, and integral equations to an arbitrary time scale; see, for instance, the papers [4–16] and the references cited therein.

In what follows, let us briefly comment on a number of closely related results which motivated our study. Li and Sheng [8] established several integral inequalities and studied the boundedness properties of some nonlinear dynamic equations, one of which we present below for convenience of the reader. In what follows, we use the following notation (some other concepts related to the notion of time scales; see Bohner and Peterson [2]):

ℝ denotes the set of real numbers, \(\mathbb{R}_{+}=[0,\infty)\), \(\mathbb{T}\) is an arbitrary time scale, and the set \(\mathbb{T}^{k}\) is derived from \(\mathbb{T}\) as follows: if \(\mathbb{T}\) has a left-scattered maximum \(m_{0}\), then \(\mathbb{T}^{k}=\mathbb{T}-\{m_{0}\}\), otherwise \(\mathbb{T}^{k}=\mathbb{T}\).

Theorem 1.1

[8, Theorem 3.2]

Assume that \(u,a,b,f,g:\mathbb{T}^{k}\rightarrow\mathbb{R}_{+}\) are rd-continuous functions and let p and q be real constants satisfying \(p\geq q>0\). Then the inequality

$$u^{p}(t)\leq a(t)+b(t)\int^{t}_{t_{0}} \bigl[f(s)u^{p}(s)+g(s)u^{q}(s)\bigr]\Delta s,\quad t\in \mathbb{T}^{k} $$

implies that, for any \(K>0\),

$$u(t)\leq \biggl\{ a(t)+b(t)\int^{t}_{t_{0}}e_{A} \bigl(t,\sigma(s)\bigr) \biggl[a(s)f(s)+g(s) \biggl(\frac{q}{p}K^{(q-p)/p}a(s)+ \frac{p-q}{p}K^{q/p} \biggr) \biggr]\Delta s \biggr\} ^{1/p}, $$

where \(t\in\mathbb{T}^{k}\) and \(A(t)=b(t)(f(t)+qK^{(q-p)/p}g(t)/p)\).

Sun and Hassan [15] studied the following dynamic integral inequality with mixed nonlinearities.

Theorem 1.2

[15, Theorem 1]

Assume that \(u,a,b,g,h_{1},h_{2}:\mathbb{T}^{k}\rightarrow \mathbb{R}_{+}\) are rd-continuous functions and let \(\lambda_{1}\) and \(\lambda_{2}\) be real constants satisfying \(0<\lambda_{1}<1<\lambda_{2}\). Then, for any rd-continuous functions \(k_{1}(t)>0\) and \(k_{2}(t)\geq0\) on \(\mathbb{T}^{k}\) satisfying \(k(t)=k_{1}(t)-k_{2}(t)\geq0\) and \(\mu(t)k(t)b(\sigma(t))<1\) for \(t\in\mathbb{T}^{k}\), the inequality

$$u(t)\leq a(t)+b(t)\int^{t}_{t_{0}} \bigl[g(s)u(s)+h_{1}(s)u^{\lambda _{1}}\bigl(\sigma(s) \bigr)-h_{2}(s)u^{\lambda_{2}}\bigl(\sigma(s)\bigr)\bigr]\Delta s,\quad t \in \mathbb{T}^{k} $$

implies that

$$u(t)\leq a(t)+b(t)\int^{t}_{t_{0}}e_{A\oplus B} \bigl(t,\sigma(s)\bigr)D(s)\Delta s,\quad t\in\mathbb{T}^{k}, $$

where

$$A(t)=b(t)g(t),\qquad B(t)=\frac{k(t)b(\sigma(t))}{1-\mu (t)k(t)b(\sigma(t))},\qquad D(t)=\bigl(1+\mu(t)B(t) \bigr)C(t), $$

and

$$C(t)=a(t)g(t)+a\bigl(\sigma(t)\bigr)k(t)+\theta_{1}( \lambda_{1},h_{1},k_{1})+ \theta_{2}( \lambda_{2},h_{2},k_{2}). $$

The aim of this paper is to further generalize some integral inequalities on time scales that have been reported in [7, 8, 15]. We consider the following dynamic integral inequalities with mixed nonlinearities

$$\begin{aligned} u^{p}(t)\leq{}& a(t)+b(t)\int^{t}_{t_{0}} \biggl[f(s)u^{p}(s)+g(s)u^{q}(s)+h_{1}(s)u^{\lambda_{1}} \bigl(\sigma(s)\bigr) \\ &{}-h_{2}(s)u^{\lambda_{2}}\bigl(\sigma(s)\bigr)+l(s)+\int ^{s}_{t_{0}}m(\tau )u^{r}(\tau)\Delta\tau \biggr]\Delta s,\quad t\in\mathbb{T}^{k} \end{aligned}$$
(I)

and

$$\begin{aligned} u^{p}(t)\leq{}& a(t)+b(t)\int^{t}_{t_{0}}w(t,s) \biggl[f(s)u^{p}(s)+g(s)u^{q}(s)+h_{1}(s)u^{\lambda_{1}} \bigl(\sigma(s)\bigr) \\ &{}-h_{2}(s)u^{\lambda_{2}}\bigl(\sigma(s)\bigr)+l(s)+\int ^{s}_{t_{0}}m(\tau )u^{r}(\tau)\Delta\tau \biggr]\Delta s,\quad t\in\mathbb{T}^{k}, \end{aligned}$$
(II)

where \(p\geq q>0\), \(p\geq r>0\), \(0<\lambda_{1}<p<\lambda_{2}\), p, q, r, \(\lambda_{1}\), and \(\lambda_{2}\) are real constants, \(u,a,b,f,g,h_{1},h_{2},l,m:\mathbb{T}^{k}\rightarrow\mathbb{R}_{+}\) are rd-continuous functions, and \(w:\mathbb{T}\times \mathbb{T}^{k}\rightarrow\mathbb{R}\) is a continuous function.

2 Main results

In what follows, ℤ denotes the set of integers, \(\mathbb{N}_{0}\) denotes the set of nonnegative integers, \(\mathrm{C}_{\mathrm{rd}}\) denotes the set of rd-continuous functions. We say that a function \(p: \mathbb{T}\rightarrow\mathbb{R}\) is regressive provided \(1+\mu(t)p(t)\neq0\), for all \(t\in\mathbb{T}^{k}\). The set of all regressive and rd-continuous functions will be denoted in this paper by ℛ, and \(\mathcal{R}^{+}=\{p\in \mathcal{R}:1+\mu(t)p(t)>0, \mbox{for all } t\in\mathbb{T}\}\).

The following lemmas are very useful in the proof of our main results.

Lemma 2.1

[2, Theorem 6.1]

Let \(u, b\in\mathrm{C}_{\mathrm{rd}} \) and \(a\in\mathcal{R}^{+}\). Then

$$u^{\Delta}(t)\leq a(t)u(t)+b(t), \quad\textit{for all } t\in\mathbb{T} $$

yields

$$u(t)\leq u(t_{0})e_{a}(t,t_{0})+\int ^{t}_{t_{0}}b(\tau)e_{a}\bigl(t,\sigma(\tau )\bigr)\Delta \tau, \quad\textit{for all } t\in\mathbb{T}. $$

Lemma 2.2

[2, Theorem 1.117]

Let \(t_{0}\in\mathbb {T}^{k}\) and \(w:\mathbb{T}\times\mathbb{T}^{k}\rightarrow\mathbb{R}\) be continuous at \((t,t)\), \(t\in\mathbb{T}^{k}\) with \(t>t_{0}\). Assume that \(w^{\Delta}(t,\cdot)\) is rd-continuous on \([t_{0},\sigma(t)]\). Suppose that, for each \(\varepsilon>0\), there exists a neighborhood \({\mathcal{U}}\) of t, independent of \(\tau\in[t_{0},\sigma(t)]\), such that

$$\bigl|w\bigl(\sigma(t),\tau\bigr)-w(s,\tau)-w^{\Delta}(t,\tau) \bigl( \sigma(t)-s\bigr)\bigr|\leq \varepsilon\bigl|\sigma(t)-s\bigr|, \quad\textit{for all } s\in{\mathcal{U}}, $$

where \(w^{\Delta}\) denotes the derivative of w with respect to the first variable. Then

$$v(t)=\int^{t}_{t_{0}}w(t,\tau)\Delta\tau $$

implies that

$$v^{\Delta}(t)=\int^{t}_{t_{0}}w^{\Delta}(t, \tau)\Delta\tau+w\bigl(\sigma(t),t\bigr). $$

Lemma 2.3

[5, Lemma 2.1]

Assume that \(a\geq0\) and \(p\geq q>0\). Then

$$a^{q/p}\leq\frac{q}{p}K^{(q-p)/p}a+\frac{p-q}{p}K^{q/p} $$

for any \(K>0\).

Lemma 2.4

Let u be a nonnegative function, \(0<\lambda_{1}<p<\lambda_{2}, c_{1}\geq0, c_{2}> 0\), \(k_{1}>0\), and \(k_{2}\geq0\). Then, for \(i=1,2\),

$$(-1)^{i+1}c_{i}u^{\lambda_{i}}+(-1)^{i}k_{i}u^{p} \leq\theta_{i}(\lambda _{i},c_{i},k_{i},p), $$

where

$$\theta_{i}(\lambda_{i},c_{i},k_{i},p)=(-1)^{i} \biggl(\frac{\lambda _{i}}{p}-1 \biggr) \biggl(\frac{\lambda_{i}}{p} \biggr)^{\frac{\lambda _{i}}{p-\lambda_{i}}} c^{\frac{p}{p-\lambda_{i}}}_{i}k^{\frac{\lambda_{i}}{\lambda_{i}-p}}_{i}. $$

Proof

Set \(F_{i}(u)=(-1)^{i+1}c_{i}u^{\lambda _{i}}+(-1)^{i}k_{i}u^{p}\). It is not difficult to verify that \(F_{i}\) obtains its maximum at \(u=({\lambda_{i}c_{i}}/{(k_{i}p}))^{{1}/{(p-\lambda_{i})}}\) and

$$(F_{i})_{\max}=(-1)^{i} \biggl(\frac{\lambda_{i}}{p}-1 \biggr) \biggl(\frac {\lambda_{i}}{p} \biggr)^{\frac{\lambda_{i}}{p-\lambda_{i}}} c^{\frac{p}{p-\lambda_{i}}}_{i}k^{\frac{\lambda_{i}}{\lambda _{i}-p}}_{i}\quad \mbox{for } i=1,2. $$

The proof is complete. □

Theorem 2.1

Assume that \(u,a,b,f,g,h_{1},h_{2},l,m:\mathbb{T}^{k}\rightarrow\mathbb{R}_{+}\) are rd-continuous functions. Then, for any rd-continuous functions \(k_{1}(t)>0\) and \(k_{2}(t)\geq0\) on \(\mathbb{T}^{k}\) satisfying \(k(t)=k_{1}(t)-k_{2}(t)\geq0\) and \(\mu(t)k(t)b(\sigma(t))<1\) for \(t\in\mathbb{T}^{k}\), the inequality (I) implies that

$$ u(t)\leq \biggl\{ a(t)+b(t)\int^{t}_{t_{0}}e_{A\oplus B} \bigl(t,\sigma(s)\bigr)D(s)\Delta s \biggr\} ^{1/p} \quad\textit{for any } K>0, t\in\mathbb{T}^{k}, $$
(2.1)

where

$$\begin{aligned}& A(t)=b(t)f(t)+\frac{q}{p}K^{(q-p)/p}b(t)g(t)+ \frac{r}{p}K^{(r-p)/p}\int^{t}_{t_{0}}b( \tau)m(\tau)\Delta\tau,\\& B(t)=\frac{k(t)b(\sigma(t))}{1-\mu(t)k(t)b(\sigma(t))}, \qquad D(t)=\bigl(1+\mu (t)B(t)\bigr)C(t), \end{aligned}$$

and

$$\begin{aligned} C(t)={}&g(t) \biggl(\frac{q}{p}K^{(q-p)/p}a(t)+ \frac{p-q}{p}K^{q/p} \biggr) +\int^{t}_{t_{0}}m( \tau) \biggl(\frac{r}{p}K^{(r-p)/p}a(\tau)+\frac {p-r}{p}K^{r/p} \biggr)\Delta\tau\\ &{}+\theta_{1}(\lambda_{1},h_{1},k_{1},p)+ \theta_{2}(\lambda_{2},h_{2},k_{2},p)+a(t)f(t)+a \bigl(\sigma (t)\bigr)k(t)+l(t). \end{aligned}$$

Proof

Define a function y by

$$\begin{aligned} y(t)={}&\int^{t}_{t_{0}} \biggl[f(s)u^{p}(s)+g(s)u^{q}(s)+h_{1}(s)u^{\lambda _{1}} \bigl(\sigma(s)\bigr)-h_{2}(s)u^{\lambda_{2}}\bigl(\sigma(s)\bigr)\\ &{}+l(s)+\int^{s}_{t_{0}}m(\tau)u^{r}(\tau) \Delta\tau \biggr]\Delta s,\quad t\in\mathbb{T}^{k}. \end{aligned}$$

Then \(y(t_{0})=0\) and

$$ u(t)\leq\bigl(a(t)+b(t)y(t)\bigr)^{1/p}. $$
(2.2)

On the basis of a straightforward computation and Lemma 2.4, we have

$$\begin{aligned} y^{\Delta}(t)={}&f(t)u^{p}(t)+g(t)u^{q}(t)+\int ^{t}_{t_{0}}m(\tau )u^{r}(\tau)\Delta \tau+h_{1}(t)u^{\lambda_{1}}\bigl(\sigma (t)\bigr) \\ &{}-h_{2}(t)u^{\lambda_{2}} \bigl(\sigma(t)\bigr)+l(t) \\ \leq{}& f(t)u^{p}(t)+g(t)u^{q}(t)+\int^{t}_{t_{0}}m( \tau)u^{r}(\tau )\Delta\tau+k(t)u^{p}\bigl(\sigma(t)\bigr) \\ &{}+\theta_{1}(\lambda _{1},h_{1},k_{1},p)+ \theta_{2}(\lambda_{2},h_{2},k_{2},p) +l(t). \end{aligned}$$
(2.3)

By virtue of Lemma 2.3, for any \(K > 0\), we obtain

$$ \begin{aligned} & u^{q}(t)\leq\bigl(a(t)+b(t)y(t)\bigr)^{q/p}\leq \frac{q}{p}K^{(q-p)/p}\bigl(a(t)+b(t)y(t)\bigr)+\frac{p-q}{p}K^{q/p},\\ &u^{r}(t)\leq\bigl(a(t)+b(t)y(t)\bigr)^{r/p}\leq \frac{r}{p}K^{(r-p)/p}\bigl(a(t)+b(t)y(t)\bigr)+\frac{p-r}{p}K^{r/p}. \end{aligned} $$
(2.4)

Using inequalities (2.2)-(2.4), we conclude that

$$\begin{aligned} y^{\Delta}(t)\leq{}& f(t)u^{p}(t)+g(t)u^{q}(t)+ \int^{t}_{t_{0}}m(\tau)u^{r}(\tau)\Delta \tau+k(t)u^{p}\bigl(\sigma(t)\bigr)\\ &{}+\theta _{1}(\lambda_{1},h_{1},k_{1},p)+ \theta_{2}(\lambda_{2},h_{2},k_{2},p) +l(t)\\ \leq{}& f(t) \bigl(a(t)+b(t)y(t)\bigr)+g(t) \biggl(\frac {q}{p}K^{(q-p)/p} \bigl(a(t)+b(t)y(t)\bigr)+\frac{p-q}{p}K^{q/p} \biggr)\\ &{}+\int^{t}_{t_{0}}m(\tau) \biggl(\frac{r}{p}K^{(r-p)/p} \bigl(a(\tau)+b(\tau )y(\tau)\bigr)+\frac{p-r}{p}K^{r/p} \biggr) \Delta\tau\\ &{}+k(t) \bigl[a\bigl(\sigma(t)\bigr)+b\bigl(\sigma(t)\bigr)y\bigl(\sigma(t)\bigr) \bigr]+\theta _{1}(\lambda_{1},h_{1},k_{1},p)+ \theta_{2}(\lambda_{2},h_{2},k_{2},p)+l(t)\\ \leq{}& f(t) \bigl(a(t)+b(t)y(t)\bigr)+g(t) \biggl(\frac {q}{p}K^{(q-p)/p} \bigl(a(t)+b(t)y(t)\bigr)+\frac{p-q}{p}K^{q/p} \biggr)\\ &{}+ \biggl(\frac{r}{p}K^{(r-p)/p}\int^{t}_{t_{0}}b( \tau)m(\tau)\Delta\tau \biggr) y(t)\\ &{}+\int^{t}_{t_{0}}m( \tau) \biggl(\frac{r}{p}K^{(r-p)/p}a(\tau )+\frac{p-r}{p}K^{r/p} \biggr)\Delta\tau\\ &{}+k(t)\bigl[a\bigl(\sigma(t)\bigr)+b\bigl(\sigma (t)\bigr)y\bigl(\sigma(t)\bigr) \bigr]+\theta_{1}(\lambda_{1},h_{1},k_{1},p) +\theta_{2}(\lambda_{2},h_{2},k_{2},p)+l(t)\\ ={}&A(t)y(t)+\frac{B(t)}{1+\mu(t)B(t)}y\bigl(\sigma(t)\bigr)+C(t)\\ ={}&A(t)y(t)+\frac{B(t)}{1+\mu (t)B(t)}\bigl(y(t)+\mu(t)y^{\Delta}(t)\bigr)+C(t), \end{aligned}$$

which implies that

$$\frac{1}{1+\mu(t)B(t)}y^{\Delta}(t)\leq \biggl(A(t)+\frac{B(t)}{1+\mu (t)B(t)} \biggr)y(t)+C(t), $$

that is,

$$y^{\Delta}(t)\leq(A\oplus B) (t)y(t)+D(t),\quad t\in\mathbb{T}^{k}, $$

where \(D(t)=[1+\mu(t)B(t)]C(t)\). Note that \(y,D\in\mathrm{C}_{\mathrm{rd}}\) and \(A\oplus B\in\Re^{+}\). By Lemma 2.1, we get the desired inequality (2.1). This completes the proof. □

Remark 2.1

If \(p=1\) and \(g(t)=m(t)=l(t)=0\), then (2.1) reduces to the inequality established in Theorem 1.2.

Remark 2.2

If \(m(t)=0\), \(l(t)=0\), and \(h_{i}(t)=0\) (\(i=1,2\)), then Theorem 2.1 reduces to Theorem 1.1.

Remark 2.3

Theorem 2.1 can be applied on an arbitrary time scale. Thus, we immediately obtain the following corollaries for some peculiar time scales.

Corollary 2.1

Let \(\mathbb{T}=\mathbb{R}\) and assume that \(u,a,b,f,g,h_{1},h_{2},l,m:[t_{0},\infty)\rightarrow\mathbb{R}_{+}\) are continuous. Then, for any continuous functions \(k_{1}(t)>0\) and \(k_{2}(t)\geq0\) satisfying \(k(t)=k_{1}(t)-k_{2}(t)\geq0\) on \([t_{0},\infty)\), the inequality (I) yields

$$u(t)\leq \biggl\{ a(t)+b(t)\int^{t}_{t_{0}}\exp \biggl(\int^{t}_{s}\bigl(A_{1}(\tau )+B_{1}(\tau)\bigr)\,d\tau \biggr)C_{1}(s)\,ds \biggr\} ^{1/p}\quad\textit{for any } K>0, t\geq t_{0}, $$

where

$$A_{1}(t)=b(t)f(t)+\frac{q}{p}K^{(q-p)/p}b(t)g(t)+ \frac {r}{p}K^{(r-p)/p}\int^{t}_{t_{0}}b( \tau)m(\tau)\,d\tau,\qquad B_{1}(t)=k(t)b(t), $$

and

$$\begin{aligned} C_{1}(t)={}&g(t) \biggl(\frac{q}{p}K^{(q-p)/p}a(t)+ \frac {p-q}{p}K^{q/p} \biggr) +\int^{t}_{t_{0}}m( \tau) \biggl(\frac{r}{p}K^{(r-p)/p}a(\tau)+\frac {p-r}{p}K^{r/p} \biggr)\,d\tau\\ &{}+\theta_{1}(\lambda_{1},h_{1},k_{1},p)+ \theta_{2}(\lambda_{2},h_{2},k_{2},p)+a(t) \bigl(f(t)+k(t)\bigr)+l(t). \end{aligned}$$

Corollary 2.2

Let \(\mathbb{T}=\mathbb{Z}\) and \(u,a,b,f,g,h_{1},h_{2},l,m:\mathbb{N}_{0}\rightarrow \mathbb{R}_{+}\). Then, for any functions \(k_{1}(t)>0\) and \(k_{2}(t)\geq0\) satisfying \(k(t)=k_{1}(t)-k_{2}(t)\geq0\) and \(k(t)b(t+1)<1\) on \(\mathbb{N}_{0}\), the inequality (I) implies that

$$u(t)\leq \Biggl\{ a(t)+b(t)\sum^{t-1}_{s=t_{0}} \Biggl(\prod^{t-1}_{\tau =s+1}\bigl(1+(A_{2} \oplus B_{2}) (\tau)\bigr) \Biggr)D_{2}(s) \Biggr\} ^{1/p} \quad\textit{for any } K>0, t\in \mathbb{N}_{0}, $$

where

$$\begin{aligned}& A_{2}(t)=b(t)f(t)+\frac{q}{p}K^{(q-p)/p}b(t)g(t)+ \frac{r}{p}K^{(r-p)/p}\sum^{t-1}_{s=t_{0}}b(s)m(s),\\& B_{2}(t)=\frac{k(t)b(t+1)}{1-k(t)b(t+1)},\qquad D_{2}(t)= \bigl(1+B_{2}(t)\bigr)C_{2}(t), \end{aligned}$$

and

$$\begin{aligned} C_{2}(t)={}&g(t) \biggl(\frac{q}{p}K^{(q-p)/p}a(t)+ \frac {p-q}{p}K^{q/p} \biggr) +\sum^{t-1}_{s=t_{0}}m(s) \biggl(\frac{r}{p}K^{(r-p)/p}a(s)+\frac {p-r}{p}K^{r/p} \biggr)\\ &{}+\theta_{1}(\lambda_{1},h_{1},k_{1},p)+ \theta_{2}(\lambda _{2},h_{2},k_{2},p)+a(t)f(t)+a(t+1)k(t)+l(t). \end{aligned}$$

Theorem 2.2

Assume that \(u,a,b,f,g,h_{1},h_{2},l,m:\mathbb{T}^{k}\rightarrow\mathbb{R}_{+}\) are rd-continuous functions, \(w(t,s)\) is defined as in Lemma 2.2 such that \(w(\sigma(t),t)\geq0\) and \(w^{\Delta}(t,s)\geq0\) for \(t,s\in\mathbb{T}\) with \(s\leq t\). Then, for any rd-continuous functions \(k_{1}(t)>0\) and \(k_{2}(t)\geq0\) on \(\mathbb{T}^{k}\) satisfying \(k(t)=k_{1}(t)-k_{2}(t)\geq0\) and \(\mu(t)\hat{B}(t)<1\) for \(t\in\mathbb{T}^{k}\) with

$$\hat{B}(t)=w\bigl(\sigma(t),t\bigr)k(t)b\bigl(\sigma(t)\bigr)+ \int ^{t}_{t_{0}}w^{\Delta}(t,s)k(s)b\bigl(\sigma(s) \bigr)\Delta s, $$

the inequality (II) implies that

$$ u(t)\leq \biggl\{ a(t)+b(t)\int^{t}_{t_{0}}e_{\tilde{A}\oplus \tilde{B}} \bigl(t,\sigma(s)\bigr)\tilde{D}(s)\Delta s \biggr\} ^{1/p} \quad\textit{for any } K>0, t\in \mathbb{T}^{k}, $$
(2.5)

where

$$\begin{aligned}& \tilde{A}(t)=w\bigl(\sigma(t),t\bigr)A(t)+\int^{t}_{t_{0}}w^{\Delta }(t,s)A(s)\Delta s, \qquad\tilde{B}(t)=\frac{\hat{B}(t)}{1-\mu(t)\hat{B}(t)},\\& \tilde {C}(t)=w\bigl(\sigma(t),t\bigr)C(t)+\int^{t}_{t_{0}}w^{\Delta}(t,s)C(s) \Delta s,\qquad \tilde{D}(t)=\bigl(1+\mu(t)\tilde{B}(t)\bigr)\tilde{C}(t), \end{aligned}$$

A and C are defined as in Theorem 2.1.

Proof

Define a new function z by

$$\begin{aligned} z(t)={}&\int^{t}_{t_{0}}w(t,s) \biggl[f(s)u^{p}(s)+g(s)u^{q}(s)+h_{1}(s)u^{\lambda_{1}} \bigl(\sigma (s)\bigr)-h_{2}(s)u^{\lambda_{2}}\bigl(\sigma(s)\bigr) \\ &{}+l(s)+\int^{s}_{t_{0}}m(\tau)u^{r}(\tau) \Delta\tau \biggr]\Delta s,\quad t\in\mathbb{T}^{k}. \end{aligned}$$
(2.6)

Then \(z(t_{0})=0\) and

$$ u(t)\leq\bigl(a(t)+b(t)z(t)\bigr)^{1/p}. $$
(2.7)

Using Lemmas 2.2-2.4 and combining (2.6) and (2.7), we deduce that

$$\begin{aligned} z^{\Delta}(t)={}&w\bigl(\sigma(t),t\bigr) \biggl[f(t)u^{p}(t)+g(t)u^{q}(t)+h_{1}(t)u^{\lambda_{1}} \bigl(\sigma(t)\bigr) -h_{2}(t)u^{\lambda_{2}}\bigl(\sigma(t)\bigr)\\ &{}+l(t)+\int^{t}_{t_{0}}m(\tau )u^{r}(\tau) \Delta\tau \biggr] \\ &{}+ \int^{t}_{t_{0}}w^{\Delta}(t,s) \biggl[f(s)u^{p}(s)+g(s)u^{q}(s)+h_{1}(s)u^{\lambda_{1}} \bigl(\sigma (s)\bigr)-h_{2}(s)u^{\lambda_{2}}\bigl(\sigma(s)\bigr) \\ &{}+l(s)+\int^{s}_{t_{0}}m(\tau)u^{r}(\tau) \Delta\tau \biggr]\Delta s \\ \leq{}& \biggl(w\bigl(\sigma(t),t\bigr)k(t)b\bigl(\sigma(t)\bigr)+ \int ^{t}_{t_{0}}w^{\Delta}(t,s)k(s)b\bigl(\sigma(s) \bigr)\Delta s \biggr)z\bigl(\sigma(t)\bigr) \\ &{}+ \biggl(w\bigl(\sigma(t),t\bigr)A(t)+\int^{t}_{t_{0}}w^{\Delta}(t,s)A(s) \Delta s \biggr)z(t)\\ &{}+w\bigl(\sigma(t),t\bigr)C(t)+\int^{t}_{t_{0}}w^{\Delta }(t,s)C(s) \Delta s \\ ={}&\tilde{A}(t)z(t)+\frac{\tilde{B}(t)}{1+\mu(t)\tilde{B}(t)}z\bigl(\sigma (t)\bigr) +\tilde{C}(t),\quad t\in \mathbb{T}^{k}. \end{aligned}$$

Similar to the proof of Theorem 2.1, we obtain (2.5). The proof is complete. □

Remark 2.4

The inequality established in Theorem 2.2 generalizes that reported in [7, Theorem 3.2].

On the basis of Theorem 2.2, the following two corollaries are easily obtained.

Corollary 2.3

Let \(\mathbb{T}=\mathbb{R}\) and assume that \(u,a,b,f,g,h_{1},h_{2},l,m:[t_{0},\infty)\rightarrow\mathbb{R}_{+}\) are continuous. Suppose further that \(w(t,s)\) and its partial derivative \(\partial w(t,s)/\partial t\) are real-valued nonnegative continuous functions for \(t,s\in[t_{0},\infty)\) with \(s\leq t\). Then, for any continuous functions \(k_{1}(t)>0\) and \(k_{2}(t)\geq0\) on \([t_{0},\infty)\) satisfying \(k(t)=k_{1}(t)-k_{2}(t)\geq0\), the inequality (II) implies that

$$u(t)\leq \biggl\{ a(t)+b(t)\int^{t}_{t_{0}}\exp \biggl(\int^{t}_{s}\bigl(\tilde {A}_{1}( \tau)+\tilde{B}_{1}(\tau)\bigr)\,d\tau \biggr)\tilde{C}_{1}(s)\,ds \biggr\} ^{1/p}\quad\textit{for any } K>0, t\geq t_{0}, $$

where

$$\begin{aligned}& \tilde{A}_{1}(t)=w(t,t)A_{1}(t)+\int ^{t}_{t_{0}}\frac{\partial w (t,s)}{\partial t}A_{1}(s)\,ds, \\& \tilde{B}_{1}(t)=w(t,t)k(t)b(t)+ \int^{t}_{t_{0}} \frac{\partial w(t,s)}{\partial t}k(s)b(s)\,ds, \\& \tilde{C}_{1}(t)=w(t,t)C_{1}(t)+\int^{t}_{t_{0}} \frac {\partial w(t,s)}{\partial t}C_{1}(s)\,ds, \end{aligned}$$

\(A_{1}\) and \(C_{1}\) are the same as in Corollary 2.1.

Corollary 2.4

Let \(\mathbb{T}=\mathbb{Z}\) and \(u,a,b,f,g,h_{1},h_{2},l,m:\mathbb{N}_{0}\rightarrow \mathbb{R}_{+}\). Assume that \(w(t,s)\) and \(\Delta_{1}w(t,s)\) are real-valued nonnegative functions for \(t,s \in\mathbb{N}_{0}\) with \(s\leq t\). Then, for any functions \(k_{1}(t)>0\) and \(k_{2}(t)\geq0\) satisfying \(k(t)=k_{1}(t)-k_{2}(t)\geq0\) and \(\hat{B}_{2}(t)<1\) on \(\mathbb{N}_{0}\) with

$$\hat{B}_{2}(t)=w(t+1,t)k(t)b(t+1)+ \sum^{t-1}_{s=t_{0}} \Delta_{1}w(t,s)k(s)b(s+1), $$

the inequality (II) yields

$$u(t)\leq \Biggl\{ a(t)+b(t)\sum^{t-1}_{s=t_{0}} \Biggl(\prod^{t-1}_{\tau =s+1}\bigl(1+( \tilde{A}_{2}\oplus \tilde{B}_{2}) (\tau)\bigr) \Biggr) \tilde{D}_{2}(s) \Biggr\} ^{1/p} \quad\textit{for any } K>0, t\in \mathbb{N}_{0}, $$

where \(\Delta_{1}w(t,s)=w(t+1,s)-w(t,s)\) for \(t,s\in\mathbb{N}_{0}\) with \(s\leq t\),

$$\begin{aligned}& \tilde{A}_{2}(t)=w(t+1,t)A_{2}(t)+\sum ^{t-1}_{s=t_{0}}\Delta_{1}w (t,s)A_{2}(s),\qquad \tilde{B}_{2}(t)=\frac{\hat{B}_{2}(t)}{1-\hat{B}_{2}(t)},\\& \tilde{C}_{2}(t)=w(t+1,t)C_{2}(t)+\sum ^{t-1}_{s=t_{0}}\Delta_{1}w(t,s)C_{2}(s),\qquad \tilde{D}_{2}(t)=\bigl(1+\tilde{B}_{2}(t)\bigr) \tilde{C}_{2}(t), \end{aligned}$$

\(A_{2}\) and \(C_{2}\) are defined as in Corollary 2.2.

Remark 2.5

By choosing possible values of \(k_{1}\) and \(k_{2}\), one can derive many explicit estimates for dynamic integral inequalities of types (I) and (II). For instance, if we let \(k_{1}=k_{2}>0\), then \(B(t)=\tilde{B}(t)=0\). In this case, Theorems 2.1 and 2.2 take simpler forms.

3 Example

The following example illustrates possible applications of our main results.

Example 3.1

Consider the following dynamic equation

$$ \begin{aligned} &\bigl[u^{p}(t)\bigr]^{\Delta}=F \biggl(t,U\bigl(t,u(t),u\bigl( \sigma(t)\bigr)\bigr),\int^{t}_{t_{0}}H\bigl(\tau,u( \tau)\bigr)\Delta\tau \biggr),\\ & u^{p}(t_{0})=C_{0},\quad t\in \mathbb{T}^{k}, \end{aligned} $$
(3.1)

where \(C_{0}\) is a real constant, \(F, U:\mathbb{T}^{k}\times \mathbb{R}\times\mathbb{R}\rightarrow\mathbb{R}\), \(H:\mathbb{T}^{k}\times\mathbb{R}\rightarrow\mathbb{R}\) are continuous functions. Assume that, for \(t\in\mathbb{T}^{k}\),

$$ \begin{aligned} &\bigl|F(t,U,V)\bigr|\leq|U|+|V|,\\ &\bigl|U(t,x,y)\bigr|\leq f(t)|x|^{p}+g(t)|x|^{q}+h_{1}(t)|y|^{\lambda _{1}}-h_{2}(t)|y|^{\lambda_{2}},\\ &\bigl|H(t,z)\bigr|\leq m(t)|z|^{r}, \end{aligned} $$
(3.2)

where \(p\geq q>0\), \(p\geq r>0\), \(0<\lambda_{1}<p<\lambda_{2}\), p, q, r, \(\lambda_{1}\), and \(\lambda_{2}\) are real constants, f, g, \(h_{1}\), \(h_{2}\), and m are nonnegative rd-continuous functions on \(\mathbb{T}^{k}\). Then every solution u of (3.1) satisfies, for any \(K>0\),

$$ \bigl|u(t)\bigr|\leq \biggl\{ |C_{0}|+\int^{t}_{t_{0}}e_{A} \bigl(t,\sigma(s)\bigr)C(s)\Delta s \biggr\} ^{1/p},\quad t\in \mathbb{T}^{k}, $$
(3.3)

where

$$A(t)=f(t)+\frac{q}{p}K^{(q-p)/p}g(t)+\frac{r}{p}K^{(r-p)/p} \int^{t}_{t_{0}}m(\tau)\Delta\tau $$

and

$$\begin{aligned} C(t)={}& \biggl(\frac{q}{p}K^{(q-p)/p}|C_{0}|+ \frac {p-q}{p}K^{q/p} \biggr)g(t) + \biggl(\frac{r}{p}K^{(r-p)/p}|C_{0}|+ \frac{p-r}{p}K^{r/p} \biggr)\int^{t}_{t_{0}}m( \tau)\Delta\tau\\ &{}+\theta_{1}(\lambda_{1},h_{1},k_{1},p)+ \theta_{2}(\lambda_{2},h_{2},k_{2},p)+|C_{0}|f(t), \end{aligned}$$

where \(k_{1}(t)>0\) and \(k_{2}(t)\geq0\) are any rd-continuous functions satisfying \(k(t)=k_{1}(t)-k_{2}(t)=0\) for \(t\in \mathbb{T}^{k}\).

As a matter of fact, the solution u of (3.1) satisfies the following equivalent equation

$$ u^{p}(t)=C_{0}+\int^{t}_{t_{0}}F \biggl(s,U\bigl(s,u(s),u\bigl(\sigma(s)\bigr)\bigr),\int^{s}_{t_{0}}H \bigl(\tau,u(\tau)\bigr)\Delta\tau \biggr)\Delta s,\quad t\in\mathbb {T}^{k}. $$
(3.4)

It follows now from (3.2) and (3.4) that

$$\begin{aligned} \bigl|u^{p}(t)\bigr|\leq{}&|C_{0}|+\int^{t}_{t_{0}} \biggl\vert F \biggl(s,U\bigl(s,u(s),u\bigl(\sigma(s)\bigr)\bigr),\int ^{s}_{t_{0}}H\bigl(\tau,u(\tau)\bigr)\Delta\tau \biggr)\biggr\vert \Delta s \\ \leq{}&|C_{0}|+\int^{t}_{t_{0}} \biggl[f(s)\bigl|u(s)\bigr|^{p}+g(s)\bigl|u(s)\bigr|^{q}+\int^{s}_{t_{0}}m( \tau)\bigl|u(\tau)\bigr|^{r}\Delta\tau \\ &{}+h_{1}(s)\bigl|u\bigl(\sigma (s)\bigr)\bigr|^{\lambda_{1}}-h_{2}(s)\bigl|u \bigl(\sigma(s)\bigr)\bigr|^{\lambda_{2}} \biggr]\Delta s,\quad t\in\mathbb{T}^{k}. \end{aligned}$$
(3.5)

Using Theorem 2.1 in (3.5), we conclude that (3.3) is satisfied.