Theoretical and experimental investigation of hydro-mechanical deep drawing of hemi-prolate spheroid cups

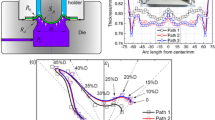
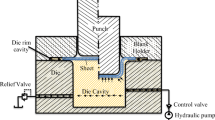
Abstract

In this paper, a theoretical model was proposed to stress analysis and calculate the critical fluid pressure path corresponding to rupture instability in hydro-mechanical deep drawing (HMDD) of hemi-prolate spheroid (HPS) cups. This model is based on Barlat and Lian yield criterion and maximum drawing force condition was utilized to rupture instability analysis. The effects of material and process parameters were investigated on critical fluid pressure path. The results demonstrated that maximum permissible fluid pressure is increased by enhancement of sheet thickness, strain hardening exponent, anisotropy and reduction of friction coefficient. Decrease the difference between semi-major and semi-minor axis of the hemi-prolate spheroid, enlarges safe zone. Finally, a serial of HMDD experiments were accomplished to verify the theoretical critical fluid pressure path. Theoretical results agree well with experimental data.

Appendix: The proofs of geometrical relations

• (a) Calculation of b, $r_{0}^{\text{I}}$ and $r_{0}^{\text{II}}$:

  Volume of zone I:

  $$V_{\text{I}} = \pi \left[ {b^{2} - \left( {x_{0} + \left( {\rho + t} \right)\cos \alpha } \right)^{2} } \right]\,t$$

  (41)

  Volume of zone II:

  From the Pappus–Guldinus theorem [24], the area of a surface of revolution is equal to:

  $$S = 2\pi L\bar{r}$$

  (42)

  where L and $\bar{r}$ are the length of the generating curve and the distance of the centroid of the curve from axis of revolution, respectively. As shown in Fig. 18a, the value of L for zone II is:

  $$L = \widehat{AB} = \left( {\rho + t} \right)\left( {\frac{\pi }{2} - \alpha } \right)$$

  (43)

  Fig. 18

  

  a Generating curve of zone II and its centroid position. b Centroid of a circular arc

  According to Fig. 18b and using trigonometric relations, the distance of the centroid of the arc $\widehat{AB}$ from the y-axis ($\bar{r}$) is determined through the following relation:

  $$\bar{r} = x_{0} + \left( {\rho + t} \right)\cos \alpha - \frac{{\left( {\rho + t} \right)\sin \left( { \frac{\pi }{4} - \frac{\alpha }{2} } \right)}}{{ \frac{\pi }{4} - \frac{\alpha }{2} }}\cos \left( { \frac{\pi }{4} + \frac{\alpha }{2} } \right)$$

  (44)

  Substituting Eqs. (43) and (44) into Eq. (42), the surface of the product in zone II can be calculated as follows:

  $$\begin{aligned}S^{\text{II}}& = 2\pi \left[ {\left( {\rho + t} \right)\left( {\frac{\pi }{2} - \alpha } \right)} \right]\left\{ x_{0} + \left( {\rho + t} \right)\cos \alpha \right. \\ &\quad \left.- \frac{{\left( {\rho + t} \right)\sin \left( { \frac{\pi }{4} - \frac{\alpha }{2} } \right)}}{{ \frac{\pi }{4} - \frac{\alpha }{2} }}\cos \left( { \frac{\pi }{4} + \frac{\alpha }{2} } \right) \right\} \end{aligned}$$

  (45)

  Finally, the volume of zone II is:

  $$\begin{aligned}V^{\text{II}}& = S^{\text{II}} \times t = 2\pi \left[ {\left( {\rho + t} \right)\left( {\frac{\pi }{2} - \alpha } \right)} \right]\\&\quad\left\{ {x_{0} + \left( {\rho + t} \right)\cos \alpha - \frac{{\left( {\rho + t} \right)\sin \left( { \frac{\pi }{4} - \frac{\alpha }{2} } \right)}}{{ \frac{\pi }{4} - \frac{\alpha }{2} }}\cos \left( { \frac{\pi }{4} + \frac{\alpha }{2} } \right)} \right\}t \end{aligned}$$

  (46)

  Volume of zone III:

  The area of the surface of revolution about y-axis is [24]:

  $$S = \int {2\pi x\sqrt {1 + \left( {\frac{{{\text{d}}x}}{{{\text{d}}y}}} \right)^{2} {\text{d}}y} }$$

  (47)

  Differentiation from Eq. (1) with respect to x and substituting into Eq. (47) yields:

  $$S^{\text{III}} = \mathop \int \limits_{t}^{{y_{0} }} 2\pi B\left( {\sqrt {1 - \frac{1}{{A^{2} }}(y - \beta )^{2} } \sqrt {1 + \frac{{B^{2} }}{{A^{2} }} \frac{{(y - \beta )^{2} }}{{A^{2} - (y - \beta )^{2} }}} } \right)\,{\text{d}}y$$

  (48)

  Eventually, the volume of zone III is equal to:

  $$\begin{aligned} V^{\text{III}} & = S^{\text{III}} \times t \\ & = \left[ {\mathop \int \limits_{t}^{{y_{0} }} 2\pi B\left( {\sqrt {1 - \frac{1}{{A^{2} }}(y - \beta )^{2} } \sqrt {1 + \frac{{B^{2} }}{{A^{2} }} \frac{{(y - \beta )^{2} }}{{A^{2} - (y - \beta )^{2} }}} } \right)\,{\text{d}}y} \right]t \\ \end{aligned}$$

  (49)

  The total volume of the formed cup is equal to V _t  = V ^I + V ^II + V ^III. Equating the volume of initial blank and volume of the formed cup (volume constancy rule), the current outer radius of the cup, see Fig. 17, can be determined as follows:

  $$\begin{aligned} b^{2} & = b_{0}^{2} + \left[ {x_{0} + \left( {\rho + t} \right)\cos \alpha } \right]^{2} \\ & \quad - 2(\rho + t)\left( {\frac{\pi }{2} - \alpha } \right)\left[\vphantom{\frac{{\left( {\rho + t} \right)\sin \left( { \frac{\pi }{4} - \frac{\alpha }{2} } \right)}}{{ \frac{\pi }{4} - \frac{\alpha }{2} }}} {x_{0} + \left( {\rho + t} \right)\cos \alpha } \right. \\ {\kern 1pt} & \left. {\quad - \frac{{\left( {\rho + t} \right)\sin \left( { \frac{\pi }{4} - \frac{\alpha }{2} } \right)}}{{ \frac{\pi }{4} - \frac{\alpha }{2} }}\cos \left( { \frac{\pi }{4} + \frac{\alpha }{2} } \right)} \right] \\ & \quad - \mathop \int \limits_{t}^{{y_{0} }} 2B\left( {\sqrt {1 - \frac{1}{{A^{2} }}(y - \beta )^{2} } \sqrt {1 + \frac{{B^{2} }}{{A^{2} }} \frac{{(y - \beta )^{2} }}{{A^{2} - (y - \beta )^{2} }}} } \right) {\text{d}}y \\ \end{aligned}$$

  (50)

  Also, the relation between r and r ₀ can be obtained by replacing r and r ₀ by b and b ₀ respectively, in Eq. (50), as follows:

  $$\begin{aligned} r_{0}^{{{\text{I}}^{2} }} & = r^{2} - \left[ {x_{0} + \left( {\rho + t} \right)\cos \alpha } \right]^{2} + 2(\rho + t)\left( {\frac{\pi }{2} - \alpha } \right)\left[ \vphantom{{\frac{{\left( {\rho + t} \right)\sin \left( { \frac{\pi }{4} - \frac{\alpha }{2} } \right)}}{{ \frac{\pi }{4} - \frac{\alpha }{2} }}}}{x_{0} + \left( {\rho + t} \right)\cos \alpha } \right. \\ & \quad \left. { - \frac{{\left( {\rho + t} \right)\sin \left( { \frac{\pi }{4} - \frac{\alpha }{2} } \right)}}{{ \frac{\pi }{4} - \frac{\alpha }{2} }}\cos \left( { \frac{\pi }{4} + \frac{\alpha }{2} } \right)} \right] \\ & \quad + \mathop \int \limits_{t}^{{y_{0} }} 2B\left( {\sqrt {1 - \frac{1}{{A^{2} }}(y - \beta )^{2} } \sqrt {1 + \frac{{B^{2} }}{{A^{2} }} \frac{{(y - \beta )^{2} }}{{A^{2} - (y - \beta )^{2} }}} } \right){\text{d}}y \\ \end{aligned}$$

  (51)

  With replacing $\frac{\pi }{2}$ by φ in Eq. (46), the volume of the portion of zone II which is located in x ₀ < r < r ^II is calculated through the following relation:

  $$\begin{aligned} V^{\text{II}}_{{x_{0} \to r^{\text{II}} }} & = S^{\text{II}}_{{x_{0} \to r^{\text{II}} }} \times t = 2\pi l\bar{r}t \\ & & = 2\pi \left( {\rho + t} \right)\left( {\varphi - \alpha } \right)\left[ {x_{0} + \left( {\rho + t} \right)\cos \alpha } \right. \\ & \quad \left. { - \frac{{\left( {\rho + t} \right) sin\left( { \frac{\varphi - \alpha }{2} } \right)}}{{ \frac{\varphi - \alpha }{2}}}\cos \left( { \frac{\varphi + \alpha }{2} } \right)} \right]t \\ \end{aligned}$$

  (52)

  where

  $$\begin{aligned} \varphi & = \cos^{ - 1} \left( { \frac{{x_{0} + \left( {\rho + t} \right)\cos \alpha - r}}{\rho + t} } \right) \\& \alpha \le \varphi \le \frac{\pi }{2} \\ \end{aligned}$$

  (53)

  For calculation of r ^II₀ , sum of the volumes of zone III (Eq. (49)) and the portion of zone II which is located in x ₀ < r < r ^II (Eq. (52)) is equated with the volume of a circular blank with r ^II₀ radius. Finally, the following relation is obtained:

  $$\begin{aligned} r_{0}^{{{\text{II}}^{2} }} & = 2\left( {\rho + t} \right)\left( {\varphi - \alpha } \right)\left[ {x_{0} + \left( {\rho + t} \right)\cos \alpha - \frac{{\left( {\rho + t} \right) { \sin }\left( { \frac{\varphi - \alpha }{2} } \right)}}{{ \frac{\varphi - \alpha }{2}}}\cos \left( { \frac{\varphi + \alpha }{2} } \right)} \right] \\ & \quad + \mathop \int \limits_{t}^{{y_{0} }} 2B\left( {\sqrt {1 - \frac{1}{{A^{2} }}(y - \beta )^{2} } \sqrt {1 + \frac{{B^{2} }}{{A^{2} }} \frac{{(y - \beta )^{2} }}{{A^{2} - (y - \beta )^{2} }}} } \right) {\text{d}}y \\ \end{aligned}$$

  (54)

• (b) Calculation of $\rho$:

  according to Fig. 6, the force balance equation along y-axis direction for zone II is:

  $$\mathop \sum \nolimits F_{y} = 0 \Rightarrow P \times A_{y} - \sigma_{{r = x_{0} }}^{\text{II}} \times A_{t} \times \cos \alpha = 0$$

  (55)

  where

  $$\begin{aligned} A_{y} & = \pi \left[ {\left( {x_{0} + \left( {\rho + t} \right)\cos \alpha } \right)^{2} - \left( {x_{0} + t\cos \alpha } \right)^{2} } \right] \hfill \\& A_{t} = 2\pi x_{0} t \hfill \\ \end{aligned}$$

  (56)

  Substituting Eq. (56) into Eq. (55) and algebraic simplification yields a quadratic equation as a function of ρ as follows:

  $$\left( {P\cos \alpha } \right)\rho^{2} + \left( {2tP\cos \alpha + 2x_{0} P} \right)\rho - 2\sigma_{{r = x_{0} }}^{\text{II}} x_{0} t = 0$$

  (57)

  The positive root of Eq. (57) gives:

  $$\rho = \frac{1}{\cos \alpha }\left[ { - \left( {t\cos \alpha + x_{0} } \right) + \sqrt {\left( {t\cos \alpha + x_{0} } \right)^{2} + \frac{{2x_{0} t(\sigma_{r}^{\text{II}} )|_{{r = x_{0} }} \cos \alpha }}{P}} } \right]$$

  (58)

• (c) Calculation of $F_{p}$:

  According to Fig. 7, the applied forces on zone III can be divided to four parts which include:

  • 1. Punch force which is denoted by F _p .

  • 2. The outcome force of radial stress at r = x ₀, which can be determined by the product of the radial stress $\sigma_{r}^{\text{III}} (r)_{{|r = x_{0} }}$ and its cross section area 2πx ₀ t cos α.

  • 3. The outcome force of applied pressure, which can be determined by the product of the pressure and the projected area of the cup along the punch axis $\pi x_{0}^{2} P$.

  • 4. Frictional force between cup wall and punch, which for each element the axial component of friction force is:

    $${\text{d}}F_{f} = \mu P{\text{d}}A\cos \alpha$$

    (59)

  Differentiating Eq. (48) and substituting it into Eq. (49) yields:

  $${\text{d}}F_{f} = \mu P2\pi B\left( {\sqrt {1 - \frac{1}{{A^{2} }}(y - \beta )^{2} } \sqrt {1 + \frac{{B^{2} }}{{A^{2} }} \frac{{(y - \beta )^{2} }}{{A^{2} - (y - \beta )^{2} }}} } \right){\text{d}}y\cos \alpha$$

  (60)

  Eventually, the frictional force between cup wall and punch can be obtained by integrating Eq. (60) as follows:

  $$F_{f} = \mu P\mathop \smallint \limits_{t}^{y} 2\pi B\left( {\sqrt {1 - \frac{1}{{A^{2} }}(y - \beta )^{2} } \sqrt {1 + \frac{{B^{2} }}{{A^{2} }} \frac{{(y - \beta )^{2} }}{{A^{2} - (y - \beta )^{2} }}} } \right){\text{d}}y\cos \alpha$$

  (61)