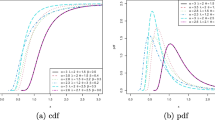
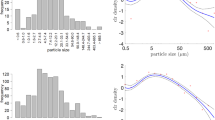
Abstract
The estimation of the distribution function of a population is an important problem in sampling finite populations. The existing literature focuses on the problem of estimating the population distribution function (p.f.d.) at a single point, or at a finite number of points. In this paper the main interest consists in estimating the whole p.d.f.. In many respects, the starting point is close to classical nonparametric statistics, although the approach to inference is based on sampling design. It is shown here that the Hájek estimator of the p.d.f., if properly centered and scaled, converges weakly to a Gaussian process with covariance kernel proportional to that of a Brownian bridge. The proportionality factor essentially depends on the sample design. Applications to (i) construction of a confidence band for the p.d.f., (i i) comparison of the p.d.f.s of two populations, and (i i i) testing for independence of two characters are provided.
Similar content being viewed by others
References
BERGER, Y.G. (1998a). Rate of convergence to asymptotic variance for the Horvitz-Thompson estimator. Journal of Statistical Planning and Inference 74, 149–168.
BERGER, Y.G. (1998b). Rate of convergence to normal distribution for the Horvitz-Thompson estimator. Journal of Statistical Planning and Inference 67, 209–226.
BERGER, Y.G. (2005). Variance estimation with Chao’s sampling scheme. Journal of Statistical Planning and Inference 127, 253–277.
BERGER, Y.G. (2011). Asymptotic consistency under large entropy sampling designs with unequal probabilities. Pakistan Journal of Statistics 27, 407–426.
BILLINGSLEY, P. (1968). Convergence of Probability Measures. Wiley, New York.
BREWER, K.R.W. and DONADIO, M.E. (2003). The high entropy variance of the Horvitz-Thompson estimator. Survey Methodology 29, 189–196.
CASSEL, C., SÄRNDAL, C., and WRETMAN J.H. (1977). Foundations of Inference in Survey Sampling. Wiley, New York.
CHEN, J. and WU, C. (2002). Estimation of distribution function and quantiles using the model-calibrated pseudo empirical likelihood method. Statistica Sinica 12, 1223–1239.
CIFARELLI, D.M., CONTI, P.L., and REGAZZINI, E. (1996). On the asymptotic distribution of a general measure of monotone dependence. The Annals of Statistics 24, 1386–1399.
CONTI, P.L. (1994). Asymptotic inference on a general measure of monotone dependence. Journal of the Italian Statistical Society 3, 213–241.
FRANCISCO, C.A. and FULLER W.A. (1991). Quantile estimation with a complex survey design. The Annals of Statistics 19, 454–469.
GENEST, C., NES̆LEHOVÁ, J., and GHORBAL, N.B. (2010). Spearman’s footrule and Gini’s gamma: a review with complements. Journal of Nonparametric Statistics 22, 937–954.
GRAFSTRÖM, A. (2010). Entropy of unequal probability sampling designs. Statistical Mathodology 7, 84–97.
HÁJEK, J. (1964). Asymptotic theory of rejective sampling with varying probabilities from a finite population. The Annals of Mathematical Statistics 35, 1491–1523.
HÁJEK J. (1981). Sampling from a finite population. Marcel Dekker, New York.
HÁJEK, J., S̆IDÁK, A., and SEN, P.K. (1999). Theory of Rank Tests, 2nd Ed. Academic Press, New York.
ISAKI, C.T. and FULLER, W.A. (1982). Survey design under the regression superpopulation model. Journal of the American Statistical Association 77, 89–96.
KRAFT, C. (1955). Some conditions for consistency and uniform consistency of statistical procedures. University of California Publications in Statistics 2, 125–142.
KUK, A.Y.C. (1988). Estimation of distribution functions and medians under sampling with unequal probabilities. Biometrika 75, 97–103.
PINSKER, M.S. (1964). Information and Information Stability for Random Variables and Processes. Holden Day, San Francisco.
PRÁS̆KOVÁ, Z. and SEN, P.K. (2009). Asymptotics in finite population sampling. In: Handbook of Statistics 29B - Sample Surveys: Inference and Analysis (Eds. D. Pfeffermann and C.R. Rao). Elsevier, Holland.
RAO, J.N.K., KOVAR, J.G., and MANTEL, H.J. (1990). On estimating distribution functions and quantiles from survey data using auxiliary information. Biometrika 77, 365–375.
ROSÉN, B. (1964). Limit theorems for sampling from finite populations. Arkiv För Matematik 5, 383–424.
RUEDA, M., MARTINEZ, S., MARTINEZ, H., and ARCOS A. (2007). Estimation of the distribution function with calibration methods. Journal of Statistical Planning and Inference 137, 435–448.
SÄRNDAL, C.-E., SWENSSON, B., and WRETMAN, J. (1992). Model Assisted Survey Sampling. Springer, New York.
SEN, P.K. (1972). Finite population sampling and weak convergence to a Brownian bridge. Sankhya A 5, 383–424.
SEN, P.K. (1995). The Hájek asymptotics for finite population sampling and their ramifications. Kybernetika 34, 251–268.
SERFLING, R.J. (1980). Approximation Theorems of Mathematical Statistics. Wiley, New York.
SHAO, J. (1994). L-statistics in complex survey problems. The Annals of Statistics 22, 946–967.
TILLÉ, Y. (2006). Sampling Algorithms. Springer, New York.
VÍŠEK, J.A. (1979). Asymptotic distribution of simple estimate for rejective, Sampford and successive sampling. In: Contributions to Statistics (Ed. J. Jurec̆ková). Holland, Reidel Publishing Company, p. 263–275.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Conti, P.L. On the Estimation of the Distribution Function of a Finite Population Under High Entropy Sampling Designs, with Applications. Sankhya B 76, 234–259 (2014). https://doi.org/10.1007/s13571-014-0083-x
Received:
Revised:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s13571-014-0083-x