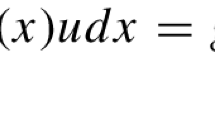Skip to main content
SpringerLink
Log in

Existence and uniqueness result for a backward stochastic differential equation whose generator is Lipschitz continuous in y and uniformly continuous in z

  • Published:
Journal of Applied Mathematics and Computing Aims and scope Submit manuscript

Abstract

In this note, we extend the result in Lepetier and San Martin (Stat. Probab. Lett. 32:425–430, 1997) by eliminating the condition that (g(t,0,0))t∈[0,T] is a bounded process. Furthermore, we prove that if g is Lipschitz continuous in y and uniformly continuous in z, and (g(t,0,0))t∈[0,T] is square integrable, then for each square integrable terminal condition ξ, there exists a unique square integrable adapted solution to the one-dimensional backward stochastic differential equation (BSDE) with the generator g, which generalizes the corresponding (one-dimensional) results in Pardoux and Peng (Syst. Control Lett. 14:55–61, 1990), Jia (C. R. Acad. Sci. Paris, Ser. I 346:439–444, 2008) and Jia (Stat. Probab. Lett. 79:436–441, 2009).

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Bahlali, K.: Backward stochastic differential equations with locally Lipschitz coefficient. C. R. Acad. Sci. Paris, Ser. I 333, 481–486 (2001)

    MathSciNet  MATH  Google Scholar 

  2. Bahlali, K., Hamadène, S., Mezerdi, B.: Backward stochastic differential equations with two reflecting barriers and continuous with quadratic growth coefficient. Stoch. Process. Appl. 115, 1107–1129 (2005)

    Article  MATH  Google Scholar 

  3. Briand, Ph., Delyon, B., Hu, Y., Pardoux, E., Stoica, L.: L p solutions of backward stochastic differential equations. Stoch. Process. Appl. 108, 109–129 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  4. Briand, Ph., Hu, Y.: Quadratic BSDEs with convex generators and unbounded terminal conditions. Probab. Theory Relat. Fields 141, 543–567 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  5. Crandall, M.G.: Viscosity solutions—a primer. In: Capuzzo Dolcetta, I., Lions, P.L. (eds.) Viscosity Solutions and Applications. Lecture Notes in Mathematics, pp. 1–43. Springer, Berlin (1997)

    Chapter  Google Scholar 

  6. El Karoui, N., Peng, S.G., Quenez, M.C.: Backward stochastic differential equations in finance. Math. Finance 7, 1–72 (1997)

    Article  MathSciNet  MATH  Google Scholar 

  7. Hamadène, S.: Multidimensional backward stochastic differential equations with uniformly continuous coefficients. Bernoulli 9(3), 517–534 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  8. Hewitt, E., Stromberg, K.R.: Real and Abstract Analysis. Springer, New York (1978)

    Google Scholar 

  9. Jia, G.Y.: A uniqueness theorem for the solution of backward stochastic differential equations. C. R. Acad. Sci. Paris, Ser. I 346, 439–444 (2008)

    MATH  Google Scholar 

  10. Jia, G.Y.: Some uniqueness results for one-dimensional BSDEs with uniformly continuous coefficients. Stat. Probab. Lett. 79, 436–441 (2009)

    Article  MATH  Google Scholar 

  11. Karatzas, I., Shreve, S.E.: Brownian Motion and Stochastic Calculus. Springer, New York (1991)

    MATH  Google Scholar 

  12. Kobylanski, M.: Backward stochastic differential equations and partial equations with quadratic growth. Ann. Probab. 28, 259–276 (2000)

    Article  MathSciNet  Google Scholar 

  13. Lepetier, J.P., San Martin, J.: Backward stochastic differential equations with continuous coefficient. Stat. Probab. Lett. 32, 425–430 (1997)

    Article  Google Scholar 

  14. Lepetier, J.P., San Martin, J.: Existence for BSDE with superlinear quadratic coefficient. Stoch. Stoch. Rep. 63, 227–240 (1998)

    Google Scholar 

  15. Mao, X.R.: Adapted solutions of backward stochastic differential equations with non-Lipschitz coefficients. Stoch. Process. Appl. 58, 281–292 (1995)

    Article  MATH  Google Scholar 

  16. Pardoux, E., Peng, S.G.: Adapted solution of a backward stochastic differential equation. Syst. Control Lett. 14, 55–61 (1990)

    Article  MathSciNet  MATH  Google Scholar 

  17. Pardoux, E., Peng, S.G.: Some backward SDE’s with non-Lipschtiz coefficients. In: Proc. Conf. Metz (1992)

  18. Pardoux, E.: Backward stochastic differential equations and viscosity solutions of system of semi-linear parabolic and elliptic PDEs of second order. In: Stochastic Analysis and Related Topics, pp. 79–128. Birkhäuser, Basel (1996)

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. College of Sciences, China University of Mining and Technology, Xuzhou, Jiangsu, 221116, P.R. China

    Sheng Jun Fan & Long Jiang

Authors
  1. Sheng Jun Fan
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Long Jiang
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Sheng Jun Fan.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Fan, S.J., Jiang, L. Existence and uniqueness result for a backward stochastic differential equation whose generator is Lipschitz continuous in y and uniformly continuous in z . J. Appl. Math. Comput. 36, 1–10 (2011). https://doi.org/10.1007/s12190-010-0384-9

Download citation

  • Received:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s12190-010-0384-9

Keywords

Mathematics Subject Classification (2000)

Search

Navigation