Abstract
This paper extends hyperkähler Floer theory with flat target manifolds from the case where the source is a 3-sphere or 3-torus, equipped with a standard frame, to the case where the source is a general closed orientable 3-manifold, equipped with a regular divergence-free frame. Regular divergence-free frames are characterized by the nonexistence of nonconstant solutions to the unperturbed linear Fueter equation. They form a dense open subset of the space of all divergence-free frames. A gauged version of the Fueter equation is introduced, which unifies various geometric equations in gauge theory.
Similar content being viewed by others
References
Atiyah, M.F., Patodi, V.K., Singer, I.M.: Spectral asymmetry and Riemannian geometry III. Math. Proc. Camb. Philos. Soc. 79, 71–99 (1976)
Cieliebak, K., Gaio, A.R., Salamon, D.A.: J-holomorphic curves, moment maps, and invariants of Hamiltonian group actions. Int. Math. Res. Not. 10, 831–882 (2000)
Cieliebak, K., Gaio, A.R., Mundet i Riera, I., Salamon, D.A.: The symplectic vortex equations and invariants of Hamiltonian group actions. J. Symplectic Geom. 1, 543–645 (2002)
Conley, C., Zehnder, E.: The Birkhoff-Lewis fixed point theorem and a conjecture of V.I. Arnold. Invent. Math. 73, 33–49 (1983)
Donaldson, S.K., Kronheimer, P.B.: The Geometry of Four-Manifolds. Oxford University Press, Oxford (1990)
Donaldson, S.K., Thomas, R.P.: Gauge theory in higher dimensions. In: The Geometric Universe, Oxford, 1996, pp. 31–47. Oxford University Press, Oxford (1998)
Donaldson, S.K., Segal, E.: Gauge theory in higher dimensions II. Preprint (2009)
Dostoglou, S., Salamon, D.A.: Self-dual instantons and holomorphic curves. Ann. Math. 139, 581–640 (1994)
Ebert, J.: A vanishing theorem for Characteristic classes of odd-dimensional manifold bundles. Preprint (2010) arXiv:0902.4719v3
Eliashberg, Y., Mishachev, N.: Introduction to the h-Principle. AMS, Providance (2002)
Floer, A.: Morse theory for Lagrangian intersections. J. Differ. Geom. 28, 513–547 (1988)
Floer, A.: The unregularized gradient flow of the symplectic action. Commun. Pure Appl. Math. 41, 775–813 (1988)
Floer, A.: Symplectic fixed points and holomorphic spheres. Commun. Math. Phys. 120, 575–611 (1989)
Floer, A.: An instanton invariant of 3-manifolds. Commun. Math. Phys. 118, 215–240 (1989)
Frauenfelder, U.: The Arnold-Givental conjecture and moment Floer homology. Int Math Res Not 2179–2269 (2004)
Fueter, R.: Analytische Funktionen einer Quaternionenvariablen. Comment. Math. Helv. 4, 9–20 (1932)
Fueter, R.: Die Theorie der regulären Funktionen einer Quaternionenvariablen. C. R. 1, 75–91 (1937). ICM Oslo (1936)
Gaio, A.R., Salamon, D.A.: Gromov–Witten invariants of symplectic quotients and adiabatic limits. J. Symplectic Geom. 3, 55–159 (2005)
Ginzburg, V., Hein, D.: Hyperkähler Arnold conjecture and its generalizations. Int. Math. Res. Not. 53–68 (2010)
Ginzburg, V., Hein, D.: Arnold conjecture for Clifford symplectic pencils. To appear in Isr. J. Math. (2013)
Gromov, M.: Partial Differential Relations. Springer, Berlin (1986)
Haydys, A.: Nonlinear Dirac operator and quaternionic analysis. Commun. Math. Phys. 281, 251–261 (2008)
Hohloch, S., Noetzel, G., Salamon, D.A.: Hypercontact structures and Floer homology. Geom. Topol. 13, 2543–2617 (2009)
Hohloch, S., Noetzel, G., Salamon, D.A.: Floer homology groups in hyperkähler geometry. In: Abreu, M., Lalonde, F., Polterovich, L. (eds.) New Perspectives and Challenges in Symplectic Field Theory. CRM, vol. 49, pp. 251–261. AMS, Providance (2009)
Joyce, D.: Compact Manifolds with Special Holonomy. Oxford University Press, Oxford (2000)
Kronheimer, P., Mrowka, T.: Monopoles and Three Manifolds. CUP, Cambridge (2007)
McDuff, D., Salamon, D.A.: J-Holomorphic Curves and Symplectic Topology, 2nd edn. AMS Colloquium Publications, vol. 52. AMS, Providance (2012)
Mundet i Riera, I.: Hamiltonian Gromov–Witten invariants. Topology 42, 525–553 (2003)
Ott, A.: Removal of singularities and Gromov compactness for symplectic vortices. J. Symplectic Geom.
Ott, A., Ziltener, F.: Gauged Gromov-Witten invariants for monotone symplectic manifolds
Pidstrigatch, V.Y.: Hyperkähler manifolds and the Seiberg–Witten equations. Proc. Steklov Inst. Math. 246, 249–262 (2004)
Pidstrigatch, V.Y., Tyurin, A.N.: Localization of Donaldson invariants along the Seiberg–Witten basic classes. Preprint (1995). arXiv:dg-ga/9507004
Pozniak, M.: Floer homology, Novikov rings and clean intersections. Eliashberg, Y., Fuchs, D., Ratiu, T., Weinstein, A. (eds.) North. Calif. Symp. Geo. Seminar. UCB. AMS, Providance (1999)
Robbin, J.W., Salamon, D.A.: The spectral flow and the Maslov index. Bull. Lond. Math. Soc. 27, 1–33 (1995)
Salamon, D.A., Walpuski, T.: Notes on the octonians. Preprint (2010)
Taubes, C.H.: Nonlinear generalizations of a 3-manifold’s Dirac operator. In: Trends in Mathematical Physics, Knoxville, TN, 1998. AMS/IP Stud. Adv. Math., vol. 13, pp. 475–486 (1999)
Walpuski, T.: Private communication. February (2011)
Walpuski, T.: G2-instantons on generalized Kummer constructions. Preprint (2011). arXiv:1109.6609
Walpuski, T.: G2-instantons, associative submanifolds and Fueter sections. Preprint (2012). arXiv:1205.5350
Acknowledgements
These are expanded notes for a lecture given at Imperial College on 10 February 2012. Thanks to Simon Donaldson and Thomas Walpuski for many helpful discussions and for their hospitality during my visit. Thanks to Yasha Eliashberg for his explanation of the h-principle, and thanks to the referee and editor for helpful comments on the exposition.
Author information
Authors and Affiliations
Corresponding author
Additional information
Communicated by J. Latschev.
Partially supported by the Swiss National Science Foundation Grant 200021-127136.
Appendices
Appendix A: Divergence-free frames
Let M be a closed connected oriented 3-manifold and \(\operatorname {dvol}_{M}\in {\varOmega}^{3}(M)\) be a positive volume form. Denote the set of positive frames by

and the set of divergence-free positive frames by

Given three deRham cohomology classes a 1,a 2,a 3∈H 2(M;ℝ), denote the set of divergence-free positive frames that represent the classes a i by

The following theorem is an application of Gromov’s h-principle and is proved in [21, page 182] and [10, Corollary 20.4.3]. Although the result is stated in these references in the weaker form that every frame can be deformed in  to a divergence-free frame, the proofs give the stronger result stated below (as explained to the author by Yasha Eliashberg).
to a divergence-free frame, the proofs give the stronger result stated below (as explained to the author by Yasha Eliashberg).
Theorem A.1
(Gromov)
The inclusion
 is a homotopy equivalence for all
a
1,a
2,a
3∈H
2(M;ℝ), and so is the inclusion
is a homotopy equivalence for all
a
1,a
2,a
3∈H
2(M;ℝ), and so is the inclusion
 .
.
Lemma A.2
(Gromov)
Let V be a 3-dimensional real vector space and S:V×V→V be a skew-symmetric bilinear map. Let \(\mathcal{R}\subset\operatorname{Hom}(V,\operatorname{End}(V))\) be the set of all linear maps \(L:V\to\operatorname{End}(V)\) such that the map
is a vector space isomorphism. Fix a 2-dimensional linear subspace E⊂V and a linear map \(\lambda:E\to\operatorname{End}(V)\). Define
If \(\mathcal{L}\cap\mathcal{R}\) is nonempty, then it has two connected components and the convex hull of each connected component of \(\mathcal{L}\cap\mathcal{R}\) is equal to \(\mathcal{L}\).
Proof
The proof is a special case of the argument given by Eliashberg–Mishachev in [10, pages 183/184]. Assume without loss of generality that
Write S in the form
and write a linear map \(L:{\mathbb{R}}^{3}\to\operatorname {End}({\mathbb{R}}^{3})\) in the form
Then \(L\in\mathcal{R}\) if and only if
Denote by \(\mathcal{R}^{+}\), respectively \(\mathcal{R}^{-}\), the set of all \(L\in\mathcal{R}\) for which the sign of the determinant in (52) is positive, respectively negative.
Fix a linear map \(\lambda:E\to\operatorname{End}({\mathbb{R}}^{3})\). This map is determined by the coefficients L ij with i=2,3. Thus an element \(L\in\mathcal{L}\cap\mathcal{R}\) is determined by the choice of L 11, L 12, L 13. If S 23+L 23−L 32=0 then the determinant in (52) vanishes for every \(L\in\mathcal{L}\) and so \(\mathcal{L}\cap\mathcal{R}=\emptyset\). Hence assume S 23+L 23−L 32≠0. Then \(\mathcal{L}\cap\mathcal{R}^{+}\) and \(\mathcal{L}\cap\mathcal {R}^{-}\) are nonempty connected submanifolds of ℝ3×ℝ3×ℝ3. (Namely, L 11 is any vector in ℝ3, L 12 is required to be in the complement of an affine line, and then L 13 is required to be in the complement of an affine plane depending smoothly on L 12.)
Choose x,y∈ℝ3 such that
Then, for t>0 sufficiently large,

Given \(L\in\mathcal{L}\) choose \(L',L''\in\mathcal{L}\) such that

Then \(L',L''\in\mathcal{L}\cap\mathcal{R}^{+}\) and \(L=\frac{1}{2}(L'+L'')\). Hence the convex hull of \(\mathcal{L}\cap\mathcal{R}^{+}\) is equal to \(\mathcal{L}\). A similar argument shows that the convex hull of \(\mathcal{L}\cap \mathcal{R}^{-}\) is also equal to \(\mathcal{L}\). This proves Lemma A.2. □
Proof of Theorem A.1
Fix a Riemannian metric on M, let a∈H 2(M;ℝ)3, and let σ i ∈Ω 2(M) be the harmonic representative of a i , i=1,2,3. Define

Let

be the projection defined by π
a
(β)=v for  , where \(\iota(v_{i})\operatorname{dvol}_{M}:=\sigma_{i}+d\beta_{i}\). This is a homotopy equivalence. A homotopy inverse assigns to
, where \(\iota(v_{i})\operatorname{dvol}_{M}:=\sigma_{i}+d\beta_{i}\). This is a homotopy equivalence. A homotopy inverse assigns to  the unique co-exact triple of 1-forms \(\beta\in\pi_{a}^{-1}(v)\).
the unique co-exact triple of 1-forms \(\beta\in\pi_{a}^{-1}(v)\).
Consider the vector bundle
over M and denote by X (1) the 1-jet bundle. Use the Riemannian metric on M to identify X (1) with the set of tuples (y,β 1,β 1,β 3,L 1,L 2,L 3) with y∈M, \(\beta_{i}\in T_{y}^{*}M\), and \(L_{i}\in\operatorname {Hom}(T_{y}M,T_{y}^{*}M)\). Denote by

the open subset of all (y,β,L)∈X (1) such that the 2-forms \(\tau_{i}\in\varLambda^{2}T_{y}^{*}M\), defined by
are linearly independent. Denote by  the space of sections of
the space of sections of  . Thus an element of
. Thus an element of  is a tuple (β,L)=(β
1,β
2,β
3,L
1,L
2,L
3) with β
i
∈Ω
1(M) and L
i
∈Ω
1(M,T
∗
M) such that the 2-forms τ
i
∈Ω
2(M), defined by (53) are everywhere linearly independent. Then
is a tuple (β,L)=(β
1,β
2,β
3,L
1,L
2,L
3) with β
i
∈Ω
1(M) and L
i
∈Ω
1(M,T
∗
M) such that the 2-forms τ
i
∈Ω
2(M), defined by (53) are everywhere linearly independent. Then  is a bundle over
is a bundle over  . The projection
. The projection  is given by π
a
(β,L)=v, where \(\iota(v_{i})\operatorname{dvol}_{M}:=\tau_{i}\) and τ
i
is as in (53). This map is a homotopy equivalence. A homotopy inverse of π
a
is the inclusion
is given by π
a
(β,L)=v, where \(\iota(v_{i})\operatorname{dvol}_{M}:=\tau_{i}\) and τ
i
is as in (53). This map is a homotopy equivalence. A homotopy inverse of π
a
is the inclusion  given by ι
a
(v):=(0,L), where \(L_{i}(u):=\frac{1}{2} (\operatorname{dvol}_{M}(v_{i},u,\cdot )-\sigma_{i}(u,\cdot ) )\). Namely,
given by ι
a
(v):=(0,L), where \(L_{i}(u):=\frac{1}{2} (\operatorname{dvol}_{M}(v_{i},u,\cdot )-\sigma_{i}(u,\cdot ) )\). Namely,  , both maps are linear between open subsets of topological vector spaces, and the kernel of π
a
is the space of tuples (β,L) such that each L
i
is symmetric.
, both maps are linear between open subsets of topological vector spaces, and the kernel of π
a
is the space of tuples (β,L) such that each L
i
is symmetric.
The previous discussion shows that there is a commutative diagram

where the vertical maps are homotopy equivalences and the differential operator  is given by
is given by  . Thus
. Thus  is the space of all sections β of X such that
is the space of all sections β of X such that  satisfies the differential relation
satisfies the differential relation  . By Lemma A.2,
. By Lemma A.2,  is ample in the sense of [10, page 167]. Hence
is ample in the sense of [10, page 167]. Hence  satisfies the h-principle (see [10, Theorem 18.4.1]). In particular, every section of
satisfies the h-principle (see [10, Theorem 18.4.1]). In particular, every section of  is homotopic, through sections of
is homotopic, through sections of  , to a section of the form (β,∇β). Equivalently, every frame
, to a section of the form (β,∇β). Equivalently, every frame  can be deformed within
can be deformed within  to a divergence-free frame in
to a divergence-free frame in  . In fact, by the parametric h-principle, the inclusion
. In fact, by the parametric h-principle, the inclusion  induces isomorphisms on all homotopy groups, and is therefore a homotopy equivalence (see [10, 6.2.A]). Hence the inclusion
induces isomorphisms on all homotopy groups, and is therefore a homotopy equivalence (see [10, 6.2.A]). Hence the inclusion  is a homotopy equivalence.
is a homotopy equivalence.
To explain the extension of this result to the inclusion of  into
into  , it is convenient to spell out the details of the parametric h-principle in the present setting. Choose a smooth manifold Λ and a smooth map a:Λ→H
2(M;ℝ)3. Consider the vector bundle
, it is convenient to spell out the details of the parametric h-principle in the present setting. Choose a smooth manifold Λ and a smooth map a:Λ→H
2(M;ℝ)3. Consider the vector bundle
Define  as the set of tuples \((\lambda,y,\beta_{1},\beta_{2},\beta_{3},{\widetilde{L}}_{1},{\widetilde {L}}_{2},{\widetilde{L}}_{3})\), with λ∈Λ, y∈M, \(\beta_{i}\in T^{*}_{y}M\), and \({\widetilde{L}}_{i}\in\operatorname{Hom}(T_{\lambda}\varLambda\times T_{y}M,T_{y}^{*}M)\), such that the 2-forms \(\tau_{i}=\tau_{\lambda,i}\in\varLambda^{2}T_{y}^{*}M\) in (53) are linearly independent. Here σ
i
=σ
λ,i
is the harmonic representative of the class a
i
(λ) and \(L_{i}\in\operatorname{Hom}(T_{y}M,T_{y}^{*}M)\) is the restriction of \({\widetilde{L}}_{i}\) to 0×T
y
M. Define the operator
as the set of tuples \((\lambda,y,\beta_{1},\beta_{2},\beta_{3},{\widetilde{L}}_{1},{\widetilde {L}}_{2},{\widetilde{L}}_{3})\), with λ∈Λ, y∈M, \(\beta_{i}\in T^{*}_{y}M\), and \({\widetilde{L}}_{i}\in\operatorname{Hom}(T_{\lambda}\varLambda\times T_{y}M,T_{y}^{*}M)\), such that the 2-forms \(\tau_{i}=\tau_{\lambda,i}\in\varLambda^{2}T_{y}^{*}M\) in (53) are linearly independent. Here σ
i
=σ
λ,i
is the harmonic representative of the class a
i
(λ) and \(L_{i}\in\operatorname{Hom}(T_{y}M,T_{y}^{*}M)\) is the restriction of \({\widetilde{L}}_{i}\) to 0×T
y
M. Define the operator  from sections of \({\widetilde{X}}\) to sections of \({\widetilde{X}}^{(1)}\) as the covariant derivative
from sections of \({\widetilde{X}}\) to sections of \({\widetilde{X}}^{(1)}\) as the covariant derivative

Let  be the space of sections of
be the space of sections of  and denote by
and denote by  its preimage under
its preimage under  . Thus an element of
. Thus an element of  is a map Λ→Ω
1(M)3:λ↦β
λ
such that the 2-forms
is a map Λ→Ω
1(M)3:λ↦β
λ
such that the 2-forms
are everywhere linearly independent for every λ. An element of  is a smooth section that assigns to λ∈Λ a tuple
is a smooth section that assigns to λ∈Λ a tuple
such that the 2-forms τ λ,i ∈Ω 2(M), defined by (53), are everywhere linearly independent for every λ∈Λ. As before there is a commutative diagram

Here  is the space of maps
is the space of maps  such that
such that  and
and  is the space of all smooth maps from Λ to
is the space of all smooth maps from Λ to  . The projection
. The projection  , respectively
, respectively  , assigns to the section λ↦β
λ
, respectively (55), the section λ↦v
λ
with \(\iota(v_{\lambda,i})\operatorname {dvol}_{M}=\tau _{\lambda,i}\), where τ
λ,i
is given by (54), respectively (53). Both projections are homotopy equivalences.
, assigns to the section λ↦β
λ
, respectively (55), the section λ↦v
λ
with \(\iota(v_{\lambda,i})\operatorname {dvol}_{M}=\tau _{\lambda,i}\), where τ
λ,i
is given by (54), respectively (53). Both projections are homotopy equivalences.
By Lemma A.2, the open differential relation  is ample. Hence it follows from the h-principle in [10, Theorem 18.4.1] that every smooth map from Λ to
is ample. Hence it follows from the h-principle in [10, Theorem 18.4.1] that every smooth map from Λ to  can be deformed within
can be deformed within  to a smooth map
to a smooth map  that satisfies
that satisfies  . With Λ=S
k this implies that the homomorphism
. With Λ=S
k this implies that the homomorphism  is surjective for all a
1,a
2,a
3∈H
2(M;ℝ).
is surjective for all a
1,a
2,a
3∈H
2(M;ℝ).
The relation  also satisfies the relative h-principle in [10, 6.2.C]. For the (k+1)-ball Λ=B
k+1 with boundary ∂B
k+1=S
k this means that, if a map
also satisfies the relative h-principle in [10, 6.2.C]. For the (k+1)-ball Λ=B
k+1 with boundary ∂B
k+1=S
k this means that, if a map  extends over B
k+1 in
extends over B
k+1 in  , and one chooses any smooth extension of the projection
, and one chooses any smooth extension of the projection  over B
k+1, then this extension lifts to a smooth map
over B
k+1, then this extension lifts to a smooth map  , equal to the given map over the boundary (and homotopic to the given map in
, equal to the given map over the boundary (and homotopic to the given map in  ). Hence the homomorphism
). Hence the homomorphism  is injective. This proves Theorem A.1. □
is injective. This proves Theorem A.1. □
Appendix B: Self-adjoint Fredholm operators
This appendix is included for the benefit of the reader. It discusses two well known results about self-adjoint Fredholm operators, that are used in Sect. 2. Lemma B.1 characterizes unbounded self-adjoint Fredholm operators and Lemma B.2 shows that regular crossings are isolated. While Lemma B.2 follows from the Kato selection theorem (see [34, Lemma 4.7]), the proof given below is simpler and more direct.
Let H be a Hilbert space and V⊂H be a dense linear subspace that is a Hilbert space in its own right. Suppose that the inclusion V↪H is a compact operator. Denote the inner product on H by 〈⋅,⋅〉, the norm on H by \(\Vert x\Vert _{H}:=\sqrt{\langle x,x\rangle}\) for x∈H, and the norm on V by ∥x∥
V
for x∈V. Let  be the space of symmetric bounded linear operators A:V→H and
be the space of symmetric bounded linear operators A:V→H and  be the subset of self-adjoint operators. Thus a bounded linear operator D:V→H is an element of
be the subset of self-adjoint operators. Thus a bounded linear operator D:V→H is an element of  if and only if 〈Dx,ξ〉=〈x,Dξ〉 for all x,ξ∈V and, for every x∈H, the following holds
if and only if 〈Dx,ξ〉=〈x,Dξ〉 for all x,ξ∈V and, for every x∈H, the following holds
Every  is a Fredholm operator of index zero and regular crossings of differentiable paths
is a Fredholm operator of index zero and regular crossings of differentiable paths  are isolated. Proofs of these well known observations are included here for completeness of the exposition.
are isolated. Proofs of these well known observations are included here for completeness of the exposition.
Lemma B.1
Let
 . Then the following are equivalent.
. Then the following are equivalent.
-
(i)
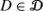 .
. -
(ii)
\((\operatorname{im}\,D)^{\perp}\subset V\) and there is a constant c>0 such that, for all x∈V,
$$ \Vert x\Vert _V\le c \bigl(\Vert Dx\Vert _H+\Vert x\Vert _H \bigr). $$(57) -
(iii)
D is a Fredholm operator of index zero.
In particular,  is an open subset of
is an open subset of
 in the norm topology.
in the norm topology.
Proof
We prove that (i) implies (ii). Assume  . By (56) \((\operatorname{im}\,D)^{\perp}\subset V\). We show that the graph of D is a closed subspace of H×H. Let x
n
∈V and x,y∈H be such that lim
n→∞∥x−x
n
∥
H
=0 and lim
n→∞∥y−Dx
n
∥
H
=0. Then 〈x,Dξ〉=lim
n→∞〈x
n
,Dξ〉=lim
n→∞〈Dx
n
,ξ〉=〈y,ξ〉 for ξ∈V. Hence x∈V by (56) and, since D is symmetric, it follows that Dx=y. Thus D has a closed graph. Now V→graph(D):x↦(x,Ax) is a bijective bounded linear operator and so has a bounded inverse. This proves (57).
. By (56) \((\operatorname{im}\,D)^{\perp}\subset V\). We show that the graph of D is a closed subspace of H×H. Let x
n
∈V and x,y∈H be such that lim
n→∞∥x−x
n
∥
H
=0 and lim
n→∞∥y−Dx
n
∥
H
=0. Then 〈x,Dξ〉=lim
n→∞〈x
n
,Dξ〉=lim
n→∞〈Dx
n
,ξ〉=〈y,ξ〉 for ξ∈V. Hence x∈V by (56) and, since D is symmetric, it follows that Dx=y. Thus D has a closed graph. Now V→graph(D):x↦(x,Ax) is a bijective bounded linear operator and so has a bounded inverse. This proves (57).
We prove that (ii) implies (iii). Since V↪H is a compact operator, it follows from (57) that D has a finite-dimensional kernel and a closed image (see [27, Lemma A.1.1]). Since \((\operatorname{im}\,D)^{\perp}\subset V\) and D is symmetric, it follows that \((\operatorname{im}\,D)^{\perp}=\ker\,D\). Hence \(\dim\,\operatorname{coker}\,D=\dim\,\ker\,D\).
We prove that (iii) implies (i). Let  be a Fredholm operator of index zero. Then D has a finite-dimensional kernel and a closed image. Since D is symmetric, \(\ker\,D\subset(\operatorname{im}\,D)^{\perp}\). Since D has Fredholm index zero, \(\ker\,D = (\operatorname{im}\, D)^{\perp}\) and hence \(\operatorname{im}\,D=(\ker\,D)^{\perp}\). Now let x∈H and suppose that there is a constant c such that |〈x,Dξ〉|≤c∥ξ∥
H
for every ξ∈V. By the Riesz representation theorem, there exists an element y∈H such that 〈x,Dξ〉=〈y,ξ〉 for ξ∈V. Choose y
0∈ker D such that y−y
0⊥ker D. Then \(y-y_{0}\in\operatorname{im}\,D\). Choose x
1∈V such that Dx
1=y−y
0. Then 〈x−x
1,Dξ〉=〈y,ξ〉−〈Dx
1,ξ〉=〈y
0,ξ〉 for every ξ∈V. Given ξ∈V choose ξ
0∈ker D such that ξ−ξ
0⊥ kerD. Then
be a Fredholm operator of index zero. Then D has a finite-dimensional kernel and a closed image. Since D is symmetric, \(\ker\,D\subset(\operatorname{im}\,D)^{\perp}\). Since D has Fredholm index zero, \(\ker\,D = (\operatorname{im}\, D)^{\perp}\) and hence \(\operatorname{im}\,D=(\ker\,D)^{\perp}\). Now let x∈H and suppose that there is a constant c such that |〈x,Dξ〉|≤c∥ξ∥
H
for every ξ∈V. By the Riesz representation theorem, there exists an element y∈H such that 〈x,Dξ〉=〈y,ξ〉 for ξ∈V. Choose y
0∈ker D such that y−y
0⊥ker D. Then \(y-y_{0}\in\operatorname{im}\,D\). Choose x
1∈V such that Dx
1=y−y
0. Then 〈x−x
1,Dξ〉=〈y,ξ〉−〈Dx
1,ξ〉=〈y
0,ξ〉 for every ξ∈V. Given ξ∈V choose ξ
0∈ker D such that ξ−ξ
0⊥ kerD. Then
Hence \(x-x_{1}\in(\operatorname{im}\,D)^{\perp}=\ker\,D\subset V\) and hence x∈V.
Since (i) and (iii) are equivalent it follows from the perturbation theory for Fredholm operators (see [27, Theorem A.1.5]) that  is an open subset of
is an open subset of  with respect to the norm topology. This proves Lemma B.1. □
with respect to the norm topology. This proves Lemma B.1. □
Let I⊂ℝ be an open interval and  be a continuous path with respect to the norm topology on
be a continuous path with respect to the norm topology on  . The path is called weakly differentiable if the map I→ℝ:s↦〈x,D(s)ξ〉 is differentiable for every x∈H and every ξ∈V. A crossing is an element s∈I such that D(s) has a nontrivial kernel. A crossing s∈I is called regular if the quadratic form
. The path is called weakly differentiable if the map I→ℝ:s↦〈x,D(s)ξ〉 is differentiable for every x∈H and every ξ∈V. A crossing is an element s∈I such that D(s) has a nontrivial kernel. A crossing s∈I is called regular if the quadratic form
is nondegenerate.
Lemma B.2
Let
 be a weakly differentiable path of self-adjoint operators and let
s
0∈I
be a regular crossing. Then there is a
δ>0 such that
D(s):V→H
is bijective for every
s∈I
with 0<|s−s
0|<δ.
be a weakly differentiable path of self-adjoint operators and let
s
0∈I
be a regular crossing. Then there is a
δ>0 such that
D(s):V→H
is bijective for every
s∈I
with 0<|s−s
0|<δ.
Proof
Assume without loss of generality that s 0=0. By Lemma B.1 there is a constant c>0 such that
for every x∈V and every s in some neighborhood of zero. Shrinking I, if necessary, we may assume that (58) holds for every s∈I.
Assume, by contradiction, that there is a sequence s n ∈I such that s n →0 and D(s n ) is not injective for every n. Then there is a sequence x n ∈V such that D(s n )x n =0 and ∥x n ∥ H =1. Thus ∥x n ∥ V ≤c by (58). Passing to a subsequence we may assume that x n converges in H to x 0. Then ∥x 0∥ H =1 and 〈x 0,D(0)ξ〉=lim n→∞〈x n ,D(s n )ξ〉=0 for ξ∈V. Hence x 0∈ker D(0). Moreover, for every ξ∈ker D(0), the sequence D(s n )ξ/s n converges weakly to \(\dot{D}(0)\xi\) and is therefore bounded, so
This contradicts the nondegeneracy of Γ 0 and proves Lemma B.2. □
Let I be a compact interval and  be a weakly differentiable path with only regular crossings such that D(s) is bijective at the endpoints of I. The spectral flow is the sum of the signatures of the crossing forms Γ
s
over all crossings. It is invariant under homotopy with fixed endpoints and is additive under catenation. (See [34] for an exposition.)
be a weakly differentiable path with only regular crossings such that D(s) is bijective at the endpoints of I. The spectral flow is the sum of the signatures of the crossing forms Γ
s
over all crossings. It is invariant under homotopy with fixed endpoints and is additive under catenation. (See [34] for an exposition.)
Rights and permissions
About this article
Cite this article
Salamon, D. The three-dimensional Fueter equation and divergence-free frames. Abh. Math. Semin. Univ. Hambg. 83, 1–28 (2013). https://doi.org/10.1007/s12188-013-0075-1
Received:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s12188-013-0075-1




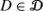 .
.