1 Introduction

Algebra serves as a “gatekeeper” in school mathematics, with consequences for students’ advancement in mathematics, access to higher education, and future earnings (Adelman, 2006; Chen, 2013; National Mathematics Advisory Panel, 2008). High failure rates in algebra have kept many students from educational, career, and economic opportunities (Kaput, 1998; Moses & Cobb, 2001; Stigler et al., 1999). This has led to calls to introduce students to algebraic concepts earlier in their mathematics careers (e.g., Carpenter & Levi, 2000; Kaput, 1998; National Council of Teachers of Mathematics [NCTM], 2000; National Governors Association Center for Best Practices & Council of Chief State School Officers [NGA & CCSSO], 2010). These are not calls to shift the formalism of traditional algebra courses to earlier grades, but rather to “algebrafy” (Kaput, 1998) early mathematics by building informal thinking about structure and algebraic relationships into formal ways of reasoning at an earlier developmental point (Kaput et al., 2008; Kieran, 2004). Structural thinking can be built by encouraging students to use number and operation sense to reflect on mathematical expressions as objects rather than as arithmetic procedures to be carried out (Carpenter et al., 2003; Sfard, 1991). For example, students who understand problem structure can recognize expressions such as 8(x + 3) and 8x + 24 as equivalent without performing individual computations.

The concept of mathematical equivalence—specifically, the use of the equal sign to symbolically represent an equivalence relation—is widely accepted as foundational to algebraic thinking (Baroody & Ginsburg, 1983; Carpenter et al., 2003; NCTM, 2000; NGA & CCSSO, 2010; Stephens et al., 2021). Deep understanding of the equal sign is connected to early algebraic competence in the elementary grades (e.g., Byrd et al., 2015; Carpenter et al., 2003; Hornburg et al., 2022; Matthews & Fuchs, 2020) and to equation-solving success in the middle grades (Fyfe et al., 2018; Knuth et al., 2006). Further, when students are provided with problem-solving experiences that highlight the equal sign's meaning, they grow in their understanding (e.g., Blanton et al., 2019; Stephens et al., 2021).

2 Students’ conceptions of mathematical equivalence

A relational conception of the equal sign is the understanding that the symbol denotes the “sameness” of two quantities or expressions (e.g., Baroody & Ginsburg, 1983; Behr et al., 1980; Carpenter et al., 2003; Kieran, 1981; Knuth et al., 2005; McNeil et al., 2011; Molina & Ambrose, 2008). Students who hold a relational view of the equal sign interpret and flexibly work both (a) with equations in standard forms, in which operations appear to the left of the equal sign and the “result” to the right (e.g., 5 + 2 = 7, 6 + 3 = 9), and (b) with equations in nonstandard forms, in which operations may appear on either, neither, or both sides of the equal sign (e.g., 10 + 4 = __ + 7, 12 = 12, 14 = 9 + 5). When asked what the equal sign means, students with a relational view offer definitions such as “the same as” or “what is to the left and right of the sign mean the same thing” (Knuth et al., 2006).

Many students instead hold an operational conception of the equal sign, interpreting the symbol as meaning the “the total” or “the answer” (e.g., Baroody & Ginsburg, 1983; Knuth et al., 2006; McNeil, 2008; McNeil & Alibali, 2005; Rittle-Johnson et al., 2011). Students who hold an operational view tend to solve missing value equations by performing the given operations to the left of the equal sign or by performing all the given operations (e.g., for 8 + 4 = __ + 5, writing 12 or 17 in the blank; Carpenter et al., 2003). They also may refuse to endorse nonstandard equations, for example, rejecting 8 = 8 because it lacks an operation, or rejecting 9 = 5 + 4 because it is “backwards” (Falkner et al., 1999; Stephens et al., 2021, Stephens et al., 2022).

Although students’ conceptions of the equal sign have generally been characterized as relational or operational, Jones and Pratt (2012; see also Jones et al., 2012) assert that a complete relational understanding of the equal sign involves more than understanding “sameness”. They suggest understanding that one expression can be substituted by an equivalent one is another component of a sophisticated understanding—one that is distinct from the idea of sameness. They propose that a complete relational view of the equal sign includes both sameness and substitutive components. The substitutive conception arises from the transitive and symmetric properties of equivalence (Simsek et al., 2019) that allow equivalent expressions to replace one another in mathematical equations, and it sanctions interpretations of the equal sign such as “the right side can be swapped for the left side”. For example, if 5x + 8 = 2x − 4, then 5x + 8 can replace 2x − 4 and vice versa. A substitutive view also allows one to replace 21 in the expression 21 + 9 with 11 + 10, because 21 = 11 + 10 (Jones & Pratt, 2012).

To determine whether sameness and substitutive views are distinct, Jones et al. (2012) used a principal components analysis (PCA) to explore whether assessment items asking students to evaluate the “cleverness” of substitutive definitions clustered differently from items asking students to evaluate sameness and operational definitions of the equal sign. Based on data from 11- and 12-year-olds, they concluded that a relational view comprises distinct substitutive and sameness components. They did not find consistency in the order in which these two views developed; rather, they concluded that students held a more sophisticated relational understanding of the equal sign when they explicitly endorsed substitutive definitions.

Other work also suggests that the sameness and substitutive views of the equal sign are cognitively distinct. Jones and Pratt (2012) found that 9- to 12-year-old students were able to operate with the substitutive view independently of the sameness view. For example, students were able to use the given equations 30 + 7 = 37 and 50 + 8 = 58 as rules for making notational transformations to the expression 37 + 58 without considering the “sameness” of the sides of the equations.

Some research has suggested that the “sameness” conception develops prior to the substitutive conception. In an analysis of older students’ endorsements of definitions of the equal sign, Simsek et al. (2019) found that among students who accepted sameness, 56.1% rejected substitution, and among those who accepted substitution, only 3.5% rejected sameness. They also found that students who accepted both a sameness and a substitutive view of the equal sign were more successful on an algebra assessment than those who only accepted a sameness view.

In our prior work, we did not treat substitutive and sameness views as distinct, because we viewed the substitutive conception as a logical consequence of the sameness conception: two sides of an equation can be swapped, or one expression can be substituted for another, because they are the same. However, a recent laboratory study led us to reconsider the implications of a substitutive view. In this study, we presented third- and sixth-grade children with a lesson on the sameness conception of the equal sign (Donovan et al., 2019). Although the substitutive view was not presented in the lesson, the assessment included items in which children rated substitutive definitions of the equal sign as “good” or “not good” (see Jones et al., 2012). Third- and sixth-grade students who endorsed a substitutive definition (i.e., who rated “the two sides can be swapped” as “good”) performed better on items assessing equivalence understanding than did those who did not endorse a substitutive definition. In addition, sixth-grade students who endorsed a substitutive definition outperformed those who did not on algebraic items such as identifying a function rule from a table and solving linear equations with variables (Szkudlarek et al., 2021). Coupled with the findings of Simsek et al. (2019), these findings raised questions about the benefits of holding a dual conception—both a sameness and  a substitutive view—of the equal sign, rather than a sameness conception alone.

In the present study, we examined the impact of instruction about a sameness or a sameness and a substitutive view of the equal sign. Our outcome measures included oral definitions of the equal sign, endorsement of definitions, and strategies on missing value equations. We were interested in whether students would adopt structural equation-solving strategies for missing value equations. We use “structural” to describe strategies that involve “looking at expressions and equations in their entirety, noticing number relations among and within these expressions and equations” (Jacobs et al., 2007, p. 260). Our research questions were:

  1. 1.

    Do interventions focused on either a singular conception (sameness) or a dual conception (sameness and substitutive) of the equal sign promote greater equal sign understanding and increased application of structural equation-solving strategies, compared to a control condition?

  2. 2.

    Does an intervention focused on a dual conception of the equal sign promote greater equal sign understanding and increased application of structural equation-solving strategies, compared to an intervention focused solely on a sameness conception?

We hypothesized that students who received an intervention promoting a relational (sameness or dual) conception of the equal sign would show greater gains in their equal sign understanding and in the sophistication of their equation-solving strategies, as compared to students who did not receive an intervention. We further hypothesized that students who received an intervention promoting sameness and substitutive views of the equal sign would show greater gains in their equal sign understanding and in the sophistication of their strategies than students who received an intervention focused only on sameness.

3 Methods

3.1 Participants

Participants were 96 fourth- and 67 fifth-grade students (n = 163) recruited via Peachjar, a virtual flyer used to contact families through their school districts. We sent Peachjar flyers to 203 schools from 44 districts in four states in the midwestern United States. Please see (https://osf.io/gf5dc/?view_only=11dd753b384a4c64b334092d9f5a6bac) for participant demographic information.

Seventeen students were excluded from the final data set due to attrition at the second session (n = 4), technical difficulties (n = 8), or parents helping students with assessment items (n = 5). The final sample consisted of 86 fourth graders and 60 fifth graders (n = 146).

3.2 Data collection procedure

Data were collected during two one-on-one sessions conducted via Zoom by one of five experimenters. Students took a pretest at the beginning of Session 1, and this pretest started with the experimenter asking students to orally define the equal sign. The remainder of the pretest was presented via the online platform Qualtrics and included 61 items addressing understanding of mathematical equivalence and algebraic problem solving. The assessment was divided into a 17-min and a 20-min section to allow for a short break. Students were not able to skip items and were required to respond to all items, even if typing “I don’t know,” to progress. The experimenter was online throughout the assessment to address technical difficulties and offer general encouragement but did not see student responses. Parents were asked not to influence their children’s answers. Students participated in Session 2 of the intervention 3 to 7 days after Session 1, and they completed a posttest identical to the pretest.

3.3 Intervention

Participants were randomly assigned to one of three intervention conditions: (1) Sameness, focused on the “sameness” conception of the equal sign, (2) Sameness + Substitutive, focused on both sameness and substitutive conceptions, or (3) Control. The intervention consisted of a 7–10-min lesson at Session 1 and a 9–12-min lesson at Session 2. Lessons were delivered to individual students by an experimenter using an animated PowerPoint presentation. Students in the Control condition did not receive a lesson.

Session 1 In both the Sameness and Sameness + Substitutive conditions, Session 1 began with the experimenter asking, “What does the equal sign mean?” After the student gave their answer, the experimenter said, “The equal sign means that both sides of the equation are equal, or the same amount”. For students in the Sameness + Substitutive condition, the experimenter then said, “Another way to think about the equal sign is that amounts that are the same can be swapped or substituted”. Animations within the PowerPoint lesson were used to highlight the “sameness” or “swappability” of the sides.

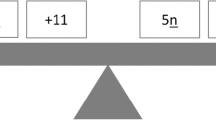
After the introduction, students engaged in a card activity (see Fig. 1) in which they were shown expression cards and asked to make true equations. To encourage students to form equations that were not in standard form, the experimenter suggested the first expression on some trials. For example, on one trial the experimenter put “10” on the left side of the equal sign, and in another trial put “0 + 8” on the right side, both times asking, “Can you pick a card to make this a true equation?” The Sameness intervention emphasized making sure that the equation was in fact true by checking for sameness of the two sides. The Sameness + Substitutive intervention emphasized that when students know an equation is true, the sides can be swapped to find another equation that is also true. Students completed a total of 5 trials with standard and nonstandard forms.

Fig. 1
figure 1

Card activity used in the intervention conditions

Full size image

Session 2 Session 2 included equations such as 24 + 15 = 24 + __ to encourage students to notice the underlying structure of sameness and to help them realize they do not need to compute the sums on both sides of the equal sign to find the missing value. Equations with identical or nearly identical numbers on either side of the equal sign have been found to encourage such structural thinking (e.g., Stephens et al., 2013).

The interventions diverged as the equations 47 + 26 = 44 + __ and 24 + __ = 28 + 58 were introduced. Students were asked how they could find the missing values without adding the numbers together (see Table 1). In both interventions, students were first asked how they would solve the equations. They then viewed slides narrated by the experimenter illustrating different strategies. The Sameness intervention used arrows to emphasize a compensation strategy for finding the missing values (see Fig. 2). The Sameness + Substitutive intervention focused on decomposition and the substitution of one expression for an equivalent one to reveal an underlying structure that made finding the missing value possible without calculating the sums on both sides (see Fig. 2).

Table 1 Sameness and Sameness + Substitutive lesson treatments of 24 + __ = 28 + 58
Full size table
Fig. 2
figure 2

Materials for lessons focused on sameness and dual sameness and substitutive conceptions of the equal sign

Full size image

The final equation posed during the lesson was 15 + 22 = 9 + __. This was a less “obvious” equation in terms of the distance between the values on each side of the equal sign and thus might invoke a computational strategy. The experimenter said, “This time, none of the numbers in this equation are particularly close together, but we can still look for the relationship between numbers to figure out what number goes in the blank, like before”. Students were asked their thoughts about how this equation could be solved with a focus on numerical relationships. The experimenter then presented either a compensation strategy (in the Sameness condition) or a substitution strategy (in the Sameness + Substitutive condition). Depending on whether students focused on the relationship between 15 and 9 or between 22 and 9 when first asked how they would solve the problem, one of two differing paths of instruction and accompanying animated slides were presented.

3.4 Assessment items and coding

We focused our coding and analysis on a subset of seven items. Three items addressed understanding of the equal sign: an equal sign definition item and two equal sign definition endorsement items (see Fig. 3). Four were missing value equations (see Fig. 4), with two in which the structural relationships were highly salient (i.e., numbers on either side of the equal sign were very close together), and two in which the structural relationships were non-salient.

Fig. 3
figure 3

Equal sign definition and equal sign definition endorsement items. In the results shared here, we focus on the italicized items

Full size image
Fig. 4
figure 4

Missing value equations

Full size image

3.4.1 Equal sign items

Students were shown an equal sign and asked what it means and if it could mean anything else (see Fig. 3). Items requiring students to produce definitions are common in studies exploring students’ understanding of the equal sign (e.g., Knuth et al., 2006; Madej, 2022; Matthews et al., 2012; McNeil & Alibali, 2005). Two additional items solicited students’ endorsements of various equal sign definitions (see Fig. 3). The endorsement items were modeled after those used in prior work (e.g., Donovan et al., 2019; Jones et al., 2013; Matthews et al., 2012). We presented each relational definition (i.e., the substitutive definition and the sameness definition) along with an operational definition and a distractor definition. These two items (each a group of three definitions to evaluate) were spaced so that students did not see them consecutively.

Students’ oral definitions were coded in terms of whether they included sameness and substitutive definitions. A response was coded as sameness if a student expressed the idea that the equal sign means “the same as” and as substitutive if the student expressed the idea that the equal sign means the two sides of an equation can be swapped or substituted for each other. Students’ responses to the definition endorsement items were coded for whether they endorsed “The equal sign means two amounts are the same” and “The equal sign means the two sides can be swapped” as “good” definitions. For the equal sign definition item, a primary coder coded all responses, and a reliability coder coded a randomly selected 20% of responses. Agreement between coders was 99%. The coders discussed all disagreements and came to consensus on final codes.

3.4.2 Missing value equations items

The four missing value equations examined students’ use of the structural strategies taught during the intervention. The first two items included numbers on either side of the equal sign that were only one apart from each other (salient items), and the third and fourth items included numbers that were farther apart (non-salient items). These items offered opportunities for students to apply both “sameness” and “substitutive” ways of thinking (see Fig. 4). The scheme for coding students’ responses was based on prior work (e.g., Donovan et al., 2019; Matthews et al., 2012) and modified to include substitution strategies. Responses were coded for correctness and strategy use.

For strategy use, students’ explanations were coded as structural if they attended to and correctly made use of relationships between numbers across the equal sign to find the missing value. The structural strategies that students used were a compensation strategy and a decomposition/substitution strategy. For example, a student using compensation for 67 + 84 = __ + 83 might notice that 84 is one more than 83 and conclude that the number in the box must be one more than 67, or 68, to maintain equivalence. A student using decomposition/substitution might rewrite the equation as 67 + 83 + 1 = __ + 83 and then find the value in the box by adding 67 + 1. In structural-incorrect strategies, students noticed relationships between numbers across the equal sign but then “compensated” in the wrong direction; for example, solving 67 + 84 = __ + 83 by saying, “84 is one more than 83; 67 is one more than 66” and placing 66 in the box.

Two coders initially coded the responses to all four missing value equations for a randomly selected 20% sample of students. Agreement was 96%. The coders came to consensus on all disagreements and clarified the meanings of the codes. In discussing discrepancies, a systematic disagreement on one explanation type for one item was noted. The coders came to a consensus on this explanation type, the primary coder then rechecked the full dataset, and the reliability coder recoded a new randomly selected 20% sample. Agreement between coders for this new reliability sample was 97%.

4 Results

4.1 Equal sign items

To evaluate the effects of the lesson conditions on the equal sign definition and endorsement items, we analyzed performance at posttest, controlling for performance on the corresponding items at pretest. We used logistic regression because the variables were dichotomous (e.g., offering a sameness definition or not). We analyzed the effect of condition using two planned orthogonal contrasts, one comparing the two lesson conditions (combined) to the control condition (coded − 0.67, 0.33, 0.33), and one comparing the Sameness condition to the Sameness + Substitutive condition (coded − 0.5, 0.5).

4.1.1 Equal sign definitions

About half of the students in all conditions offered sameness definitions of the equal sign prior to instruction (see Fig. 5). Students in the lesson conditions were more likely than students in the control condition to offer sameness definitions at posttest, b = 0.86, \({\chi }^{2}\)(1) = 4.46, p = 0.03, OR = 2.37. For example, a student in the Sameness condition stated “The equal sign means the answer” at pretest and “[The equal sign means] that both sides of the equation are the same” at posttest.

Fig. 5
figure 5

Percent of students offering sameness and substitutive definitions of the equal sign by condition and test

Full size image

No students offered substitutive definitions before instruction, so we controlled only for grade in analyzing this outcome. As predicted, students in the lesson conditions were more likely than students in the control condition to offer substitutive definitions at posttest, b = 3.02, \({\chi }^{2}\)(1) = 15.97, p < 0.001, OR = 20.52, and students in the Sameness + Substitutive condition were more likely to offer substitutive definitions than students in the Sameness condition, b = 3.16, \({\chi }^{2}\)(1) = 39.93, p < 0.001, OR = 23.53 (see Fig. 5). For example, a student in the Sameness + Substitutive condition stated “The equal sign means it like tells you the answer… for example 1 plus 1 equals, it tells you after the equal, it tells you the answer” at pretest and “[The equal sign] means that both sides of the equal sign is [sic] the same, you can swap it and it will still be the same” at posttest.

4.1.2 Endorsement of sameness and substitutive definitions

Students in all conditions were highly likely to endorse the sameness definition, even before instruction, and there were no differences in endorsement of the sameness definition across conditions following instruction (see Fig. 6).

Fig. 6
figure 6

Percent of students endorsing “the equal sign means two amounts are the same” and “the equal sign means the two sides can be swapped” by condition and test

Full size image

As predicted, students in lesson conditions were more likely than students in the control condition to endorse the substitutive definition at posttest, b = 1.83, \({\chi }^{2}\)(1) = 10.38, p = 0.001, OR = 6.26, and students in the Sameness + Substitutive condition were more likely to endorse a substitutive definition than students in the Sameness condition, b = 3.28, \({\chi }^{2}\)(1) = 16.43, p < 0.001, OR = 26.61 (see Fig. 6).

Thus, as predicted, students in the Sameness + Substitutive condition both generated and endorsed substitutive definitions of the equal sign in response to instruction that focused on the substitutive conception of equivalence.

4.2 Missing value items

We next considered the missing value items. We first present results regarding correctness and strategy use, and we then consider strategy use over time.

4.2.1 Correctness and use of structural strategies

The four missing value items were among the last items on the assessment, so not all students had time to complete them. We therefore present (in Table 2) the percent of students in each condition who correctly solved each item at pretest and posttest, among those who completed that specific item on both pretest and posttest. Note that in some cases, performance declined from pretest to posttest.

Table 2 Percent of students correctly solving the missing value items by condition and test
Full size table

Because students could have arrived at correct solutions via computation or via structural strategies, students’ numerical solutions alone do not reflect the potential impact of the lessons on structural thinking about the equations. We therefore focused our analyses on whether students employed structural strategies. Table 3 provides examples of correct structural strategies for each of the missing value equations, and Fig. 7 presents the proportion of students in each condition who used correct and incorrect structural strategies at pretest and posttest on each of the four items.

Table 3 Examples of correct structural strategies on missing value equations items
Full size table
Fig. 7
figure 7

Percent of students using correct and incorrect structural strategies for each item by condition and test

Full size image

We used a mixed-effects model to examine the likelihood that students used a structural strategy (either correct or incorrect) as a function of test (pretest or posttest), condition, item type (salient/non-salient), and the interactions of these factors. As for the analysis of equal sign definitions, we analyzed condition in terms of two contrasts, one comparing the two lesson conditions (combined) to the control condition, and one comparing the Sameness condition to the Sameness + Substitutive condition. We also included pretest equal sign definition (relational or not) and its interaction with test (pretest or posttest) as predictors, and we controlled for grade level. We included random effects (slopes and intercepts) within participants for test and item type.

Overall, students were more likely to use structural strategies at posttest than at pretest, B = 1.435, SE = 0.587, \({\chi }^{2}\)(1) = 5.968, p = 0.01, OR = 4.199. Students were also less likely to use structural strategies on non-salient items than salient items, B = -5.806, SE = 0.980, \({\chi }^{2}\)(1) = 35.085, p < 0.001, OR = 0.003 (see Fig. 7).

Students who offered a relational definition of the equal sign at pretest were more likely to use structural strategies than participants who did not, B = 3.218, SE = 0.951, \({\chi }^{2}\)(1) = 11.442, p < 0.001, OR = 24.96. Students who did not offer a relational definition at pretest were also more likely to increase their use of structural strategies from pretest to posttest, compared to those who did offer a relational definition at pretest (see Fig. 8), yielding a significant interaction of pretest definition and test, B = -4.159, SE = 1.522, \({\chi }^{2}\)(1) = 7.46, p = 0.006, OR = 0.016.

Fig. 8
figure 8

Percent of missing value items on which students used structural strategies as a function of pretest relational definition and test

Full size image

For missing value items, there were no significant effects that involved either of the condition contrasts. There was no evidence for greater change in use of structural strategies from pretest to posttest in the lesson conditions than in the control condition, B = 0.875, SE = 1.101, \({\chi }^{2}\)(1) = 0.631, p = 0.427, OR = 1.06, and no evidence for a difference between the Sameness and Sameness + Substitutive conditions, B = -0.538, SE = 1.467, \({\chi }^{2}\)(1) = 0.135, p = 0.714, OR = 0.58. There was also no effect of grade level on use of structural strategies.

Given the by-item variability in use of structural strategies, we also examined patterns of performance for each item separately (see Fig. 7). We used the same model structure as for the overall analysis, omitting item type. As in the overall analysis, these problem-by-problem analyses also did not reveal any significant effects of the condition contrasts.

4.2.2 Paths of change from pretest to posttest in structural strategy use

Finally, we examined patterns of change from pretest to posttest for individual learners. We first identified students who completed at least one salient and at least one non-salient item at both pretest and posttest (n = 28 Control students; n = 31 Sameness students; n = 33 Sameness + Substitutive students). We then classified each student’s performance on the salient and non-salient items. Students were classified as using structural strategies for each item type at each test if they used a (correct or incorrect) structural strategy for at least one of the two items in that category. We then classified each student’s performance at each test into one of the following categories: (1) no use of structural strategies, (2) use of structural strategies on salient items only, and (3) use of structural strategies on both salient and non-salient items. In a very small number of cases (1 at pretest, 2 at posttest, out of 92 at each time point), a student used a structural strategy on non-salient items only; we classified these cases as structural strategies on both types of items. The number of participants in each condition who demonstrated each path from pretest to posttest is presented in Fig. 9.

Fig. 9
figure 9

Shifts in use of structural strategies on salient and non-salient items by condition. Pretest is indicated on the left and posttest on the right. The lengths of the black, gray, and white bars correspond to the percent of students in each category at the relevant time point

Full size image

Among students who did not use structural strategies at pretest, the percent of students who progressed to using structural strategies (either on salient items or on both types of items) was greatest in the Sameness condition (7 of 8, 88%), followed by the Sameness + Substitutive condition (7 of 14, 50%), followed by the Control condition (2 of 7, 29%). These descriptive findings suggest that the lesson conditions, and in particular, the Sameness condition, helped students who did not initially notice numerical relationships begin to attend to structure and use these relationships to solve missing value items at posttest. However, some students in the Control condition also began to use structural strategies, and the value of the lessons must be considered in that light.

Among students who used structural strategies only on salient items at pretest, the percent of students who progressed to using structural strategies on both types of items at posttest was also greatest in the Sameness condition (6 of 9, 67%), followed by the Control condition (4 of 13, 31%), followed by the Sameness + Substitutive condition (2 of 10, 20%). Thus, for students who already used structural strategies on some items at pretest, the Sameness lesson was strikingly more beneficial than the Sameness + Substitutive lesson, with more than three times as many students progressing to using structural strategies on both item types at posttest.

Examples from one student in the Sameness condition classified as Neither-to-Salient and one student in the Sameness condition classified as Salient-to-Both are presented in Table 4. Corresponding examples for students in the Sameness + Substitutive condition are presented in Table 5. Note that in addition to using a computational strategy at pretest, Student 1 incorrectly used the equal sign to represent the results of calculations.

Table 4 Examples from students in the Sameness condition showing the neither-to-salient and salient-to-both structural strategy paths
Full size table
Table 5 Examples from students in the Sameness + Substitutive condition showing the neither-to-salient and salient-to-both structural strategy paths
Full size table

5 Discussion

The importance of a relational view of the equal sign for students’ success in algebra has been well established. Although relational has traditionally been construed in terms of sameness (Carpenter et al., 2003; Kieran, 1981; Knuth et al., 2006; McNeil et al., 2011), recent research suggests that a substitutive view is a distinct aspect of a full relational understanding (Jones et al., 2012; Simsek et al., 2019). Situated in this context, we investigated the impact of lessons focused on a sameness or a sameness and substitutive view of the equal sign on fourth- and fifth-grade students’ understanding.

More students in the lesson conditions than in the Control condition offered a sameness definition of the equal sign at posttest. Further, more students in the lesson conditions than in the Control condition offered and endorsed a substitutive definition at posttest, with more students in the Sameness + Substitutive condition than in the Sameness condition doing so. Neither lesson had a statistically significant impact on students’ likelihood of using structural strategies on the four missing value equation items. However, descriptive analyses of shifts in structural strategy use from pretest to posttest suggest that the lessons—in particular, the Sameness lessons—may have had some impact. We discuss shifts observed in students’ structural strategy use, reflect on the relationship between sameness and substitutive conceptions of the equal sign, consider our characterization of “algebraic structure” and “structural strategies” in the context of existing literature, and consider limitations and future directions.

5.1 Shifts in students’ use of structural equation-solving strategies

We found greater use of structural approaches on items in which the structural relationships in the equation were salient (e.g., __ + 55 = 37 + 54) than on items in which the structural relationships were non-salient (e.g., 60 + __ = 48 + 24), regardless of condition and test. This confirms findings (Carpenter et al., 2003; Stephens et al., 2013) that number choice can influence students’ equation-solving strategies and encourage attention to structure, or “looking” before “doing” (Hoch & Dreyfus, 2004). We did not find a statistically significant impact of condition on students’ likelihood of using structural strategies, but we did observe descriptive differences by condition when analyzing shifts in approaches to salient and non-salient items at the individual level (Fig. 9). We found that students in the lesson conditions, especially those in the Sameness condition, showed more movement towards structural strategies than Control students. Compared to students in the Sameness + Substitutive and Control conditions, students in the Sameness condition were more apt to shift from not using structural strategies on either item type to using structural strategies on salient items or to shift from using structural strategies only on salient items to using structural strategies on both types of items. Thus, the Sameness lesson may have influenced students’ tendency to notice numerical relationships across the equal sign when solving problems.

Why might the Sameness lesson have been beneficial for encouraging the adoption of structural equation-solving strategies? Although the sameness and substitutive conceptions may both be necessary components of a full relational view of the equal sign (Jones et al., 2012), it may be that teaching both conceptions in a relatively short amount of time put an unreasonable cognitive demand on students. Adopting a sameness conception alone might have been sufficient to support students in equation solving, whereas considering two conceptions at once may have been counterproductive for some. The short time allotted to the intervention may have been better spent focused on a single definition and equation-solving approaches consistent with this single definition than on dual conceptions of the equal sign.

5.2 Sameness and substitutive conceptions of the equal sign

It may also be the case that a sameness conception of the equal sign is a necessary precursor that must be in place prior to adoption of a meaningful substitutive conception. Although early findings were ambiguous regarding developmental ordering (e.g., Jones et al., 2012), more recent findings (Simsek et al., 2019) suggest that the sameness view develops prior to the substitutive view. Students who held a substitutive view almost always simultaneously held a sameness view, although the reverse was not true.

This sameness-before-substitution stance is consistent with our initial perspective that substitution does not constitute a wholly different conception of mathematical equivalence but rather logically follows from the sameness conception. That is, two amounts can be swapped or substituted because they represent the same values. This view is also consistent with Kieran and Martinez-Hernández’s (2022a, this issue) argument that “exchanging depends on the support of sameness”. Holding a substitutive view without a sameness view may be possible (e.g., Lee & Pang, 2021) but is potentially problematic. Although one may be taught procedural rules for substitution, these rules can be applied in ways that are inconsistent with a sameness conception of the equal sign. For example, Jones and Pratt (2012) found that students were adept at substituting in the context of solving puzzles to find a given sum, but they rarely noticed when these puzzles included false equalities. This finding that “children…engaged with making substitutions…but were not engaged with the numerical sameness of statements or the conservation of quantity” (p. 17) illustrates that teaching a substitutive view alone is not necessarily productive.

5.3 Attending to algebraic structure and using “structural strategies”

At the outset of this paper, we described structural thinking as using number and operation sense to reflect on mathematical expressions as objects rather than as arithmetic procedures to be carried out (Carpenter et al., 2003; Sfard, 1991), and we noted that students who think structurally can recognize expressions such as 8(x + 3) and 8x + 24 as equivalent without performing computations. This is consistent with Hoch and Dreyfus’s (2004) characterization of “structure sense” as a set of abilities that includes seeing an algebraic expression as an entity, dividing an entity into sub-structures, recognizing connections between structures, and recognizing which manipulations are both possible and useful. We also concur with Kieran’s (2018) assertion that structural thinking and generalizing are closely linked. To identify an algebraic generality (e.g., a “give and take” strategy described by a student in response to __ + 55 = 37 + 54) requires “identifying, lifting out, and expressing algebraic structure” (p. 81). Finally, our thinking about structure—especially as evidenced in the Sameness + Substitutive condition—aligns with Kieran and Martinez-Hernández’s (2022b) emphasis on decomposing, composing, and recomposing as a “dynamic and appropriate” approach that “may be at the heart of students’ structuring activity in primary school” (p. 40).

It is important to note, however, that strategies we characterized as structural in this study included both correct and incorrect approaches–so long as they indicated attention to structure. Some of the shifts illustrated in Fig. 9 (e.g., from no use of structural strategies to use on salient items, or from use of structural strategies only on salient items to use on both salient and non-salient items) were due in part to what we view as an explicit attention to structure coupled with an incorrect strategic attempt to account for that structure. What we characterized as an incorrect structural strategy (e.g., “84 is one more than 83; 67 is one more than 66” in response to 67 + 84 = __ + 83) might indicate an observation of a numerical relationship without the conceptual understanding necessary to guide action on the relationship, evoking Kirschner and Awtry’s (2004) caution against the “notational seductions of nonreflective visual pattern matching” (p. 248).

One might argue that a student who understands the relational meaning behind the strategy would not compensate in the wrong direction, but we contend that this is too strict an interpretation. Understanding of the equivalence construct is continuous and can be thought of in terms of a probabilistic Guttman scale (Rittle-Johnson et al., 2011). This means that understanding need not be complete to indicate some level of advancement (Matthews et al., 2012). It may be that simply recognizing structural relationships between numbers in an equation is an important first step, even if differences across the equal sign are not yet correctly coordinated.

While students may not initially know what to do with the observed relationships, this noticing may be an important step on the way to conceptual appreciation and use of structure. We echo calls to engage elementary and middle grades students in activities that encourage the identification and use of structures with numbers and operations (Kieran, 2018; Schifter, 2018) and to engage older students in explicitly analyzing algebraic expressions and equations and clearly articulating transformational processes derived from these analyses. Such activities can support students’ abilities to perform useful manipulations grounded in a deep understanding of structure (Kirschner & Awtry, 2004).

5.4 Limitations

Although we observed different patterns of shifts in strategy use for different item types across conditions, we found that change from pretest to posttest in the overall likelihood of using structural strategies did not differ for students in the lesson conditions (Sameness or Sameness + Substitutive), relative to students in the Control condition. There are several possible reasons for this null finding. First, the time spent on instruction—less than 30 min—may not have been enough for students in either lesson condition to become comfortable with the strategies and to integrate these strategies with the presented conceptions of the equal sign. For students in the Sameness + Substitutive condition, the short time frame may have been even more problematic, given the challenge of integrating two distinct conceptions of the equal sign.

Second, our intervention may have been insufficiently responsive to students’ mathematical thinking in the moment. Our interdisciplinary team of psychologists and mathematics educators aimed to strike a balance between conducting a controlled experiment in which all students had the same experience, and one in which students shared and expounded upon their mathematical thinking. It is largely accepted that mathematics instruction should build on students’ existing knowledge (Carpenter et al., 1996) and that students should have ample opportunities to ask questions, choose problem-solving methods, and engage in mathematical discussions (Carpenter et al., 2015; Ghousseini, 2015; Hiebert et al., 1997). Although our lessons included a few places where “next steps” depended on student responses, in most cases we offered students the opportunity to share their thinking but did not proceed in a way that hinged on these responses.

Finally, online data collection posed challenges. The project was originally conceived as an in-person, school-based study with small student groups participating in multiple sessions that encouraged student interaction and discourse. However, COVID-19-related school closures required that we move the entire study online. Apart from the orally presented equal sign definition item, our assessments were administered via Qualtrics. This meant that participants responded to the prompts “show your work” and “explain your thinking” by typing in text boxes. Although some students gave articulate and detailed explanations, many students did not. Student comfort with typing, the Qualtrics platform, and communication via Zoom all contributed to the quality and detail of the responses given. Had this study been conducted in person, structural strategies might have been illustrated with drawings and arrows on paper, rather than in a text box with limited affordances. It is also possible that the requirement to type inhibited higher-order reasoning by preventing students from producing gestures and using their bodies (Nathan & Martinez, 2015).

6 Future investigations and conclusion

In this paper, we reported findings from a subset of our assessment items. Future reports will examine results from less complex items (e.g., true/false equations and missing value equations with smaller numerical values) as well as more traditional algebra items (e.g., equation solving with variables, completing function tables, identifying function rules) by condition. We will also examine relationships between students’ conceptions of the equal sign and their success and strategy use on a variety of problem-solving items regardless of experimental condition.

Despite the lack of support for our hypothesis that a Sameness + Substitutive lesson would be most beneficial for developing students’ conceptions of the equal sign and related equation-solving strategies, we believe that the role of the substitutive view of the equal sign in equation solving and algebra learning is worthy of further investigation. Our intervention did not yield significant effects of lesson condition on use of structural strategies, but it does provide direction for future work. A longer-term intervention that allows for greater time on lessons and increased opportunity for interaction with peers around mathematical ideas might prove fruitful. Assessments that allow students to more fully explain their problem-solving strategies would also allow for greater insight into student thinking. If holding a substitutive view of the equal sign does help students integrate a new dimension of relational thinking about the equal sign, it is possible that shifts in students’ problem-solving strategies may follow.

In sum, we found that a short online intervention focused on a sameness or a dual sameness and substitutive conception of the equal sign enhanced students’ abilities to produce sameness and substitutive definitions of the equal sign. The impact of these lessons on students’ equation-solving strategies was less clear. Although there was some evidence that a focus on the sameness conception was more supportive of advancing students’ attention to equation structure, further research is needed to investigate more thoroughly the connections between students’ conceptions of equivalence and their use of structural equation-solving strategies.