Abstract
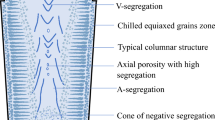
Positive centerline macrosegregation is an undesired casting defect that frequently occurs in the continuous casting process of steel strands. Mechanical softreduction (MSR) is a generally applied technology to avoid this casting defect in steel production. In the current paper, the mechanism of MSR is numerically examined. Therefore, two 25-m long horizontal continuous casting strand geometries of industrial scale are modeled. Both of these strand geometries have periodically bulged surfaces, but only one of them considers the cross-section reduction due to a certain MSR configuration. The macrosegregation formation inside of these strands with and without MSR is studied for a binary Fe-C-alloy based on an Eulerian multiphase model. Comparing the macrosegregation patterns obtained for different casting speed definitions allows investigating the fundamental influence of feeding, bulging and MSR mechanisms on the formation of centerline macrosegregation.












Similar content being viewed by others
Abbreviations
- \( A_{\text{SL}} \) :
-
Surface area of the cylindrical dendrites (mm2)
- \( C_{\text{L}} \) :
-
Species concentration in the liquid phase (wt pct)
- \( C_{\text{S}} \) :
-
Species concentration in the solid phase (wt pct)
- \( C_{\text{L}}^{*} \) :
-
Species concentration in the liquid at the solidification interface (wt pct)
- \( C_{\text{S}}^{*} \) :
-
Species concentration in the solid at the solidification interface (wt pct)
- \( C_{\text{L}}^{\text{C}} \) :
-
Concentration of carbon in the liquid (wt pct)
- \( C_{{{\text{L}},0}}^{\text{C}} \) :
-
Initial carbon concentration in the melt (liquid phase) (wt pct)
- \( C_{\text{S}}^{\text{C}} \) :
-
Concentration of carbon in the solid (wt pct)
- \( C_{{{\text{S}},{\text{E}}}}^{\text{C}} \) :
-
Eutectic carbon concentration (wt pct)
- \( C_{\text{M}}^{\text{C}} \) :
-
Mixture concentration of carbon (wt pct)
- \( \tilde{C}_{\text{M}} \) :
-
Volume specific mixture concentration (kg m−3)
- \( C_{\text{LS}}^{\text{D}} \) :
-
Diffusive species transfer rate from liquid to solid (kg m−3 s)
- \( C_{\text{SL}}^{\text{D}} \) :
-
Diffusive species transfer rate from solid to liquid (kg m−3 s)
- \( C_{\text{LS}}^{\text{M}} \) :
-
Species transfer due to phase change from liquid to solid (kg m−3 s)
- \( C_{\text{SL}}^{\text{M}} \) :
-
Species transfer due to phase change from solid to liquid (kg m−3 s)
- \( D_{\text{L}} \) :
-
Diffusion coefficient of the liquid phase (m2 s−1)
- \( H^{*} \) :
-
Volumetric heat transfer coefficient (W m−3 K)
- \( \Updelta H_{\text{m}} \) :
-
Latent heat of fusion (J kg−1)
- \( K \) :
-
Permeability of the mushy zone (mm2)
- \( Q_{\text{LS}}^{\text{D}} \) :
-
Energy exchange from liquid to solid due to heat transfer (J m−3 s)
- \( Q_{\text{SL}}^{\text{D}} \) :
-
Energy exchange from solid to liquid due to heat transfer (J m−3 s)
- \( Q_{\text{L}}^{\text{M}} \) :
-
Phase change energy of the liquid (J m−3 s)
- \( Q_{\text{S}}^{\text{M}} \) :
-
Phase change energy of the solid (J m−3 s)
- \( M_{\text{LS}} \) :
-
Net mass transfer rate from the liquid to the solid (kg s−1)
- \( M_{\text{SL}} \) :
-
Net mass transfer rate from the solid to the liquid (kg s−1)
- \( S_{{{\text{V}},{\text{T}}}} \) :
-
Ratio between dendritic surface area and total phase volume (mm−1)
- \( T_{\text{L}} \) :
-
Temperature of the liquid phase (K)
- \( T_{\text{S}} \) :
-
Temperature of the solid phase (K)
- \( T^{\text{envi}} \) :
-
Temperature of the strand environment (K)
- \( T^{\text{cast}} \) :
-
Casting temperature (initial melt temperature) (K)
- \( T_{\text{m}}^{\text{Fe}} \) :
-
Melting temperature of pure iron (K)
- \( \vec{V}_{\text{LS}}^{\text{M}} \) :
-
Momentum exchange due to the phase change from liquid to solid (kg m−2 s−2)
- \( \vec{V}_{\text{LS}}^{\text{D}} \) :
-
Momentum exchange due to drag force (kg m−2 s−2)
- \( V_{\text{T}} \) :
-
Total volume of the solid and the liquid (mm3)
- a, b :
-
Empirical constants (−)
- \( c_{{{\text{p}},{\text{L}}}} \) :
-
Specific heat capacity of the liquid phase (J kg−1 K−1)
- \( c_{{{\text{p}},{\text{S}}}} \) :
-
Specific heat capacity of the solid phase (J kg−1 K−1)
- \( d_{0} \) :
-
Initial bulging height (mm)
- \( f_{\text{L}} \) :
-
Volume fraction of the liquid phase (−)
- \( f_{{{\text{L}},0}} \) :
-
Initial liquid fraction (−)
- \( f_{\text{S}} \) :
-
Volume fraction of the solid phase (−)
- \( f_{\text{S}}^{\text{SPM}} \) :
-
Solid fraction for SPM calculations (−)
- \( f_{\text{S}}^{\text{zero}} \) :
-
Zero-strength solid fraction (−)
- \( \vec{g} \) :
-
Gravity acceleration (m s−2)
- \( h \) :
-
Distance between guiding rolls (mm)
- \( h_{\text{L}} \) :
-
Enthalpy of the liquid phase (J kg−1)
- \( h_{\text{S}} \) :
-
Enthalpy of the solid phase (J kg−1)
- \( k \) :
-
Partition coefficient (−)
- \( k_{\text{L}} \) :
-
Thermal conductivity of the liquid (W m−1 K−1)
- \( k_{\text{S}} \) :
-
Thermal conductivity of the solid (W m−1 K−1)
- l :
-
Strand length (mm)
- m :
-
Slope of the liquidus line in the linearized Fe–C phase diagram (K wt pct−1)
- n :
-
Total number of rolls (−)
- p :
-
Melt pressure (N mm−2)
- \( p^{\text{envi}} \) :
-
Pressure of the strand environment (atmospheric pressure) (K)
- q :
-
Mold length (mm)
- \( r_{1} \) :
-
Dendrite trunk radius (mm)
- \( r_{\infty } \) :
-
Maximum dendrite radius (mm)
- s :
-
Strand thickness reduction due to MSR (mm)
- t :
-
Time (s)
- \( \vec{v}_{\text{S}} \) :
-
Velocity vector of the solid phase (mm s−1)
- \( \vec{v}_{\text{L}} \) :
-
Velocity vector of the liquid phase (mm s−1)
- \( v^{\text{cast}} \) :
-
Casting velocity (mm s−1)
- \( v^{\text{pull}} \) :
-
Pull velocity (mm s−1)
- \( v_{{{\text{S}},x}} \) :
-
Internal solid phase velocity parallel to the cast direction (mm s−1)
- \( v_{{{\text{S}},y}} \) :
-
Internal solid phase velocity perpendicular to the cast direction (mm s−1)
- \( v_{{{\text{S}},x}}^{\text{surf}} \) :
-
Strand surface velocity parallel to the cast direction (mm s−1)
- \( v_{{{\text{S}},y}}^{\text{surf}} \) :
-
Strand surface velocity perpendicular to the cast direction (mm s−1)
- \( v_{{r_{1} }} \) :
-
Growth velocity of the dendrites in their thickness direction (mm s−1)
- w :
-
Overall strand thickness (mm)
- x :
-
Strand coordinate parallel to the cast direction (mm)
- \( x_{ - 1} \) :
-
Melt meniscus coordinate (mm)
- \( x_{0} \) :
-
Bulging start coordinate (mm)
- \( x_{1} \) :
-
MSR start coordinate (mm)
- \( x_{ 2} \) :
-
Bulging & MSR end coordinate (mm)
- \( x_{3} \) :
-
Strand end coordinate (mm)
- \( y \) :
-
Strand coordinate perpendicular to the cast direction (mm)
- \( y^{\text{surf}} \) :
-
Strand surface coordinate perpendicular to the cast direction (mm)
- \( \Upphi \) :
-
Impingement factor to consider dendritic overlapping (−)
- \( \gamma \) :
-
MSR efficiency factor (−)
- \( \eta_{\text{L}} \) :
-
Dynamic viscosity of the melt (kg m−1 s−1)
- \( \lambda_{1} \) :
-
Primary dendrite arm spacing (mm)
- \( \rho_{\text{L}} \) :
-
Density of the liquid phase (kg m−3)
- \( \rho_{\text{S}} \) :
-
Density of the solid phase (kg m−3)
- \( \bar{\rho }_{\text{S}} \) :
-
Average density of the solid phase (kg m−3)
References
K. Schwerdtfeger: Metallurgie des Stranggießens - Gießen und Erstarren von Stahl, Verlag Stahleisen, Düsseldorf, Germany, 1992.
R. Thome and K. Harste: Steel Res. Int., 2004, vol. 75, pp. 693-700.
R. Thome and K. Harste: ISIJ Int., 2006, vol. 46, pp. 1839-44.
W. Bleck, W. Wang and R. Bülte: Steel Res. Int., 2006, vol. 77, pp. 485-91.
H. Preßlinger, S. Ilie, P. Reisinger, A. Schiefermüller, A. Pissenberger, E. Parteder and C. Bernhard: ISIJ Int., 2006, vol. 46, pp. 1845-51.
O. Bode, K. Schwerdtfeger, H.G. Geck and F. Höfer: Ironmak. Steelmak., 2008, vol. 35, pp. 137-45.
S. Ogibayashi, M. Kobayashi, M. Yamada and T. Mukai: ISIJ Int., 1991, vol. 31, pp. 1400-07.
C. Beckermann: ASM Handbook, Volume 15: Casting, ASM International, Materials Park, OH, 2008, pp. 348-52.
C. Beckermann: Int. Mater. Rev., 2002, vol. 47, pp. 243-61.
M.C. Flemings and G.E. Nereo: Trans. Met. Soc. AIME, 1967, vol. 239, pp. 1449-61.
M.C. Flemings, R. Mehrabian and G.E. Nereo: Trans. Met. Soc. AIME, 1968, vol. 242, pp. 41-49.
M.C. Flemings and G.E. Nereo: Trans. Met. Soc. AIME, 1968, vol. 242, pp. 50-55.
K. Miyazawa and K. Schwerdtfeger: Arch. Eisenhüttenwesen, 1981, vol. 52, pp. 415-22.
P. Stadler, K. Hagen, P. Hammerschmid and K. Schwerdtfeger: Stahl Eisen, 1982, vol. 102, pp. 451-59.
T. Kajitani, J.-M. Drezet and M. Rappaz: Metall. Mater. Trans. A, 2001, vol. 32A, pp. 1479-91.
G. Lesoult: Mater. Sci. Eng. A, 2005, vol. 413-414, pp. 19-29.
F. Mayer, M. Wu, and A. Ludwig: in Modeling of Casting, Welding and Advanced Solidification Processes XII, S.L. Cockcroft and D.M. Maijer, eds., TMS, Warrendale, PA, 2009, pp. 279–86.
F. Mayer, M. Wu and A. Ludwig: Steel Res. Int., 2010, vol. 81, pp. 660-67.
M. Wu, J. Domitner and A. Ludwig: Metall. Mater. Trans. A, 2012, vol. 43A, pp. 945-64.
A. Ludwig and M. Wu: Mater. Sci. Eng. A, 2005, vol. 413-414, pp. 109-14.
M. Wu and A. Ludwig: Metall. Mater. Trans. A, 2006, vol. 37A, pp. 1613-31.
M. Wu and A. Ludwig: Metall. Mater. Trans. A, 2007, vol. 38A, pp. 1465-75.
J. Ni and C. Beckermann: Metall. Trans. B, 1991, vol. 22B, pp. 349-61.
C. Beckermann and R. Viskanta: Appl. Mech. Rev., 1993, vol. 46, pp. 1-27.
M.C. Schneider, J.P. Gu, C. Beckermann, W.J. Boettinger and U.R. Kattner: Metall. Mater. Trans. A, 1997, vol. 28A, pp. 1517-31.
A.K. Dahle and D.H. StJohn: Acta Mater., 1999, vol. 47, pp. 31-41.
J. Domitner, J.-M. Drezet, M. Wu and A. Ludwig: IOP Conf. Ser.: Mater. Sci. Eng., 2012, vol. 33, 012058.
N.N.: voestalpine Stahl GmbH, Linz (unpublished research).
Acknowledgments
The authors would like to thank the Christian Doppler Research Association (CDG) as well as the industrial partners voestalpine Stahl GmbH, voestalpine Stahl Donawitz GmbH & Co KG and Siemens VAI Metals Technologies GmbH & Co for the financial support of the project.
Author information
Authors and Affiliations
Corresponding author
Additional information
Manuscript submitted September 25, 2012.
Appendix
Appendix
The derivation of Eq. [53] is based on the assumption of steady-state conditions (\( \partial /\partial t = 0 \)), different densities for the liquid and the solid (\( \rho_{\text{L}} \ne \rho_{\text{S}} \)) as well as the non-divergence-free deformation of the solid (\( \nabla \cdot \vec{v}_{\text{S}} \ne 0 \)). For steady-state conditions, combining the species transfer equations [16] and [17] results in
By applying the chain rule to both terms, Eq. [A1] can be modified to
The continuity law for two contributing phases is given as
which can be modified by applying the chain rule to
Then, inserting the right-hand side of Eq. [A4] into Eq. [A2] results in
which can be simplified to
Based on the definition of the mixture concentration for carbon given in Eq. [52], the volume specific mixture concentration \( \tilde{C}_{\text{M}} \) is introduced:
Multiplying Eq. [A7] with \( \nabla ( \ldots ) \cdot \vec{v}_{\text{S}} \) results in
which is then used to extend Eq. [A6] in the following way:
One can simplify Eq. [A9] to
Applying the chain rule on the last term of Eq. [A10] results in
which is equivalent to
Since the densities \( \rho_{\text{S}} \) and \( \rho_{\text{L}} \) are different but constant and \( f_{\text{L}} = 1 - f_{\text{S}} \), the following simplification can be made:
Hence, one can write Eq. [A12] as
Rights and permissions
About this article
Cite this article
Domitner, J., Wu, M., Kharicha, A. et al. Modeling the Effects of Strand Surface Bulging and Mechanical Softreduction on the Macrosegregation Formation in Steel Continuous Casting. Metall Mater Trans A 45, 1415–1434 (2014). https://doi.org/10.1007/s11661-013-2060-9
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11661-013-2060-9