Study of Circular Dichroism Modes Through Decomposition of Planar Nanostructures

Abstract

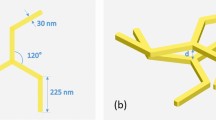
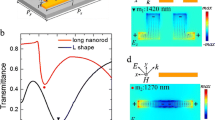
We extend the plasmon hybridization method from a single nanoparticle to a complex planar nanostructure, decomposing the complex nanostructure into fundamental nanoparticle building blocks. Using gammadion nanostructure as an example, we validated the theory by comparing the field profile in the gammadion’s arms under the influence of an incident circularly polarized wave. This allows us to address the origin of the plasmonics modes in the circular dichroism (CD) spectrum. The use of this hybridization method provides a simple and intuitive explanation on how conductive and inductive coupling may result from complex planar nanostructures, allowing us to study its optical properties. Using our approach, top down hybridization studies can be applied to other complex planar structures to gain further insight on the origin of the CD modes and enhance ultrasensitive sensing of chiral micro and macro molecules.

Appendix

Correlation of Mode m1 with Period of the Gammadion Array, a ₀

The period of the gammadion array, a ₀ is varied in the equation [18].

$$ {\lambda}_{\mathrm{SPP}}=\frac{n_{\mathrm{SPP}}{a}_0}{\sqrt{n^2+{m}^2}} $$

(6)

Since our structure is only fundamental, we only consider the fundamental SPP modes. For fundamental SPP modes, n = m = 1 for the mode on the gammadion. The effective localized surface plasmon is given as

$$ {n}_{\mathrm{SPP}}=\sqrt{\frac{\varepsilon_{\mathrm{Au}}{\varepsilon}_d}{\varepsilon_{\mathrm{Au}}+{\varepsilon}_d}} $$

(7)

We plot the graph of Eq. 6 with the wavelength positions of mode m1 in the CD spectra for different periods of the gammadion array. We simulate the gammadion array with periods of 850, 800, 750, 700 and 650 nm. Figure 8 shows the comparison between the model and simulation results.

Fig. 8

Plot of the Eq. 6 and mode 1 from CD spectra

From Fig. 8, we observed that Eq. 6 and the wavelength positions of mode m1 in CD spectra match excellently.

Calculation of Potential/Interaction Energy, U Between a Transverse Dipole and a Longitudinal Dipole to Account for Conductive Coupling in a Gammadion Structure

Circularly polarized light involves the superposition of both E _x and E _y polarized waves, assuming that the wave propagates in the z direction. Hence, it requires the consideration of both longitudinal and transverse coupling.

We can represent the transverse dipole as a separation of positive and negative charge + q ₁ and − q ₁, respectively. Similarly, the longitudinal dipole can be considered as a separation of positive and negative charge + q ₂ and − q ₂, respectively. Hence, the interaction energy, U, can be represented as follows, whereby $\frac{1}{4\pi {\varepsilon}_0}$ refers to the electrostatic constant. We have also defined x ₁ to be the distance between − q ₁ and − q ₂, x ₂ to be the distance between − q ₁ and + q ₂, x ₃ to be the distance between + q ₁ and − q ₂ and x ₄ to be the distance between + q ₁ and + q ₂.

$$ U=\frac{1}{4\pi {\varepsilon}_0}\left[\frac{\left(-{q}_1\right)\left(-{q}_2\right)}{x_1}+\frac{\left(-{q}_1\right){q}_2}{x_2}+\frac{q_1\left(-{q}_2\right)}{x_3}+\frac{q_1{q}_2}{x_4}\right]=\frac{q_1{q}_2}{4\pi {\varepsilon}_0}\left(\frac{1}{x_1}-\frac{1}{x_2}-\frac{1}{x_3}+\frac{1}{x_4}\right) $$

We need to obtain values for x ₁, x ₂, x ₃ and x ₄.

Using the Pythagoras theorem,

$$ \begin{array}{l}{\left({x}_1+\frac{d}{2}\right)}^2+{\left(\frac{d}{2}\right)}^2={r}^2\hfill \\ {}{x}_1=\sqrt{r^2-{\left(\frac{d}{2}\right)}^2}-\frac{d}{2}=r\left(\sqrt{1-{\left(\frac{d}{2r}\right)}^2}-\frac{d}{2r}\right)\hfill \end{array} $$

(8)

$$ {x}_2={x}_1+d=r\left(\sqrt{1-{\left(\frac{d}{2r}\right)}^2}-\frac{d}{2r}\right)+d=r\left(\sqrt{1-{\left(\frac{d}{2r}\right)}^2}+\frac{d}{2r}\right) $$

(9)

$$ \begin{array}{l}{x_3}^2={d}^2+{x_1}^2={d}^2+{\left(\sqrt{r^2-{\left(\frac{d}{2}\right)}^2}-\frac{d}{2}\right)}^2={d}^2+{r}^2-{\left(\frac{d}{2}\right)}^2-d\sqrt{r^2-{\left(\frac{d}{2}\right)}^2}+{\left(\frac{d}{2}\right)}^2={d}^2+{r}^2-d\sqrt{r^2-{\left(\frac{d}{2}\right)}^2}\hfill \\ {}{x}_3=\sqrt{d^2+{r}^2-d\sqrt{r^2-{\left(\frac{d}{2}\right)}^2}}=r\sqrt{{\left(\frac{d}{r}\right)}^2+1-\frac{d}{r}\sqrt{1-{\left(\frac{d}{2r}\right)}^2}}\hfill \end{array} $$

(10)

$$ \begin{array}{l}{x_4}^2={d}^2+{x_2}^2={d}^2+{\left(\sqrt{r^2-{\left(\frac{d}{2}\right)}^2}+\frac{d}{2}\right)}^2={d}^2+{r}^2-{\left(\frac{d}{2}\right)}^2+d\sqrt{r^2-{\left(\frac{d}{2}\right)}^2}+{\left(\frac{d}{2}\right)}^2={d}^2+{r}^2+d\sqrt{r^2-{\left(\frac{d}{2}\right)}^2}\hfill \\ {}{x}_4=\sqrt{d^2+{r}^2+d\sqrt{r^2-{\left(\frac{d}{2}\right)}^2}}=r\sqrt{{\left(\frac{d}{r}\right)}^2+1+\frac{d}{r}\sqrt{1-{\left(\frac{d}{2r}\right)}^2}}\hfill \end{array} $$

(11)

To simplify the expression, let $x=\frac{d}{2r}$

$$ {x}_1=r\left(\sqrt{1-{x}^2}-x\right) $$

(12)

$$ {x}_2=r\left(\sqrt{1-{x}^2}+x\right) $$

(13)

$$ {x}_3=r\sqrt{(2x)^2+1-2x\sqrt{1-{x}^2}}=r\sqrt{4{x}^2+1-2x\sqrt{1-{x}^2}} $$

(14)

$$ {x}_4 = r\sqrt{4{x}^2+1+2x\sqrt{1-{x}^2}} $$

(15)

Using Taylor series expansion,

$$ \frac{1}{x_1}\approx \frac{1}{r}\left\{1+x+\frac{3}{2}{x}^2+2{x}^3+\frac{23}{8}{x}^4+O\left({x}^5\right)\right\} $$

(16)

$$ \frac{1}{x_2}\approx \frac{1}{r}\left\{1-x+\frac{3}{2}{x}^2-2{x}^3+\frac{23}{8}{x}^4+O\left({x}^5\right)\right\} $$

(17)

$$ \frac{1}{x_3}\approx \frac{1}{r}\left\{1+x-\frac{1}{2}{x}^2-4{x}^3-\frac{49}{8}{x}^4+O\left({x}^5\right)\right\} $$

(18)

$$ \begin{array}{l}\frac{1}{x_4}\approx \frac{1}{r}\left\{1-x-\frac{1}{2}{x}^2+4{x}^3-\frac{49}{8}{x}^4+O\left({x}^5\right)\right\}\hfill \\ {}U=\frac{q_1{q}_2}{4\pi {\varepsilon}_0}\left(\frac{1}{x_1}-\frac{1}{x_2}-\frac{1}{x_3}+\frac{1}{x_4}\right)\approx \frac{q_1{q}_2}{4\pi {\varepsilon}_0r}\left(12{x}^3\right)\hfill \\ {}U\approx \frac{q_1{q}_2}{4\pi {\varepsilon}_0r}12{\left(\frac{d}{2r}\right)}^3\approx \frac{\left(\frac{3}{2}\right)d{q}_1d{q}_2d}{4\pi {\varepsilon}_0{r}^4}\approx \frac{\left(\frac{3}{2}d\right){p}_1{p}_2}{4\pi {\varepsilon}_0{r}^4}\hfill \end{array} $$

(19)

where p ₁ = q ₁ d and p ₂ = q ₂ d

p _1,2 = dipole moment of 1,2

Relation Between Energy Difference and Wavelength Difference

$$ \begin{array}{l}E=\frac{\mathrm{hc}}{\lambda}\hfill \\ {}\frac{\partial E}{\partial \lambda }=-\frac{\mathrm{hc}}{\lambda^2}\hfill \\ {}\partial \lambda =-\frac{\lambda^2}{\mathrm{hc}}\partial E\hfill \end{array} $$

When ∂E decreases, ∂λ decreases as well.