On the Performance of Downlink Multiuser Cognitive Radio Inspired Cooperative NOMA

Abstract

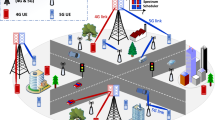
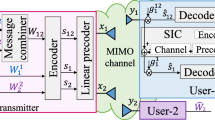
This paper is considered as an application of a centralized control non orthogonal multiple access (NOMA) based cognitive radio network. Here, a base station (BS) sends simultaneously two information signals by employing the superposition coding scheme to two different types of users, i.e., group of near users and one far user. The near users, namely, the secondary users, exchange cooperatively their own received information among themselves ensuring the realization of maximal diversity gain. Besides, they are responsible for relaying information to the far user, namely, the primary user. One potential secondary user is selected to decode and forward the BS information signal to the primary user and the rest of the secondary users to reinforce the reliability, as well as, mitigate the non-decodable messages. Two equivalent cases of a relay (secondary user) selection scheme are proposed. In the first case, the selection aims at maximizing the minimum of the joint secondary to secondary (S to S) and secondary to primary (S to P) channels’ coefficients under a certain limit of interference condition. In the second case, the selection aims at maximizing the minimum of the BS to S and S to S paths while a certain quality of service of the primary user is strictly guaranteed. Assuming Rayleigh fading channels, new closed form expressions are derived for the achievable capacity associated with the two information signals. Simulation results reveal the advantage of our proposed schemes over the conventional orthogonal max–min approach and confirm the validity of our analysis.

Appendices

Appendix 1

Let $\frac{{\left| {h_{ip} } \right|^{2} P_{R} - N_{0}^{2} {\mathbb{I}}}}{{\left| {h_{ip} } \right|^{2} P_{R} \left( {{\mathbb{I}} + 1} \right)}} > 0$, then, the R.V. ${\text{Z}}_{\text{i}}$ can be expressed by substitution as, ${\text{Z}}_{\text{i}} \triangleq \left( {min\left( {\gamma_{bi}^{\left( s \right)} ,\gamma_{ij} } \right)} \right) = min\left( {\frac{{\left| {h_{bi} } \right|^{2} P_{b} a_{si} }}{{N_{0}^{2} }},\frac{{\left| {h_{ij} } \right|^{2} P_{R} }}{{N_{0}^{2} }}\frac{{\left| {h_{ip} } \right|^{2} P_{R} - N_{0}^{2} {\mathbb{I}}}}{{\left| {h_{ip} } \right|^{2} P_{R} \left( {{\mathbb{I}} + 1} \right)}}} \right)$. Thus, we have to derive the CDF of $\gamma_{bi}^{\left( s \right)} \;{\text{and}}\; \gamma_{ij}$, separately.

Let $X_{i} = \frac{{\left| {h_{ip} } \right|^{2} P_{R} - N_{0}^{2} {\mathbb{I}}}}{{\left| {h_{ip} } \right|^{2} P_{R} \left( {{\mathbb{I}} + 1} \right)}}$, then, the CDF of $X_{i}$ will take the form, $Pr\left( {\frac{{\left| {h_{ip} } \right|^{2} P_{R} - N_{0}^{2} {\mathbb{I}}}}{{\left| {h_{ip} } \right|^{2} P_{R} \left( {{\mathbb{I}} + 1} \right)}} \le x} \right) \Rightarrow Pr\left( {\left| {h_{ip} } \right|^{2} P_{R} - N_{0}^{2} {\mathbb{I}} \le \left( {\left| {h_{ip} } \right|^{2} P_{R} \left( {{\mathbb{I}} + 1} \right)} \right)x} \right) \Rightarrow Pr\left( {\left| {h_{ip} } \right|^{2} \le \frac{{N_{0}^{2} {\mathbb{I}}}}{{P_{R} \left( {1 - x\left( {\left( {{\mathbb{I}} + 1} \right)} \right)} \right)}}} \right)$. Then, such that $\left( {1 - x\left( {\left( {{\mathbb{I}} + 1} \right)} \right)} \right) > 0$, the CDF is,

$F_{{X_{i} }} \left( {\frac{{N_{0}^{2} {\mathbb{I}}}}{{P_{R} \left( {1 - x\left( {\left( {{\mathbb{I}} + 1} \right)} \right)} \right)}}} \right) = 1 - \exp\left( { - \frac{{{{\Omega }}_{ip} N_{0}^{2} {\mathbb{I}}}}{{P_{R} \left( {1 - x\left( {\left( {{\mathbb{I}} + 1} \right)} \right)} \right)}}} \right)$.

Let $Y_{ij} = \frac{{\left| {h_{ij} } \right|^{2} P_{R} }}{{N_{0}^{2} }}$, then, the probability density function (PDF) of $Y_{i}$ can be expressed as,

$f_{{Y_{ij} }} \left( y \right) = \frac{{N_{0}^{2} }}{{P_{R} {{\Omega }}_{ij} }}\exp\left( { - \frac{{N_{0}^{2} }}{{P_{R} {{\Omega }}_{ij} }}y} \right)$.

The CDF of $\gamma_{ij} \triangleq X_{i} Y_{ij}$, $j \in {\mathcal{N}} - \left\{ i \right\}$, can be calculated using the integral, $F_{{\gamma_{ij} }} \left( z \right) = \mathop \int \limits_{0}^{\infty } F_{{X_{i} }} \left( {\frac{z}{x}} \right)f_{{Y_{ij} }} \left( x \right)dx$, as, $F_{{\gamma_{ij} }} \left( z \right) = 1 - \mathop \int \limits_{0}^{\infty } \exp\left( { - \frac{{\Omega_{ip} N_{0}^{2} {\mathbb{I}}}}{{P_{R} \left( {1 - \frac{z}{x}\left( {\left( {{\mathbb{I}} + 1} \right)} \right)} \right)}}} \right)\frac{{N_{0}^{2} }}{{P_{R} \Omega_{ij} }}\exp\left( { - \frac{{N_{0}^{2} }}{{P_{R} \Omega_{ij} }}x} \right)dx = 1 - \mathop \int \limits_{{z\left( {\left( {{\mathbb{I}} + 1} \right)} \right)}}^{\infty } \exp\left( { - \frac{{\Omega_{ip} N_{0}^{2} {\mathbb{I}}x}}{{P_{R} \left( {x - z\left( {\left( {{\mathbb{I}} + 1} \right)} \right)} \right)}}} \right)\frac{{N_{0}^{2} }}{{P_{R} \Omega_{ij} }}\exp\left( { - \frac{{N_{0}^{2} }}{{P_{R} \Omega_{ij} }}x} \right)dx$, such that $x > z\left( {\left( {{\mathbb{I}} + 1} \right)} \right)$, $\mathop \int \limits_{0}^{\infty } \frac{{N_{0}^{2} }}{{P_{R} \Omega_{ij} }}\exp\left( { - \frac{{N_{0}^{2} }}{{P_{R} \Omega_{ij} }}x} \right)dx = 1$. Putting $\theta = x - z\left( {\left( {{\mathbb{I}} + 1} \right)} \right)$, the CDF of $\gamma_{ij}$ becomes, $F_{{\gamma_{ij} }} \left( z \right) = 1 - \frac{{\Omega_{ij} N_{0}^{2} }}{{P_{R} }}\exp\left( { - \frac{{N_{0}^{2} }}{{P_{R} }}\left( {{\mathbb{I}}{{\Omega }}_{ip} + z\left( {{\mathbb{I}} + 1} \right){{\Omega }}_{ij} } \right)} \right) \times \mathop \int \limits_{0}^{\infty } \exp\left( { - \frac{{N_{0}^{2} }}{{P_{R} }}\left( {\Omega_{ij} \theta + \frac{{\Omega_{ip} z{\mathbb{I}}\left( {\left( {{\mathbb{I}} + 1} \right)} \right)}}{\theta }} \right)} \right)d\theta$.

Thus, $\bar{F}_{{\gamma_{ij} }} \left( z \right)$ follows after some algebraic manipulations and by using [22, 3.471.9]. Similarly, the CDF of $\gamma_{bi}^{\left( s \right)}$ can be expressed as,

$F_{{\gamma_{bi}^{\left( s \right)} }} \left( z \right) = 1 - \exp\left( { - \frac{{{{\Omega }}_{bi} N_{0}^{2} z}}{{P_{b} a_{si} }}} \right) = 1 - \bar{F}_{{\gamma_{bi}^{\left( s \right)} }} \left( z \right)$. Consequently, the CDF of ${\text{Z}}_{\text{i}} \triangleq \left( {min\left( {\gamma_{bi} ,\gamma_{ij} } \right)} \right)$ is obtained as,

$$F_{{Z_{i} }} \left( z \right) = Pr\left( {\left( {\gamma_{bi}^{\left( s \right)} > z,\gamma_{ij} > z} \right)} \right) = 1 - \bar{F}_{{Z_{i} }} \left( z \right) = 1 - \bar{F}_{{\gamma_{bi}^{\left( s \right)} }} \left( z \right)\bar{F}_{{\gamma_{ij} }} \left( z \right).$$

Finally, by applying, $Pr\left( {max_{i} \left( {Z_{i} } \right) \le z} \right) \Rightarrow max_{i} \left( {F_{{Z_{1} }} \left( z \right), F_{{Z_{2} }} \left( z \right), \ldots F_{{Z_{N} }} \left( z \right)} \right) = \mathop \prod \nolimits_{i = 1}^{N} F_{{Z_{i} }} \left( z \right)$, (17) follows and the proof is completed.

Appendix 2

From (20), let $Z_{p} \triangleq \left( {min\left( {\gamma_{bi}^{\left( p \right)} ,\gamma_{ip} + \gamma_{bp} } \right)} \right) = min\left( {\frac{{\left| {h_{bi} } \right|^{2} P_{b} a_{sp} }}{{\left| {h_{bi} } \right|^{2} P_{b} a_{si} + N_{0}^{2} }},\frac{{\left| {h_{ip} } \right|^{2} P_{R} a_{ip} }}{{\left| {h_{ip} } \right|^{2} P_{R} a_{ij} + N_{0}^{2} }} + \frac{{\left| {h_{bp} } \right|^{2} P_{b} a_{sp} }}{{\left| {h_{bp} } \right|^{2} P_{b} a_{si} + N_{0}^{2} }}} \right)$, then, the CDF of the R.V. $Z_{p}$ can be expressed as, $F_{{Z_{p} }} \left( {z_{1} ,z_{2} } \right) = 1 - \bar{F}_{{Z_{p} }} \left( {z_{1} ,z_{2} } \right) = 1 - Pr\left( {\left( {\gamma_{bi}^{\left( p \right)} > z_{1} ,\gamma_{ip} + \gamma_{bp} > z_{2} } \right)} \right) = 1 - \bar{F}_{{\gamma_{bi}^{\left( p \right)} }} \left( {z_{1} } \right)\bar{F}_{{\gamma_{ip} + \gamma_{bp} }} \left( {z_{2} } \right)$, where $\left\{ {\left\{ {\gamma_{bi}^{\left( p \right)} } \right\} \;{\text{and}}\; \left\{ {\gamma_{ip} + \gamma_{bp} } \right\}} \right\}$ are two disjoint events, $z_{1} \;{\text{and}}\; z_{2}$ are two distinct predefined SINRs. When the communication requires one desired or predefined SINR level, $z = z_{1} = z_{2}$, which leads to $\bar{F}_{{Z_{p} }} \left( z \right) = \bar{F}_{{\gamma_{bi}^{\left( p \right)} }} \left( z \right)\bar{F}_{{\gamma_{ip} + \gamma_{bp} }} \left( z \right)$. Thus, we have to derive the CDF of $\gamma_{bi}^{\left( p \right)} \;{\text{and}}\; \gamma_{ip} + \gamma_{bp}$, separately.

Let $P_{b} = P_{R} = P$, $\bar{F}_{{\gamma_{bi}^{\left( p \right)} }} \left( z \right)$ can be obtained simply as,

$$\bar{F}_{{\gamma_{bi}^{\left( p \right)} }} \left( z \right) = Pr\left( {\gamma_{bi}^{\left( p \right)} > z} \right) = Pr\left( {\frac{{\left| {h_{bi} } \right|^{2} Pa_{sp} }}{{\left| {h_{bi} } \right|^{2} Pa_{si} + N_{0}^{2} }} > z} \right) \Rightarrow \bar{F}_{{\gamma_{bi}^{\left( p \right)} }} \left( z \right) = Pr\left( {\left| {h_{bi} } \right|^{2} > \frac{{zN_{0}^{2} }}{{P\left( {a_{sp} - za_{si} } \right)}}} \right) = \exp\left( { - \frac{{{{\Omega }}_{bi} zN_{0}^{2} }}{{P\left( {a_{sp} - za_{si} } \right)}}} \right)$$

Since $\gamma_{ip} \,\,and\,\, \gamma_{bp}$ are two disjoint random variables and $\bar{F}_{{\gamma_{ip} + \gamma_{bp} }} \left( z \right) = Pr\left( {\frac{{\left| {h_{ip} } \right|^{2} Pa_{ip} }}{{\left| {h_{ip} } \right|^{2} Pa_{ij} + N_{0}^{2} }} + \frac{{\left| {h_{bp} } \right|^{2} Pa_{sp} }}{{\left| {h_{bp} } \right|^{2} Pa_{si} + N_{0}^{2} }} > z} \right)$, the CDF $\bar{F}_{{\gamma_{ip} + \gamma_{bp} }} \left( z \right)$ can be determined using the integral, $\bar{F}_{{\gamma_{ip} + \gamma_{bp} }} \left( z \right) = 1 - \mathop \int \limits_{0}^{\infty } F_{{\gamma_{bp} }} \left( {z - x} \right)f_{{\gamma_{ip} }} \left( z \right)dx$.

By following similar steps as $\bar{F}_{{\gamma_{bi}^{\left( p \right)} }} \left( z \right)$, $\bar{F}_{{\gamma_{bp} }} \left( z \right)$ and $\bar{F}_{{\gamma_{ip} }} \left( z \right)$ will take the forms of, $\bar{F}_{{\gamma_{bp} }} \left( z \right) = \exp\left( { - \frac{{{{\Omega }}_{bp} zN_{0}^{2} }}{{P\left( {a_{sp} - za_{si} } \right)}}} \right)$ and $\bar{F}_{{\gamma_{ip} }} \left( z \right) = \exp\left( { - \frac{{{{\Omega }}_{ip} zN_{0}^{2} }}{{P\left( {a_{ip} - za_{ij} } \right)}}} \right)$, respectively.

Taking the derivative of $F_{{\gamma_{ip} }} \left( z \right) = 1 - \bar{F}_{{\gamma_{ip} }} \left( z \right)$, the PDF can be expressed as, $f_{{\gamma_{ip} }} \left( z \right) = \frac{{a_{ip} N_{0}^{2} }}{{P\left( {a_{ip} - za_{ij} } \right)^{2} }}\exp\left( { - \frac{{{{\Omega }}_{ip} zN_{0}^{2} }}{{P\left( {a_{ip} - za_{ij} } \right)}}} \right)$.$\bar{F}_{{\gamma_{ip} + \gamma_{bp} }} \left( z \right) = 1 - \mathop \int \limits_{0}^{\infty } F_{{\gamma_{bp} }} \left( {z - x} \right)f_{{\gamma_{ip} }} \left( z \right)dx = \exp\left( {\frac{{\Omega_{ip} N_{0}^{2} }}{{Pa_{ij} }}} \right) + \underbrace {{\mathop \int \limits_{0}^{\infty } \frac{{a_{ip} N_{0}^{2} }}{{P\left( {a_{ip} - xa_{ij} } \right)^{2} }}\exp\left( { - \frac{{N_{0}^{2} }}{P}\left( {\frac{{\Omega_{ip} x}}{{\left( {a_{ip} - xa_{ij} } \right)}} + \frac{{\Omega_{bp} \left( {z - x} \right)}}{{\left( {a_{sp} - \left( {z - x} \right)a_{si} } \right)}}} \right)} \right)dx}}_{{{\mathcal{J}}\left( z \right)}}$.

The integral ${\mathcal{J}}\left( z \right)$ can be solved for certain $z$ by numerical software programming or it can be approximated by expanding $\exp\left( . \right)$ for $\frac{{N_{0}^{2} }}{P} \ll 1$.

For arbitrary $z \in \left[ {0,\infty } \right]$, since $\frac{{\left| {h_{ip} } \right|^{2} Pa_{ip} }}{{\left| {h_{ip} } \right|^{2} Pa_{ij} + N_{0}^{2} }} \le \frac{{a_{ip} }}{{a_{ij} }}$, $\frac{{\left| {h_{bp} } \right|^{2} Pa_{sp} }}{{\left| {h_{bp} } \right|^{2} Pa_{si} + N_{0}^{2} }} \le \frac{{a_{sp} }}{{a_{si} }}$ and noting that $Pr\left( {\left( {\gamma_{ip} + \gamma_{bp} } \right) = z} \right) = 0$, i.e., for continuous R.V.s, $\bar{F}_{{\gamma_{ip} + \gamma_{bp} }} \left( z \right)$ can be obtained by the following three cases:

• I. $z < \frac{{a_{ip} }}{{a_{ij} }} < \frac{{a_{sp} }}{{a_{si} }}$

In such a case, if $\frac{{\left| {h_{bp} } \right|^{2} Pa_{sp} }}{{\left| {h_{bp} } \right|^{2} Pa_{si} + N_{0}^{2} }} > z$ or $\frac{{\left| {h_{ip} } \right|^{2} Pa_{ip} }}{{\left| {h_{ip} } \right|^{2} Pa_{ij} + N_{0}^{2} }} > z$, then, $Pr\left( {\frac{{\left| {h_{ip} } \right|^{2} Pa_{ip} }}{{\left| {h_{ip} } \right|^{2} Pa_{ij} + N_{0}^{2} }} + \frac{{\left| {h_{bp} } \right|^{2} Pa_{sp} }}{{\left| {h_{bp} } \right|^{2} Pa_{si} + N_{0}^{2} }} > z} \right) = 1$. Thus, $\begin{aligned} \bar{F}_{{\gamma_{ip} + \gamma_{bp} }} \left( z \right) = Pr\left( {\frac{{\left| {h_{bp} } \right|^{2} Pa_{sp} }}{{\left| {h_{bp} } \right|^{2} Pa_{si} + N_{0}^{2} }} > z} \right) + Pr\left( {\frac{{\left| {h_{bp} } \right|^{2} Pa_{sp} }}{{\left| {h_{bp} } \right|^{2} Pa_{si} + N_{0}^{2} }} < z} \right)Pr\left( {\frac{{\left| {h_{ip} } \right|^{2} Pa_{ip} }}{{\left| {h_{ip} } \right|^{2} Pa_{ij} + N_{0}^{2} }} > z} \right) +\, Pr\left( {\frac{{\left| {h_{ip} } \right|^{2} Pa_{ip} }}{{\left| {h_{ip} } \right|^{2} Pa_{ij} + N_{0}^{2} }} < z} \right) \times \hfill \\ Pr\left( {\frac{{\left| {h_{bp} } \right|^{2} Pa_{sp} }}{{\left| {h_{bp} } \right|^{2} Pa_{si} + N_{0}^{2} }} < z} \right) \times Pr\left( {\frac{{\left| {h_{ip} } \right|^{2} Pa_{ip} }}{{\left| {h_{ip} } \right|^{2} Pa_{ij} + N_{0}^{2} }} + \frac{{\left| {h_{bp} } \right|^{2} Pa_{sp} }}{{\left| {h_{bp} } \right|^{2} Pa_{si} + N_{0}^{2} }} > z} \right) = \exp\left( { - \frac{{\Omega_{bp} zN_{0}^{2} }}{{P\left( {a_{sp} - za_{si} } \right)}}} \right) +\, \exp\left( { - \frac{{\Omega_{ip} zN_{0}^{2} }}{{P\left( {a_{ip} - za_{ij} } \right)}}} \right) - \hfill \\ \exp\left( { - \frac{{\Omega_{ip} zN_{0}^{2} }}{{P\left( {a_{ip} - za_{ij} } \right)}}} \right) \times \exp\left( { - \frac{{\Omega_{bp} zN_{0}^{2} }}{{P\left( {a_{sp} - za_{si} } \right)}}} \right) + \left( {\exp\left( {\frac{{\Omega_{ip} N_{0}^{2} }}{{Pa_{ij} }}} \right) + {\mathcal{J}}\left( z \right)} \right) \times \hfill \\ \left( {1 - \exp\left( { - \frac{{\Omega_{ip} zN_{0}^{2} }}{{P\left( {a_{ip} - za_{ij} } \right)}}} \right) - \exp\left( { - \frac{{\Omega_{bp} zN_{0}^{2} }}{{P\left( {a_{sp} - za_{si} } \right)}}} \right) + \exp\left( { - \frac{{\Omega_{ip} zN_{0}^{2} }}{{P\left( {a_{ip} - za_{ij} } \right)}}} \right) \times \exp\left( { - \frac{{\Omega_{bp} zN_{0}^{2} }}{{P\left( {a_{sp} - za_{si} } \right)}}} \right)} \right) \hfill \\ \end{aligned}$

• II. $\frac{{a_{ip} }}{{a_{ij} }} < z < \frac{{a_{sp} }}{{a_{si} }}$

$$\begin{aligned} \bar{F}_{{\gamma_{ip} + \gamma_{bp} }} \left( z \right) & = \Pr \left( {\frac{{\left| {h_{bp} } \right|^{2} Pa_{sp} }}{{\left| {h_{bp} } \right|^{2} Pa_{si} + N_{0}^{2} }} > z} \right) + \Pr \left( {\frac{{\left| {h_{bp} } \right|^{2} Pa_{sp} }}{{\left| {h_{bp} } \right|^{2} Pa_{si} + N_{0}^{2} }} < z} \right) \times \Pr \left( {\frac{{\left| {h_{ip} } \right|^{2} Pa_{ip} }}{{\left| {h_{ip} } \right|^{2} Pa_{ij} + N_{0}^{2} }} + \frac{{\left| {h_{bp} } \right|^{2} Pa_{sp} }}{{\left| {h_{bp} } \right|^{2} Pa_{si} + N_{0}^{2} }} > z} \right) \\ & = \exp \left( { - \frac{{\Omega_{bp} zN_{0}^{2} }}{{P\left( {a_{sp} - za_{si} } \right)}}} \right) + \left( {\exp \left( {\frac{{\Omega_{ip} N_{0}^{2} }}{{Pa_{ij} }}} \right) + {\mathcal{J}}\left( z \right)} \right) - \left( {\exp \left( {\frac{{\Omega_{ip} N_{0}^{2} }}{{Pa_{ij} }}} \right) + {\mathcal{J}}\left( z \right)} \right) \times \exp \left( { - \frac{{\Omega_{bp} zN_{0}^{2} }}{{P\left( {a_{sp} - za_{si} } \right)}}} \right) \\ \end{aligned}$$

• III. $\frac{{a_{ip} }}{{a_{ij} }} < \frac{{a_{sp} }}{{a_{si} }} < z$

$\bar{F}_{{\gamma_{ip} + \gamma_{bp} }} \left( z \right) = Pr\left( {\frac{{\left| {h_{ip} } \right|^{2} Pa_{ip} }}{{\left| {h_{ip} } \right|^{2} Pa_{ij} + N_{0}^{2} }} + \frac{{\left| {h_{bp} } \right|^{2} Pa_{sp} }}{{\left| {h_{bp} } \right|^{2} Pa_{si} + N_{0}^{2} }} > z} \right) = \left( {\exp\left( {\frac{{\Omega_{ip} N_{0}^{2} }}{{Pa_{ij} }}} \right) + {\mathcal{J}}\left( z \right)} \right)$.

The proof is completed.