Skip to main content
SpringerLink
Log in

Effects of Time-Periodic Thermal Boundary Conditions and Internal Heating on Heat Transport in a Porous Medium

  • Published:
Transport in Porous Media Aims and scope Submit manuscript

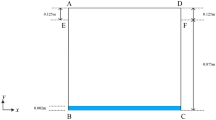
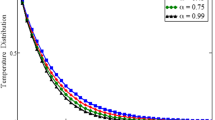
Abstract

The effects of time-periodic boundary temperatures and internal heating on Nusselt number in the Bénard–Darcy convective problem has been considered. The amplitudes of temperature modulation at the lower and upper surfaces are considered to be very small. By performing a weakly non-linear stability analysis, the Nusselt number is obtained in terms of the amplitude of convection, which is governed by the non-autonomous Ginzburg–Landau equation, derived for the stationary mode of convection. The effects of internal Rayleigh number, amplitude and frequency of modulation, thermo-mechanical anisotropies, and Vadasz number on heat transport have been analyzed and depicted graphically. Increasing values of internal Rayleigh number results in the enhancement of heat transport in the system. Further, the study establishes that the heat transport can be controlled effectively by a mechanism that is external to the system.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6

Similar content being viewed by others

Abbreviations

\(A\) :

Amplitude of convection

\(d\) :

Height of the fluid layer

\(Da\) :

Darcy number \(Da=K_{z}/d^{2}\)

\({g}\) :

Acceleration due to gravity

\(Q\) :

Internal heat source

\(k_\mathrm{c}\) :

Critical wavenumber

\(Nu\) :

Nusselt number

\(p\) :

Reduced pressure

\(Pr\) :

Prandtl number, \(Pr=\nu /\kappa _{{T}_{z}}\)

\(Ra\) :

Thermal Rayleigh number, \(Ra=\alpha _{T}\text{ g}K_{z}(\Delta T)d/\nu {\kappa _{T}}_{z}\)

\(R_\mathrm{0c}\) :

Critical Rayleigh number

\(R_{i}\) :

Internal Rayleigh number, \(R_{i}=Qd^{2}/{\kappa _{T}}_{z}\)

\(Va\) :

Vadász number, \(Va=\phi Pr/Da\)

\(t\) :

Time

\(T\) :

Temperature

\(\Delta T\) :

Temperature difference across the fluid layer

x,y,z:

Space co-ordinates

\(\alpha _T\) :

Coefficient of thermal expansion

\(\delta ^2\) :

Horizontal wave number \(k_\mathrm{c}^2 +\pi ^{2}\)

\(\delta _{1}\) :

Amplitude of temperature modulation

\(\Omega \) :

Frequency of modulation

\(\epsilon \) :

Perturbation parameter

\(\gamma \) :

Heat capacity ratio \(\frac{(\rho c_{p})_{m}}{(\rho c_{p})_{f}}\)

\(\mathbf K \) :

Permeability tensor

\(\kappa _{T}\) :

Effective thermal diffusivity

\(\mu \) :

Effective dynamic viscosity of the fluid

\(\nu \) :

Effective kinematic viscosity, \(\left({\frac{\mu }{\rho _{0}}} \right)\)

\(\phi \) :

Porosity

\(\theta \) :

Phase angle

\(\psi \) :

Stream function

\(\rho \) :

Fluid density

\(\tau \) :

Slow time \(\tau =\epsilon ^{2}t\)

\(\nabla ^{2}\) :

\(\frac{\partial ^{2}}{\partial x^{2}}+\frac{\partial ^{2}}{\partial y^{2}}+\frac{\partial ^{2}}{\partial z^{2}}\)

\(\nabla ^{2}_{1}\) :

\(\frac{\partial ^{2}}{\partial x^{2}}+\frac{\partial ^{2}}{\partial z^{2}}\)

\(\nabla ^{2}_{\xi }\) :

\(\frac{\partial ^{2}}{\partial x^{2}}+\frac{1}{\xi }\frac{\partial ^{2}}{\partial z^{2}}\)

\(\nabla ^{2}_{\eta }\) :

\(\eta \frac{\partial ^{2}}{\partial x^{2}}+\frac{\partial ^{2}}{\partial z^{2}}\)

\(b\) :

Basic state

\(c\) :

Critical

\(0\) :

Reference value

\(^{\prime }\) :

Perturbed quantity

\(*\) :

Dimensionless quantity

\(st\) :

Stationary

References

  • Bhadauria, B.S., Bhatia, P.K.: Time periodic heating of Rayleigh–Bénard convection. Phys. Scripta 66, 59–65 (2002)

    Article  Google Scholar 

  • Bhadauria, B.S.: Time-periodic heating of Rayleigh–Benard convection in a vertical magnetic field. Phys. Scripta 73(3), 296–302 (2006)

    Article  Google Scholar 

  • Bhadauria, B.S.: Thermal modulation of Rayleigh–Bénard convection in a sparsely packed porous medium. J. Porous Media 10, 175–188 (2007)

    Article  Google Scholar 

  • Bhadauria, B.S., Bhatia, P.K., Debnath, L.: Weakly non-linear analysis of Rayleigh–Bénard convection with time periodic heating. Int. J. Non-Linear Mech. 44(1), 58–65 (2009)

    Article  Google Scholar 

  • Bhadauria, B.S., Anoj Kumar, Jogendra Kumar, Pallath Chandran : Natural convection in a rotating anisotropic porous layer with internal heat generation. Transp. Porous Media 90, 687–705 (2011)

    Google Scholar 

  • Bhadauria, B.S.: Double diffusive convection in a saturated anisotropic porous layer with internal heat source. Transp. Porous Med. 92, 299–320 (2012)

    Article  Google Scholar 

  • Bhadauria, B.S., Siddheshwar, P.G., Suthar, O.P.: Weak nonlinear stability analysis of temperature/gravity modulated stationary Rayleigh–Bénard convection in a rotating porous medium. Transp. Porous Media 92, 633–647 (2012)

    Article  Google Scholar 

  • Bhattacharya, S.P., Jena, S.K.: Thermal instability of a horizontal layer of micropolar fluid with heat source. Proc. Indian Acad. Sci. (Math. Sci.) 93(1), 13–26 (1984)

    Article  Google Scholar 

  • Caltagirone, J.P.: Stabilite d’une couche poreuse horizontale soumise a des conditions aux limites periodiques. Int. J. Heat Mass Transf. 19, 815–820 (1976)

    Article  Google Scholar 

  • Chandrasekhar, S.: Hydrodynamic and hydromagnetic stability. Oxford University Press, London (1961)

    Google Scholar 

  • Chhuon, B., Caltagirone, J.P.: Stability of a horizontal porous layer with timewise periodic boundary conditions. J. Heat Transf. 101, 244–248 (1979)

    Article  Google Scholar 

  • Epherre, J.F.: Crit’ere d’apparition de la convection naturalle dans une couche poreuse anisotrope. Rev. Gén. Thermique, 168, 949–950 (1975) [English translation, Int. Chem. Eng. 17, 615–616 (1977)]

    Google Scholar 

  • Gershuni, G.Z., Zhukhovitskii, E.M.: On parametric excitation of convective instability. J. Appl. Math. Mech. 27, 1197–1204 (1963)

    Article  Google Scholar 

  • Haajizadeh, M., Ozguc, A.F., Tien, C.L.: Natural convection in a vertical porous enclosure with internal heat generation. Int. J. Heat Mass Transf. 27, 1893–1902 (1984)

    Article  Google Scholar 

  • Herron Isom, H.: Onset of convection in a porous medium with internal heat source and variable gravity. Int. J. Eng. Sci. 39, 201–208 (2001)

    Article  Google Scholar 

  • Ingham, D.B., Pop, I.: Transport phenomena in porous media. Pergamon, Oxford (1998)

    Google Scholar 

  • Ingham, D.B., Pop, I.: Transport phenomena in porous media, vol. III. Elsevier, Oxford (2005)

    Google Scholar 

  • Joshi, M.V., Gaitonde, U.N., Mitra, S.K.: Analytical study of natural convection in a cavity with volumetric heat generation. ASME J. Heat Transf. 128, 176–182 (2006)

    Article  Google Scholar 

  • Khalili, A., Huettel, M.: Effects of throughflow and internal heat generation on convective instabilities in an anisotropic porous layer. J. Porous Media 5(3), 64–75 (2002)

    Google Scholar 

  • Kuznetsov, A.V., Nield, D.A.: The effects of combined horizontal and vertical heterogenity on the onset of convection in a porous medium: double diffusive case. Transp. Porous Media 72, 157–170 (2008)

    Article  Google Scholar 

  • Lapwood, E.R.: Convection of a fluid in porous medium. Proc. Camb. Philos. Soc. 44, 508–521 (1948)

    Article  Google Scholar 

  • Malashetty, M.S., Wadi, V.S.: Rayleigh–Bénard convection subject to time dependent wall temperature in a fluid saturated porous layer. Fluid Dyn. Res. 24, 293–308 (1999)

    Article  Google Scholar 

  • Nield, D.A., Bejan, A.: Convection in porous media, 3rd edn. Springer, New York (2006)

    Google Scholar 

  • Parthiban, C., Patil, P.R.: Thermal instability in an anisotropic porous medium with internal heat source and inclined temperature gradient. Int. Commun. Heat Mass Transf. 24(7), 1049–1058 (1997)

    Article  Google Scholar 

  • Rajagopal, K.R., Saccomandib, G., Vergoric, L.: A systematic approximation for the equations governing convection–diffusion in a porous medium. Nonlinear Anal. Real World Appl. 11(4), 2366–2375 (2010)

    Article  Google Scholar 

  • Raju, V.R.K., Bhattacharya, S.N.: Onset of thermal instability in a horizontal layer of fluid with modulated boundary temperatures. J. Eng. Math. 66, 343–351 (2010)

    Article  Google Scholar 

  • Rao, Y.F., Wang, B.X.: Natural convection in vertical porous enclosures with internal heat generation. Int. J. Heat Mass Transf. 34, 247–252 (1991)

    Article  Google Scholar 

  • Rionero, S., Straughan, B.: Convection in a porous medium with internal heatsource and variable gravity effects. Int. J. Eng. Sci. 28(6), 497–503 (1990)

    Article  Google Scholar 

  • Roppo, M.H., Davis, S.H., Rosenblat, S.: Bénard convection with time-periodic heating. Phys. Fluids 27(4), 796–803 (1974)

    Article  Google Scholar 

  • Rosenblat, S., Herbert, D.M.: Low frequency modulation of thermal instability. J. Fluid Mech. 43, 385–398 (1970)

    Article  Google Scholar 

  • Rosenblat, S., Tanaka, G.A.: Modulation of thermal convection instability. Phys. Fluids 14(7), 1319–1322 (1971)

    Article  Google Scholar 

  • Siddheshwar, P.G.: A series solution for the Ginzburg–Landau equation with a time-periodic coefficient. Appl. Math. 6, 542–554 (2010)

    Google Scholar 

  • Siddheshwar, P.G., Vanishree, R.K., Melson, A.C.: Study of heat transport in Bénard–Darcy convection with g-jitter and thermomechanical anisotropy in variable viscosity liquids. Transp. Porous Media 92(2), 277–288 (2012a)

  • Siddheshwar, P.G., Bhadauria, B.S., Srivastava, A.: An analytical study of nonlinear double diffusive convection in a porous medium under temperature/gravity modulation. Transp. Porous Media 91, 585–604 (2012b)

  • Siddheshwar, P.G., Bhadauria, B.S., Suthar, O.P.: Synchronous and asynchronous boundary temperature modulations of Bénard–Darcy convection. Int. J. Non-Linear Mech. 49, 84–89 (2013)

    Article  Google Scholar 

  • Straughan, B.: The energy method, stability, and nonlinear convection, Applied mathematical sciences series, 2nd edn. Springer, New York (2004)

  • Vadasz, P.: Coriolis effect on gravity-driven convection in a rotating porous layer heated from below. J. Fluid Mech. 376, 351–375 (1998)

    Article  Google Scholar 

  • Vadasz, P. (ed.) Emerging topics in heat and mass transfer in porous media. Springer, New York (2008)

  • Vafai, K. (ed.): Handbook of porous media. Marcel Dekker, New York (2000)

    Google Scholar 

  • Vafai K. (ed.), Handbook of porous media. Taylor and Francis (CRC), Boca Raton (2005)

  • Venezian, G.: Effect of modulation on the onset of thermal convection. J. Fluid Mech. 35, 243–254 (1969)

    Article  Google Scholar 

Download references

Acknowledgments

This work was done during the visit of the author B. S. Bhadauria (BSB) to the Universiti Kebangsaan Malaysia (UKM), in June, 2012, as Visiting Professor of Mathematics. The author BSB gratefully acknowledges the grant provided by UKM out of the University Research Fund OUP-2012-61. Further, the author BSB is also grateful to the Banaras Hindu University, Varanasi, for sanctioning the lien to work as Professor of Mathematics at Department of Applied Mathematics, BB Ambedkar University, Lucknow, India. The authors are grateful to the two reviewers for their comments on the papers that helped us refine the paper into the present revised form.

Author information

Authors and Affiliations

  1. Department of Applied Mathematics, School for Physical Sciences, Babasaheb Bhimrao Ambedkar University, Lucknow, 226 025, India

    B. S. Bhadauria

  2. School of Mathematical Sciences, Faculty of Science and Technology, Universiti Kebangsaan Malaysia, 43600 , Bangi, Selangor, Malaysia

    I. Hashim

  3. Department of Mathematics, Bangalore University, Central College Campus, Bangalore, 560 001, India

    P. G. Siddheshwar

Authors
  1. B. S. Bhadauria
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. I. Hashim
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. P. G. Siddheshwar
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to B. S. Bhadauria.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Bhadauria, B.S., Hashim, I. & Siddheshwar, P.G. Effects of Time-Periodic Thermal Boundary Conditions and Internal Heating on Heat Transport in a Porous Medium. Transp Porous Med 97, 185–200 (2013). https://doi.org/10.1007/s11242-012-0117-7

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11242-012-0117-7

Keywords

Search

Navigation