Long Term Evolution of Solar Meridional Circulation and Phase Synchronization Viewed Through a Symmetrical Kuramoto Model

Abstract

The solar-cycle oscillations of the toroidal and poloidal components of the solar magnetic field in the northern solar hemisphere have a persistent phase difference of about $\pi$. We propose a symmetrical Kuramoto model with three coupled oscillators as a simple way to understand this anti-synchronization. We solve an inverse problem and reconstruct natural frequencies of the top and bottom oscillators under the conditions of a constant coupling strength and a non-delayed coupling. These natural frequencies are associated with angular velocities of the meridional flow circulation near the solar surface and in the deep layer of the solar convection zone. A relationship between our reconstructions of the shallow and the deep meridional flow speed during recent Solar Cycles 21 – 23 is in agreement with estimates obtained in helioseismology and flux-transport dynamo modeling. The reconstructed top oscillator speed presents significant solar-cycle like variations that agree with recent helioseismical reconstructions. The evolution of reconstructed natural frequencies strongly depends on the coupling strength. We find two stable regimes in the case of strong coupling with a change of regime during anomalous solar cycles. We see the onset of a new transition in Solar Cycle 24. We estimate the admitted range of coupling values and find evidence of cross-equatorial coupling between solar hemispheres not accounted for by the model.

Appendix

In this section, we investigate the reconstruction of the natural frequencies $\omega _{i} ( t )$ with respect to the initial value of the middle oscillator phase $\theta _{2} ( t_{0} )$ used in the inverse problem solution. We perform the reconstruction from the evolution of synchronized phases $\theta _{1} ( t )$ and $\theta _{3} ( t )$ generated by the Kuramoto model (see Equation 4) and consider the middle oscillator phase $\theta _{2} ( t )$ as nonobservable. The reconstruction is unique if we get the same values of reconstructed natural frequencies for all given initial values $\theta _{2} ( t_{0} )$. If the reconstructed natural frequencies depend on $\theta _{2} ( t_{0} )$, the reconstruction is not unique.

1.1 A.1 Constant Natural Frequencies

Let us start with phases $\theta _{1} ( t ) = \Omega t + \Phi _{1} ( t )$ and $\theta _{3} ( t ) = \Omega t + \Phi _{3} ( t )$ generated by Equation 4 with constant frequencies $\omega _{i}$ and strong coupling $\kappa$. The three oscillators will be synchronized and the phase difference $\theta = \theta _{1} - \theta _{3} = \Phi _{1} - \Phi _{3}$ will be constant. Let us consider a reconstruction for a constant coupling $\hat{\kappa }$. The phase of the middle oscillator $\Phi _{2} ( t )$ may then be determined from the middle equation in 15,

$$ \dot{\Phi }_{2} = \hat{\omega }_{2} - \Omega + 2\hat{\kappa } \sin \biggl( \frac{\Phi _{1} + \Phi _{3}}{2} - \Phi _{2} \biggr) \cos \biggl( \frac{\Phi _{3} - \Phi _{1}}{2} \biggr) . $$

(20)

When $\hat{\omega }_{2} = \Omega$ the solution of the differential equation 20 is

$$ \Phi _{2} ( t ) = \frac{\Phi _{1} + \Phi _{3}}{2} - 2\arctan \biggl( \exp \biggl[ - 2\hat{\kappa } \cos \biggl( \frac{\theta }{2} \biggr) ( t - c ) \biggr] \biggr) , $$

(21)

where the constant $c$ is determined from the initial value $\Phi _{2} ( t_{0} )$. It is clear that when $t \gg c$ the phase $\Phi _{2} ( t )$ is not influenced by the choice of $\Phi _{2} ( t_{0} )$.

When $\hat{\omega }_{2} = \mathrm{const} \ne \Omega$ and the coupling $\kappa$ is strong enough to provide the solution of the differential Equation 20 it may be expressed using the equations

$$ \begin{aligned} \Phi _{2} ( t ) &= \frac{\Phi _{1} + \Phi _{3}}{2} - 2 \arctan \bigl( u ( t ) \bigr), \\ u ( t ) &= \frac{b}{a} + \sqrt{ \biggl( \frac{b}{a} \biggr) ^{2} - 1} \biggl( \frac{1 + \exp ( \sqrt{b^{2} - a^{2}} [ t - c ] ) }{1 - \exp ( \sqrt{b^{2} - a^{2}} [ t - c ] ) } \biggr), \end{aligned} $$

(22)

where $a = \hat{\omega }_{2} - \Omega$, $b = 2\hat{\kappa } \cos ( \theta / 2 )$, $c$ is a constant determined from the initial value $\Phi _{2} ( t_{0} )$. It is clear that when $t \gg c$ this solution becomes independent from the initial value $\Phi _{2} ( t_{0} )$.

Consequently, in the case of constant $\omega _{2}$ the reconstructed values of the natural frequencies (see Equation 16 of the main text) do not depend on the initial value $\Phi _{2} ( t_{0} )$, and we get a unique reconstruction.

1.2 A.2 Variable Natural Frequencies

When $\hat{\omega }_{2} = \omega _{2} = \mathrm{const}$ and $\omega _{1} + \omega _{2} + \omega _{3} = 3\Omega$, the reconstruction of the natural frequencies $\hat{\omega }_{1}$ and $\hat{\omega }_{3}$ from synchronized phases $\theta _{1} ( t ) = \Omega t + \Phi _{1} ( t )$ and $\theta _{3} ( t ) = \Omega t + \Phi _{3} ( t )$ is unique, even if original frequencies $\omega _{1}$ and $\omega _{3}$ present a slow long-term variation. Figure 8 presents an example of a constant frequency of the middle oscillator $\omega _{2} = \Omega$ and slowly oscillating natural frequencies of the top and bottom oscillators:

$$ \begin{aligned} \omega _{1} ( t ) &= \Omega + \varepsilon \sin ( \Gamma t ), \\ \omega _{3} ( t ) &= \Omega - \varepsilon \sin ( \Gamma t ). \end{aligned} $$

(23)

When coupling $\hat{\kappa }$ used for the reconstruction through Equation 16 is less than the original coupling $\kappa$, the reconstructed frequencies have the same temporal variations as the original ones but with smaller amplitude (Figure 8a, solid lines). When $\hat{\kappa } > \kappa$ the amplitudes of $\hat{\omega }_{1}$ and $\hat{\omega }_{3}$ are larger than that of $\omega _{1}$ and $\omega _{3}$. If the coupling $\hat{\kappa }$ is too weak to provide synchronization, then the reconstruction of the natural frequencies is not successful (Figure 8(b)).

Figure 8

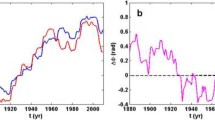
Reconstruction of the natural frequencies of the top (blue) and bottom (red) oscillators. Dashed curves represent the evolution of the original natural frequencies. (a) Influence of coupling for $\hat{\omega }_{2} = \Omega$: $\hat{\kappa } = 0.25 < \kappa = 0.5$ (solid line), $\hat{\kappa } = 1 > \kappa = 0.5$ (dotted line). (b) Reconstruction for the weak coupling $\hat{\kappa } = 0.05$.

We characterize the quality of the natural frequency reconstruction with the normalized deviation $D ( \hat{\omega }_{i},\omega _{i} )$ determined as $L_{2}$, the distance between $\hat{\omega }_{i}$ and $\omega _{i}$ normalized to the dispersion of $\omega _{i}$:

$$ D ( \omega _{i},\hat{\omega }_{i} ) = \frac{\int _{t_{0}} ^{t_{0} + T} ( \omega _{i} ( t ) - \hat{\omega }_{i} ( t ) ) ^{2}\,\mathrm{d}t}{\int _{t_{0}}^{t_{0} + T} ( \omega _{i} ( t ) - \langle \omega _{i} ( t ) \rangle ) ^{2}\,\mathrm{d}t}. $$

(24)

A unique reconstruction exists for any constant value $\hat{\omega } _{2}$ and strong coupling $\hat{\kappa }$, and it is the best ($D ( \hat{\omega }_{1},\omega _{1} )$ and $D ( \hat{\omega }_{3},\omega _{3} )$ are minimal) when $\hat{\omega }_{2} = \omega _{2}$ and $\hat{\kappa } = \kappa$. Assuming that $\hat{\omega }_{2}$ is not constant we get a non-unique solution, which depends on the initial value $\Phi _{2} ( t_{0} )$, because the solutions given by Equations 21 and 22 will not be valid. Although we cannot properly reconstruct the variable frequency of the middle oscillator $\omega _{2} ( t )$, we can get a unique solution with $\hat{\omega }_{2} = \mathrm{const}$ which is the best when it is equal to the mean value of the original natural frequency $\hat{\omega }_{2} = \langle \omega _{2} ( t ) \rangle$. Figure 9 presents the reconstruction for

$$ \begin{aligned} \omega _{1} ( t ) &= \Omega + a_{1} + \varepsilon \sin ( \Gamma t ) \\ \omega _{2} ( t ) & = \Omega - a_{1} - a_{3} - \varepsilon \bigl( \sin ( \Gamma t ) - \sin ( \Gamma t + \Psi ) \bigr) \\ \omega _{3} ( t ) &= \Omega + a_{3} - \varepsilon \sin ( \Gamma t + \Psi ) \end{aligned} $$

(25)

Figure 9

Evolution of the natural frequencies (a), their reconstruction with variable (b), and constant (c) middle oscillator frequency $\omega _{2}$, and the quality (d) $D ( \omega ,\hat{\omega } )$ of the reconstruction performed for constant $\omega _{2}$ of the top (blue), middle (yellow), and bottom (red) oscillators. The parameters of the modulation of the natural frequencies (see text and Equation 25) are $\varepsilon = 0.15$, $\Gamma = \Omega /4$, $\Omega = 2\pi /10.75y^{ - 1}$, $a_{1} = 0.1$; $a_{3} = 0.3$, $\kappa = 0.5$, $\Psi = \pi / 4$.