Optical solitons with Kudryashov’s quintuple power–law coupled with dual form of non–local law of refractive index with extended Jacobi’s elliptic function

Abstract

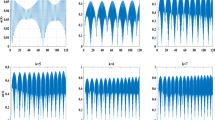
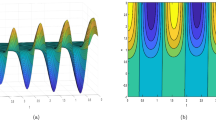
The extended Jacobi’s elliptic function scheme is implemented in this paper to recover a wide range of optical solitons for nonlinear Schrödinger’s equation that comes with two forms of self–phase modulation effect. They are Kudryashov’s quintuple power law together with dual–form of non–local nonlinearity. The extended Jacobi’s elliptic function approach reveals bright, dark and singular soliton solutions along dark–singular form of straddled solitons.

Appendices

Appendix A

$$\begin{aligned} \begin{array}{c} b_1= \displaystyle \frac{a \alpha _1^2 (m-1)}{m^2} , \quad b_3= \displaystyle 4 b_6 (k+1) -12 b_7 \alpha _1^2 -\frac{a k (m+1)}{m^2\alpha _1^2 } , \quad b_4= \displaystyle 16 b_7 (k+1)-\frac{6 b_6 k}{\alpha _1^2} , \quad b_5= \displaystyle -\frac{20 b_7 k}{\alpha _1^2} \end{array} \end{aligned}$$

(50)

$$\begin{aligned} \begin{array}{c} b_1= \displaystyle \frac{a k \beta _1^2 (m-1)}{m^2} , \quad b_3= \displaystyle 4 b_6 (k+1) -12 b_7 k \beta _1^2 -\frac{a (m+1)}{m^2 \beta _1^2 } , \quad b_4= \displaystyle 16 b_7 (k+1)-\frac{6 b_6}{\beta _1^2} , \quad b_5= \displaystyle -\frac{20 b_7}{\beta _1^2} \end{array} \end{aligned}$$

(51)

$$\begin{aligned} \begin{array}{c} b_1= \displaystyle \frac{4 a \beta _1^2 \sqrt{k}\left( \sqrt{k}+1\right) ^2 (m-1)}{m^2} , \quad b_3= \displaystyle 4 b_6 \left( k+6\sqrt{k}+1\right) -48 b_7 \beta _1^2 \sqrt{k} \left( \sqrt{k}+1\right) ^2 -\frac{a (m+1)}{m^2\beta _1^2 }, \\ \\ b_4= \displaystyle 16 b_7 \left( k+6 \sqrt{k}+1\right) -\frac{6 b_6}{\beta _1^2} , \quad b_5= \displaystyle -\frac{20 b_7}{\beta _1^2} . \end{array} \end{aligned}$$

(52)

Appendix B

$$\begin{aligned} \begin{array}{c} b_1= \displaystyle -\frac{a \alpha _1^2 (k-1) (m-1)}{m^2} , \quad b_3= \displaystyle 4b_6 (1-2 k) +12 b_7\alpha _1^2 (k-1) + \frac{a k (m+1)}{m^2\alpha _1^2 } , \\ \\ b_4= \displaystyle 16 b_7 (1- 2 k) + \frac{6 b_6 k}{\alpha _1^2} , \quad b_5= \displaystyle \frac{20 b_7 k}{\alpha _1^2} \end{array} \end{aligned}$$

(53)

$$\begin{aligned} \begin{array}{c} b_1= \displaystyle -\frac{a k \beta _1^2 (m-1)}{m^2} , \quad b_3= \displaystyle 4b_6 (1-2 k) +12 b_7 k \beta _1^2 + \frac{a (k-1) (m+1)}{m^2 \beta _1^2 } , \\ \\ b_4= \displaystyle 16 b_7 (1- 2 k) + \frac{6 b_6 (k-1)}{\beta _1^2} , \quad b_5= \displaystyle \frac{20 b_7 (k-1)}{\beta _1^2} \end{array} \end{aligned}$$

(54)

$$\begin{aligned} \begin{array}{c} b_1= \displaystyle -\frac{4 a k_1 \alpha _1^2 (m-1)}{\sqrt{k} m^2} , \quad b_3= \displaystyle 4 \left( b_6 k_2-12 b_7 k_3 \alpha _1^2\right) + \frac{a k (m+1)}{m^2 \alpha _1^2} , \quad b_4= \displaystyle 16 b_7 k_2 + \frac{6 b_6 k}{\alpha _1^2} , \quad b_5= \displaystyle \frac{20 b_7 k}{\alpha _1^2} . \end{array} \end{aligned}$$

(55)

Here, the abbreviations $k_1$, $k_2$ and $k_3$ are given by

$$\begin{aligned} k_1= 2 \sqrt{k} \left( k+\sqrt{k(k-1)}-1\right) -\sqrt{k-1} , \quad k_2= 1 -2 k-6 \sqrt{k(k-1)} , \quad k_3= 2 -2 \left( k+\sqrt{k(k-1)} \right) +\sqrt{\frac{k-1}{k}} . \end{aligned}$$

(56)

Appendix C

$$\begin{aligned} \begin{array}{c} b_1= \displaystyle \frac{a \alpha _1^2 (k-1) (m-1)}{m^2} , \quad b_3= \displaystyle 4 b_6 (k-2) -12 b_7 \alpha _1^2 (k-1) + \frac{a (m+1)}{m^2\alpha _1^2 } , \quad b_4= \displaystyle 16 b_7 (k-2) + \frac{6 b_6}{\alpha _1^2} , \quad b_5= \displaystyle \frac{20 b_7}{\alpha _1^2} \end{array} \end{aligned}$$

(57)

$$\begin{aligned} \begin{array}{c} b_1= \displaystyle -\frac{a \beta _1^2 (m-1)}{m^2} , \quad b_3= \displaystyle 4 b_6 (k-2) +12 b_7 \beta _1^2 -\frac{a (k-1) (m+1)}{m^2 \beta _1^2 } , \\ \\ b_4= \displaystyle 16 b_7 (k-2)-\frac{6 b_6 (k-1)}{\beta _1^2} , \quad b_5= \displaystyle -\frac{20 b_7 (k-1)}{\beta _1^2} \end{array} \end{aligned}$$

(58)

$$\begin{aligned} \begin{array}{c} b_1= \displaystyle \frac{4 a k_4 \alpha _1^2 (m-1)}{m^2} , \quad b_3= \displaystyle 4 \left( b_6 k_5-12 b_7 k_4\alpha _1^2\right) + \frac{a (m+1)}{m^2\alpha _1^2} , \quad b_4= \displaystyle 16 b_7 k_5 + \frac{6 b_6}{\alpha _1^2} , \quad b_5= \displaystyle \frac{20 b_7}{\alpha _1^2} . \end{array} \end{aligned}$$

(59)

Here, the abbreviations $k_4$ and $k_5$ are indicated as follows:

$$\begin{aligned} k_4 = k \left( 2 + \sqrt{1-k}\right) -2 \left( 1 + \sqrt{1-k}\right) , \quad k_5 = k-2-6 \sqrt{1-k} . \end{aligned}$$

(60)

Appendix D

$$\begin{aligned} \begin{array}{c} b_1= \displaystyle -\frac{a \alpha _1^2 (k-1) (m-1)}{m^2} , \quad b_3= \displaystyle 4 b_6 (k-2) +12 b_7 \alpha _1^2 (k-1) -\frac{a (m+1)}{ m^2\alpha _1^2} , \quad b_4= \displaystyle 16 b_7 (k-2)-\frac{6 b_6}{\alpha _1^2} , \quad b_5= \displaystyle -\frac{20 b_7}{\alpha _1^2} \end{array} \end{aligned}$$

(61)

$$\begin{aligned} \begin{array}{c} b_1= \displaystyle \frac{a \beta _1^2 (m-1)}{m^2} , \quad b_3= \displaystyle 4 b_6 (k-2) -12 b_7 \beta _1^2 + \frac{a (k-1) (m+1)}{m^2 \beta _1^2 } , \\ \\ b_4= \displaystyle 16 b_7 (k-2) + \frac{6 b_6 (k-1)}{\beta _1^2} , \quad b_5= \displaystyle \frac{20 b_7 (k-1)}{\beta _1^2} \end{array} \end{aligned}$$

(62)

$$\begin{aligned} \begin{array}{c} b_1= \displaystyle \frac{4 a k_6 \alpha _1^2 (m-1)}{m^2} , \quad b_3= \displaystyle 4 \left( b_6 k_7-12 b_7 k_6 \alpha _1^2 \right) -\frac{a (m+1)}{m^2\alpha _1^2} , \quad b_4= \displaystyle 16 b_7 k_7-\frac{6 b_6}{\alpha _1^2} , \quad b_5= \displaystyle -\frac{20 b_7}{\alpha _1^2} . \end{array} \end{aligned}$$

(63)

Here, the abbreviations $k_6$ and $k_7$ are denoted as

$$\begin{aligned} k_6 = 2 + k \left( \sqrt{1-k}-2\right) -2 \sqrt{1-k} , \quad k_7 = k-2+6 \sqrt{1-k} . \end{aligned}$$

(64)

Appendix E

$$\begin{aligned} \begin{array}{c} b_1= \displaystyle \frac{a \alpha _1^2 k (k-1) (m-1)}{m^2} , \quad b_3= \displaystyle 4 b_6 (1-2 k) -12 b_7 \alpha _1^2 k (k-1) -\frac{a (m+1)}{m^2\alpha _1^2 } , \\ \\ b_4= \displaystyle 16 b_7 (1-2 k)-\frac{6 b_6}{\alpha _1^2} , \quad b_5= \displaystyle -\frac{20 b_7}{\alpha _1^2} \end{array} \end{aligned}$$

(65)

$$\begin{aligned} \begin{array}{c} b_1= \displaystyle \frac{a \beta _1^2 (m-1)}{m^2} , \quad b_3= \displaystyle 4b_6 (1-2 k) -12 b_7 \beta _1^2 -\frac{a k (k-1) (m+1)}{m^2\beta _1^2 } , \\ \\ b_4= \displaystyle 16b_7 (1- 2 k)-\frac{6 b_6 k (k-1) }{\beta _1^2} , \quad b_5= \displaystyle -\frac{20 b_7 k (k-1) }{\beta _1^2} \end{array} \end{aligned}$$

(66)

$$\begin{aligned} \begin{array}{c} b_1= \displaystyle -\frac{4 a k_8 \alpha _1^2 (m-1)}{m^2} , \quad b_3= \displaystyle 4 \left( b_6 k_9 + 12 b_7 k_8\alpha _1^2\right) -\frac{a (m+1)}{m^2\alpha _1^2} , \quad b_4= \displaystyle 16 b_7 k_9-\frac{6 b_6}{\alpha _1^2} , \quad b_5= \displaystyle -\frac{20 b_7}{\alpha _1^2} . \end{array} \end{aligned}$$

(67)

Here, the following abbreviations $k_8$ and $k_9$ are used:

$$\begin{aligned} k_8 = 2 k \left( 1-k+\sqrt{k(k-1) }\right) -\sqrt{k(k-1) } , \quad k_9 = 1 -2 k+6 \sqrt{ k(k-1)} . \end{aligned}$$

(68)