Skip to main content

Advertisement

SpringerLink
Log in

Novel analytical and numerical techniques for fractional temporal SEIR measles model

  • Original Paper
  • Published:
Numerical Algorithms Aims and scope Submit manuscript

Abstract

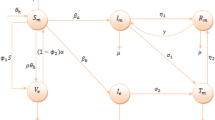
In this paper, a fractional temporal SEIR measles model is considered. The model consists of four coupled time fractional ordinary differential equations. The time-fractional derivative is defined in the Caputo sense. Firstly, we solve this model by solving an approximate model that linearizes the four time fractional ordinary differential equations (TFODE) at each time step. Secondly, we derive an analytical solution of the single TFODE. Then, we can obtain analytical solutions of the four coupled TFODE at each time step, respectively. Thirdly, a computationally effective fractional Predictor-Corrector method (FPCM) is proposed for simulating the single TFODE. And the error analysis for the fractional predictor-corrector method is also given. It can be shown that the fractional model provides an interesting technique to describe measles spreading dynamics. We conclude that the analytical and Predictor-Corrector schemes derived are easy to implement and can be extended to other fractional models. Fourthly, for demonstrating the accuracy of analytical solution for fractional decoupled measles model, we applied GMMP Scheme (Gorenflo-Mainardi-Moretti-Paradisi) to the original fractional equations. The comparison of the numerical simulations indicates that the solution of the decoupled and linearized system is close enough to the solution of the original system. And it also indicates that the linearizing technique is correct and effective.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Baleanu, D., Diethelm, K., Scalas, E., Trujillo, J.J.: Fractional Calculus: Models and Numerical Methods. World Scientific Publishing, Singapore (2012)

    Book  MATH  Google Scholar 

  2. Podlubny, I.: Fractional Differential Equations. Academic Press, New York (1999)

    MATH  Google Scholar 

  3. Liu, F., Anh, V., Turner, I.: Numerical Solution Of The space fractional Fokker-Planck equation. J. Comput. Appl. Math. 166(1), 209–219 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  4. Liu, F., Zhuang, P., Anh, V., Turner, I., Burrage, K.: Stability and Convergence of the difference Methods for the space-time fractional advection-diffusion equation. Appl. Math. Comput. 191, 12–20 (2007)

    MathSciNet  MATH  Google Scholar 

  5. Zheng, M., Liu, F., Turner, I., Anh, V.: A Novel High-Order Space-Time Spectral Method for the Time Fractional Fokker-Planck Equation. SIAM J. Sci. Comput. 37(2), A701–A724 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  6. Yu, Q., Liu, F., Turner, I., Burrage, K.: Numerical simulation of the fractional bloch equations. J. Comput. Appl. Math. 255, 635–651 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  7. Skwara, U., Martins, J., Ghaffari, P., Aguiar, M., Boto, J., Stollenwerk, N.: Fractional Calculus and Superdiffusion in Epidemiology: Shift of Critical Thresholds. In: Proceedings of the 12Th International Conference on Computational and Mathematical Methods in Science and Engineering, CMMSE 2012 La Manga, Spain (2012)

  8. Liu, F., Zhuang, P., Liu, Q.: Numerical Methods of Fractional Partial Differential Equations and Applications. Science Press, Beijing (2015)

    Google Scholar 

  9. Ross, B.: The development of fractional calculus 1695–1900. Hist. Math. 4(1), 75–89 (1977)

    Article  MathSciNet  MATH  Google Scholar 

  10. Oldham, K.B., Spanier, J.: The Fractional Calculus. Academic Press, New York (1974)

    MATH  Google Scholar 

  11. Chen, J., Liu, F., Burrage, K., Shen, S.: Numerical techniques for simulating a fractional model of epidermal wound healing. Appl. Math. Comput. 41, 33–47 (2012)

    MathSciNet  MATH  Google Scholar 

  12. Diethelm, K., Ford, N.J., Freed, A.D.: A predictor-corrector approach for the numerical solution of fractional differential equations. Nonlinear Dynam. 29(1), 3–22 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  13. Rihan, F.A., Baleanu, D., Lakshmanan, S., Rakkiyappan, R.: On fractional SIRC model with salmonella bacterial infection. Abstr. Appl. Anal. 2014, 136263 (2014)

    Article  MathSciNet  Google Scholar 

  14. Area, I., Batarfi, H., Losada, J., Nieto, J.J., Shammakh, W., Torres, A.: On a fractional order Ebola epidemic model. Adv. Difference Equ. 278, 1–12 (2015)

    MathSciNet  MATH  Google Scholar 

  15. Huo, J., Zhao, H., Zhu, L.: The effect of vaccines on backward bifurcation in fractional order HIV model. Nonlinear Anal. Real World Appl. 26, 289–305 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  16. Carvalho dos Santos, J.P., Cardoso, L.C., Monteiro, E., Lemes, N.H.T.: A fractional order epidemic model for Bovine Babesiosis Disease and Tick populations. Abstr. Appl. Anal. 729894, 2015 (2015)

    MathSciNet  Google Scholar 

  17. Diethelm, K.: A fractional calculus based model for the simulation of an outbreak of dengue fever. Nonlinear Dynam. 71(4), 613–619 (2013)

    Article  MathSciNet  Google Scholar 

  18. Pooseh, S., Rodrigues, H.S., Torres, D.F.M.: Fractional derivatives in dengue epidemics. AIP conf. Proc. 1389, 739–742 (2011)

    Article  Google Scholar 

  19. Erturk, V.S., Odibat, Z.M., Momani, S.: An approximate solution of a fractional order differential equation model of human T-cell lymphotropic virus i(HTLV-i) infection of CD4+ T-cells. Comput. Math. Appl. 62, 996C1002 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  20. Mpande, L.C., Kajunguri, D., Mpolya, E.A.: Modeling and stability analysis for measles metapopulation models with vaccination. Applied and Computational Mathematics 4(6), 431–444 (2015)

    Article  Google Scholar 

  21. Abubakar, S., Akinwande, N.I., Abdulrahman, S., Oguntolu, F.A.: Bifurcation analysis on the mathematical model of measles disease dynamics. Universal Journal of Applied Mathematics 1(4), 212–216 (2013)

    Google Scholar 

  22. Adewale, S.O., Mohammed, I.T., Olopade, I.A.: Mathematical analysis of effect of area on the dynamical spread of measles. IOSR Journal of Engineering 4(3), 43–57 (2014)

    Article  Google Scholar 

  23. Momoh, A.A., Ibrahim, M.O., Uwanta, I.J., Manga, S.B.: Mathematical model for control of measles epidemiology. International Journal of Pure and Applied Mathematics 87(5), 707–718 (2013)

    Article  Google Scholar 

  24. Ma, Z., Zhou, Y., Wu, J.: Modeling and Dynamics of Infectious Diseases: Series in Contemporary Applied Mathematics CAM 11. Higher Education Press, Beijing (2009)

    Book  Google Scholar 

  25. Doungmo, E.F., Oukouomi, S.C., Mugisha, S.: A fractional SEIR epidemic model for spatial and temporal spread of measles in populations. Abstr. Appl. Anal. 2014, 781028 (2014)

    Google Scholar 

  26. Lewis, J.: Warning Over Measles Outbreak, Otago Daily Times, http://www.odt.co.nz/news/dunedin/293581/warning-over-measles-outbreak (2014)

  27. MacIntyre, C.R., Gay, N.J., Gidding, H.F., Hull, B.P., Gilbert, G.L., MacIntyre, P.B.: A mathematical model to measure the impact of the measles control campaign on the potential for measles transmission in australia. Int. J. Infect. Dis. 6(4), 277–282 (2002)

    Article  Google Scholar 

  28. Roberts, M.G., Tobias, M.I.: Predicting and preventing measles epidemics in New Zealand. Epidemiol. Infect. 124, 279–287 (2000)

    Article  Google Scholar 

  29. Jiang, H., Liu, F., Turner, I., Burrage, K.: Analytical solutions for the multi-term time- fractional diffusion-wave/diffusion equations in a finite domain. Comput. Math. Appl. 64(10), 3377–3388 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  30. Jiang, H., Liu, F., Meerschaaert, M.M., McGough, R.: Fundamental solutions for the multi-term modified power law wave equations in a finite domain. Electron. J. Math. Anal. Appl. 1, 55–66 (2013)

    MATH  Google Scholar 

  31. Srivastava, V.K., Kumar, S., Awasthi, M.K., Singh, B.K.: Two-dimensional time fractional order biological populations model and its analytical solution. Egyptian Journal of Basic and Applied Sciences 1(1), 71–76 (2014)

    Article  Google Scholar 

  32. Petráš, I.: Systems Fractional-Order Nonlinear Modeling, Analysis and Simulation. Springer, Beijing (2010)

    MATH  Google Scholar 

  33. Liu, F., Zhuang, P., Turner, I., Anh, V., Burrage, K.: A semi-alternating direction method for a 2-D fractional FitzHugh-Nagumo monodomain model on an approximate irregular domain. J. Comp Physics 293, 252–263 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  34. Yang, Z., Yuan, Z., Nie, Y., Wang, J., Zhu, X., Liu, F.: Finite element method for nonlinear Riesz space fractional diffusion equations on irregular domains. J. Comp. Phys. (2016). https://doi.org/10.1016/j.jcp.2016.10.053

  35. Luchko, Y., Gorenflo, R.: An operational method for solving fractional differential equations with the Caputo derivatives. Acta. Math. Vietnam. 24(2), 207–233 (1999)

    MathSciNet  MATH  Google Scholar 

  36. Gorenflo, R., Mainardi, F., Moretti, D., Paradisi, P.: Time fractional diffusion: a discrete random walk approach. Nonlinear Dyn. 29, 129–143 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  37. Murillo, J.Q., Yuste, S.B.: On three explicit difference schemes for fractional diffusion and diffusion-wave equations. Phys. Scr. 014025, T136 (2009)

    Google Scholar 

  38. Hairer, E., Norsett, S.P., Wanner, G.: Solving Ordinary Differential Equations II-Stiff and Algebraic Problems. Springer, Switzerland (1993)

    MATH  Google Scholar 

  39. Yang, C., Liu, F.: A computationally effective predictor-corrector method for simulating fractional order dynamic control system. ANZIAM J. 47, C168–C184 (2006)

    Article  Google Scholar 

  40. Diethelm, K., Ford, N.J., Freed, A.D.: A detailed error analysis for a fractional Adams method. Numer. Algorithms 36, 31–52 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  41. Ochoche, J.M., Gweryina, R.I.: A mathematical model of measles with vaccination and two phases of infectiousness. IOSR Journal of Mathematics 10(1), 95–105 (2014)

    Article  Google Scholar 

  42. Salmani, M., Van den Driessche, P.: A model for disease transmission in a patchy environment. Discrete Contin. Dyn. Syst. Ser. B 6(1), 185–202 (2006)

    MathSciNet  MATH  Google Scholar 

  43. Kilbas, A.A., Srivastava, H.M., Trujillo, J.J.: Theory and Applications of Fractional Differential Equations. Elsevier, The Netherlands (2006)

    MATH  Google Scholar 

  44. Bonilla, B., Rivero, M., Trujillo, J.J.: On systems of linear fractional differential equations with constant coefficients. Appl. Math. Comput. 187(1), 68–78 (2007)

    MathSciNet  MATH  Google Scholar 

  45. Kermack, W.O., McKendrick, A.G.: Contributions to the mathematical theory of epidemics. Bull. Math. Biol. 53, 33–55 (1991)

    Google Scholar 

  46. Odibat, Z.M.: Analytic study on linear systems of fractional differential equations. Comput. Math. Appl. 59(3), 1171–1183 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  47. Gorenflo, R., Mainardi, F., Moretti, D., Paradisi, P.: Time fractional diffusion: a discrete random walk approach. Nonlinear Dyn. 29, 129–143 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  48. Yuste, S., Murillo, J.: On three explicit difference schemes for fractional diffusion and diffusion-wave equations. Phys. Scr. T136, 14–25 (2009)

    Google Scholar 

Download references

Acknowledgments

This research is partially supported by the National Natural Science Foundation of China under grant 11772046. Abdullah would like to acknowledge the financial support from Fundamental Research Grant Scheme (FRGS 203/PMATHS/6711570) by Ministry of Higher Education, Malaysia, RCMO Universiti Sains Malaysia and School of Mathematical Sciences, Universiti Sains Malaysia, Penang Malaysia. Abdullah F. A. also wish to thank School of Mathematical Sciences, Queensland University of Technology, QLD Australia for providing computing facilities. We would like to thank the referees for their careful reading of the paper and many constructive comments and suggestions.

Author information

Authors and Affiliations

  1. School of Mathematical Sciences, Universiti Sains Malaysia, 11800 USM, Pulau Pinang, Malaysia

    F. A. Abdullah

  2. School of Mathematical Sciences, Queensland University of Technology, Brisbane, QLD, 4001, Australia

    F. Liu & P. Burrage

  3. ACEMS ARC Centre of Excellence, Queensland University of Technology, Brisbane, QLD, 4001, Australia

    K. Burrage

  4. School of Mathematics and Statistics, Sichuan university of Science and Engineering, Zigong, 643000, China

    T. Li

Authors
  1. F. A. Abdullah
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. F. Liu
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. P. Burrage
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. K. Burrage
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. T. Li
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to F. Liu.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Abdullah, F.A., Liu, F., Burrage, P. et al. Novel analytical and numerical techniques for fractional temporal SEIR measles model. Numer Algor 79, 19–40 (2018). https://doi.org/10.1007/s11075-017-0426-6

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11075-017-0426-6

Keywords

Mathematics Subject Classification (2010)

Search

Navigation