N-fold generalized Darboux transformation and breather–rogue waves on the constant/periodic background for a generalized mixed nonlinear Schrödinger equation

Abstract

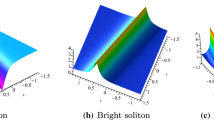
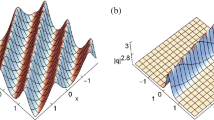
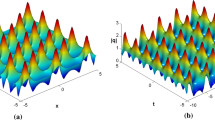
In this paper, a generalized mixed nonlinear Schrödinger equation, which arises in several physical applications including fluid mechanics (for the weakly nonlinear dispersive water waves), quantum field theory and nonlinear optics, is investigated. An N-fold generalized Darboux transformation (GDT) is constructed, where N is a positive integer. Based on that N-fold GDT, we derive the higher-order rational soliton solutions with the non-vanishing background. Semirational solutions on the constant/periodic background, which are composed of the mth-order rogue wave, the $(k-m-r)$th-order so-called nondegenerate breather and the rth-order so-called degenerate breather, are constructed, where $k=2,3,\ldots ,N$, $m=1,2,\ldots ,k-1$ and $r=0,2,3,\ldots$. Breathers on the dark/bright soliton or periodic wave background are presented. Based on the semirational solutions, breather–rogue waves on the constant/periodic background are discussed analytically and graphically. Classification conditions for different types of the breather–rogue waves on the constant/periodic background are given. Triangular structure of the higher-order rogue wave on the periodic background is studied and presented.

Appendix

Lax pair corresponding to Eq. (1) has been constructed as [9, 11]

$$\begin{aligned} \begin{aligned}&\Phi _x=(\lambda ^2U_2+\lambda U_1+U_0)\Phi =U\Phi ,\\&\Phi _t=V\Phi , \end{aligned} \end{aligned}$$

(A.1)

where $\Phi$ is the eigenfunction corresponding to the complex spectral parameter $\lambda$,

$$\begin{aligned}&\Phi =\begin{pmatrix} \phi (x,t,\lambda ) \\ \varphi (x,t,\lambda ) \end{pmatrix},~~~ V=\begin{pmatrix} v_1 &{} v_2 \\ v_3 &{} -v_1 \\ \end{pmatrix},~~~\\&U_2=\begin{pmatrix} -\frac{i}{a-b} &{} 0 \\ 0 &{} \frac{i}{a-b} \\ \end{pmatrix},~~~ U_1=\begin{pmatrix} 0 &{} -u^* \\ u &{} 0 \\ \end{pmatrix},~~~\\&U_0=\begin{pmatrix} -\frac{id}{2(a-b)}+(\frac{ia}{4}-\frac{ib}{2})|u|^2 &{} 0 \\ 0 &{} \frac{id}{2(a-b)}-(\frac{ia}{4}-\frac{ib}{2})|u|^2 \\ \end{pmatrix},~~~\\&v_1=-\frac{2i}{(a-b)^2}\lambda ^4-\left[ \frac{2id}{(a-b)^2}-i|u|^2\right] \lambda ^2 \\&\qquad -\frac{id^2}{2(a-b)^2}\\&\qquad -\frac{i}{8}(a^2-ab-2b^2)|u|^4-\frac{a-2b}{4}(-u^*u_x+uu^*_x),\\&v_2=-\frac{2}{a-b}u^*\lambda ^3+\left( -\frac{d}{a-b}u^*+\frac{a}{2}|u|^2u^*-iu^*_x\right) \lambda ,\\&v_3=\frac{2}{a-b}u\lambda ^3+\left( \frac{d}{a-b}u-\frac{a}{2}|u|^2u-iu_x\right) \lambda , \end{aligned}$$

$\phi (x,t,\lambda )$ and $\varphi (x,t,\lambda )$ are the functions of x, t and $\lambda$, and $a\ne b$. It can be verified that the compatibility condition $U_t-V_x+UV-VU=0$ leads to Eq. (1).

The Nth-order analytic solutions in terms of the determinant expression for Eq. (1) have been given as [11]

$$\begin{aligned} \begin{aligned}&u[N]=\frac{1}{\rho _N^2}\left( u-\frac{2i}{a-b}\frac{\text {Det}(\delta _{11})}{\text {Det}(\delta _{12})}\right) , \end{aligned} \end{aligned}$$

(A.2)

where the sign “[N]” means that the relevant solutions are the Nth-order analytic solutions, $\delta _{11}=(A_1,A_2,\ldots ,A_N)^{\mathrm{T}}$, $\delta _{12}=(B_1,B_2,\ldots ,B_N)^{\mathrm{T}}$,

$$\begin{aligned}&A_k=(\varphi _k,\lambda _k\phi _k,\ldots ,\lambda _k^{N-3}\phi _k,\lambda _k^{N-2}\varphi _k,-\lambda _k^N\varphi _k)^{\mathrm{T}},\nonumber \\&B_k=(\varphi _k,\lambda _k\phi _k,\ldots ,\lambda _k^{N-3}\phi _k,\lambda _k^{N-2}\varphi _k,\lambda _k^{N-1}\phi _k)^{\mathrm{T}},\nonumber \\ \end{aligned}$$

(A.3)

for $N=2m$; $\delta _{11}=(C_1,C_2,\ldots ,C_N)^{\mathrm{T}}$, $\delta _{12}=(D_1,D_2,\ldots ,D_N)^{\mathrm{T}}$,

$$\begin{aligned}&C_k=(\phi _k,\lambda _k\varphi _k,\ldots ,\lambda _k^{N-3}\phi _k,\lambda _k^{N-2}\varphi _k,-\lambda _k^N\varphi _k)^{\mathrm{T}},\nonumber \\&D_k=(\phi _k,\lambda _k\varphi _k,\ldots ,\lambda _k^{N-3}\phi _k,\lambda _k^{N-2}\varphi _k,\lambda _k^{N-1}\phi _k)^{\mathrm{T}},\nonumber \\ \end{aligned}$$

(A.4)

for $N=2m+1$, m is a nonnegative integer and $k=1,2,\ldots ,N$. Additionally, similar to the results in Refs. [10, 11], it can check that $\rho _N$ has the form of

$$\begin{aligned} \begin{aligned}&\rho _N=\exp [i\varpi (x,t)], \end{aligned} \end{aligned}$$

(A.5)

where $\varpi (x,t)$ is a real function. Therefore, we have

$$\begin{aligned} \begin{aligned}&|\rho _N|=1\Longleftrightarrow \rho _N^*=\rho _N^{-1}. \end{aligned} \end{aligned}$$

This indicates that $\rho _N$ does not contribute to the calculation of $|u[N]|^2$.

Eigenvalues and eigenfunctions for Lax Pair (A.1) have to admit the symmetry conditions as

$(1)\lambda _k=-\lambda _k^*$, and $\phi _k^*(x,t,\lambda _k)=\varphi _k(x,t,\lambda _k)$;

$(2)\lambda _{2k}{=}\lambda _{2k{-}1}^*$, and $\phi _{2k}(x,t,\lambda _{2k}){=}{-}\varphi _{2k{-}1}^*(x,t,\lambda _{2k{-}1})$, $\varphi _{2k}(x,t,\lambda _{2k}){=}\phi _{2k-1}^*(x,t,\lambda _{2k-1})$,

where $\lambda _k$’s are the complex spectral parameters, $\phi _k(x,t,\lambda _k)$ and $\varphi _k(x,t,\lambda _k)$ are the functions of x, t and $\lambda _k$,

$$\begin{aligned} \begin{aligned}&\Phi _k(x,t,\lambda _k)=\begin{pmatrix} \phi _k(x,t,\lambda _k) \\ \varphi _k(x,t,\lambda _k) \end{pmatrix}~ \end{aligned} \end{aligned}$$

is the vector eigenfunction which solves Lax Pair (A.1) with $\lambda =\lambda _k$.