Secure communication on fractional-order chaotic systems via adaptive sliding mode control with teaching–learning–feedback-based optimization

Abstract

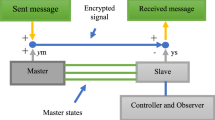
Due to the significance of secure communication, we do a research about this problem based on fractional-order chaotic systems, where a communication scheme is presented for encryption and decryption of the signal. Through applying Lyapunov stability theory and property of fractional calculus, an adaptive sliding mode controller is designed to achieve the synchronization phase between encryption system with encryption source and decryption system, which offers a tool to the decryption process. To improve the precision and speed of the decryption, we further put forward an optimization strategy for some parameters of the developed controller based on root mean square error of certain variable as a performance indicator. Meanwhile, as an improved teaching–learning-based optimization algorithm, teaching–learning–feedback-based optimization (TLFBO) algorithm is proposed to optimize the parameters more excellently. Subsequently, the simulation experiments, which contain performance test for TLFBO algorithm and secure communication of the signal, are, respectively, conducted on the benchmark functions as well as fractional-order Lorenz system with encryption source and fractional-order Lü system. At last, the experiment results illustrate the feasibility and practicability of the provided method by comparing with some other ones.

Appendix

Lemma 2

Letting $a_{i}\in \mathbb {R}$ for $i=1,2,\ldots ,n$, $r\in [0,\infty )$, there exists the inequality described as follows

$$\begin{aligned} \sum \limits _{i=1}^{n}|a_{i}|^{r}\ge \left( \sum \limits _{i=1}^{n}a_{i}^{2}/n^{2}\right) ^{\frac{r}{2}} \end{aligned}$$

(51)

Particularly, another inequality holds in the following

$$\begin{aligned} \sum \limits _{i=1}^{n}|a_{i}|\ge \sqrt{\sum \limits _{i=1}^{n}a_{i}^{2}/n^{2}} \end{aligned}$$

(52)

Proof

It is known that there is the following inequality meeting the condition of this lemma

$$\begin{aligned} \left( \sum \limits _{i=1}^{n}|a_{i}|\right) ^{r} \le \bigl (n\underset{i=1,2,\ldots ,n}{\mathrm {max}}\{|a_{i}|\}\bigr )^{r} \le n^{r}\sum \limits _{i=1}^{n}|a_{i}|^{r} \end{aligned}$$

(53)

So we have

$$\begin{aligned} \sum \limits _{i=1}^{n}|a_{i}|^{r}\ge \left( \sum \limits _{i=1}^{n}|a_{i}|\right) ^{r}/n^{r} \end{aligned}$$

(54)

Since $\bigl (\sum \nolimits _{i=1}^{n}|a_{i}|\bigr )^{2}\ge \sum \nolimits _{i=1}^{n}a_{i}^{2}$, (51) can be obtained according to (54). For (51), let $r=1$, then (52) would be derived. Thus, the proof of this lemma is complete. $\square$

Theorem 4

Based on the conditions in Sect. 3.1 and Remark 5, in addition to corresponding adjustments for (12) and (13), the rest of this theorem is the same as that in Theorem 1, in which the sliding mode surface is set as

$$\begin{aligned} \varvec{s}(t)=\varvec{e}(t)-\int _{t_{0}}^{t}\varvec{K}\varDelta (\mathrm {sign} (\varvec{e}(\tau )),|\varvec{e}(\tau )|^{\mu })\, \mathrm{d}\tau \end{aligned}$$

(55)

where $\mu >0$, $|\varvec{e}(\tau )|^{\mu }\triangleq [|e_{1}(\tau )|^{\mu },|e_{2}(\tau )|^{\mu },\ldots ,|e_{n+1}(\tau )|^{\mu }]^{T}$, $\mathrm {sign}(\varvec{e}(\tau ))\triangleq [\mathrm {sign}(e_{1}(\tau )),\mathrm {sign}(e_{2}(\tau )),\ldots ,\mathrm {sign}(e_{n+1}(\tau ))]^{T}$, $\Delta (\mathrm {sign}(\varvec{e}(\tau )),|\varvec{e}(\tau )|^{\mu })\triangleq \mathrm {diag}\{\mathrm {sign}(\varvec{e}(\tau ))\}\cdot |\varvec{e}(\tau )|^{\mu }$. Besides, the controller is developed as

$$\begin{aligned} \varvec{u}(t)&=(\varvec{H}^{T}\varvec{H})^{-1}\varvec{H}^{T}J^{\varvec{1}-\varvec{q}}\{D^{\varvec{1}-\breve{\varvec{p}}}\breve{\varvec{f}}(\breve{\varvec{x}}(t-\tau ))\nonumber \\&\quad -\varvec{H}D^{\varvec{1}-\varvec{q}}\varvec{g}(\varvec{y}(t))+D^{\varvec{1}-\breve{\varvec{p}}}[\breve{\varvec{F}}(\breve{\varvec{x}}(t-\tau ))\widetilde{\varvec{\alpha }}(t-\tau )]\nonumber \\&\quad -\varvec{H}D^{\varvec{1}-\varvec{q}}[\varvec{G}(\varvec{y}(t))\widetilde{\varvec{\beta }}(t)]+\varvec{K}{\Delta }(\mathrm {sign}(\varvec{e}(t)),|\varvec{e}(t)|^{\mu })\nonumber \\&\quad -(\widetilde{\varvec{D}}(t)+\varvec{C})\mathrm {sign}(\varvec{s}(t))-[\widetilde{\varvec{M}}(t)+\sigma (\Vert \widetilde{\varvec{A}}(t-\tau )\Vert \nonumber \\&\quad +\overline{A}+\Vert \widetilde{\varvec{B}}(t)\Vert +\overline{B}+\sqrt{2\sigma _{1}}\Vert \widetilde{\varvec{d}}(t)\Vert +\sqrt{2\sigma _{1}}\overline{d}\nonumber \\&\quad +\sqrt{2\sigma _{2}}\Vert \widetilde{\varvec{m}}(t)\Vert +\sqrt{2\sigma _{2}}\overline{m})/\Vert \varvec{s}(t)\Vert ^{2}]\varvec{s}(t)\}+\varvec{u}_{0} \end{aligned}$$

(56)

where $\varvec{C}=\mathrm {diag}\{c_{1},c_{2},\ldots ,c_{n+1}\}>0$, $0<\sigma \le c_{\text {min}}=\mathrm {min}_{i=1,2,\ldots ,n+1}\{c_{i}\}$, $\sigma _{i}\in \mathbb {R}^{+}$ for $i=1,2$, $\Vert \cdot \Vert$ represents Euclidean norm. Besides, $\overline{A}=\mathrm {sup}_{t\ge t_{0}+\tau }\{\Vert \varvec{A}(t-\tau )\Vert \}$, $\overline{B}=\mathrm {sup}_{t\ge t_{0}}\{\Vert \varvec{B}(t)\Vert \}$, $\overline{d}=\mathrm {sup}_{t\ge t_{0}}\{\Vert \varvec{d}(t)\Vert \}$, and $\overline{m}=\mathrm {sup}_{t\ge t_{0}}\{\Vert \varvec{m}(t)\Vert \}$, in which $\overline{A}$, $\overline{B}$, $\overline{d}$ and $\overline{m}$ are known positive constants. Then, when $\mu \in (0,1)$, the drive system and response system can realize finite-time synchronization within T. When $\mu \ge 1$, the asymptotic synchronization would be reached between the two systems.

Proof

We divide this theorem into two processes to prove, that is, before (i.e., $\varvec{s}(t)\not \equiv \varvec{0}$) and after (i.e., $\varvec{s}(t)\equiv \varvec{0}$) sliding mode surface entering steady state. Above all, the first process is proved as follows. We choose the same Lyapunov function candidate (i.e., $\breve{V}_{1}(t)=V(t)$) with proof of Theorem 1. Under the conditions (i), (ii) and (iii), based on proof process of the previous theorem, it is easy to obtain

$$\begin{aligned} \dot{\breve{V}}_{1}(t)&\le -\varvec{s}^{T}(t)\varvec{C}\mathrm {sign}(\varvec{s}(t))-\sigma (\Vert \widetilde{\varvec{A}}(t-\tau )\Vert \nonumber \\&\quad +\overline{A}+\Vert \widetilde{\varvec{B}}(t)\Vert +\overline{B}+\sqrt{2\sigma _{1}}\Vert \widetilde{\varvec{d}}(t)\Vert \nonumber \\&\quad +\sqrt{2\sigma _{1}}\overline{d}+\sqrt{2\sigma _{2}}\Vert \widetilde{\varvec{m}}(t)\Vert +\sqrt{2\sigma _{2}}\overline{m})\nonumber \\&\le -c_{\text {min}}\sum \limits _{i=1}^{n+1}|s_{i}(t)|-\sigma (\Vert \widetilde{\varvec{A}}(t-\tau )\Vert \nonumber \\&\quad +\overline{A}+\Vert \widetilde{\varvec{B}}(t)\Vert +\overline{B}+\sqrt{2\sigma _{1}}\Vert \widetilde{\varvec{d}}(t)\Vert \nonumber \\&\quad +\sqrt{2\sigma _{1}}\overline{d}+\sqrt{2\sigma _{2}}\Vert \widetilde{\varvec{m}}(t)\Vert +\sqrt{2\sigma _{2}}\overline{m})\nonumber \\&\le -\sigma \left( \sum \limits _{i=1}^{n+1}|s_{i}(t)|+\Vert \widetilde{\varvec{A}}(t-\tau )\Vert \right. \nonumber \\&\quad +\overline{A}+\Vert \widetilde{\varvec{B}}(t)\Vert +\overline{B}+\sqrt{2\sigma _{1}}\Vert \widetilde{\varvec{d}}(t)\Vert \nonumber \\&\quad \left. +\sqrt{2\sigma _{1}}\overline{d}+\sqrt{2\sigma _{2}}\Vert \widetilde{\varvec{m}}(t)\Vert +\sqrt{2\sigma _{2}}\overline{m}\right) \nonumber \\ \end{aligned}$$

(57)

According to property of the norm, we know that $\Vert \widetilde{\varvec{A}}(t-\tau )-\varvec{A}(t-\tau )\Vert \le \Vert \widetilde{\varvec{A}}(t-\tau )\Vert +\Vert \varvec{A}(t-\tau )\Vert \le \Vert \widetilde{\varvec{A}}(t-\tau )\Vert +\overline{A}$. Therefore, $-(\Vert \widetilde{\varvec{A}}(t-\tau )\Vert +\overline{A})\le -(\Vert \widetilde{\varvec{A}}(t-\tau )-\varvec{A}(t-\tau )\Vert )=-\Vert \widehat{\varvec{A}}(t-\tau )\Vert$. Similarly, we can obtain that $-(\Vert \widetilde{\varvec{B}}(t)\Vert +\overline{B})\le -\Vert \widehat{\varvec{B}}(t)\Vert$, $-(\Vert \widetilde{\varvec{d}}(t)\Vert +\overline{d})\le -\Vert \widehat{\varvec{d}}(t)\Vert$ and $-(\Vert \widetilde{\varvec{m}}(t)\Vert +\overline{m})\le -\Vert \widehat{\varvec{m}}(t)\Vert$. Furthermore, in combination with Lemma 2, (57) is turned into

$$\begin{aligned} \dot{\breve{V}}_{1}(t)&\le -\sigma \left( \sum \limits _{i=1}^{n+1}|s_{i}(t)|+\Vert \widehat{\varvec{A}}(t-\tau )\Vert +\Vert \widehat{\varvec{B}}(t)\Vert \right. \nonumber \\&\quad \left. +\sqrt{2\sigma _{1}}\Vert \widehat{\varvec{d}}(t)\Vert +\sqrt{2\sigma _{2}}\Vert \widehat{\varvec{m}}(t)\Vert \right) \nonumber \\&\le -\frac{\sigma }{n} [\varvec{s}^{T}(t)\varvec{s}(t)+\widehat{\varvec{A}}^{T}(t-\tau )\widehat{\varvec{A}}(t-\tau )+\widehat{\varvec{B}}^{T}(t)\widehat{\varvec{B}}(t)\nonumber \\&\quad +2\sigma _{1}\widehat{\varvec{d}}^{T}(t)\widehat{\varvec{d}}(t)+2\sigma _{2}\widehat{\varvec{m}}^{T}(t)\widehat{\varvec{m}}(t)]^{1/2} \end{aligned}$$

(58)

We select $\sigma _{1}=\mathrm {max}\{\lambda (\varvec{U})\}$ and $\sigma _{2}=\mathrm {max}\{\lambda (\varvec{V})\}$, where $\lambda (\cdot )$ represents all the eigenvalues of the matrix. On the basis of (58), one has

$$\begin{aligned} \dot{\breve{V}}_{1}(t)&\le -\frac{\sqrt{2}\sigma }{n} [\varvec{s}^{T}(t)\varvec{P}\varvec{s}(t)+\widehat{\varvec{A}}^{T}(t-\tau )\varvec{Q}\widehat{\varvec{A}}(t-\tau )\nonumber \\&\quad +\widehat{\varvec{B}}^{T}(t)\varvec{R}\widehat{\varvec{B}}(t)+\widehat{\varvec{d}}^{T}(t)\varvec{U}\widehat{\varvec{d}}(t)+\widehat{\varvec{m}}^{T}(t)\varvec{V}\widehat{\varvec{m}}(t)]^{1/2}\nonumber \\&=-\frac{\sqrt{2}\sigma }{n}\sqrt{\breve{V}_{1}(t)} \end{aligned}$$

(59)

In the above, $\varvec{P}$, $\varvec{Q}$, $\varvec{R}$, $\varvec{U}$ and $\varvec{V}$ are the same as that in the proof about Theorem 1. Combined with Lemma 2 of [29], the states of the system would move toward sliding mode surface and converge to $\varvec{s}(t)\equiv \varvec{0}$ in a finite time $T_{1}$, which is given by

$$\begin{aligned} T_{1}=t_{0}+\frac{\sqrt{2}n}{\sigma }\sqrt{\breve{V}_{1}(t_{0})} \end{aligned}$$

(60)

Obviously, for $\mu >0$, the first process can be reached within $T_{1}$. Next, we prove the second process. Since $\varvec{s}(t)\equiv \varvec{0}$, it is known that $\dot{\varvec{s}}(t)\equiv \varvec{0}$, and thus, we obtain

$$\begin{aligned} \dot{\varvec{e}}(t)=\varvec{K}\Delta (\mathrm {sign}(\varvec{e}(t)),|\varvec{e}(t)|^{\mu }) \end{aligned}$$

(61)

For the same $\varvec{P}$ as (59), the Lyapunov function candidate is chosen as $\breve{V}_{2}(t)=\varvec{e}^{T}(t)\varvec{P}\varvec{e}(t)$, then it can be obtained

$$\begin{aligned} \dot{\breve{V}}_{2}(t)&=\varvec{e}^{T}(t)\dot{\varvec{e}}(t)\nonumber \\&=\sum \limits _{i=1}^{n+1}k_{i}|e_{i}|^{\mu +1}\nonumber \\&\le k_{\text {max}}\left( \frac{\sqrt{2}}{n}\right) ^ {\mu +1}\bigl (\sum \limits _{i=1}^{n+1}\frac{1}{2}e_{i}^{2}\bigr )^{\frac{\mu +1}{2}}\nonumber \\&=k_{\text {max}}\left( \frac{\sqrt{2}}{n}\right) ^ {\mu +1}\left( \breve{V}_{2}(t)\right) ^{\frac{\mu +1}{2}}\nonumber \\ \end{aligned}$$

(62)

where $k_{\text {max}}=\mathrm {max}\{\lambda (\varvec{K})\}<0$. Through Lemma 2 in [29], if $\mu \in (0,1)$, the state of the error system can reach the equilibrium point $\varvec{e}_{0}=\varvec{0}$ in a finite time $T_{2}$, which is decided by

$$\begin{aligned} T_{2}=T_{1}+\frac{2}{k_{\text {max}}(\mu -1)}\bigl (\frac{n}{\sqrt{2}}\bigr )^{\mu +1}\bigl (\breve{V}_{2}(T_{1})\bigr )^{\frac{1-\mu }{2}} \end{aligned}$$

(63)

Visibly, when $\mu \in (0,1)$, the drive system and response system can achieve finite-time synchronization within $T=T_{2}$. When $\mu \ge 1$, the second process may not be implemented in a finite time, which cannot make sure that the synchronization is a finite-time one, but must be an asymptotic one. To sum up, Theorem 4 is proved completely, which also confirms the rationality of Remark 7. $\square$