Regenerative and frictional chatter in plunge grinding

Abstract

This paper studies self-excited vibrations, regenerative and frictional chatters, in a plunge grinding process. In consideration of lateral and torsional workpiece movements, a dynamic model with four degrees of freedom is proposed, which involves state-dependent time delays and Stribeck effect for regenerative and frictional effects, respectively. With this model, by linearising contact angle, regenerative grinding depth, frictional velocity and the state-dependent delays, eigenvalue analysis yields stability diagrams for the grinding, where boundaries for both regenerative and frictional instabilities are determined. Then, near the boundaries, simulations and bifurcation analyses are performed to reveal patterns of chatter onset. Bifurcation diagrams show coexistence of stable grinding and the regenerative chatter near the regenerative boundary, and a sudden switch between the stable and the frictionally unstable on the frictional boundary. Moreover, simulation results also show various dynamical properties in the grinding chatters, such as effect of losing contact and stick–slip motion.

Appendices

Appendix 1: Coefficient of the discrete model

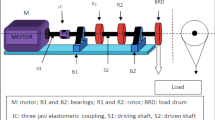
In Fig. 1, the workpiece is spatial–temporal continuum. Thus, kinetic energy of the grinding system is

$$\begin{aligned}&T=\int _{0}^{L}{\frac{1}{2}\rho \pi r_\text {w}^2\left( \left( \frac{\text {d}X_\text {w}(t,S)}{\text {d}t}\right) ^2 +\left( \frac{\text {d}Y_\text {w}(t,S)}{\text {d}t}\right) ^2\right) \text {d}S}\nonumber \\&\quad +\,\frac{1}{2}\rho \pi (r_p^2-r_\text {w}^2)\left( \left( \frac{\text {d}X_\text {w}(t,P)}{\text {d}t}\right) ^2 +\left( \frac{\text {d}Y_\text {w}(t,P)}{\text {d}t}\right) ^2\right) W\nonumber \\&\quad +\,\int _{0}^L{\frac{1}{4}\rho \pi r_\text {w}^4 \left( \frac{\text {d}\phi _\text {w}(t,S)}{\text {d}t}\right) ^2\text {d}S}\nonumber \\&\quad +\,\frac{1}{4}\rho \pi \left( r_p^4-r_\text {w}^4\right) \left( \frac{\text {d}\phi _\text {w}(t,P)}{\text {d}t}\right) ^2 W\nonumber \\&\quad +\,\frac{1}{2}m_\text {g}\left( \frac{\text {d}X_\text {g}(t)}{\text {d}t}\right) ^2. \end{aligned}$$

(41)

In Eq. (41), the first and the second terms are translational kinetic energy of the workpiece and the disc, while the third and the forth terms represent rotational kinetic energy of the workpiece and the disc, respectively. The last term is the translational kinetic energy of the wheel. In consideration of linear elasticity of the workpiece, potential energy of the grinding is given by

$$\begin{aligned} V= & {} \frac{1}{2}\int _0^L\bigg (G I_o \left( \frac{\text {d}^2\phi _\text {w}(t,S)}{\text {d}S^2}\right) ^2+E I_x\left( \frac{\text {d}^2X_\text {w}(t,S)}{\text {d}S^2}\right) ^2\nonumber \\&+\,E I_y\left( \frac{\text {d}^2Y_\text {w}(t,S)}{\text {d}S^2}\right) ^2\bigg )\text {d}S +\frac{1}{2}k_\text {g}\left( \frac{\text {d}X_\text {g}(t)}{\text {d}t}\right) ^2,\nonumber \\ \end{aligned}$$

(42)

where G and E are the shear and Young’s modulus of the workpiece material and $I_o=\pi r_\text {w}^4/2$, $I_x=\pi r_\text {w}^4/4$ and $I_y=\pi r_\text {w}^4/4$ are the area moment of inertia of cross section of the workpiece. Given inherent material damping and interfacial damping of the grinding, the dissipation function is obtained as [14]

$$\begin{aligned} D= & {} \frac{1}{2}\left( c_\text {w}\left( \frac{\text {d}X_\text {p}}{\text {d}t}\right) ^2 +c_\text {w}\left( \frac{\text {d}Y_\text {p}}{\text {d}t}\right) ^2\right. \nonumber \\&\left. +\,c_\text {t}\left( \frac{\text {d}\phi _\text {p}}{\text {d}t}\right) ^2 +c_\text {g}\left( \frac{\text {d}X_\text {g}}{\text {d}t}\right) ^2\right) , \end{aligned}$$

(43)

where $c_\text {w}$ and $c_\text {t}$ are the equivalent viscous damping coefficients with respect to the bending and torsional motion of the workpiece and $c_\text {g}$ is the damping coefficient of the wheel.

To discretize the dynamic model of the grinding, the bending displacement field of the workpiece in the x direction can be defined as

$$\begin{aligned}&X_\text {w}(t,S)\nonumber \\&\quad ={\left\{ \begin{array}{ll} a_{x0}(t)+a_{x1}(t)S+a_{x2}(t)S^2+a_{x3}(t)S^3, &{} \text {if }0\le S \le P,\\ b_{x0}(t)+b_{x1}(t)S+b_{x2}(t)S^2+b_{x3}(t)S^3, &{} \text {if }P\le S \le L, \end{array}\right. }\nonumber \\ \end{aligned}$$

(44)

where $a_{xi}$ and $b_{xi}$ ($i=0,1,2,3$) are functions of time. Corresponding to the model presented in Fig. 1, the boundary conditions with respect to $X_\text {w}(t,S)$ are

$$\begin{aligned} {\left\{ \begin{array}{ll} X_\text {w}(t,0)=0,\\ \frac{\text {d}X_\text {w}(t,0)}{\text {d}S}=0,\\ X_\text {w}(t,S)\mid _{S\rightarrow P^-}=X_p(t),\\ X_\text {w}(t,S)\mid _{S\rightarrow P^+}=X_p(t),\\ \frac{\text {d}X_\text {w}(t,S)}{\text {d}S}\mid _{S\rightarrow P^-}=\frac{\text {d}X_\text {w}(t,S)}{\text {d}S}\mid _{S\rightarrow P^+},\\ \frac{\text {d}^2X_\text {w}(t,S)}{\text {d}S^2}\mid _{S\rightarrow P^-}=\frac{\text {d}^2X_\text {w}(t,S)}{\text {d}S^2}\mid _{S\rightarrow P^+},\\ X_\text {w}(t,L)=0,\\ \frac{\text {d}^2X_\text {w}(t,0)}{\text {d}S^2}=0, \end{array}\right. } \end{aligned}$$

(45)

where $X_\text {p}(t)=X_\text {w}(t,P)$ represents the horizontal displacement of the disc. Considering Eq. (44), solving Eq. (45) yields

$$\begin{aligned}&X_\text {w}(t,S)\nonumber \\&\quad ={\left\{ \begin{array}{ll} \frac{3L(2L-P)PS^2+(P^2-2LP-2L^2)S^3}{P^3(4L-P)(L-P)}X_\text {p}(t),&{}\text {if }0\le S \le P,\\ \frac{-2L^3P+6L^3S+3L(P-3L)S^2+(3L-P)S^3}{(L-P)^2P(4L-P)}X_\text {p}(t),&{}\text {if }P\le S \le L. \end{array}\right. }\nonumber \\ \end{aligned}$$

(46)

Symmetrically, the vertical bending deformation of the workpiece is given by

$$\begin{aligned}&Y_\text {w}(t,S)\nonumber \\&\quad ={\left\{ \begin{array}{ll} \frac{3L(2L-P)PS^2+(P^2-2LP-2L^2)S^3}{P^3(4L-P)(L-P)}Y_\text {p}(t),&{}\text {if }0\le S \le P,\\ \frac{-2L^3P+6L^3S+3L(P-3L)S^2+(3L-P)S^3}{(L-P)^2P(4L-P)}Y_\text {p}(t),&{}\text {if }P\le S \le L, \end{array}\right. }\nonumber \\ \end{aligned}$$

(47)

where $Y_\text {p}(t)=Y_\text {w}(t,P)$. Similarly, the torsional displacement field $\phi (t,S)$ of the workpiece can be defined as

$$\begin{aligned} \phi _\text {w}(t,S)= {\left\{ \begin{array}{ll} a_{\phi 0}(t)+a_{\phi 1}(t)S,&{}\text {if }0\le S \le P,\\ b_{\phi 0}(t)+b_{\phi 1}(t)S,&{}\text {if }P\le S \le L, \end{array}\right. } \end{aligned}$$

(48)

where coefficients $a_{\phi i}$ and $b_{\phi i}$ ($i=0,1$) are functions of time. Its boundary conditions are

$$\begin{aligned} {\left\{ \begin{array}{ll} \phi (t,0)=\varOmega _\text {w}t,\\ \phi (t,S)\mid _{S\rightarrow P^-}=\phi _\text {p}(t),\\ \phi (t,S)\mid _{S\rightarrow P^+}=\phi _\text {p}(t),\\ \frac{\text {d}\phi (t,S)}{\text {d}S}\mid _{S\rightarrow P^-}=\frac{\text {d}\phi (t,S)}{\text {d}S}\mid _{S\rightarrow P^+}. \end{array}\right. } \end{aligned}$$

(49)

Solving Eqs. (48) and (49) yields

$$\begin{aligned} \phi _\text {w}(t,S)=t\varOmega _\text {w}+\frac{S(\phi _\text {p}(t)-\varOmega _\text {w}t)}{P}, (0\le S \le L).\nonumber \\ \end{aligned}$$

(50)

Next, to obtain the governing equation of the grinding process, one can use Lagrange’s equations

$$\begin{aligned} \frac{\text {d}}{\text {d}t}\frac{\partial L}{\partial (\text {d}q_i/\text {d}t)}-\frac{\partial L}{\partial q_i}+\frac{\partial D}{\partial (\text {d}q_i/\text {d}t)}=Q_{q_i}, \end{aligned}$$

(51)

where the Lagrange L is $L=T-V$, $q_i$ represents generalised coordinates $X_\text {g}(t)$, $X_\text {p}(t)$, $Y_\text {p}(t)$ and $\phi _\text {p}(t)$ and $Q_{q_i}$ stands for generalised force with respect to $q_i$, respectively. Then, substituting Eqs. (41), (42), (43), (46), (47) and (50) into Eq. (51) yields Eq. (2), where the coefficients are given as follows:

$$\begin{aligned}&k_\text {w}=\frac{12 E I_x L^3}{(L-P)^2(4L-P)P^3},\nonumber \\&k_\text {t}=\frac{G I_o L}{P^2},\nonumber \\&m_\text {w}=\frac{70\pi \rho P^2 W (R_\text {p}^2-R_\text {w}^2)(4L^2-5LP+P^2)^2}{35(L-P)^2P^2(4L-P)^2}\nonumber \\&\quad +\,\frac{\pi \rho L^3 R_\text {w}^2 (24L^4\!-\!24L^3P\!-\!4L^2P^2\!+\!8LP^3\!-\!P^4)}{35(L\!-\!P)^2P^2(4L\!-\!P)^2}, \nonumber \\&J_\text {t}=\frac{\pi \rho \left( 6P^2WR_\text {p}^4+(L^3-6P^2W)R_\text {w}^4\right) }{6P^2}. \end{aligned}$$

(52)

Appendix 2: Coefficient matrices

In Eq. (33), the matrices $\mathbf {M}$, $\mathbf {C}$, $\mathbf {K}$ and $\mathbf {A}$ are

$$\begin{aligned} \mathbf {M}&=\begin{pmatrix} 1&{} 0&{} 0&{} 0\\ 0&{} 1&{} 0&{} 0\\ 0&{} 0&{} 1&{} 0\\ 0&{} 0&{} 0&{} 1 \end{pmatrix},\quad \mathbf {C}=\begin{pmatrix} \xi _\text {g}&{} 0&{} 0&{} 0\\ 0&{} \xi _\text {w}&{} 0&{} 0\\ 0&{} 0&{} \xi _\text {w}&{} 0\\ 0&{} 0&{} 0&{} \xi _\text {t} \end{pmatrix}, \nonumber \\ \mathbf {K}&=\begin{pmatrix} \kappa _\text {g}&{} 0&{} 0&{} 0\\ 0&{} \kappa _\text {w}&{} 0&{} 0\\ 0&{} 0&{} \kappa _\text {w}&{} 0\\ 0&{} 0&{} 0&{} \kappa _\text {t} \end{pmatrix}, \quad \mathbf {A}=\left( a_{ij}\right) _{4\times 5}, \end{aligned}$$

(53)

where

$$\begin{aligned} a_{11}= & {} d_\text {g0}^\mu k_\mu \left( \frac{\tau _\text {g0}}{\tau _\text {w0}}\right) ^{2\mu -1} \left( -\sin (\gamma _0)+k_\text {f0}\cos (\gamma _0)\right) ,\nonumber \\ a_{12}= & {} d_\text {g0}^\mu k_\mu \left( \frac{\tau _\text {g0}}{\tau _\text {w0}}\right) ^{2\mu -1}\mu \left( \cos (\gamma _0)+k_\text {f0}\sin (\gamma _0) \right) ,\nonumber \\ a_{13}= & {} d_\text {g0}^\mu k_\mu \left( \frac{\tau _\text {g0}}{\tau _\text {w0}}\right) ^{2\mu -1}\sin (\gamma _0),\nonumber \\ a_{14}= & {} d_\text {g0}^\mu k_\mu \left( \frac{\tau _\text {g0}}{\tau _\text {w0}}\right) ^{2\mu -1}\frac{\tau _\text {w0}}{2\pi }(2\mu -1) \left( \cos (\gamma _0)\right. \nonumber \\&\left. +\,k_\text {f0}\sin (\gamma _0)\right) ,\nonumber \\ a_{15}= & {} d_\text {g0}^\mu k_\mu \left( \frac{\tau _\text {g0}}{\tau _\text {w0}}\right) ^{2\mu -1}\frac{\tau _\text {w0}+\tau _\text {g0}}{2\pi }(1-2\mu )\left( \cos (\gamma _0)\right. \nonumber \\&\left. +\,k_\text {f0}\sin (\gamma _0) \right) ,\nonumber \\ a_{21}= & {} -\gamma _\text {w}a_{11},\quad a_{22}=-\gamma _\text {w}a_{12},\quad a_{23}=-\gamma _\text {w}a_{13},\nonumber \\ a_{24}= & {} -\gamma _\text {w}a_{14},\quad a_{25}=-\gamma _\text {w}a_{15},\nonumber \\ a_{31}= & {} d_\text {g0}^\mu k_\mu \left( \frac{\tau _\text {g0}}{\tau _\text {w0}}\right) ^{2\mu -1} \gamma _\text {w}\left( \cos (\gamma _0)+\,k_\text {f0}\sin (\gamma _0)\right) ,\nonumber \\ a_{32}= & {} d_\text {g0}^{\mu -1} k_\mu \left( \frac{\tau _\text {g0}}{\tau _\text {w0}}\right) ^{2\mu -1}\nonumber \\&\quad \mu \gamma _\text {w}\left( \sin (\gamma _0)\!-\!k_\text {f0}\cos (\gamma _0) \right) ,\nonumber \\ a_{33}= & {} -d_\text {g0}^\mu k_\mu \left( \frac{\tau _\text {g0}}{\tau _\text {w0}}\right) ^{2\mu -1}\gamma _\text {w}\cos (\gamma _0), \nonumber \\ a_{34}= & {} d_\text {g0}^\mu k_\mu \left( \frac{\tau _\text {g0}}{\tau _\text {w0}}\right) ^{2\mu -1}\gamma _\text {w}\frac{\tau _\text {w0}}{2\pi }(2\mu -1)\left( \sin (\gamma _0)\right. \nonumber \\&\left. -\,k_\text {f0}\cos (\gamma _0)\right) ,\nonumber \\ a_{35}= & {} d_\text {g0}^\mu k_\mu \left( \frac{\tau _\text {g0}}{\tau _\text {w0}}\right) ^{2\mu -1}\nonumber \\&\times \gamma _\text {w}\frac{\tau _\text {w0}+\tau _\text {g0}}{2\pi }(1-2\mu )\left( \sin (\gamma _0)\right. \nonumber \\&\left. -\,k_\text {f0}\cos (\gamma _0)\right) ,\nonumber \\ a_{41}= & {} 0,\quad a_{42}=\mu d_\text {g0}^{\mu -1} k_\text {f0}\kappa _\mu \left( \frac{\tau _\text {g0}}{\tau _\text {w0}}\right) ^{2\mu -1}\gamma _\text {t},\nonumber \\ a_{43}= & {} d_\text {g0}^{\mu }\kappa _\mu \left( \frac{\tau _\text {g0}}{\tau _\text {w0}}\right) ^{2\mu -1}\gamma _\text {t}, \nonumber \\ a_{44}= & {} {a_{43}(2\mu -1)k_\text {f0}\frac{\tau _\text {w0}}{2\pi }},\nonumber \\ a_{45}= & {} {a_{43}(1-2\mu )k_\text {f0}\frac{\tau _\text {w0}+\tau _\text {g0}}{2\pi }}. \end{aligned}$$

(54)

The matrix, $\mathbf {D}$, introduced in Eq. (34) is

$$\begin{aligned} \mathbf {D}=\begin{pmatrix} d_{11}&{} d_{12}&{} d_{13}&{} 0\\ d_{21}&{} d_{22}&{} d_{23}&{} 0\\ 0&{} 0&{} 0&{} 0\\ 0&{} 0&{} 0&{} 0\\ 0&{} 0&{} 0&{} 0 \end{pmatrix} \end{aligned}$$

(55)

where

$$\begin{aligned}&d_{11}=\frac{-y_\text {p0}}{(1-\nu +x_\text {g0}-x_\text {p0})^2+y_\text {p0}^2}, \quad d_{12}=-d_{11},\\&d_{13}=\frac{1-\nu +x_\text {g0}-x_\text {p0}}{(1-\nu +x_\text {g0} -x_\text {p0})^2+y_\text {p0}^2},\\&d_{21}=\frac{-(1\!-\!\nu \!+\!x_\text {g0}\!-\!x_\text {p0})^3\!-\!(-\nu \!+\!x_\text {g0} \!-\!x_\text {p0})y_\text {p0}^2}{\left( (1-\nu +x_\text {g0} -x_\text {p0})^2+y_\text {p0}^2 \right) ^{\frac{3}{2}}},\\&d_{22}=-d_{21}, \end{aligned}$$

$$\begin{aligned} d_{23}=-y_\text {p0}\frac{(\nu -1)(\nu -2)+(x_\text {p0}-x_\text {g0})^2+y_\text {p0}^2+(2\nu -3)(x_\text {p0} -x_\text {g0})}{\left( (1-\nu +x_\text {g0} -x_\text {p0})^2+y_\text {p0}^2 \right) ^{\frac{3}{2}}}.\nonumber \\ \end{aligned}$$

(56)

$\mathbf {D_\text {w}}$ is

$$\begin{aligned} D_\text {w}=\begin{pmatrix} 0&{} 0&{} 0&{} 0\\ w_{21}&{} w_{22}&{} w_{23}&{} 0\\ 0&{} 0&{} 0&{} 0\\ 0&{} 0&{} 0&{} 0\\ 0&{} 0&{} 0&{} 0 \end{pmatrix}, \end{aligned}$$

(57)

where

$$\begin{aligned} w_{21}= & {} \frac{(1-\nu +x_\text {g0}-x_\text {p0})^3+(x_\text {g0}-x_\text {p0})y_\text {p0}^2}{\left( (1-\nu +x_\text {g0} -x_\text {p0})^2+y_\text {p0}^2\right) ^\frac{3}{2}},\\ w_{22}= & {} -w_{21}, \end{aligned}$$

$$\begin{aligned} w_{23}=\frac{(1-\nu +x_\text {g0}-x_\text {p0})(2-2\nu +x_\text {g0}-x_\text {p0})y_\text {p0}+y_\text {p0}^3}{\left( (1-\nu +x_\text {g0} -x_\text {p0})^2+y_\text {p0}^2\right) ^\frac{3}{2}}.\nonumber \\ \end{aligned}$$

(58)

$\mathbf {D_\text {g}}$ is

$$\begin{aligned} \mathbf {D_\text {g}}=\begin{pmatrix} 0&{} 0&{} 0&{} 0\\ g_{21}&{} g_{22}&{} g_{23}&{} 0\\ 0&{} 0&{} 0&{} 0\\ 0&{} 0&{} 0&{} 0\\ 0&{} 0&{} 0&{} 0 \end{pmatrix}, \end{aligned}$$

(59)

where

$$\begin{aligned} \begin{aligned} g_{21}&=g w_{21},\quad g_{22}=g w_{22}, \quad g_{23}=g w_{23}. \end{aligned} \end{aligned}$$

(60)

$\mathbf {D_\text {v}}$ is

$$\begin{aligned} \mathbf {D_\text {v}}=\begin{pmatrix} 0&{} 0&{} 0&{} 0\\ 0&{} 0&{} 0&{} 0\\ v_{31}&{} v_{32}&{} v_{33}&{} v_{34}\\ 0&{} 0&{} 0&{} v_{44}\\ v_{51}&{} v_{52}&{} v_{53}&{} 0\\ \end{pmatrix}, \end{aligned}$$

(61)

where

$$\begin{aligned} v_{31}= & {} \frac{(k_\text {s}-k_\text {d}) \exp \left( \frac{2\pi }{v_\text {s}}\left( \frac{r_\text {p}}{\tau _\text {w0}}-\frac{r_\text {g}}{\tau _\text {g0}}\right) \right) y_\text {p0}}{ v_\text {s}\sqrt{(1-\nu +x_\text {g0}-x_\text {p0})^2+y_\text {p0}^2}},\\ v_{32}= & {} -v_{31}, \end{aligned}$$

$$\begin{aligned} v_{33}= & {} \frac{(k_\text {s}-k_\text {d}) \exp \left( \frac{2\pi }{v_\text {s}}\left( \frac{r_\text {p}}{\tau _\text {w0}}-\frac{r_\text {g}}{\tau _\text {g0}}\right) \right) (1-\nu +x_\text {g0}-x_\text {p0})}{ v_\text {s}\sqrt{(1-\nu +x_\text {g0}-x_\text {p0})^2+y_\text {p0}^2}}, \end{aligned}$$

$$\begin{aligned} v_{34}= & {} \frac{(k_\text {s}-k_\text {d}) \exp \left( \frac{2\pi }{v_\text {s}}\left( \frac{r_\text {p}}{\tau _\text {w0}}-\frac{r_\text {g}}{\tau _\text {g0}}\right) \right) r_\text {p}}{v_\text {s}},\quad v_{44}=1\nonumber \\ v_{51}= & {} -\frac{y_\text {p0}}{(1-\nu +x_\text {g0}-x_\text {p0})^2+y_\text {p0}^2},\quad v_{52}=-v_{51},\nonumber \\ v_{53}= & {} \frac{1-\nu +x_\text {g0}-x_\text {p0}}{(1-\nu +x_\text {g0}-x_\text {p0})^2+y_\text {p0}^2}. \end{aligned}$$

(62)

Appendix 3: Continuation

The continuation scheme is illustrated in Fig. 17. As shown, to start the continuation algorithm, two adjacent solutions (the ith and the $i+1$st solutions) are required. Then, the initial guess for the third one (the $i+2$nd) is given by using a relaxation parameter r; thereafter, the guess is corrected by Newton–Raphson iteration. If the iteration does not converge, the relaxation parameter is decreased for a new prediction of the third solution. On the contrary, the convergent solution is accepted so long as it is located in the predefined region. Lastly, the algorithm goes to next loop ($i=i+1$) if $i<$max_steps, or the loop is ended when sufficient solutions are obtained.

Fig. 17

Continuation scheme for eigenvalue calculation

Fig. 18

Extended stability diagram for Fig. 7a ($p=0.1$ and $\nu =0.006$). More specifically, regenerative and frictional stabilities for $v_\text {f0}<0$ ($\omega _\text {g}r_\text {g}<\omega _\text {g}r_\text {w}$) are investigated. In addition, Arrows V ($\tau _\text {w0}=14.2$ and $\kappa _\mu \in [1.1, 2]$) and VI ($\tau _\text {w0}=15$ and $\kappa _\mu \in [1.1, 2]$) are marked for further analysis

Appendix 4: Grinding dynamics for $v_\text {f0}<0$

Sections 3 and 4 discuss the grinding stability and the grinding dynamics for positive frictional velocity ($v_\text {f0}>0$). Besides, due to the non-smoothness in the frictional coefficient given in Eq. (14) and Fig. 6, one would be curious about the grinding dynamics for negative frictional velocity ($v_\text {f0}<0$). In that case, the frictional coefficient in Eqs. 19 and 32 is transformed into

$$\begin{aligned} k_\text {f0}=-\left( k_\text {d}+(k_\text {s}-k_\text {d})\exp \left( \frac{\omega _\text {g}r_\text {g}-\omega _\text {w}r_\text {p}}{v_s}\right) \right) , \end{aligned}$$

(63)

and

$$\begin{aligned} k_\text {f1}= & {} (k_\text {s}-k_\text {d})\exp \left( -\frac{2\pi r_\text {p}}{v_\text {s}\tau _\text {w0}}+\frac{2\pi r_\text {g}}{v_\text {s}\tau _\text {g0}}\right) \nonumber \\&\times \left( \frac{y_\text {p0}\frac{\text {d}x_\text {g}}{\text {d}\tau }-y_\text {p0}\frac{\text {d}x_\text {p}}{\text {d}\tau } +(1-\nu +x_\text {g0}-x_\text {p0})\frac{\text {d} y_\text {p}}{\text {d}\tau }}{v_\text {s}\sqrt{(1-\nu +x_\text {g0}-x_\text {p0})^2+y_\text {p0}^2}}\right. \nonumber \\&\left. +\frac{r_\text {p}\frac{\text {d}\theta _\text {p}}{\text {d}\tau }}{v_\text {s}}\right) . \end{aligned}$$

(64)

Before the analysis, it should be remarked that this is only a mathematical problem, because a high rotary speed for the wheel ($\omega _\text {g}r_\text {g}>\omega _\text {w}r_\text {p}$) is always required for cutting the workpiece. However, this discussion is still valuable for us to understand the relationship between our model and a real grinding process in application.

As an example, Fig. 18 extends Fig. 7a to $\tau _\text {w0}=10$. It is seen that the stability boundaries are discontinuous at $\tau _\text {w0}=20$, where $k_\text {f0}$ is non-smooth for zero frictional velocity. Moreover, Fig. 18 illustrates another difference between the regenerative and the frictional instabilities: the regenerative boundaries move downwards uniformly for the decrease of $\tau _\text {w0}$, but the frictional boundaries reach the lowest point near $\tau _\text {w0}=20$, where the Stribeck effect is more significant than any other places. Next, to represent the grinding dynamics for $v_\text {f0}<0$, Arrows V ($\tau _\text {w0}=14.2$ and $\kappa _\mu \in [1.1, 2]$) and VI ($\tau _\text {w0}=15$ and $\kappa _\mu \in [1.1, 2]$) are marked for further bifurcation analysis.

For $\kappa _\mu$ increasing along Arrow V, a regenerative boundary is crossed before it reaches a frictional one. Therefore, the corresponding bifurcation pattern in Fig. 19 is similar to that along Arrow I, which is depicted in Fig. 12. However, the bifurcation diagram for $\min (v_\text {f})$ presents some new features when the frictional chatter is incurred. Here, as shown in Fig. 19f, the frictional velocity of the chatter motion keeps negative and the corresponding $\min (v_\text {f})$ recorded in Fig. 19b cluster round two locations. One indicates the smallest velocities and located at the bottom of the bifurcation diagram, and another is due to the stick motion and sited at the top of the bifurcation diagram.

Fig. 19

Bifurcation diagrams with respect to Arrow V marked in Fig. 18. In addition, time series or phase portraits for $\kappa _\nu =1.4$ and 1.8 are plotted as well

The bifurcation pattern along Arrow VI is plotted in Fig. 20. As seen, this is similar to those in Figs. 13 and 14. As the increase of $\kappa _\mu$, the frictional chatter is firstly promoted before the regenerative chatter. Still, the frictional chatter is of negative frictional velocity, and the distribution of $min(v_\text {f})$ is split by the frictional chatter. In addition, the periodic frictional vibration plotted in Fig. 20e is twisted, so that two maximums of the grinding depth ($\max (d_\text {g})$) are recorded.

Fig. 20

Bifurcation diagrams with respect to Arrow VI marked in Fig. 18. In addition, time series or phase portraits for $\kappa _\nu =1.3$, 1.5 and 1.8 are plotted as well