Size-dependent melting of nanocrystals: a self-consistent statistical approach

Abstract

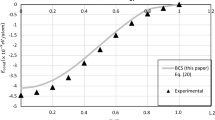
A self-consistent statistical method is used to describe size effects on melting of free nanocrystals. The melting transition is assumed to be directly related to evolution of high-temperature instability of the phonon subsystem of the crystal, caused by strong anharmonicity of atomic vibrations. We show that depression of the melting temperature of small free particles is mainly due to presence of surface atoms which are bound to smaller numbers of atoms than those of the interior. The melting temperatures of spherical nanocrystals of Ar and Au were calculated as functions of the inverse of their radii and compared with experimental and molecular dynamics data.

Appendix: Distribution functions of atoms in a simple crystal

Here, we give expressions for distribution functions of atomic displacements in a simple perfect crystal consisting of N atoms of mass M (Karasevskii and Lubashenko 2002). In the harmonic approximation, an N-particle normalized function f _N can be represented as a product of 3N independent real normal coordinates. In the coordinate representation, it is written as

$$ f_{\rm N}=C_{\rm N} \exp \left(-\sum_{nn{^\prime}\beta\beta{^\prime}} B_{nn{^\prime}}^{\beta\beta{^\prime}}q_{n\beta}q_{n{^\prime}\beta{^\prime}}\right), $$

(13)

where C _N is a normalization factor, q _nβ is the βth Cartesian coordinate of displacement ${\mathbf q}_{n}$ of atom n from its equilibrium position ${\mathbf R}_n,$ and coefficients B ^ββ′_nn′ are given by

$$ B_{nn{^\prime}}^{\beta \beta{^\prime}} = \frac{M}{N\hbar}\sum_{{\mathbf k},j} \omega_{j}({\mathbf k}) \tanh \left(\frac{\hbar\omega_{j}({\mathbf k})}{2k_{\rm B}T}\right) \times e_{j\beta}({\mathbf {k}}) e_{j\beta{^\prime}}({\mathbf k}) \cos [{\mathbf k}\cdot ({\mathbf{R}}_n-{\mathbf R}_{n{^\prime}})]. $$

(14)

Here $e_{j}({\mathbf k})$ is a polarization vector, summation over ${\mathbf k}$ is taken over the first Brillouin zone. A diagonal element of the matrix (14), B ^ββ _nn , determines vibrational amplitudes of atom n in the β direction, and off-diagonal elements (n ≠n′) characterize correlations between displacements of different atoms.

For fcc and bcc lattices, $B_{nn}^{\beta \beta{^\prime}}=\gamma \delta_{\beta \beta{^\prime}},$ where

$$ \gamma = \frac{M}{N \hbar}\sum_{{\mathbf k},j} \omega_{j}({\mathbf k}) \tanh \left(\frac{\hbar \omega_{j}({\mathbf k})}{2k_{\rm B} T}\right) e_{j \beta}^2({\mathbf k}) $$

(15)

is the inverse square of the distribution width of atomic displacements which is independent of n and β. It was also found that all the off-diagonal elements of the matrix (14) are negligibly small except for that describing correlations between longitudinal components q _nx and q_n′x of displacements of two neighbouring atoms. Introducing a dimensionless correlation parameter ζ such that B ^xx_nn′  = −γζ (0≤ζ < 1), we obtain

$$ \zeta =- \frac{M}{N \hbar \gamma}\sum_{{\mathbf k},j} \omega_{j}({\mathbf k}) \tanh \left(\frac{\hbar \omega_{j}({\mathbf k})}{2k_{\rm B}T}\right) e_{j x}^2({\mathbf k}) \cos [{\mathbf k}\cdot ({\mathbf R}_n-{\mathbf R}_{n{^\prime}})], $$

(16)

where the x axis goes through the sites ${\mathbf R}_n$ and ${\mathbf R}_{n{^\prime}}.$ Neglecting all the other off-diagonal elements, we get the N-particle distribution function of atomic coordinates in the form

$$ f_{\rm N}=C_{\rm N} \exp \left[-\gamma \sum_{n} \left(q_n^2-\zeta \sum_{n{^\prime}} q_{nx}q_{n{^\prime}x}\right)\right], $$

(17)

with subscript n′ running over the first coordination sphere of atom n.

In order to calculate the average potential energy of pairwise interatomic interaction, we need a normalized two-particle (binary) distribution function $f_2({\mathbf q}_n,{\mathbf q}_{n{^\prime}})$ of neighbouring atoms which is obtained by averaging the function f _N over coordinates of all the atoms except n and n′. The result is given by

$$ f_2({\mathbf q}_n,{\mathbf q}_{n{^\prime}})=C_2 \exp \left[-\gamma \left(g_{\rm l} \left(q_{nx}^2+q_{n{^\prime}x}^2\right)-\right.2\chi \zeta\right. q_{nx}q_{n{^\prime}x} \left.\left.+g_{\rm t} \left(q_{ny}^2+q_{n{^\prime}y}^2+q_{nz}^2+q_{n{^\prime}z}^2\right)\right)\right], $$

(18)

where factors g _l(ζ), g _t(ζ) and χ(ζ) (all positive and ≤1) determine effective reduction of parameters of the distribution function (17) due to interatomic correlations. Their functional form is defined by the lattice type. For example, for the fcc lattice, these functions appear as

$$ g_{\rm l} \approx 1-3 \zeta^2+\frac{\zeta^3}{2},\quad g_{\rm t} \approx 1-4 \zeta^2+\frac{3\zeta^3}{2},\quad\chi \approx 1- \frac{\zeta} {2}+\frac{\zeta^2}{4}. $$

The average energy of interaction of neighbouring atoms n and n′ is expressed as

$$ \langle u \rangle=\int u(r_{nn{^\prime}}) f_2({\mathbf q}_n, {\mathbf q}_{n{^\prime}}) \hbox{d}{\mathbf q}_n \hbox{d}{\mathbf q}_{n{^\prime}}, $$

(19)

where u(r _nn′) is interatomic potential. For the Morse potential (5), the integration in (19) can be done analytically, and so we obtain

$$ \frac{\langle u \rangle}{A}=\frac{\exp \left[-2 b+ \frac{q\alpha^2} {\gamma}\right]}{1+\frac{{2 \alpha}}{\gamma g_{\rm t} R}}- \frac{2 \exp \left[-b+\frac{{q\alpha^2}}{4\gamma}\right]} {1+\frac{{\alpha}}{2\gamma g_{\rm t} R}}, $$

(20)

with q(ζ) = 2/(g _l + ζχ). Since contribution of the transverse displacements into $\langle U \rangle$ (the fractions in the denominators) is negligible for both RGC and metals ($\alpha/(\gamma g_{\rm t} R) \sim 10^{-2}),$ we discard it in computation of the average potential energy and use Eq. 6.