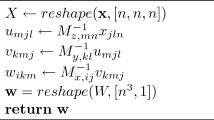Skip to main content

Advertisement

SpringerLink
Log in

A Family of Energy Stable, Skew-Symmetric Finite Difference Schemes on Collocated Grids

A Simple Way to Avoid Odd–Even Decoupling

  • Published:
Journal of Scientific Computing Aims and scope Submit manuscript

Abstract

A simple scheme for incompressible, constant density flows is presented, which avoids odd-even decoupling for the Laplacian on collocated grids. Energy stability is implied by guaranteeing strict energy conservation. Momentum is conserved. Arbitrary order in space and time can easily be obtained. These conservation properties also hold on transformed grids.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7

Similar content being viewed by others

Notes

  1. Strictly one sided first order derivatives led to a quick dissolution of the flow structures.

  2. The there depicted time is measured in the vortex turn-over time leading to a factor of four.

References

  1. Brouwer, J., Reiss, J., Sesterhenn, J.: Fully conservative finite-difference schemes of arbitrary order for compressible flow. AIP Conf. Proc. 1479(1), 2290–2293 (2012). doi:10.1063/1.4756651

    Article  Google Scholar 

  2. Brouwer, J., Reiss, J., Sesterhenn, J.: Conservative time integrators of arbitrary order for skew-symmetric finite-difference discretizations of compressible flow. Comput. Fluids 100, 1–12 (2014). doi:10.1016/j.compfluid.2014.04.019

    Article  Google Scholar 

  3. Chorin, A.: Numerical solution of the navier–stokes equations. Math. Comput. 22(104), 745–762 (1968)

    Article  MATH  Google Scholar 

  4. Dick, E., Linden, J.: A multigrid method for steady incompressible navier–stokes equations based on flux difference splitting. Int. J. Numer. Methods Fluids 14(11), 1311–1323 (1992). doi:10.1002/fld.1650141104

    Article  MATH  Google Scholar 

  5. Ellison, J.H., Hall, C.A., Porsching, T.A.: An unconditionally stable convergent finite difference method for navier–stokes problems on curved domains. SIAM J Numer. Anal. 24(6), 1233–1248 (1987)

    Article  MATH  Google Scholar 

  6. Feiereisen, W.J., Reynolds, W.C., Ferziger, J.H.: Numerical simulation of a compressible homogeneous, turbulent shear flow, nasa-cr-164953; su-tf-13. Tech. Rep. NASA-CR-164953; SU-TF-13, NASA (1981)

  7. Felten, F.N., Lund, T.S.: Kinetic energy conservation issues associated with the collocated mesh scheme for incompressible flow. J. Comput. Phys. 215(2), 465–484 (2006). doi:10.1016/j.jcp.2005.11.009

    Article  MATH  Google Scholar 

  8. Gresho, P.M., Sani, R.L.: On pressure boundary conditions for the incompressible navier–stokes equations. Int. J. Numer. Methods Fluids 7(10), 1111–1145 (1987). doi:10.1002/fld.1650071008

    Article  MATH  Google Scholar 

  9. Ham, F., Lien, F., Strong, A.: A fully conservative second-order finite difference scheme for incompressible flow on nonuniform grids. J. Comput. Phys. 177(1), 117–133 (2002). doi:10.1006/jcph.2002.7006

    Article  MATH  Google Scholar 

  10. Harlow, F.H., Welch, J.E.: Numerical calculation of time-dependent viscous incompressible flow of fluid with free surface. Phys. Fluids 8(12), 2182–2189 (1965). doi:10.1063/1.1761178

    Article  MATH  Google Scholar 

  11. J.F. Thompson Z.U.A., Warsi C.M.: Numerical Grid Generation. Elsevier, Amsterdam (1985)

  12. Keetels, G., DOrtona, U., Kramer, W., Clercx, H., Schneider, K., van Heijst, G.: Fourier spectral and wavelet solvers for the incompressible navier–stokes equations with volume-penalization: convergence of a dipolewall collision. J. Comput. Phys. 227(2), 919–945 (2007). doi:10.1016/j.jcp.2007.07.036

    Article  MATH  Google Scholar 

  13. Kevlahan, N.K.R., Farge, M.: Vorticity filaments in two-dimensional turbulence: creation, stability and effect. J. Fluid Mech. 346, 49–76 (1997). doi:10.1017/S0022112097006113

    Article  MATH  Google Scholar 

  14. Lubich, E.H.C., Wanner, G.: Geometric numerical integration: structure-preserving algorithms for ordinary differential equations, vol. 31. Springer, New York (2006)

    Google Scholar 

  15. Morinishi, Y., Lund, T., Vasilyev, O., Moin, P.: Fully conservative higher order finite difference schemes for incompressible flow. J. Comput. Phys. 143(1), 90–124 (1998). doi:10.1006/jcph.1998.5962

    Article  MATH  Google Scholar 

  16. Perot, J.B.: Discrete conservation properties of unstructured mesh schemes. Ann. Rev. Fluid Mech. 43(1), 299–318 (2011). doi:10.1146/annurev-fluid-122109-160645

    Article  Google Scholar 

  17. Reiss, J.: Energy stable, collocated high order schemes for incompressible flows on distorted grids. AIP Conf. Proc. 1479(1), 2270–2273 (2012). doi:10.1063/1.4756646

    Article  Google Scholar 

  18. Reiss, J., Sesterhenn, J.: Calculation of shocks with skew symmetric schemes. AIP Conf. Proc. 1389(1), 1894–1897 (2011). doi:10.1063/1.3636981

    Article  Google Scholar 

  19. Reiss, J., Sesterhenn, J.: A conservative, skew-symmetric finite difference scheme for the compressible navier–stokes equations. Comput. Fluids 101, 208–219 (2014). doi:10.1016/j.compfluid.2014.06.004

    Article  Google Scholar 

  20. Rempfer, D.: On boundary conditions for incompressible navier–stokes problems. Appl. Mech. Rev. 59, 107–125 (2006). doi:10.1115/1.2177683

    Article  Google Scholar 

  21. Rhie, C., Chow, W.: Numerical study of the turbulent flow past an airfoil with trailing edge separation. AIAA J. 21(11), 1525–1532 (1983)

    Article  MATH  Google Scholar 

  22. Sanderse, B.: Energy-conserving runge–kutta methods for the incompressible navier–stokes equations. J. Comput. Phys. 233, 100–131 (2013). doi:10.1016/j.jcp.2012.07.039

    Article  MATH  Google Scholar 

  23. Sani, R.L., Shen, J., Pironneau, O., Gresho, P.M.: Pressure boundary condition for the time-dependent incompressible navier–stokes equations. Int. J. Numer. Methods Fluids 50(6), 673–682 (2006). doi:10.1002/fld.1062

    Article  MATH  Google Scholar 

  24. Tadmor, E.: Skew-selfadjoint form for systems of conservation laws. J. Math. Ana. Appl. 103, 428 (1984)

    Article  MATH  Google Scholar 

  25. Thomas, P.D., Lombard, C.K.: Geometric conservation law and its application to flow computations on moving grids. AIAA J. 17, 1030–1037 (1979). doi:10.2514/3.61273

    Article  MATH  Google Scholar 

  26. Trias, F., Lehmkuhl, O., Oliva, A., Prez-Segarra, C., Verstappen, R.: Symmetry-preserving discretization of navier–stokes equations on collocated unstructured grids. J. Comput. Phys. 258, 246–267 (2014). doi:10.1016/j.jcp.2013.10.031

    Article  Google Scholar 

  27. Veldman, A.E.P., Rinzema, K.: Playing with nonuniform grids. J. Eng. Math. 26, 119–130 (1992)

    Article  MATH  Google Scholar 

  28. Verstappen, R., Veldman, A.: Symmetry-preserving discretization of turbulent flow. JCP 187(1), 343 (2003). doi:10.1016/S0021-9991(03)00126-8

    Article  MATH  Google Scholar 

  29. Visbal, M.R., Gaitonde, D.V.: On the use of higher-order finite-difference schemes on curvilinear and deforming meshes. J. Comput. Phys. 181(1), 155–185 (2002). doi:10.1006/jcph.2002.7117

    Article  MATH  Google Scholar 

  30. Wesseling, P.: Principles of computational fluid dynamics. Springer, Berlin (2001)

    Book  Google Scholar 

  31. Zhang, X., Schmidt, D., Perot, B.: Accuracy and conservation properties of a three-dimensional unstructured staggered mesh scheme for fluid dynamics. J. Comput. Phys. 175(2), 764–791 (2002). doi:10.1006/jcph.2001.6973

    Article  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. ISTA, TU-Berlin, Müller-Breslau-Str 8, 10623, Berlin, Germany

    Julius Reiss

Authors
  1. Julius Reiss
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Julius Reiss.

Additional information

This is modified or added.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Reiss, J. A Family of Energy Stable, Skew-Symmetric Finite Difference Schemes on Collocated Grids. J Sci Comput 65, 821–838 (2015). https://doi.org/10.1007/s10915-015-9985-7

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10915-015-9985-7

Keywords

Mathematics Subject Classification

Search

Navigation